Skip to main content
Springer Nature Link
Log in

A Quasi-Conservative Discontinuous Galerkin Method for Multi-component Flows Using the Non-oscillatory Kinetic Flux II: ALE Framework

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

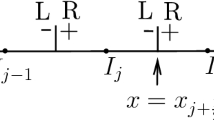
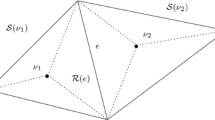
Abstract

A high-order quasi-conservative discontinuous Galerkin (DG) method is proposed for the numerical simulation of compressible multi-component flows. A distinct feature of the method is a predictor-corrector strategy to define the grid velocity. A Lagrangian mesh is first computed based on the flow velocity and then used as an initial mesh in a moving mesh method (the moving mesh partial differential equation or MMPDE method ) to improve its quality. The fluid dynamic equations are discretized in the direct arbitrary Lagrangian-Eulerian framework using DG elements and the non-oscillatory kinetic flux while the species equation is discretized using a quasi-conservative DG scheme to avoid numerical oscillations near material interfaces. A selection of one- and two-dimensional examples are presented to verify the convergence order and the constant-pressure-velocity preservation property of the method. They also demonstrate that the incorporation of the Lagrangian meshing with the MMPDE moving mesh method works well to concentrate mesh points in regions of shocks and material interfaces.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16

Similar content being viewed by others

References

  1. Abgrall, R.: How to prevent pressure oscillations in multicomponent flow calculations: A quasi conservative approach. J. Comput. Phys. 125, 150–160 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  2. Addessio, F. L., Carroll, D. E., Dukowicz, J. K., Harlow, F. H., Johnson, J. N., Kashiwa, B. A., Maltrud, M. E., Ruppel, H.: CAVEAT: A computer code for fluid dynamics problems with large distortion and internal slip, Los Alamos National Laboratory LA-10613-MSREVISED, (1990)

  3. Baines, M.J.: Moving Finite Elements. Oxford University Press, Oxford (1994)

    MATH  Google Scholar 

  4. Baines, M.J., Hubbard, M.E., Jimack, P.K.: Velocity-based moving mesh methods for nonlinear partial differential equations. Comm. Comput. Phys. 10, 509–576 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  5. Boscheri, W.: High order direct Arbitrary-Lagrangian-Eulerian (ALE) finite volume schemes for hyperbolic systems on unstructured meshes. Arch. Comput. Methods Eng. 24, 751–801 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  6. Budd, C.J., Huang, W., Russell, R.D.: Adaptivity with moving grids. Acta Numer. 18, 111–241 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  7. Chen, Y.: A study of GKS method for multi-component flows (in Chinese), Ph.D thesis, Beijing, China Academy of Engineering Physics (2010)

  8. Chen, Y., Jiang, S.: A non-oscillatory kinetic scheme for multi-component flows with the equation of state for a stiffened gas. J. Comput. Math. 29, 661–683 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  9. Chen, Y., Jiang, S.: Modified kinetic flux vector splitting schemes for compressible flows. J. Comput. Phys. 228, 3582–3604 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  10. Cheng, J., Zhang, F., Liu, T.G.: A discontinuous Galerkin method for the simulation of compressible gas-gas and gas-water two-medium flows. J. Comput. Phys. 403, 109059 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  11. Cheng, J., Shu, C.-W.: A high order ENO conservative Lagrangian type scheme for the compressible Euler equations. J. Comput. Phys. 227, 1567–1596 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  12. Cheng, J., Shu, C.-W.: Positivity-preserving Lagrangian scheme for multi-material compressible flow. J. Comput. Phys. 257, 143–168 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  13. Cockburn, B., Hou, S., Shu, C.-W.: The Runge-Kutta local projection discontinuous Galerkin finite element method for conservation laws IV: The multidimensional case. Math. Comp. 54, 545–581 (1990)

    MathSciNet  MATH  Google Scholar 

  14. Cockburn, B., Lin, S.-Y., Shu, C.-W.: TVB Runge-Kutta local projection discontinuous Galerkin finite element method for conservation laws III: One dimensional systems. J. Comput. Phys. 84, 90–113 (1989)

    Article  MathSciNet  MATH  Google Scholar 

  15. Cockburn, B., Shu, C.-W.: TVB Runge-Kutta local projection discontinuous Galerkin finite element method for conservation laws II: General framework. Math. Comp. 52, 411–435 (1989)

    MathSciNet  MATH  Google Scholar 

  16. Cockburn, B., Shu, C.-W.: The Runge-Kutta discontinuous Galerkin method for conservation laws V: Multidimensional systems. J. Comput. Phys. 141, 199–224 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  17. Farhat, C., Geuzaine, P., Granndmont, C.: The discrete geometric conservation law and the nonlinear stability of ALE schemes for the solution of flow problems on moving grids. J. Comput. Phys. 174, 669–694 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  18. Fu, P., Schnücke, G., Xia, Y.: Arbitrary Lagrangian-Eulerian discontinuous Galerkin method for conservation laws on moving simplex meshes. Math. Comp. 88, 2221–2255 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  19. Henry de Frahan, M. T., Varadan, S., Johnsen, E.: A new limiting procedure for discontinuous Galerkin methods applied to compressible multiphase flows with shocks and interfaces, J. Comput. Phys. 280, 489-509 (2015)

  20. Hirt, C.W., Amsden, A.A., Cook, J.L.: An arbitrary Lagrangian-Eulerian computing method for all flow speed. J. Comput. Phys. 14, 227–253 (1974)

    Article  MATH  Google Scholar 

  21. Huang, W., Kamenski, L.: A geometric discretization and a simple implementation for variational mesh generation and adaptation. J. Comput. Phys. 301, 322–337 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  22. Huang, W., Kamenski, L.: On the mesh nonsingularity of the moving mesh PDE method. Math. Comput. 87, 1887–1911 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  23. Huang, W., Ren, Y., Russell, R.D.: Moving mesh methods based on moving mesh partial differential equations. J. Comput. Phys. 113, 279–290 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  24. Huang, W., Ren, Y., Russell, R.D.: Moving mesh partial differential equations (MMPDEs) based upon the equidistribution principle. SIAM J. Numer. Anal. 31, 709–730 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  25. Huang, W., Russell, R.D.: Adaptive moving mesh methods. Springer-Verlag, New York (2011)

    Book  MATH  Google Scholar 

  26. Huang, W.: Mathematical principles of anisotropic mesh adaptation. Comm. Comput. Phys. 1, 276–310 (2006)

    MATH  Google Scholar 

  27. Huang, W.: Variational mesh adaptation: isotropy and equidistribution. J. Comput. Phys. 174, 903–924 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  28. Huang, W.: Variational mesh adaptation II: error estimates and monitor functions. J. Comput. Phys. 184, 619–648 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  29. Jimack, P., Wathen, A.: Temporal derivatives in the finite-element method on continuously deforming grids. SIAM J. Numer. Anal. 28, 990–1003 (1991)

    Article  MathSciNet  MATH  Google Scholar 

  30. Klingenberg, C., Schnücke, G., Xia, Y.: Arbitrary Lagrangian-Eulerian discontinuous Galerkin method for conservation laws: analysis and application in one dimension. Math. Comp. 86, 1203–1232 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  31. Kucharik, M., Shashkov, M.J.: One-step hybrid remapping algorithm for multi-material arbitrary Lagrangian-Eulerian methods. J. Comput. Phys. 231, 2851–2864 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  32. Lesoinne, M., Farhat, C.: Geometric conservation laws for flow problems with moving boundaries and deformable meshes, and their impact on aeroelastic computations. Comput. Methods Appl. Mech. Engrg. 134, 71–90 (1996)

    Article  MATH  Google Scholar 

  33. Liu, H., Xu, K.: A Runge-Kutta discontinuous Galerkin method for viscous flow equations. J. Comput. Phys. 224, 1223–1242 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  34. Liu, N., Xu, X., Chen, Y.: High-order spectral volume scheme for multi-component flows using non-oscillatory kinetic flux. Comput. Fluids 152, 120–133 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  35. Lomtev, I., Kirby, R.M., Karniadakis, G.E.: A discontinuous Galerkin ALE method for compressible viscous flows in moving domains. J. Comput. Phys. 155, 128–159 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  36. Luo, D., Huang, W., Qiu, J.X.: A quasi-Lagrangian moving mesh discontinuous Galerkin method for hyperbolic conservation laws. J. Comput. Phys. 396, 544–578 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  37. Luo, D., Qiu, J.X., Zhu, J., Chen, Y.: A quasi-conservative discontinuous Galerkin method for multi-component flows using the non-oscillatory kinetic flux. J. Sci. Comput. 87, 1–32 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  38. Maire, P.H., Breil, J., Galera, S.: A cell-centered arbitrary Lagrangian-Eulerian (ALE) method. Int. J. Numer. Meth. Fluids 56, 1161–1166 (2008)

    Article  MATH  Google Scholar 

  39. Maire, P.H., Abgrall, R., Breil, J., Ovadia, J.: A cell-centered Lagrangian scheme for two-dimensional compressible flow problems. SIAM J. Sci. Comput. 29, 1781–1824 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  40. Miller, K., Miller, R.N.: Moving finite elements I. SIAM J. Numer. Anal. 18, 1019–1032 (1981)

    Article  MathSciNet  MATH  Google Scholar 

  41. Nguyen, V.T.: An arbitrary Lagrangian-Eulerian discontinuous Galerkin method for simulations of flows over variable geometries. J. Fluids Struct. 26, 312–329 (2010)

    Article  Google Scholar 

  42. Pandare, A. K., Wang, C., Luo, H.: An arbitrary Lagrangian-Eulerian reconstructed discontinuous Galerkin method for compressible multiphase flows, 46th AIAA Fluid Dynamics Conference (2016)

  43. Persson, P.O., Bonet, J., Peraire, J.: Discontinuous Galerkin solution of the Navier-Stokes equations on deformable domains. Comput. Methods Appl. Mech. Eng. 198, 1585–1595 (2009)

    Article  MATH  Google Scholar 

  44. Qiu, J.X., Liu, T.G., Khoo, B.C.: Runge-Kutta discontinuous Galerkin methods for compressible two-medium flow simulations: one-dimensional case. J. Comput. Phys. 222, 353–373 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  45. Qiu, J.X., Liu, T.G., Khoo, B.C.: Simulations of compressible two-medium flow by Runge-Kutta discontinuous Galerkin methods with the ghost fluid method. Comm. Comput. Phys. 3, 479–504 (2008)

    MathSciNet  MATH  Google Scholar 

  46. Qiu, J.X., Shu, C.-W.: Runge-Kutta discontinuous Galerkin method using WENO limiters. SIAM J. Sci. Comput. 26, 907–929 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  47. del Razo, M.J., Leveque, R.J.: Numerical methods for interface coupling of compressible and almost incompressible media. SIAM J. Sci. Comput. 39, B486–B507 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  48. Reed, W. H., Hill, T. R.: Triangular mesh methods for neutron transport equation, Los Alamos Scientific Laboratory Report LA-UR-73-479 (1973)

  49. Ren, X., Xu, K., Shyy, W.: A multi-dimensional high-order DG-ALE method based on gas-kinetic theory with application to oscillating bodies. J. Comput. Phys. 316, 700–720 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  50. Saleem, M.R., Ali, I., Qamar, S.: Application of discontinuous Galerkin method for solving a compressible five-equation two-phase flow model. Results Phys. 8, 379–390 (2018)

    Article  Google Scholar 

  51. Shu, C.-W.: Total-variation-diminishing time discretizations. SIAM J. Sci. Stat. Comput. 9, 1073–1084 (1988)

    Article  MathSciNet  MATH  Google Scholar 

  52. Shu, C.-W., Osher, S.: Efficient implementation of essentially non-oscillatory shock capturing schemes II. J. Comput. Phys. 83, 32–78 (1989)

    Article  MathSciNet  MATH  Google Scholar 

  53. Shyue, K.M.: An efficient shock-capturing algorithm for compressible multicomponent problems. J. Comput. Phys. 142, 208–242 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  54. Shyue, K.M.: A wave-propagation based volume tracking method for compressible multicomponent flow in two space dimensions. J. Comput. Phys. 215, 219–244 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  55. Tang, T.: Moving mesh methods for computational fluid dynamics flow and transport, Recent Advances in Adaptive Computation (Hangzhou, 2004), Volume 383 of AMS Contemporary Mathematics, pages 141-173. Amer. Math. Soc., Providence, RI, (2005)

  56. Wang, C.-W., Shu, C.-W.: An interface treating technique for compressible multi-medium flow with Runge-Kutta discontinuous Galerkin method. J. Comput. Phys. 229, 8823–8843 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  57. Xu, K.: BGK-based scheme for multicomponent flow calculations. J. Comput. Phys. 134, 122–133 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  58. Xu, X., Ni, G., Jiang, S.: A high-order moving mesh kinetic scheme based on WENO reconstruction for compressible flows on unstructured meshes. J. Sci. Comput. 57, 278–299 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  59. Zhang, Z., Naga, A.: A new finite element gradient recovery method: superconvergence property. SIAM J. Sci. Comput. 26, 1192–1213 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  60. Zhao, X., Yu, X., Qiu, M., Qing, F., Zou, S.: An arbitrary Lagrangian-Eulerian RKDG method for multi-material flows on adaptive unstructured meshes. Comput. Fluids 207, 104589 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  61. Zhu, J., Qiu, J.X., Liu, T.G., Khoo, B.C.: High-order RKDG methods with WENO type limiters and conservative interfacial procedure for one-dimensional compressible multi-medium flow simulations. Appl. Numer. Math. 61, 554–580 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  62. Zhu, J., Qiu, J.X., Shu, C.-W.: High-order Runge-Kutta discontinuous Galerkin methods with a new type of multi-resolution WENO limiters. J. Comput. Phys. 404, 109105 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  63. Zhu, J., Shu, C.-W., Qiu, J.X.: High-order Runge-Kutta discontinuous Galerkin methods with a new type of multi-resolution WENO limiters on triangular meshes. Appl. Numer. Math. 153, 519–539 (2020)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institute of Applied Physics and Computational Mathematics, Beijing, 100088, China

    Dongmi Luo, Shiyi Li & Yibing Chen

  2. Department of Mathematics, University of Kansas, Lawrence, KS, 66045, USA

    Weizhang Huang

  3. School of Mathematical Sciences and Fujian Provincial Key Laboratory of Mathematical Modeling and High-Performance Scientific Computing, Xiamen University, Xiamen, 361005, Fujian, China

    Jianxian Qiu

Authors
  1. Dongmi Luo
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Shiyi Li
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Weizhang Huang
    View author publications

    You can also search for this author inPubMed Google Scholar

  4. Jianxian Qiu
    View author publications

    You can also search for this author inPubMed Google Scholar

  5. Yibing Chen
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Yibing Chen.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

The research is supported partly by National Natural Science Foundation of China (Grant Nos. 12101063, 11901044 and 12071392), Science Challenge Project (China), No. TZ2016002, National Key Project (GJXM92579)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Luo, D., Li, S., Huang, W. et al. A Quasi-Conservative Discontinuous Galerkin Method for Multi-component Flows Using the Non-oscillatory Kinetic Flux II: ALE Framework. J Sci Comput 90, 46 (2022). https://doi.org/10.1007/s10915-021-01732-4

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10915-021-01732-4

Keywords

Mathematics Subject Classification