Skip to main content
Springer Nature Link
Log in

A Conforming Discontinuous Galerkin Finite Element Method for Linear Elasticity Interface Problems

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

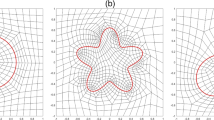
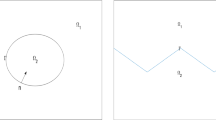
Abstract

A new conforming discontinuous Galerkin method is studied for the linear elasticity interface problems with discontinuous coefficients and displacement. This new method is based on a new definition of weak gradient operator and has no stabilizer. The weak divergence operator used in the scheme is different from the weak Galerkin finite element method, which significantly reduces the computational cost. The error estimates of optimal order in discrete \(L^2\) and \(H^1\) norms are established. Numerical examples are presented to demonstrate the efficiency and accuracy of the numerical method, and to illustrate the locking-free property.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

Data Availability

Enquiries about data availability should be directed to the authors.

References

  1. Asaro, R.J., Tiller, W.A.: Interface morphology development during stress corrosion cracking: Part I. Via surface diffusion. Metall. Mater. Trans. B 3, 1789–1796 (1972)

    Article  Google Scholar 

  2. Gong, Y., Li, Z.L.: Immersed interface finite element methods for elasticity interface problems with non-homogeneous jump conditions. Numer. Math. Theory, Methods Appl. 3(1), 23–39 (2010)

    Article  MathSciNet  Google Scholar 

  3. Grinfeld, M.A.: The stress driven instability in elastic crystals: mathematical models and physical manifestations. J. Nonlinear Sci. 3, 35–83 (1993)

    Article  MathSciNet  Google Scholar 

  4. Li, X.F., Lowengrub, J., Nie, Q., Cristini, V., Leo, P.: Microstructure evolution in three-dimensional inhomogeneous elastic media. Metall. Mater. Trans. 34A(7), 1421–1431 (2003)

    Article  Google Scholar 

  5. Mura, T.: Micromechanics of Defects in Solids. Springer, Berlin (1987)

    Book  Google Scholar 

  6. Wang, B., Xia, K.L., Wei, G.W.: Matched interface and boundary method for elasticity interface problems. J. Comput. Appl. Math. 285, 203–225 (2015)

    Article  MathSciNet  Google Scholar 

  7. Wang, C.M., Wang, J.P., Wang, R.S., Zhang, R.: A locking-free weak Galerkin finite element method for elasticity problems in the primal formulation. J. Comput. Appl. Math. 307, 346–366 (2016)

    Article  MathSciNet  Google Scholar 

  8. Wang, J.P., Wang, C.M.: Weak Galerkin finite element methods for elliptic PDEs (in Chinese). Sci. Sin. Math. 45(7), 1061–1092 (2015)

    Article  Google Scholar 

  9. Wang, J.P., Ye, X.: A weak Galerkin finite element method for second-order elliptic problems. J. Comput. Appl. Math. 241, 103–115 (2013)

    Article  MathSciNet  Google Scholar 

  10. Wang, J.P., Ye, X.: A weak Galerkin mixed finite element method for second order elliptic problems. Math. Comput. 83(289), 2101–2126 (2014)

    Article  MathSciNet  Google Scholar 

  11. Wang, Y., Gao, F.Z., Cui, J.T.: A conforming discontinuous Galerkin finite element method for elliptic interface problems. J. Comput. Appl. Math. 412, 114304 (2022)

    Article  MathSciNet  Google Scholar 

  12. Xie, H., Li, Z.L., Qiao, Z.H.: A finite element method for elasticity interface problems with locally modified triangulations. Int. J. Numer. Anal. Model. 8(2), 189–200 (2011)

    MathSciNet  MATH  Google Scholar 

  13. Yang, X.Z.: Immersed interface method for elasticity problems with interfaces. Ph.D. thesis, North Carolina State University (2004)

  14. Ye, X., Zhang, S.Y.: A conforming discontinuous Galerkin finite element method: Part II. Int. J. Numer. Anal. Model. 17(2), 281–296 (2020)

    MathSciNet  MATH  Google Scholar 

  15. Ye, X., Zhang, S.Y.: A conforming discontinuous Galerkin finite element method: Part III. Int. J. Numer. Anal. Model. 17(6), 794–805 (2020)

    MathSciNet  MATH  Google Scholar 

  16. Ye, X., Zhang, S.Y.: A conforming discontinuous Galerkin finite element method for the Stokes problem on polytopal meshes. Int. J. Numer. Meth. Fluids 93, 1913–1928 (2021)

    Article  MathSciNet  Google Scholar 

  17. Zhang, J.F., Deng, X.W.: A robust discontinuous Galerkin high-order finite element method for elasticity problems with interfaces. Int. J. Comput. Methods 17(9), 1950076 (2020)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

The authors would like to thank the reviewers for their valuable comments and suggestions, which lead to a significant improvement of this manuscript. The authors’ work was partially supported by National Natural Science Foundation of China under Grant Nos. 11871038, 11771367 and 12131014.

Funding

The authors have not disclosed any funding.

Author information

Authors and Affiliations

  1. School of Mathematics, Shandong University, Jinan, 250100, Shandong, China

    Yue Wang & Fuzheng Gao

  2. Department of Mathematics, Jinan University, Guangzhou, 510632, Guangdong, China

    Jintao Cui

Authors
  1. Yue Wang
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Fuzheng Gao
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Jintao Cui
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Fuzheng Gao.

Ethics declarations

Conflict of interest

The authors have not disclosed any conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wang, Y., Gao, F. & Cui, J. A Conforming Discontinuous Galerkin Finite Element Method for Linear Elasticity Interface Problems. J Sci Comput 92, 9 (2022). https://doi.org/10.1007/s10915-022-01857-0

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10915-022-01857-0

Keywords

Mathematics Subject Classification