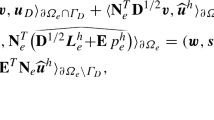Skip to main content
SpringerLink
Log in

Analysis of a Mixed DG Method for Stress-Velocity Formulation of the Stokes Equations

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

Abstract

In this paper we propose and analyze a novel mixed DG scheme for stress-velocity formulation of the Stokes equations with arbitrary polynomial orders on simplicial meshes and the symmetry of stress is strongly imposed. The optimal convergence error estimates are proved for stress and velocity measured in \(L^2\) errors. The primary difficulty is to prove \(L^2\) error of stress, and standard techniques will lead to sub-optimal convergence error estimates. As such, some new ingredients are adopted to recover the optimal convergence rates. The proposed scheme is also extended to solve the Brinkman problem, aiming to get a uniformly robust scheme for both the Stokes and Darcy limits. Finally, several numerical experiments are carried out to verify the performances of the proposed scheme. In particular, the numerical results demonstrate that the proposed scheme is robust with respect to the values of the viscosity.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2

Similar content being viewed by others

Data Availability Statements

The datasets generated during and/or analysed during the current study are available from the corresponding author on reasonable request.

References

  1. Arnold, D.N., Qin, J.: Quadratic Velocity/Linear Pressure Stokes Elements. Advances in Computer Methods for Partial Differential Equations 7, 28–34 (1992)

    Google Scholar 

  2. Cockburn, B., Kanschat, G., Schötzau, D.: A note on discontinuous Galerkin divergence-free solutions of the Navier-Stokes equations. J. Sci. Comput. 31, 1269–1286 (2007)

    Article  MathSciNet  Google Scholar 

  3. Wang, J., Ye, X.: New finite element methods in computational fluid dynamics by H(div) elements. SIAM J. Numer. Anal. 45, 1269–1286 (2007)

    Article  MathSciNet  Google Scholar 

  4. Badia, S., Codina, R., Gudi, T., Guzmán, J.: Error analysis of discontinuous Galerkin methods for the Stokes problem under minimal regularity. IMA J. Numer. Anal. 34, 800–819 (2013)

    Article  MathSciNet  Google Scholar 

  5. Jeon, Y., Park, E.-J., Sheen, D.: A hybridized finite element method for the Stokes problem. Comput. Math. Appl. 68, 2222–2232 (2014)

    Article  MathSciNet  Google Scholar 

  6. Di Pietro, D.A., Ern, A., Linke, A., Schieweck, F.: A discontinuous skeletal method for the viscosity-dependent Stokes problem. Comput. Methods Appl. Mech. Engrg. 306, 175–195 (2016)

    Article  MathSciNet  Google Scholar 

  7. Wang, J., Ye, X.: A weak Galerkin finite element method for the Stokes equations. Adv. Comput. Math. 42, 155–174 (2016)

    Article  MathSciNet  Google Scholar 

  8. Farhloul, M., Fortin, M.: A new mixed finite element for the Stokes and Elasticity problems. SIAM J. Numer. Anal. 30, 971–990 (1993)

    Article  MathSciNet  Google Scholar 

  9. Behr, M.A., Franca, L.P., Tezduyar, T.E.: Stabilized finite element methods for the velocity-pressure-stress formulation of incompressible flows. Comput. Methods Appl. Mech. Engrg. 104, 31–48 (1993)

    Article  MathSciNet  Google Scholar 

  10. Cai, Z., Lee, B., Wang, P.: Least-squares methods for incompressible Newtonian fluid flow: Linear stationary problems. SIAM J. Numer. Anal. 42, 843–859 (2005)

    Article  MathSciNet  Google Scholar 

  11. Figueroa, L.E., Gatica, G.N., Márquez, A.: Augmented mixed finite element methods for the stationary Stokes equations. SIAM J. Sci. Comput. 31, 1082–1119 (2009)

    Article  MathSciNet  Google Scholar 

  12. Carstensen, C., Kim, D., Park, E.-J.: A priori and a posteriori pseudostress-velocity mixed finite element error analysis for the Stokes problem. SIAM J. Numer. Anal. 49, 2501–2523 (2011)

    Article  MathSciNet  Google Scholar 

  13. Cai, Z., Zhang, S.: Mixed methods for stationary Navier-Stokes equations based on pseudostress-pressure-velocity formulation. Math. Comp. 81, 1903–1927 (2012)

    Article  MathSciNet  Google Scholar 

  14. Carstensen, C., Gedicke, J., Park, E.-J.: Numerical experiments for the Arnold-Winther mixed finite elements for the Stokes problem. SIAM J. Sci. Comput. 34, A2267–A2287 (2012)

    Article  MathSciNet  Google Scholar 

  15. Du, J., Chung, E.T., Lam, M.F., Wang, X.-P.: Discontinuous Galerkin method with staggered hybridization for a class of nonlinear Stokes equations. J. Sci. Comput. 76, 1547–1577 (2018)

    Article  MathSciNet  Google Scholar 

  16. Kim, D., Zhao, L., Park, E.-J.: Staggered DG methods for the pseudostress-velocity formulation of the Stokes equations on general meshes. SIAM J. Sci. Comput. 42, A2537–A2560 (2020)

    Article  MathSciNet  Google Scholar 

  17. Brezzi, F., Fortin, M.: Mixed and Hybrid Finite Element Methods. Springer-Verlag, New York (1991)

  18. Olshanskii, M.A., Reusken, A.: Grad-div stabilization for Stokes equations. Math. Comp. 73, 1699–1718 (2004)

    Article  MathSciNet  Google Scholar 

  19. Guzmán, J., Neilan, M.: Conforming and divergence-free Stokes elements in three dimensions. IMA J. Numer. Anal. 34, 1489–1508 (2013)

    Article  MathSciNet  Google Scholar 

  20. Guzmán, J., Neilan, M.: Conforming and divergence-free Stokes elements on general triangular meshes. Math. Comp. 285, 15–36 (2014)

    MathSciNet  MATH  Google Scholar 

  21. Linke, A.: A divergence-free velocity reconstruction for incompressible flows. C. R. Math. Acad. Sci. Paris 350, 837–840 (2012)

    Article  MathSciNet  Google Scholar 

  22. Linke, A.: On the role of the Helmholtz decomposition in mixed methods for incompressible flows and a new variational crime. Comput. Methods Appl. Mech. Engrg. 268, 782–800 (2014)

    Article  MathSciNet  Google Scholar 

  23. Brennecke, C., Linke, A., Merdon, C., Schöberl, J.: Optimal and pressure-independent \({L}^2\) velocity error estimates for a modified Crouzeix-Raviart Stokes element with BDM reconstructions. J. Comput. Math. 33, 191–208 (2015)

    Article  MathSciNet  Google Scholar 

  24. Linke, A., Merdon, C.: Pressure-robustness and discrete Helmholtz projectors in mixed finite element methods for the incompressible Navier-Stokes equations. Comput. Methods Appl. Mech. Engrg. 311, 304–326 (2016)

    Article  MathSciNet  Google Scholar 

  25. Frerichs, D., Merdon, C.: Divergence-preserving reconstructions on polygons and a really pressure-robust virtual element method for the Stokes problem. IMA J. Numer. Anal., (2020)

  26. Mu, L.: Pressure robust weak Galerkin finite element methods for Stokes problems. SIAM J. Sci. Comput. 42, B608–B629 (2020)

    Article  MathSciNet  Google Scholar 

  27. Zhao, L., Park, E.-J., Chung, E.T.: A pressure robust staggered discontinuous Galerkin method for the Stokes equations. arXiv:2007.00298, (2020)

  28. Wang, G., Mu, L., Wang, Y., He, Y.: A pressure-robust virtual element method for the Stokes problem. Comput. Methods Appl. Mech. Engrg. 382, (2021)

  29. Qian, Y., Wu, S., Wang, F.: A mixed discontinuous Galerkin method with symmetric stress for Brinkman problem based on the velocity-pseudostress formulation. Comput. Methods Appl. Mech. Engrg. 368, 113177 (2020)

    Article  MathSciNet  Google Scholar 

  30. Mardal, K.A., Tai, X.-C., Winther, R.: A robust finite element method for Darcy-Stokes flow. SIAM J. Numer. Anal. 40, 1605–1631 (2002)

    Article  MathSciNet  Google Scholar 

  31. Burman, E., Hansbo, P.: Stabilized Crouzeix-Raviart element for the Darcy-Stokes problem. Numer. Methods Partial Differential Equations 21(5), 986–997 (2005)

    Article  MathSciNet  Google Scholar 

  32. Könnö, J., Stenberg, R.: H(div)-conforming finite elements for the Brinkman problem. Math. Models Methods Appl. Sci. 21, 2227–2248 (2011)

    Article  MathSciNet  Google Scholar 

  33. Guzmán, J., Neilan, M.: A family of nonconforming elements for the Brinkman problem. IMA J. Numer. Anal. 32, 1484–1508 (2012)

    Article  MathSciNet  Google Scholar 

  34. Könnö, J., Stenberg, R.: Numerical computations with H(div)-finite elements for the Brinkman problem. Comput. Geosci. 16, 139–158 (2012)

    Article  MathSciNet  Google Scholar 

  35. Anaya, V., Mora, D., Oyarzúa, R., Ruiz-Baier, R.: A priori and a posteriori error analysis of a mixed scheme for the Brinkman problem. Numer. Math. 133, 781–817 (2016)

    Article  MathSciNet  Google Scholar 

  36. Raviart, P.A., Thomas, J.M.: A mixed finite element method for second order elliptic problems, Mathematical Aspects of Finite Element Method, (I. Galligani & E. Magenes eds). Lectures Notes in Math. 606. New York: Springer, pp. 292–315. (1977)

  37. Brenner, S.C.: Korn’s inequalities for piecewise \(H^1\) vector fields. Math. Comp. 73, 1067–1087 (2004)

    Article  MathSciNet  Google Scholar 

  38. Boffi, D., Brezzi, F., Fortin, M.: Mixed Finite Element Methods and Applications, vol. 1. Springer, Berlin, Heidelberg (2013)

  39. Brezzi, F., Douglas, J., Marini, L.D.: Two families of mixed finite elements for second order elliptic problems. Numer. Math. 47, 217–235 (1985)

    Article  MathSciNet  Google Scholar 

  40. Ciarlet, P.G.: The Finite Element Method for Elliptic Problems. Soc. Ind. Appl. Math. (2002)

  41. Pietro, D.A.Di, Ern, A.: Mathematical Aspects of Discontinuous Galerkin Methods, in: Mathématiques & Applications, volume 69. Springer, Berlin, Heidelberg, (2012)

  42. Girault, V., Raviart, P.A.: Finite element methods for Navier-Stokes equations: Theory and algorithms. Springer Series in Computational Mathematics, vol. 5. Springer-Verlag, Berlin, (1986)

  43. Amrouche, C., Girault, V.: On the existence and regularity of the solution of Stokes problem in arbitrary dimension. Proc. Japan Acad. 67, 171–175 (1991)

    MathSciNet  MATH  Google Scholar 

  44. Kovasznay, L.I.G.: Laminar flow behind a two-dimensional grid. Math. Proc. Camb. Philos. Soc. 44, 58–62 (1948)

    Article  MathSciNet  Google Scholar 

Download references

Funding

The research of Lina Zhao was supported by a grant from City University of Hong Kong (Project No. 7200699)

Author information

Authors and Affiliations

  1. Department of Mathematics, City University of Hong Kong, Kowloon Tong, Hong Kong SAR, China

    Lina Zhao

Authors
  1. Lina Zhao
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Lina Zhao.

Ethics declarations

Conflict of interest

There is no conflicts of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhao, L. Analysis of a Mixed DG Method for Stress-Velocity Formulation of the Stokes Equations. J Sci Comput 92, 44 (2022). https://doi.org/10.1007/s10915-022-01895-8

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10915-022-01895-8

Keywords

Search

Navigation