Optimal Error Estimates of the Local Discontinuous Galerkin Method and High-order Time Discretization Scheme for the Swift–Hohenberg Equation

Abstract

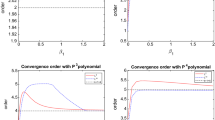
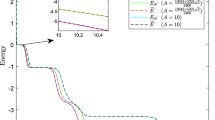
In this paper, we develop a local discontinuous Galerkin (LDG) method for the Swift–Hohenberg equation. The energy stability and optimal error estimates in $L^2$ norm of the semi-discrete LDG scheme are established. To avoid the severe time step restriction of explicit time marching methods, a first-order linear scheme based on the scalar auxiliary variable (SAV) method is employed for temporal discretization. Coupled with the LDG spatial discretization, we achieve a fully-discrete LDG method and prove its energy stability and optimal error estimates. To improve the temporal accuracy, the semi-implicit spectral deferred correction (SDC) method is adapted iteratively. Combining with the SAV method, the SDC method can be linear, high-order accurate and energy stable in our numerical tests. Numerical experiments are presented to verify the theoretical results and to show the efficiency of the proposed methods.

Appendix

In this section, we will present the proof of Lemma 3.2, the optimal error estimate of the elliptic projection, by two lemmas. Denote the errors between the exact solutions of (2.1) and the elliptic projection (3.25) by $R_{u}$, $R_{\varvec{\omega }}$, $R_q$, $R_{{\varvec{s}}}$. Suppose $(P, \Pi ) = (P^{-}, P^{+})$ is defined by (2.13)–(2.14) for the one-dimensional space, and take $(P, \Pi ) = (P^{-}, \Pi ^{+})$ defined by (2.15)–(2.16) for multidimensional Cartesian meshes. Then the errors can be divided into

$$\begin{aligned} R_{u}&=u-Pu+PR_u=\eta _u+PR_u,\;\;R_{{\varvec{\omega }}}={\varvec{\omega }}-\Pi {\varvec{\omega }}+\Pi R_{\varvec{\omega }}=\eta _{\varvec{\omega }}+\Pi R_{\varvec{\omega }},\\ R_{q}&=q-Pq+PR_q=\eta _q+PR_q,\;\;R_{{{\varvec{s}}}}={{\varvec{s}}}-\Pi {{\varvec{s}}}+\Pi R_{{\varvec{s}}}=\eta _{{\varvec{s}}}+\Pi R_{{\varvec{s}}}. \end{aligned}$$

With the definition of the elliptic projection (3.25) and the property (2.17), we obtain the error equation

$$\begin{aligned} 0&=H^{+}(\Pi R_{{\varvec{s}}},\varphi _1), \end{aligned}$$

(6.1a)

$$\begin{aligned} (R_{{\varvec{s}}},{\varvec{\theta } }_1)&=-H^{-}(\eta _q,{\varvec{\theta } }_1)-H^{-}(PR_q,{\varvec{\theta } }_1), \end{aligned}$$

(6.1b)

$$\begin{aligned} (R_q,\varphi _2)&=-H^{+}(\Pi R_{\varvec{\omega }},\varphi _2), \end{aligned}$$

(6.1c)

$$\begin{aligned} (R_{\varvec{\omega }},{\varvec{\theta } }_2)&=-H^{-}(\eta _u,{\varvec{\theta } }_2)-H^{-}(PR_u,{\varvec{\theta } }_2). \end{aligned}$$

(6.1d)

Note that for the one-dimensional space, $H^{-}(\eta _q,{\varvec{\theta } }_1)=0$ and $H^{-}(\eta _u,{\varvec{\theta } }_2)$. The analysis will be the same as that in [25]. Now we show the following estimates.

Lemma 6.1

$$\begin{aligned} \Vert \Pi R_{{\varvec{s}}}\Vert&\lesssim h^{k+1},\;\;\Vert R_{{\varvec{s}}}\Vert +h^{1/2}\Vert R_{{\varvec{s}}}\cdot {{\varvec{n}}}\Vert _{{\mathcal {E}}_h}\lesssim h^{k+1},\\ \Vert P R_q\Vert&\lesssim \Vert PR_u\Vert +h^{k+1},\;\;\Vert R_q\Vert \lesssim \Vert PR_u\Vert +h^{k+1},\\ \Vert \Pi R_{\varvec{\omega }}\Vert&\lesssim \Vert PR_u\Vert +h^{k+1},\;\;\Vert R_{\varvec{\omega }}\Vert +h^{1/2}\Vert R_{\varvec{\omega }}\cdot {{\varvec{n}}}\Vert _{{\mathcal {E}}_h}\lesssim \Vert PR_u\Vert +h^{k+1}. \end{aligned}$$

Here and below the notation $a\lesssim b$ means that, there exists a constant $C>0$ such that $a\le b$.

Proof

Taking ${\varvec{\theta } }_1=\Pi R_{{\varvec{s}}}$ in (6.1b) and by the property (2.4), (6.1a), we have

$$\begin{aligned} (R_{{\varvec{s}}},\Pi R_{{\varvec{s}}})&=-H^{-}(\eta _q,\Pi R_{{\varvec{s}}})-H^{-}(PR_q,\Pi R_{{\varvec{s}}})\\&=-H^{-}(\eta _q,\Pi R_{{\varvec{s}}})+H^{+}(\Pi R_{{\varvec{s}}},PR_q)=-H^{-}(\eta _q,\Pi R_{{\varvec{s}}}), \end{aligned}$$

which yields

$$\begin{aligned} \Vert \Pi R_{{\varvec{s}}}\Vert ^{2}&=(\Pi R_{{\varvec{s}}},\Pi R_{{\varvec{s}}}-R_{{\varvec{s}}})+(R_{{\varvec{s}}},\Pi R_{{\varvec{s}}})\\&=(\Pi R_{{\varvec{s}}},-\eta _{{\varvec{s}}})-H^{-}(\eta _q,\Pi R_{{\varvec{s}}})\\&\lesssim h^{k+1}\Vert \Pi R_{{\varvec{s}}}\Vert . \end{aligned}$$

Here we use the approximation properties (2.18) and (2.19). Thus by the trace inequality and the triangle inequality, we have

$$\begin{aligned} \Vert \Pi R_{{\varvec{s}}}\Vert +h^{1/2}\Vert \Pi R_{{\varvec{s}}}\cdot {{\varvec{n}}}\Vert _{{\mathcal {E}}_h}\lesssim h^{k+1},\;\;\Vert R_{{\varvec{s}}}\Vert +h^{1/2}\Vert R_{{\varvec{s}}}\cdot {{\varvec{n}}}\Vert _{{\mathcal {E}}_h}\lesssim h^{k+1}. \end{aligned}$$

Taking $\varphi _2=PR_q$ in (6.1c) and by (2.4) and (6.1b), we obtain

$$\begin{aligned} (R_q,PR_q)&=-H^{+}(\Pi R_{\varvec{\omega }},PR_q)=H^{-}(PR_q,\Pi R_{\varvec{\omega }})\\&=-H^{-}(\eta _q,\Pi R_{\varvec{\omega }})-(R_{{\varvec{s}}},\Pi R_{\varvec{\omega }}). \end{aligned}$$

Then

$$\begin{aligned} \Vert PR_q\Vert ^2&=(PR_q,PR_q-R_q)+(R_q,PR_q)\\&=(PR_q,-\eta _q)-H^{-}(\eta _q,\Pi R_{\varvec{\omega }})-(R_{{\varvec{s}}},\Pi R_{\varvec{\omega }})\\&\le \frac{1}{2} \Vert PR_q\Vert ^2+\frac{1}{2} \Vert \Pi R_{\varvec{\omega }}\Vert ^2+Ch^{2k+2}, \end{aligned}$$

where the Young’s inequality and (2.18)–(2.19) are used. Hence, we get

$$\begin{aligned} \Vert PR_q\Vert \lesssim \Vert \Pi R_{\varvec{\omega }}\Vert +h^{k+1},\;\;\Vert R_q\Vert \lesssim \Vert \Pi R_{\varvec{\omega }}\Vert +h^{k+1}. \end{aligned}$$

(6.2)

Finally, we take ${\varvec{\theta } }_2=\Pi R_{\varvec{\omega }}$ in (6.1d) and apply (2.4), (6.1c) to derive

$$\begin{aligned} (R_{\varvec{\omega }},\Pi R_{\varvec{\omega }})&=-H^{-}(\eta _u,\Pi R_{\varvec{\omega }})-H^{-}(PR_u,\Pi R_{\varvec{\omega }})\\&=-H^{-}(\eta _u,\Pi R_{\varvec{\omega }})-(R_q,PR_u). \end{aligned}$$

Then by Cauchy–Schwarz inequality, the Young’s inequality and (2.18)–(2.19), we have

$$\begin{aligned} \Vert \Pi R_{\varvec{\omega }}\Vert ^2&=(\Pi R_{\varvec{\omega }},\Pi R_{\varvec{\omega }}-R_{\varvec{\omega }})+(R_{\varvec{\omega }},\Pi R_{\varvec{\omega }})\\&=(\Pi R_{\varvec{\omega }},-\eta _{\varvec{\omega }})-H^{-}(\eta _u,\Pi R_{\varvec{\omega }})-(R_q,PR_u)\\&\le \frac{1}{2}\Vert \Pi R_{\varvec{\omega }}\Vert ^2+\Vert PR_u\Vert ^2+Ch^{2k+2}, \end{aligned}$$

which yields

$$\begin{aligned} \Vert \Pi R_{\varvec{\omega }}\Vert \lesssim \Vert PR_u\Vert +h^{k+1},\;\;\Vert R_{\varvec{\omega }}\Vert \lesssim \Vert PR_u\Vert +h^{k+1}. \end{aligned}$$

It follows from (6.2) that

$$\begin{aligned} \Vert PR_q\Vert \lesssim \Vert PR_u\Vert +h^{k+1},\;\;\Vert R_q\Vert \lesssim \Vert PR_u\Vert +h^{k+1}. \end{aligned}$$

A simple use of the trace inequality and the triangle inequality gives

$$\begin{aligned} h^{1/2}\Vert \Pi R_{\varvec{\omega }}\cdot {{\varvec{n}}}\Vert _{{\mathcal {E}}_h}\lesssim \Vert PR_u\Vert +h^{k+1},\;\;h^{1/2}\Vert R_{\varvec{\omega }}\cdot {{\varvec{n}}}\Vert _{{\mathcal {E}}_h}\lesssim \Vert PR_u\Vert +h^{k+1}. \end{aligned}$$

The proof is completed. $\square$

With the help of the adjoint problem (3.26), we will show the second lemma, which is used for the estimate of $PR_u$.

Lemma 6.2

For $z\in L^2(\Omega )$, we get

$$\begin{aligned} (PR_u,z)=&-H^{-}(\eta _u,\Pi {\varvec{\zeta } })-H^{-}(\eta _q,\Pi {\varvec{\theta } })\nonumber \\&-(R_{\varvec{\omega }},\Pi {\varvec{\zeta } }-{\varvec{\zeta } })+(R_q,P\varphi -\varphi )-(R_{{\varvec{s}}},\Pi {\varvec{\theta } }-{\varvec{\theta } })\nonumber \\&+(\varphi -P\varphi ,\nabla \cdot R_{\varvec{\omega }})+(\sigma -P\sigma ,\nabla \cdot R_{{\varvec{s}}})\nonumber \\&-{<}(R_{\varvec{\omega }}-{\hat{R}}_{\varvec{\omega }})\cdot {{\varvec{n}}},\varphi -P\varphi>-<(R_{{\varvec{s}}}-{\hat{R}}_{{\varvec{s}}})\cdot {{\varvec{n}}},\sigma -P\sigma >. \end{aligned}$$

(6.3)

Proof

By the adjoint problem (3.26) and integrating by part, we have

$$\begin{aligned} (PR_u,z)=(PR_u,\nabla \cdot {\varvec{\zeta } })&=-({\varvec{\zeta } },\nabla PR_u)+<{\varvec{\zeta } }\cdot {{\varvec{n}}},PR_u>\nonumber \\&=-H^{+}({\varvec{\zeta } },PR_u)-<({\hat{{\varvec{\zeta } }}}-{\varvec{\zeta } })\cdot {{\varvec{n}}},PR_u>\nonumber \\&=-H^{+}({\varvec{\zeta } }-\Pi {\varvec{\zeta } },PR_u)-H^{+}(\Pi {\varvec{\zeta } },PR_u)\nonumber \\&=H^{-}(PR_u,\Pi {\varvec{\zeta } }). \end{aligned}$$

(6.4)

Here the second line uses the definition of $H^{+}$, the third line holds since ${\varvec{\zeta } }$ is continuous across the element interface and we adopt the property (2.17) of $\Pi$ as well as (2.4) for the last line. By (6.1d), we obtain

$$\begin{aligned}&H^{-}(PR_u,\Pi {\varvec{\zeta } })\\&=-H^{-}(\eta _u,\Pi {\varvec{\zeta } })-(R_{\varvec{\omega }},\Pi {\varvec{\zeta } })=-H^{-}(\eta _u,\Pi {\varvec{\zeta } })-(R_{\varvec{\omega }},\Pi {\varvec{\zeta } }-{\varvec{\zeta } })-(R_{\varvec{\omega }},{\varvec{\zeta } }). \end{aligned}$$

Similarly as (6.4), we derive

$$\begin{aligned} -(R_{\varvec{\omega }},{\varvec{\zeta } })=-(R_{\varvec{\omega }},\nabla \varphi )=&-(R_{\varvec{\omega }},\nabla (\varphi -P\varphi ))-(R_{\varvec{\omega }},\nabla P\varphi )\nonumber \\ =&(\nabla \cdot R_{\varvec{\omega }},\varphi -P\varphi )-<R_{\varvec{\omega }}\cdot {{\varvec{n}}},\varphi -P\varphi>\nonumber \\&-H^{+}(R_{\varvec{\omega }},P\varphi )-<{\hat{R}}_{\varvec{\omega }}\cdot {{\varvec{n}}},P\varphi>\nonumber \\ =&(\nabla \cdot R_{\varvec{\omega }},\varphi -P\varphi )-<(R_{\varvec{\omega }}-{\hat{R}}_{\varvec{\omega }})\cdot {{\varvec{n}}},\varphi -P\varphi >\nonumber \\&+(R_q,P\varphi -\varphi )+(R_q,\varphi ), \end{aligned}$$

(6.5)

where the second line holds by integrating by parts, the third line uses the definition of $H^{+}$ and the fourth line uses the fact that $<{\hat{R}}_{\varvec{\omega }}\cdot {{\varvec{n}}},\varphi >=0$, since $\varphi$ is continuous and we consider the periodic boundary condition. In addition, we adopt (2.17), (6.1c) for the last line. Along the same line to obtain (6.4)–(6.5), we have

$$\begin{aligned} (R_q,\varphi )&=(\eta _q,\nabla \cdot {\varvec{\theta } })-H^{-}(\eta _q,\Pi {\varvec{\theta } })-(R_{{\varvec{s}}},\Pi {\varvec{\theta } }-{\varvec{\theta } })-(R_{{\varvec{s}}},{\varvec{\theta } }),\\ -(R_{{\varvec{s}}},{\varvec{\theta } })&=(\sigma -P\sigma ,\nabla \cdot R_{{\varvec{s}}})-<(R_{{\varvec{s}}}-{\hat{R}}_{{\varvec{s}}})\cdot {{\varvec{n}}},\sigma -P\sigma >. \end{aligned}$$

Thus we complete the proof by combining the above equalities. $\square$

Now we are ready to prove the optimal error estimates of the elliptic projections. Take $z=PR_u$ in (6.3) and denote each line of the right hand in (6.3) by ${\mathcal {S}}_i$, $i=1,2,3,4$. Then the approximation properties (2.18)–(2.19) and the triangle inequality yield

$$\begin{aligned} {\mathcal {S}}_1\le Ch^{k+1}(\Vert \Pi {\varvec{\zeta } }\Vert +\Vert \Pi {\varvec{\theta } }\Vert )\le Ch^{k+1}(\Vert {\varvec{\zeta } }\Vert _{H^1(\Omega )}+\Vert {\varvec{\theta } }\Vert _{H^3(\Omega )}). \end{aligned}$$

By Cauchy–Schwarz inequality and (2.18), we derive

$$\begin{aligned} {\mathcal {S}}_2&\le C\Vert R_{\varvec{\omega }}\Vert h^{\min \{1,k+1\}}\Vert {\varvec{\zeta } }\Vert _{H^1(\Omega )}+C\Vert R_q\Vert h^{\min \{2,k+1\}}\Vert \varphi \Vert _{H^2(\Omega )}\\&\quad +C\Vert R_{{\varvec{s}}}\Vert h^{\min \{3,k+1\}}\Vert {\varvec{\theta } }\Vert _{H^3(\Omega )}\\&\le Ch(\Vert PR_u\Vert +h^{k+1})(\Vert {\varvec{\zeta } }\Vert _{H^1(\Omega )}+\Vert \varphi \Vert _{H^2(\Omega )}+\Vert {\varvec{\theta } }\Vert _{H^3(\Omega )}) \end{aligned}$$

for $k\ge 1$. Here we use the estimates in Lemma 6.1. It follows from the triangle inequality, the inverse inequality, the property (2.18) and Lemma 6.1 that

$$\begin{aligned} {\mathcal {S}}_3&\le \Vert \varphi -P\varphi \Vert (\Vert \nabla \cdot \eta _{\varvec{\omega }}\Vert +\Vert \nabla \cdot \Pi R_{\varvec{\omega }}\Vert )+\Vert \sigma -P\sigma \Vert (\Vert \nabla \cdot \eta _{{\varvec{s}}}\Vert +\Vert \nabla \cdot \Pi R_{{\varvec{s}}}\Vert )\\&\le Ch^{\min \{1,k\}}\Vert \varphi \Vert _{H^2(\Omega )}(h^{k+1}+\Vert \Pi R_{\varvec{\omega }}\Vert )+Ch^{\min \{3,k\}}\Vert \sigma \Vert _{H^4(\Omega )}(h^{k+1}+\Vert \Pi R_{{\varvec{s}}}\Vert )\\&\le Ch(\Vert PR_u\Vert +h^{k+1})(\Vert \varphi \Vert _{H^2(\Omega )}+\Vert \sigma \Vert _{H^4(\Omega )}). \end{aligned}$$

For the last term ${\mathcal {S}}_4$, we adopt (2.18) and Lemma 6.1 to obtain

$$\begin{aligned} {\mathcal {S}}_4&\le \Vert R_{\varvec{\omega }}\cdot {{\varvec{n}}}\Vert _{{\mathcal {E}}_h}\Vert \varphi -P\varphi \Vert _{{\mathcal {E}}_h}+\Vert R_{{\varvec{s}}}\cdot {{\varvec{n}}}\Vert _{{\mathcal {E}}_h}\Vert \sigma -P\sigma \Vert _{{\mathcal {E}}_h}\\&\le Ch^{\min \{1,k\}}\Vert \varphi \Vert _{H^2(\Omega )}(h^{k+1}+\Vert PR_u\Vert )+Ch^{\min \{3,k\}}\Vert \sigma \Vert _{H^4(\Omega )}h^{k+1}\\&\le Ch(\Vert PR_u\Vert +h^{k+1})(\Vert \varphi \Vert _{H^2(\Omega )}+\Vert \sigma \Vert _{H^4(\Omega )}). \end{aligned}$$

Adding the estimates of ${\mathcal {S}}_i$, $i=1,2,3,4$ to the equality (6.3) and by the elliptic regularity (3.27), we have

$$\begin{aligned} \Vert PR_u\Vert ^{2}&\le Ch(\Vert PR_u\Vert +h^{k+1})(\Vert {\varvec{\zeta } }\Vert _{H^1(\Omega )}+\Vert \varphi \Vert _{H^2(\Omega )}+\Vert {\varvec{\theta } }\Vert _{H^3(\Omega )}+\Vert \sigma \Vert _{H^4(\Omega )})\\&\le CC_{*}h(\Vert PR_u\Vert +h^{k+1})\Vert PR_u\Vert . \end{aligned}$$

Thus we can obtain the optimal error estimate (3.28) of the elliptic projection by the Young’s equality, the triangle inequality and Lemma 6.1.