Skip to main content
Springer Nature Link
Log in

Local Discontinuous Galerkin Methods with Multistep Implicit–Explicit Time Discretization for Nonlinear Schrödinger Equations

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

Abstract

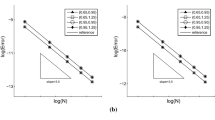
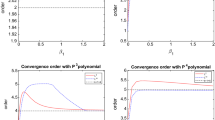
In this paper, we investigate the local discontinuous Galerkin (LDG) methods coupled with multistep implicit–explicit (IMEX) time discretization to solve one-dimensional and two-dimensional nonlinear Schrödinger equations. In this approach, the nonlinear terms are treated explicitly, while the linear terms are handled implicitly. By the skew symmetry property of LDG operators and the properties of Gauss–Radau projections, we obtain error estimates for the prime and auxiliary variables, as well as the estimate for the time difference of the prime variables. These results, together with a carefully chosen numerical initial condition, allow us to obtain the optimal error estimate in both space and time for the fully discrete scheme. Numerical experiments are performed to verify the accuracy and efficiency of the proposed methods.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

Data availability

Data sharing not applicable to this article as no datasets were generated or analysed during the current study.

References

  1. Ciarlet, P.G.: The Finite Element Method for Elliptic Problems. Society for Industrial and Applied Mathematics (SIAM), Philadelphia (2002)

    Google Scholar 

  2. Chen, A., Cheng, Y., Liu, Y., Zhang, M.: Superconvergence of ultra-weak discontinuous Galerkin methods for the linear Schödinger equation in one dimension. J. Sci. Comput. 82(1), 1–44 (2020)

    Google Scholar 

  3. Castillo, P., Cockburn, B., Schötzau, D., Schwab, C.: Optimal a priori error estimates for the hp-version of the local discontinuous Galerkin method for the convection-diffusion problems. Math. Comput. 71(238), 455–478 (2002)

    MathSciNet  Google Scholar 

  4. Castillo, P., Gómez, S.: On the conservation of fractional nonlinear Schrödinger equation’s invariants by the local discontinuous Galerkin method. J. Sci. Comput. 77(3), 1444–1467 (2018)

    MathSciNet  Google Scholar 

  5. Cockburn, B., Hou, S., Shu, C..-W.: The Runge-Kutta local projection discontinuous Galerkin finite element method for conservation laws. IV. The multidimensional case. Math. Comput. 54(190), 545–581 (1990)

    MathSciNet  Google Scholar 

  6. Chen, A., Li, F., Cheng, Y.: An ultra-weak discontinuous Galerkin method for Schrödinger equation in one dimension. J. Sci. Comput. 78(2), 772–815 (2019)

    MathSciNet  Google Scholar 

  7. Cockburn, B., Lin, S..Y., Shu, C..-W..: TVB Runge–Kutta local projection discontinuous Galerkin finite element method for conservation laws. III: One-dimensional systems. J. Comput. Phys. 84(1), 90–113 (1989)

    MathSciNet  Google Scholar 

  8. Cockburn, B., Shu, C.-W.: Runge–Kutta discontinuous Galerkin methods for convection-dominated problems. J. Sci. Comput. 16(3), 173–261 (2001)

    MathSciNet  Google Scholar 

  9. Cockburn, B., Shu, C.-W.: The local discontinuous Galerkin method for time-dependent convection–diffusion systems. SIAM J. Numer. Anal. 35(6), 2440–2463 (1998)

    MathSciNet  Google Scholar 

  10. Cockburn, B., Shu, C..-W.: The Runge–Kutta discontinuous Galerkin method for conservation laws. V: multidimensional systems. J. Comput. Phys. 141(2), 199–224 (1998)

    MathSciNet  Google Scholar 

  11. Cockburn, B., Shu, C.-W.: The Runge–Kutta local projection \(P^1\)-discontinuous Galerkin method for scalar conservation laws. Math. Model. Numer. Anal. 25, 337–361 (1991)

    Google Scholar 

  12. Cockburn, B., Shu, C..-W.: TVB Runge-Kutta local projection discontinuous Galerkin finite element method for conservation laws. II: General framework. Math. Comput. 52(186), 411–435 (1989)

    MathSciNet  Google Scholar 

  13. Daǧ, I.: A quadratic B-spline finite element method for solving nonlinear Schrödinger equation. Comput. Methods Appl. Mech. Eng. 174(1–2), 247–258 (1999)

    Google Scholar 

  14. Dong, B., Shu, C.-W., Wang, W.: A new multiscale discontinuous Galerkin method for the one-dimensional stationary Schrödinger equation. J. Sci. Comput. 66(1), 321–345 (2016)

    MathSciNet  Google Scholar 

  15. Gao, Y., Mei, L.: Implicit-explicit multistep methods for general two-dimensional nonlinear Schrödinger equations. Appl. Numer. Math. 109, 41–60 (2016)

    MathSciNet  Google Scholar 

  16. Gong, Y., Wang, Q., Wang, Y., Cai, J.: A conservative Fourier pseudo-spectral method for the nonlinear Schrödinger equation. J. Comput. Phys. 328, 354–370 (2017)

    MathSciNet  Google Scholar 

  17. Guo, L., Xu, Y.: Energy conserving local discontinuous Galerkin methods for the nonlinear Schrödinger equation with wave operator. J. Sci. Comput. 65, 622–647 (2015)

    MathSciNet  Google Scholar 

  18. Hong, J., Ji, L., Liu, Z.: Optimal error estimate of conservative local discontinuous Galerkin method for nonlinear Schrödinger equation. Applied Numerical Mathematics: Transactions of IMACS 127, 164–178 (2018)

    Google Scholar 

  19. Ji, B., Zhang, L.: Error estimate of exponential wave integrator Fourier pseudospectral methods for the nonlinear Schrödinger equation. Appl. Math. Comput. 343, 100–113 (2019)

    MathSciNet  Google Scholar 

  20. Karakashian, O., Makridakis, C.: A space-time finite element method for the nonlinear Schrödinger equation: the continuous Galerkin method. SIAM J. Numer. Anal. 36(6), 1779–1807 (1999)

    MathSciNet  Google Scholar 

  21. Li, M., Gu, X.M., Huang, C., Fei, M., Zhang, G.: A fast linearized conservative finite element method for the strongly coupled nonlinear fractional Schrödinger equations. J. Comput. Phys. 358, 256–282 (2018)

    MathSciNet  Google Scholar 

  22. Lu, W., Huang, Y., Liu, H.: Mass preserving discontinuous Galerkin methods for Schrödinger equations. J. Comput. Phys. 282, 210–226 (2015)

    MathSciNet  Google Scholar 

  23. Liu, H., Huang, Y., Lu, W., Yi, N.: On accuracy of the mass-preserving DG method to multi-dimensional Schrödinger equations. IMA J. Numer. Anal. 39(2), 760–791 (2019)

    MathSciNet  Google Scholar 

  24. Lasaint, P., Raviart, P.A.: On a finite element method for solving the neutron transport equation. Publications mathématiques et informatique de Rennes S4, 1–40 (1974)

    Google Scholar 

  25. Reed, W.H. , Hill,T.: Triangular mesh methods for the neutron transport equation, Los Alamos Scientific Lab., N. Mex.(USA). (1973)

  26. Rosales, R.R., Seibold, B., Shirokoff, D., Zhou, D.: Unconditional stability for multistep IMEX schemes-theory. SIAM J. Numer. Anal. 55(5), 2336–2360 (2017)

    MathSciNet  Google Scholar 

  27. Song, H.: Energy SSP-IMEX Runge-Kutta methods for the Cahn-Hilliard equation. J. Comput. Appl. Math. 292, 576–590 (2016)

    MathSciNet  Google Scholar 

  28. Seibold, B., Shirokoff, D., Zhou, D.: Unconditional stability for multistep IMEX schemes-practice. J. Comput. Phys. 376, 295–321 (2019)

    MathSciNet  Google Scholar 

  29. Shi, D., Wang, J.: Unconditional superconvergence analysis of a Crank-Nicolson Galerkin FEM for nonlimear Schrödinger equation. J. Sci. Comput. 72(3), 1093–1118 (2017)

    MathSciNet  Google Scholar 

  30. Tao, Q., Xia, Y.: Error estimates and post-processing of local discontinuous Galerkin method for Schrödinger equations. J. Comput. Appl. Math. 356, 198–218 (2019)

    MathSciNet  Google Scholar 

  31. Wang, J.: Multisymplectic Fourier pseudospectral method for the nonlinear Schrödinger equations with wave operator. J. Comput. Math. 01, 31–48 (2007)

    Google Scholar 

  32. Wang, H., Liu, Y., Zhang, Q., Shu, C.-W.: Local discontinuous Galerkin methods with implicit-explicit time-marching for time-dependent incompressible fluid flow. Math. Comput. 88(315), 91–121 (2019)

    MathSciNet  Google Scholar 

  33. Wang, H., Shu, C.-W., Zhang, Q.: Stability analysis and error estimates of local discontinuous Galerkin methods with implicit-explicit time-marching for nonlinear convection-diffusion problems. Appl. Math. Comput. 272, 237–258 (2016)

    MathSciNet  Google Scholar 

  34. Wang, H., Shu, C.-W., Zhang, Q.: Stability and error estimates of local discontinuous Galerkin methods with implicit-explicit time-marching for advection-diffusion problems. SIAM J. Numer. Anal. 53(1), 206–227 (2015)

    MathSciNet  Google Scholar 

  35. Wang, H., Zhang, Q., Shu, C..-W.: Stability analysis and error estimates of local discontinuous Galerkin methods with implicit-explicit time-marching for the time-dependent fourth order PDEs. ESAIM: Mathematical Modelling and Numerical Analysis 51(5), 1931–1955 (2017)

    MathSciNet  Google Scholar 

  36. Wang, H., Zhang, Q., Shu, C.-W.: Third order implicit-explicit Runge-Kutta local discontinuous Galerkin methods with suitable boundary treatment for convection-diffusion problems with Dirichlet boundary conditions. J. Comput. Appl. Math. 342, 164–179 (2018)

    MathSciNet  Google Scholar 

  37. Wang, H., Zhang, Q., Wang, S., Shu, C.-W.: Local discontinuous Galerkin methods with explicit-implicit-null time discretizations for solving nonlinear diffusion problems. SCIENCE CHINA Math. 63(1), 183–204 (2020)

    MathSciNet  Google Scholar 

  38. Wang, H., Wang, S., Zhang, Q., Shu, C..-W.: Local discontinuous Galerkin methods with implicit-explicit time-marching for multi-dimensional convection-diffusion problems. ESAIM: Mathematical Modelling and Numerical Analysis 50(4), 1083–1105 (2016)

    MathSciNet  Google Scholar 

  39. Wang, H., Zheng, J., Yu, F., Guo, H., Zhang, Q.: Local discontinuous Galerkin method with implicit-explicit time marching for incompressible miscible displacement problem in porous media. J. Sci. Comput. 78(1), 1–28 (2019)

    MathSciNet  Google Scholar 

  40. Xu, Y., Shu, C.-W.: Local discontinuous Galerkin methods for nonlinear Schrödinger equations. J. Comput. Phys. 205(1), 72–97 (2005)

    MathSciNet  Google Scholar 

  41. Xu, Y., Shu, C.-W.: Optimal error estimates of the semidiscrete local discontinuous Galerkin methods for high order wave equations. SIAM J. Numer. Anal. 50(1), 79–104 (2012)

    MathSciNet  Google Scholar 

  42. Zhang, Q., Gao, F.: A fully-discrete local discontinuous Galerkin method for convection-dominated Sobolev equation. J. Sci. Comput. 51(1), 107–134 (2012)

    MathSciNet  Google Scholar 

  43. Zhang, Q., Shu, C.-W.: Error estimates to smooth solutions of Runge-Kutta discontinuous Galerkin methods for scalar conservation laws. SIAM J. Numer. Anal. 42(2), 641–666 (2004)

    MathSciNet  Google Scholar 

  44. Zhang, Q., Shu, C.-W.: Stability analysis and a priori error estimates of the third order explicit Runge-Kutta discontinuous Galerkin method for scalar conservation laws. SIAM J. Numer. Anal. 48(3), 1038–1063 (2010)

    MathSciNet  Google Scholar 

  45. Zhang, H., Wu, B., Meng, X.: A local discontinuous Galerkin method with generalized alternating fluxes for 2D nonlinear Schrödinger equations. Communications on Applied Mathematics and Computation 4(1), 84–107 (2022)

    MathSciNet  Google Scholar 

Download references

Acknowledgements

The research of Y. Li was supported by National Key Research and Development Program of China 2021YFA1003004. The research of X. Zhong was partially supported by NSFC Grant 12272347. The authors would like to thank Prof. Qiang Zhang from Nanjing University for his valuable suggestions.

Funding

Author Y. Li was partially supported by National Key Research and Development Program of China 2021YFA1003004. Author X. Zhong was partially supported by NSFC Grant 12272347.

Author information

Authors and Affiliations

  1. School of Computer Engineering and Science, Shanghai University, Shanghai, 200444, China

    Ying Li

  2. School of Computer Engineering and Science, Shanghai University, Shanghai, China

    Hui Shi

  3. School of Mathematical Sciences, Zhejiang University, Hangzhou, 310058, Zhejiang, China

    Xinghui Zhong

Authors
  1. Ying Li
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Hui Shi
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Xinghui Zhong
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Xinghui Zhong.

Ethics declarations

Conflict of interest

All authors have no relevant financial or non-financial interests to disclose.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

A The Expression of \(Z_{41}\)

A The Expression of \(Z_{41}\)

To simplify the presentation, the parameter \(\gamma \) is omitted in the following.

By (3.16), (2.17), and (3.11), we first rewrite the expression of \(F^nR^n-f^nr^n\) as

$$\begin{aligned} \begin{aligned} F^nR^n-f^nr^n&=F^n(R^n-r^n)+(F^n-f^n)r^n\\&=F^n(R^n-r^n)+r^n((R^n)^2-(r^n)^2+(S^n)^2-(s^n)^2)\\&=\left( (R^{n})^2+(S^{n})^2\right) e_r^n+r^n\left( (R^n+r^n)e_r^n+(S^n+s^n)e_s^n\right) \\&=\left( (R^{n})^2+(S^{n})^2+(r^n)^2+r^nR^n\right) e_r^n + r^n(s^n+S^n)e_s^n. \end{aligned} \end{aligned}$$
(A.1)

Similarly, \(F^{n-1}R^{n-1}-f^{n-1}r^{n-1}\) can be rewritten as

$$\begin{aligned} \begin{aligned}&F^{n-1}R^{n-1}-f^{n-1}r^{n-1}\\&=\left( (R^{n-1})^2+(S^{n-1})^2+(r^{n-1})^2+r^{n-1}R^{n-1}\right) e_r^{n-1} + r^{n-1}(s^{n-1}+S^{n-1})e_s^{n-1}. \end{aligned} \end{aligned}$$
(A.2)

Then, by subtracting (A.2) from (A.1), we have

$$\begin{aligned}&F^nR^n-f^nr^n-\left( F^{n-1}R^{n-1}-f^{n-1}r^{n-1}\right) \\&\quad =\left( (R^{n})^2+(S^{n})^2+(r^n)^2+r^nR^n\right) e_r^n -\left( (R^{n-1})^2+(S^{n-1})^2+(r^{n-1})^2+r^{n-1}R^{n-1}\right) e_r^{n-1}\\&\qquad + r^n(s^n+S^n)e_s^n-r^{n-1}(s^{n-1}+S^{n-1})e_s^{n-1}\\&\quad =\left( (R^{n})^2+(S^{n})^2+(r^n)^2+r^nR^n-(R^{n-1})^2-(S^{n-1})^2-(r^{n-1})^2-r^{n-1}R^{n-1}\right) e_r^n \\&\qquad +\left( (R^{n-1})^2+(S^{n-1})^2+(r^{n-1})^2+r^{n-1}R^{n-1}\right) (e_r^n-e_r^{n-1})\\&\qquad + \left( r^n(s^n+S^n)-r^{n-1}(s^{n-1}+S^{n-1})\right) e_s^n+r^{n-1}(s^{n-1}+S^{n-1})(e_s^n-e_s^{n-1})\\&\quad =(S^n+S^{n-1})(S^n-S^{n-1})e_r^n+(R^n+R^{n-1})(R^n-R^{n-1})e_r^n\\&\qquad +\left( (r^n-r^{n-1})(r^n+r^{n-1})+r^n(R^n-R^{n-1})+R^{n-1}(r^n-r^{n-1})\right) e_r^n \\&\qquad +\left( (R^{n-1})^2+(S^{n-1})^2+(r^{n-1})^2+r^{n-1}R^{n-1}\right) (e_r^n-e_r^{n-1})\\&\qquad + \left( r^n(S^n-S^{n-1})+S^{n-1}(r^n-r^{n-1})+r^n(s^n-s^{n-1})+s^{n-1}(r^n-r^{n-1})\right) e_s^n\\&\qquad +r^{n-1}(s^{n-1}+S^{n-1})(e_s^n-e_s^{n-1})\\&\quad =(S^n+S^{n-1})(S^n-S^{n-1})e_r^n+(R^n+R^{n-1}+r^n)\\&\qquad (R^n-R^{n-1})e_r^n+(r^n+r^{n-1}+R^{n-1})(r^n-r^{n-1})e_r^n \\&\qquad +\left( (R^{n-1})^2+(S^{n-1})^2+(r^{n-1})^2+r^{n-1}R^{n-1}\right) (e_r^n-e_r^{n-1})\\&\qquad + \left( r^n(S^n-S^{n-1})+(S^{n-1}+s^{n-1})(r^n-r^{n-1})+r^n(s^n-s^{n-1})\right) e_s^n\\&\qquad +r^{n-1}(s^{n-1}+S^{n-1})(e_s^n-e_s^{n-1})\\&\quad =(S^n+S^{n-1})(S^n-S^{n-1})e_r^n+(R^n+R^{n-1}+r^n)(R^n-R^{n-1})e_r^n\\&\qquad +(r^n+r^{n-1}+R^{n-1})(-(R^n-r^n)+(R^{n-1}-r^{n-1})+(R^n-R^{n-1})e_r^n \\&\qquad +\left( (R^{n-1})^2+(S^{n-1})^2+(r^{n-1})^2+r^{n-1}R^{n-1}\right) (e_r^n-e_r^{n-1})\\&\qquad + (r^n(S^n-S^{n-1})+(S^{n-1}+s^{n-1})(R^n-R^{n-1}-(R^n-r^n)+R^{n-1}-r^{n-1})\\&\qquad +r^n(S^n-S^{n-1}-(S^n-s^n)+S^{n-1}\\&\qquad -s^{n-1}))e_s^n+r^{n-1}(s^{n-1}+S^{n-1})(e_s^n-e_s^{n-1})\\&\quad =(S^n+S^{n-1})(S^n-S^{n-1})e_r^n\\&\qquad +(R^n+2R^{n-1}+2r^n+r^{n-1})(R^n-R^{n-1})e_r^n\\&\qquad -(r^n+r^{n-1}+R^{n-1})(e_r^n-e_r^{n-1})e_r^n\\&\qquad +\left( (R^{n-1})^2+(S^{n-1})^2+(r^{n-1})^2+r^{n-1}R^{n-1}\right) (e_r^n-e_r^{n-1})\\&\qquad +2r^n(S^n-S^{n-1})e_s^n+(S^{n-1}\\&\qquad +s^{n-1})(R^n-R^{n-1})e_s^n-(S^{n-1}+s^{n-1})(e_r^n-e_r^{n-1})e_s^n\\&\qquad -r^n(e_s^n-e_s^{n-1})e_s^n+r^{n-1}(s^{n-1}+S^{n-1})(e_s^n-e_s^{n-1})\\&\quad =((S^{n-1})^2+(R^{n-1})^2+r^{n-1}R^{n-1}+(r^{n-1})^2-e_r^n(R^{n-1}+r^n+r^{n-1})\\&\qquad -e_s^n(S^{n-1}+s^{n-1}))(e_r^{n}-e_r^{n-1})\\&\qquad +(r^{n-1}(S^{n-1}+s^{n-1})-r^{n}e_s^n)(e_s^n-e_s^{n-1})\\&\qquad +((S^n+S^{n-1})(S^n-S^{n-1})+(2r^n+r^{n-1}+2R^{n-1}+R^n)(R^n-R^{n-1}))e_r^n\\&\qquad +\left( 2r^n(S^{n}-S^{n-1})+(S^{n-1}+s^{n-1})(R^{n}-R^{n-1})\right) e_s^n, \end{aligned}$$

which completes the details of the expression for \(Z_{41}\).

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Li, Y., Shi, H. & Zhong, X. Local Discontinuous Galerkin Methods with Multistep Implicit–Explicit Time Discretization for Nonlinear Schrödinger Equations. J Sci Comput 101, 4 (2024). https://doi.org/10.1007/s10915-024-02647-6

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10915-024-02647-6

Keywords

Mathematics Subject Classification