Skip to main content
SpringerLink
Log in

Topological Derivatives of Shape Functionals. Part II: First-Order Method and Applications

  • Invited Paper
  • Published:
Journal of Optimization Theory and Applications Aims and scope Submit manuscript

Abstract

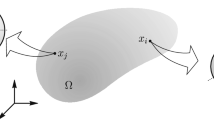
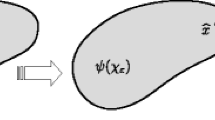
The framework of topological sensitivity analysis in singularly perturbed geometrical domains, presented in the first part of this series of review papers, allows the asymptotic expansion of a given shape functional with respect to a small parameter that measures the size of singular domain perturbations, such as holes, cavities, inclusions, source terms and cracks. This new concept in shape sensitivity analysis generalizes the shape derivatives from the domain boundary to its interior for admissible domains in two and three spatial dimensions. Therefore, the concept of topological derivative is a powerful tool for solving shape–topology optimization problems. There are now applications of topological derivative in many different fields of engineering and physics, such as shape and topology optimization in structural mechanics, inverse problems for partial differential equations, image processing, multiscale material design and mechanical modeling including damage and fracture evolution phenomena. In this second part of the review, a topology optimization algorithm based on first-order topological derivatives is presented. The appropriate level-set domain representation method is employed within the iterations in order to design an optimal shape–topology local solution. The algorithm is successfully used for numerical solution of a wide class of shape–topology optimization problems.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17

Similar content being viewed by others

References

  1. Novotny, A.A., Sokołowski, J., Żochowski, A.: Topological derivatives of shape functionals. Part I: theory in singularly perturbed geometrical domains. J. Optim. Theory Appl. 180(2), 1–30 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  2. Amstutz, S., Andrä, H.: A new algorithm for topology optimization using a level-set method. J. Comput. Phys. 216(2), 573–588 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  3. Novotny, A.A., Sokołowski, J.: Topological Derivatives in Shape Optimization. Interaction of Mechanics and Mathematics. Springer, Berlin (2013)

    Book  MATH  Google Scholar 

  4. Amstutz, S.: Analysis of a level set method for topology optimization. Optim. Methods Softw. 26(4–5), 555–573 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  5. Amigo, R.C.R., Giusti, S., Novotny, A.A., Silva, E.C.N., Sokolowski, J.: Optimum design of flextensional piezoelectric actuators into two spatial dimensions. SIAM J. Control Optim. 52(2), 760–789 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  6. Amstutz, S., Giusti, S.M., Novotny, A.A., de Souza Neto, E.A.: Topological derivative for multi-scale linear elasticity models applied to the synthesis of microstructures. Int. J. Numer. Methods Eng. 84, 733–756 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  7. Amstutz, S., Novotny, A.A.: Topological optimization of structures subject to von Mises stress constraints. Struct. Multidiscip. Optim. 41(3), 407–420 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  8. Amstutz, S., Novotny, A.A., de Souza Neto, E.A.: Topological derivative-based topology optimization of structures subject to Drucker–Prager stress constraints. Comput. Methods Appl. Mech. Eng. 233–236, 123–136 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  9. Lopes, C.G., dos Santos, R.B., Novotny, A.A.: Topological derivative-based topology optimization of structures subject to multiple load-cases. Latin Am. J. Solids Struct. 12, 834–860 (2015)

    Article  Google Scholar 

  10. Lopes, C.G., Novotny, A.A.: Topology design of compliant mechanisms with stress constraints based on the topological derivative concept. Struct. Multidiscip. Optim. 54(4), 737–746 (2016)

    Article  MathSciNet  Google Scholar 

  11. Giusti, S., Mróz, Z., Sokolowski, J., Novotny, A.: Topology design of thermomechanical actuators. Struct. Multidiscip. Optim. 55, 1575–1587 (2017)

    Article  MathSciNet  Google Scholar 

  12. Sá, L.F.N., Amigo, R.C.R., Novotny, A.A., Silva, E.C.N.: Topological derivatives applied to fluid flow channel design optimization problems. Struct. Multidiscip. Optim. 54(2), 249–264 (2016)

    Article  MathSciNet  Google Scholar 

  13. Torii, A.J., Novotny, A.A., Santos, R.B.: Robust compliance topology optimization based on the topological derivative concept. Int. J. Numer. Methods Eng. 106(11), 889–903 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  14. Allaire, G., de Gournay, F., Jouve, F., Toader, A.M.: Structural optimization using topological and shape sensitivity via a level set method. Control Cybern. 34(1), 59–80 (2005)

    MathSciNet  MATH  Google Scholar 

  15. Burger, M., Hackl, B., Ring, W.: Incorporating topological derivatives into level set methods. J. Comput. Phys. 194(1), 344–362 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  16. Eschenauer, H.A., Kobelev, V.V., Schumacher, A.: Bubble method for topology and shape optimization of structures. Struct. Optim. 8(1), 42–51 (1994)

    Article  Google Scholar 

  17. Otomori, M., Yamada, T., Izui, K., Nishiwaki, S.: Matlab code for a level set-based topology optimization method using a reaction diffusion equation. Struct. Multidiscip. Optim. 51(5), 1159–1172 (2015)

    Article  MathSciNet  Google Scholar 

  18. Sokołowski, J., Żochowski, A.: On the topological derivative in shape optimization. SIAM J. Control Optim. 37(4), 1251–1272 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  19. Bojczuk, D., Mróz, Z.: Topological sensitivity derivative and finite topology modifications: application to optimization of plates in bending. Struct. Multidiscip. Optim. 39(1), 1–15 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  20. Giusti, S.M., Novotny, A.A., Sokołowski, J.: Topological derivative for steady-state orthotropic heat diffusion problem. Struct. Multidiscip. Optim. 40(1), 53–64 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  21. Kobelev, V.: Bubble-and-grain method and criteria for optimal positioning inhomogeneities in topological optimization. Struct. Multidiscip. Optim. 40(1–6), 117–135 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  22. Leugering, G., Sokołowski, J.: Topological derivatives for elliptic problems on graphs. Control Cybern. 37, 971–998 (2008)

    MATH  Google Scholar 

  23. Novotny, A.A., Feijóo, R.A., Padra, C., Taroco, E.: Topological sensitivity analysis. Comput. Methods Appl. Mech. Eng. 192(7–8), 803–829 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  24. Novotny, A.A., Feijóo, R.A., Padra, C., Taroco, E.: Topological derivative for linear elastic plate bending problems. Control Cybern. 34(1), 339–361 (2005)

    MATH  Google Scholar 

  25. Novotny, A.A., Feijóo, R.A., Taroco, E., Padra, C.: Topological sensitivity analysis for three-dimensional linear elasticity problem. Comput. Methods Appl. Mech. Eng. 196(41–44), 4354–4364 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  26. Turevsky, I., Gopalakrishnan, S.H., Suresh, K.: An efficient numerical method for computing the topological sensitivity of arbitrary-shaped features in plate bending. Int. J. Numer. Methods Eng. 79(13), 1683–1702 (2009)

    Article  MATH  Google Scholar 

  27. Allaire, G.: Conception Optimale de Structures, Mathématiques et Applications, vol. 58. Springer, Berlin (2007)

    MATH  Google Scholar 

  28. Bendsøe, M.P., Sigmund, O.: Topology Optimization. Theory, Methods and Applications. Springer, Berlin (2003)

    MATH  Google Scholar 

  29. Eschenauer, H.A., Olhoff, N.: Topology optimization of continuum structures: a review. Appl. Mech. Rev. 54(4), 331–390 (2001)

    Article  Google Scholar 

  30. Campeão, D.E., Giusti, S.M., Novotny, A.A.: Topology design of plates consedering different volume control methods. Eng. Comput. 31(5), 826–842 (2014)

    Article  Google Scholar 

  31. Garreau, S., Guillaume, P., Masmoudi, M.: The topological asymptotic for PDE systems: the elasticity case. SIAM J. Control Optim. 39(6), 1756–1778 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  32. Giusti, S.M., Ferrer, A., Oliver, J.: Topological sensitivity analysis in heterogeneous anisotropic elasticity problem. Theoretical and computational aspects. Comput. Methods Appl. Mech. Eng. 311, 134–150 (2016)

    Article  MathSciNet  Google Scholar 

  33. Lewinski, T., Sokołowski, J.: Energy change due to the appearance of cavities in elastic solids. Int. J. Solids Struct. 40(7), 1765–1803 (2003)

    Article  MATH  Google Scholar 

  34. Sokołowski, J., Żochowski, A.: Topological derivatives of shape functionals for elasticity systems. Mech. Struct. Mach. 29(3), 333–351 (2001)

    MathSciNet  MATH  Google Scholar 

  35. Amstutz, S., Van Goethem, N.: Topology optimization methods with gradient-free perimeter approximation. Inverse Probl. Imaging 14(3), 401–430 (2012)

    MathSciNet  MATH  Google Scholar 

  36. Ammari, H., Kang, H.: Polarization and Moment Tensors with Applications to Inverse Problems and Effective Medium Theory. Applied Mathematical Sciences, vol. 162. Springer, New York (2007)

    MATH  Google Scholar 

  37. Amstutz, S.: A penalty method for topology optimization subject to a pointwise state constraint. ESAIM: control. Optim. Calc. Var. 16(3), 523–544 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  38. Bruggi, M., Duysinx, P.: Topology optimization for minimum weight with compliance and stress constraints. Struct. Multidiscip. Optim. 46(3), 369–384 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  39. Emmendoerfer Jr., H., Fancello, E.A.: A level set approach for topology optimization with local stress constraints. Int. J. Numer. Methods Eng. 99, 129–156 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  40. Fancello, E.A.: Topology optimization of minimum mass design considering local failure constraints and contact boundary conditions. Struct. Multidiscip. Optim. 32, 229–240 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  41. Le, C., Norato, J., Bruns, T.: Stress-based topology optimization for continua. Struct. Multidiscip. Optim. 41, 605–620 (2010)

    Article  Google Scholar 

  42. Pereira, J.T., Fancello, E.A., Barcellos, C.S.: Topology optimization of continuum structures with material failure constraints. Struct. Multidiscip. Optim. 26(1–2), 50–66 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  43. Allaire, G., Jouve, F., Maillot, H.: Minimum stress optimal design with the level-set method. Eng. Anal. Boundary Elem. 32(11), 909–918 (2008)

    Article  MATH  Google Scholar 

  44. Burns, R.H., Crossley, F.R.E.: Kinetostatic synthesis of flexible link mechanisms. ASME Pap. 68(36), 29–49 (1964)

    Google Scholar 

  45. Cardoso, E.L., Fonseca, J.S.O.: Strain energy maximization approach to the design of fully compliant mechanisms using topology optimization. Latin Am. J. Solids Struct. 1, 263–275 (2004)

    Google Scholar 

  46. Lee, E., Gea, H.C.: A strain based topology optimization method for compliant mechanism design. Struct. Multidiscip. Optm. 49, 199–207 (2014)

    Article  MathSciNet  Google Scholar 

  47. Luo, J., Luo, Z., Chen, S., Tong, L., Wang, M.Y.: A new level set method for systematic design of hinge-free compliant mechanisms. Comput. Methods Appl. Mech. Eng. 198, 318–331 (2008)

    Article  MATH  Google Scholar 

  48. Meneghelli, L.R., Cardoso, E.L.: Design of compliant mechanisms with stress constraints using topology optimization. Optim. Struct. Compon. Adv. Struct. Mater. 43, 35–48 (2013)

    Article  MathSciNet  Google Scholar 

  49. Sigmund, O.: On the design of compliant mechanisms using topology optimization. Mech. Struct. Mach. Int. J. 25(4), 493–524 (1997)

    Article  Google Scholar 

  50. Amstutz, S., Ciligot-Travain, M.A.: A notion of compliance robustness in topology optimization. ESAIM: control. Optim. Calc. Var. 22(1), 64–87 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  51. Dunning, P., Kim, H.: Robust topology optimization: minimization of expected and variance of compliance. Am. Inst. Aeronaut. Astronaut. J. 51(11), 2656–2664 (2013)

    Article  Google Scholar 

  52. Guo, X., Bai, W., Zhang, W., Gao, X.: Confidence structural robust design and optimization under stiffness and load uncertainties. Comput. Methods Appl. Mech. Eng. 198(41–44), 3378–3399 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  53. Zhao, J., Wang, C.: Robust topology optimization under loading uncertainty based on linear elastic theory and orthogonal diagonalization of symmetric matrices. Comput. Methods Appl. Mech. Eng. 273, 204–218 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  54. Du, X., Chen, W.: Sequential optimization and reliability assessment method for efficient probabilistic design. ASME J. Mech. Des. 126(2), 225–233 (2004)

    Article  Google Scholar 

  55. Hlaváček, I., Novotny, A.A., Sokołowski, J., Żochowski, A.: On topological derivatives for elastic solids with uncertain input data. J. Optim. Theory Appl. 141(3), 569–595 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  56. Tu, J., Choi, K.K., Park, Y.H.: A new study on reliability-based design optimization. ASME J. Mech. Des. 121(4), 557–564 (1999)

    Article  Google Scholar 

  57. Guillaume, P., Idris, K.S.: Topological sensitivity and shape optimization for the Stokes equations. SIAM J. Control Optim. 43(1), 1–31 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  58. Amstutz, S.: The topological asymptotic for the Navier–Stokes equations. ESAIM: control. Optim. Calc. Var. 11(3), 401–425 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  59. Guillaume, P., Hassine, M.: Removing holes in topological shape optimization. ESAIM: control. Optim. Calc. Var. 14(1), 160–191 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  60. Duan, X., Li, F.: Material distribution resembled level set method for optimal shape design of Stokes flow. Appl. Math. Comput. 266, 21–30 (2015)

    MathSciNet  MATH  Google Scholar 

  61. Plotnikov, P., Sokołowski, J.: Compressible Navier–Stokes Equations. Theory and Shape Optimization. Springer, Basel (2012)

    Book  MATH  Google Scholar 

  62. Giusti, S.M., Novotny, A.A., de Souza Neto, E.A., Feijóo, R.A.: Sensitivity of the macroscopic elasticity tensor to topological microstructural changes. J. Mech. Phys. Solids 57(3), 555–570 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  63. Giusti, S.M., Novotny, A.A., de Souza Neto, E.A.: Sensitivity of the macroscopic response of elastic microstructures to the insertion of inclusions. Proc. R. Soc. A Math. Phys. Eng. Sci. 466, 1703–1723 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  64. Giusti, S.M., Novotny, A.A., de Souza Neto, E.A., Feijóo, R.A.: Sensitivity of the macroscopic thermal conductivity tensor to topological microstructural changes. Comput. Methods Appl. Mech. Eng. 198(5–8), 727–739 (2009)

    Article  MATH  Google Scholar 

  65. Méndez, C.G., Podestá, J.M., Lloberas-Valls, O., Toro, S., Huespe, A.E., Oliver, J.: Computational material design for acoustic cloaking. Int. J. Numer. Methods Eng. 112, 1353–1380 (2017)

    Article  MathSciNet  Google Scholar 

  66. Novotny, A.A., Sokołowski, J., de Souza Neto, E.A.: Topological sensitivity analysis of a multi-scale constitutive model considering a cracked microstructure. Math. Methods Appl. Sci. 33(5), 676–686 (2010)

    MathSciNet  MATH  Google Scholar 

  67. Sanchez-Palencia, E.: Non-homogeneous Media and Vibration Theory. Lecture Notes in Physics, vol. 127. Springer, Berlin (1980)

    MATH  Google Scholar 

  68. Germain, P., Nguyen, Q.S., Suquet, P.: Continuum thermodynamics. Trans. ASME J. Appl. Mech. 50(4), 1010–1020 (1983)

    Article  MATH  Google Scholar 

  69. Michel, J.C., Moulinec, H., Suquet, P.: Effective properties of composite materials with periodic microstructure: a computational approach. Comput. Methods Appl. Mech. Eng. 172(1–4), 109–143 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  70. Miehe, C., Schotte, J., Schröder, J.: Computational micro–macro transitions and overall moduli in the analysis of polycrystals at large strains. Comput. Mater. Sci. 16(1–4), 372–382 (1999)

    Article  Google Scholar 

  71. Amad, A.A.S., Loula, A.F.D., Novotny, A.A.: A new method for topology design of electromagnetic antennas in hyperthermia therapy. Appl. Math. Model. 42, 209–222 (2017)

    Article  MathSciNet  Google Scholar 

  72. Amstutz, S., Horchani, I., Masmoudi, M.: Crack detection by the topological gradient method. Control Cybern. 34(1), 81–101 (2005)

    MathSciNet  MATH  Google Scholar 

  73. Abda, A.B., Hassine, M., Jaoua, M., Masmoudi, M.: Topological sensitivity analysis for the location of small cavities in Stokes flow. SIAM J. Control Optim. 48, 2871–2900 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  74. Samet, B., Amstutz, S., Masmoudi, M.: The topological asymptotic for the Helmholtz equation. SIAM J. Control Optim. 42(5), 1523–1544 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  75. Feijóo, G.R.: A new method in inverse scattering based on the topological derivative. Inverse Probl. 20(6), 1819–1840 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  76. Amstutz, S., Dominguez, N.: Topological sensitivity analysis in the context of ultrasonic non-destructive testing. Eng. Anal. Bound. Elem. 32(11), 936–947 (2008)

    Article  MATH  Google Scholar 

  77. Carpio, A., Rapún, M.L.: Solving inhomogeneous inverse problems by topological derivative methods. Inverse Probl. 24(4), 045,014 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  78. Funes, J.F., Perales, J.M., Rapún, M.L., Vega, J.M.M.: Defect detection from multi-frequency limited data via topological sensitivity. J. Math. Imaging Vis. 55, 19–35 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  79. Guzina, B.B., Bonnet, M.: Small-inclusion asymptotic of misfit functionals for inverse problems in acoustics. Inverse Probl. 22(5), 1761–1785 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  80. Jleli, M., Samet, B., Vial, G.: Topological sensitivity analysis for the modified Helmholtz equation under an impedance condition on the boundary of a hole. J. Math. Pures Appl. 103, 557–574 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  81. Tokmashev, R., Tixier, A., Guzina, B.: Experimental validation of the topological sensitivity approach to elastic-wave imaging. Inverse Probl. 29, 125,005 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  82. Ammari, H., Garnier, J., Jugnon, V., Kang, H.: Stability and resolution analysis for a topological derivative based imaging functional. SIAM J. Control Optim. 50(1), 48–76 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  83. Guzina, B.B., Pourahmadian, F.: Why the high-frequency inverse scattering by topological sensitivity may work. Proc. R. Soc. A Math. Phys. Eng. Sci. 471(2179), 20150,187 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  84. Argatov, I.I.: AFM-based indentation stiffness tomography—an asymptotic model. J. Mech. Phys. Solids 70, 190–199 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  85. Bonnet, M.: Topological sensitivity for 3D elastodynamic and acoustic inverse scattering in the time domain. Comput. Methods Appl. Mech. Eng. 195(37–40), 5239–5254 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  86. Chikichev, I., Guzina, B.B.: Generalized topological derivative for the Navier equation and inverse scattering in the time domain. Comput. Methods Appl. Mech. Eng. 194, 4467–4484 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  87. Dominguez, N., Gibiat, V.: Non-destructive imaging using the time domain topological energy method. Ultrasonics 50(3), 367–372 (2010)

    Article  Google Scholar 

  88. Van Goethem, N., Novotny, A.A.: Crack nucleation sensitivity analysis. Math. Methods Appl. Sci. 33(16), 1978–1994 (2010)

    MathSciNet  MATH  Google Scholar 

  89. Ammari, H., Kang, H., Lee, H., Lim, J.: Boundary perturbations due to the presence of small linear cracks in an elastic body. J. Elast. 113, 75–91 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  90. Allaire, G., Jouve, F., Van Goethem, N.: Damage and fracture evolution in brittle materials by shape optimization methods. J. Comput. Phys. 230(12), 5010–5044 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  91. Xavier, M., Fancello, E.A., Farias, J.M.C., Goethem, N.V., Novotny, A.A.: Topological derivative-based fracture modelling in brittle materials: a phenomenological approach. Eng. Fract. Mech. 179, 13–27 (2017)

    Article  Google Scholar 

  92. Xavier, M., Novotny, A.A., Goethem, N.V.: A simplified model of fracking based on the topological derivative concept. Int. J. Solids Struct. 139–140, 211–223 (2018)

    Article  Google Scholar 

  93. Amstutz, S., Novotny, A.A., Van Goethem, N.: Minimal partitions and image classification using a gradient-free perimeter approximation. Inverse Probl. Imaging 8(2), 361–387 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  94. Auroux, D., Belaid, L.J., Masmoudi, M.: A topological asymptotic analysis for the regularized greylevel image classification problem. ESAIM Math. Model. Numer. Anal. 41, 607–625 (2007)

    Article  MATH  Google Scholar 

  95. Belaid, L.J., Jaoua, M., Masmoudi, M., Siala, L.: Application of the topological gradient to image restoration and edge detection. Eng. Anal. Bound. Elem. 32(11), 891–899 (2008)

    Article  MATH  Google Scholar 

  96. Hintermüller, M.: Fast level set based algorithms using shape and topological sensitivity. Control Cybern. 34(1), 305–324 (2005)

    MathSciNet  MATH  Google Scholar 

  97. Hintermüller, M., Laurain, A.: Multiphase image segmentation and modulation recovery based on shape and topological sensitivity. J. Math. Imaging Vis. 35, 1–22 (2009)

    Article  MathSciNet  Google Scholar 

  98. Larrabide, I., Feijóo, R.A., Novotny, A.A., Taroco, E.: Topological derivative: a tool for image processing. Comput. Struct. 86(13–14), 1386–1403 (2008)

    Article  Google Scholar 

  99. Drogoul, A., Aubert, G.: The topological gradient method for semi-linear problems and application to edge detection and noise removal. Inverse Probl. Imaging 10(1), 51–86 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  100. Muszkieta, M.: A variational approach to edge detection. Inverse Probl. Imaging 10(2), 499–517 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  101. Novotny, A.A., Sokołowski, J., Żochowski, A.: Topological derivatives of shape functionals. Part III: second order method and applications. J. Optim. Theory Appl. 181(1), 1–22 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  102. Amstutz, S., Bonnafé, A.: Topological derivatives for a class of quasilinear elliptic equations. J. Math. Pures Appl. 107, 367–408 (2017)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

This research was partly supported by CNPq (Brazilian Research Council), CAPES (Brazilian Higher Education Staff Training Agency) and FAPERJ (Research Foundation of the State of Rio de Janeiro). The support is gratefully acknowledged. We would also like to thank Samuel Amstutz, Sebastián Giusti, Nicolas Van Goethem, Eduardo Neto, Luís Fernando Sá, Emílio Silva, André Torii and the former students Alan Amad, Cinthia Lopes, Vitor Sales, Renatha Santos and Marcel Xavier.

Author information

Authors and Affiliations

  1. Laboratório Nacional de Computação Científica LNCC/MCT, Coordenação de Matemática Aplicada e Computacional, Av. Getúlio Vargas 333, Petrópolis, RJ, 25651-075, Brazil

    Antonio André Novotny

  2. Institut Élie Cartan, UMR 7502 Laboratoire de Mathématiques, Université de Lorraine, Nancy 1, B.P. 239, 54506, Vandoeuvre Lès Nancy Cedex, France

    Jan Sokołowski

  3. Systems Research Institute of the Polish Academy of Sciences, ul. Newelska 6, 01-447, Warsaw, Poland

    Jan Sokołowski & Antoni Żochowski

Authors
  1. Antonio André Novotny
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jan Sokołowski
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Antoni Żochowski
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Jan Sokołowski.

Additional information

Communicated by Marc Bonnet.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Novotny, A.A., Sokołowski, J. & Żochowski, A. Topological Derivatives of Shape Functionals. Part II: First-Order Method and Applications. J Optim Theory Appl 180, 683–710 (2019). https://doi.org/10.1007/s10957-018-1419-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10957-018-1419-x

Keywords

Mathematics Subject Classification

Search

Navigation