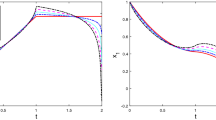
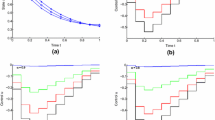
Abstract
This paper considers an optimal control problem governed by nonlinear fractional-order systems with multiple time-varying delays and subject to canonical constraints, where the fractional-order derivatives are expressed in the Caputo sense. To solve the problem by discretization scheme, an explicit numerical integration technique is proposed for solving the fractional-order system, and the trapezoidal rule is introduced to approximate the cost functional. Then, the gradients of the resulting cost and constraint functions are derived. On the basis of this result, we develop a gradient-based optimization algorithm to numerically solve the discretized problem. Finally, numerical results of several non-trivial examples are provided to illustrate the applicability and effectiveness of the proposed algorithm.





Similar content being viewed by others
References
Almeida, R., Torres, D.: A discrete method to solve fractional optimal control problems. Nonlinear Dyn. 80, 1811–1816 (2015)
Baleanu, D., Machado, J., Luo, A.: Fractional Dynamics and Control. Springer, New York (2012)
Bergounioux, M., Bourdin, L.: Pontryagin maximum principle for general Caputo fractional optimal control problems with Bolza cost and terminal constraints. ESAIM Control Optim. Calc. Var. 26, 1–38 (2020)
Bonnans, J., Gilbert, J., Lemaréchal, C., Sagastizábal, C.: Numerical Optimization: Theoretical and Practical Aspects. Springer, Berlin (2006)
Chen, C., Sun, D., Chang, C.: Numerical solution of time-delayed optimal control problems by iterative dynamic programming. Optim. Control Appl. Methods 21, 91–105 (2000)
Chen, S., Soradi-zeid, S., Alipour, M., Chu, Y., Gómez-aguilar, J., Jahanshahi, H.: Optimal control of nonlinear time-delay fractional differential equations with Dickson polynomials. Fractals 29, 2150079 (2021)
Cresson, J.: Fractional Calculus in Analysis, Dynamics and Optimal Control. Nova Science Publishers, New York (2014)
Dadebo, S., Luus, R.: Optimal control of time-delay systems by dynamic programming. Optim. Control Appl. Methods 13, 29–41 (1992)
Doha, E., Bhrawy, A., Baleanu, D., Ezz-Eldien, S., Hafez, R.: An efficient numerical scheme based on the shifted orthonormal Jacobi polynomials for solving fractional optimal control problems. Adv. Differ. Equ. 2015, 15 (2015)
Gong, Z., Liu, C., Teo, K., Wang, S., Wu, Y.: Numerical solution of free final time fractional optimal control problems. Appl. Math. Comput. 405, 126270 (2021)
Hoseini, S., Marzban, H.: Costate computation by an adaptive pseudospectral method for solving optimal control with piecewise constant time lag. J. Optim. Theory Appl. 170, 735–755 (2016)
Kamocki, R., Majewski, M.: Fractional linear control systems with Caputo derivative and their optimization. Optim. Control Appl. Methods 36, 953–967 (2015)
Li, W., Wang, S., Rehbock, V.: A 2nd-order one-step numerical integration scheme for a fractional differential equation. Numer. Algebra Control Optim. 7, 273–287 (2017)
Li, W., Wang, S., Rehbock, V.: Numerical solution of fractional optimal control. J. Optim. Theory Appl. 180, 556–573 (2019)
Liu, C., Gong, Z., Yu, C., Wang, S., Teo, K.: Optimal control computation for nonlinear fractional time-delay systems with state inequality constraints. J. Optim. Theory Appl. (2021). https://doi.org/10.1007/s10957-021-01926-8
Lotfi, A., Dehghan, M., Yousefi, S.: A numerical technique for solving fractional optimal control problems. Comput. Math. Appl. 62, 1055–1067 (2011)
Marzban, H.R., Malakoutikhah, F.: Solution of delay fractional optimal control problems using a hybrid of block-pulse functions and orthonormal Taylor polynomials. J. Frankl. Inst. 356, 8182–8215 (2019)
Mashayekhi, S., Miles, P., Hussaini, M., Oates, W.: Fractional viscoelasticity in fractal and non-fractal media: theory, experimental validation, and uncertainty analysis. J. Mech. Phys. Solids 111, 134–156 (2018)
Meng, X., Wu, Y., Li, J.: Bifurcation analysis of a singular nutrient-plankton-fish model with taxation, protected zone and multiple delays. Numer. Algebra Control Optim. 10, 391–423 (2020)
Mu, P., Wang, L., Liu, C.: A control parameterization method to solve the fractional-order optimal control problem. J. Optim. Theory Appl. 187, 234–247 (2020)
Nemati, A., Yousefi, S., Soltanian, F., Ardabili, J.: An efficient numerical solution of fractional optimal control problems by using the Ritz method and Bernstein operational matrix. Asian J. Control 18, 2272–2282 (2016)
Rabiei, K., Ordokhani, Y., Babolian, E.: Fractional-order Boubaker functions and their applications in solving delay fractional optimal control problems. J. Vib. Control 24, 3370–3383 (2017)
Rakhshan, S., Effati, S.: Fractional optimal control problems with time-varying delay: a new delay fractional Euler–Lagrange equations. J. Frankl. Inst. 357, 5954–5988 (2020)
Richard, J.: Time-delay systems: an overview of some recent advances and open problems. Automatica 39, 1667–1694 (2003)
Sipahi, R., Vyhlidal, T., Niculescu, S., Pierdomenico, P.: Time Delay Systems: Methods, Applications and New Trends. Springer, Berlin (2012)
Toledo-Hernandez, R., Rico-Ramirez, V., Iglesias-Silva, G., Diwekar, U.: A fractional calculus approach to the dynamic optimization of biological reactive systems. Part I. Fractional models for biological reactions. Chem. Eng. Sci. 117, 217–228 (2014)
Treantǎ, S.: On controlled variational inequalities involving convex functionals. In: Proceedings of World Congress on Global Optimization, Metz, France, pp. 164–174 (2019)
Treantǎ, S.: On a modified optimal control problem with first-order PDE constraints and the associated saddle-point optimality criterion. Eur. J. Control 51, 1–9 (2020)
Treantǎ, S., Arana-Jiménez, M., Antczak, T.: A necessary and sufficient condition on the equivalence between local and global optimal solutions in variational control problems. Nonlinear Anal. 191, 11640 (2020)
Treantǎ, S., Singh, S.: Weak sharp solutions associated with a multidimensional variational-type inequality. Positivity 25, 329–351 (2021)
Tseng, C.: Design of FIR and IIR fractional order Simpson digital integrators. Signal Process. 87, 1045–1057 (2007)
Tuan, H., Trinh, H.: A qualitative theory of time delay nonlinear fractional-order systems. SIAM J. Control. Optim. 58, 1491–1518 (2020)
Wu, D., Bai, Y., Xie, F.: Time-scaling transformation for optimal control problem with time-varying delay. Discrete Contin. Dyn. Syst. Ser.-S 13, 1683–1695 (2020)
Yari, A.: Numerical solution for fractional optimal control problems by Hermite polynomials. J. Vib. Control 27, 698–716 (2020)
Zhang, X., Zhang, Y.: Fault-tolerant control against actuator failures for uncertain singular fractional order systems. Numer. Algebra Control Optim. 11, 1–12 (2021)
Acknowledgements
The first author is supported by the National Natural Science Foundation of China (Grant No. 11771008). The second author is supported by the Natural Science Foundation of Shandong Province, China (Grant No. ZR2019MA031). The third and fourth authors are supported by the Australian Research Council (Discovery Grant DP190103361).
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by Qamrul Hasan Ansari.
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Liu, C., Gong, Z., Teo, K.L. et al. Optimal Control of Nonlinear Fractional-Order Systems with Multiple Time-Varying Delays. J Optim Theory Appl 193, 856–876 (2022). https://doi.org/10.1007/s10957-021-01935-7
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10957-021-01935-7