Skip to main content

Advertisement

Springer Nature Link
Log in

Minimum Mixed Time–Energy Trajectory Planning of a Nonlinear Vehicle Subject to 2D Disturbances

  • Published:
Journal of Optimization Theory and Applications Aims and scope Submit manuscript

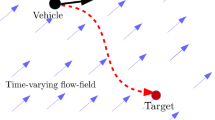
Abstract

The problem of a planar vehicle moving on a surface, such as aerial drones or small naval vessels, can be treated as a series of trajectory planning problems between way-points. While nominally the movement between each two fourth-dimensional points (positions and velocities) can be treated as a 1D projection of the movement on the vector connecting the two points, in the presence of arbitrary disturbance the full problem on a plane must be considered. The mixed minimum time–energy optimal solution is now dependent on the value and direction of the disturbance, which naturally affects the structure and completion of the movement task. In this work, we address the minimum time–energy problem of a movement on a 2D plane with quadratic drag, under norm state (velocity) and norm control (acceleration) constraints. The structure and properties of the optimal solution are found and analyzed. The Pontryagin’s maximum principle (PMP) with control and state constraints is utilized. Simulations supporting the results are provided and compared with those of the open-source academic optimal control solver Falcon.m.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17

Similar content being viewed by others

References

  1. Andreas, W., Biegler, L.T.: On the implementation of an interior-point filter line-search algorithm for large-scale nonlinear programming. Math. Program. 106(1), 25–57 (2006). https://doi.org/10.1007/s10107-004-0559-y

    Article  MathSciNet  MATH  Google Scholar 

  2. Ata, A.a.: Optimal trajectory planning of manipulators: A review. J. Eng. Sci. Technol. 2(1), 32–54 (2007). URL https://jestec.taylors.edu.my/Vol 2 Issue 1 April 07/32-54 Ata.pdf

  3. Ben-Asher, J.Z., Wetzler, M., Rimon, E.D., Diepolder, J.: Optimal trajectories for a mobile robot with bounded accelerations in the presence of a wall or a bounded obstacle. In: Editors for proceedings of the IEEE Mediterranean Conference on Control and Automation (MED), pp. 481–488 (2019). https://doi.org/10.1109/MED.2019.8798561

  4. Berger, A., Ioslovich, I., Gutman, P.O.: Time optimal trajectory planning with feedforward and friction compensation. In: Editors for proceedings of the American Control Conference (ACC), vol. 2015-July, pp. 4143–4148 (2015). https://doi.org/10.1109/ACC.2015.7171979

  5. Bertolazzi, E., Frego, M.: Semianalytical minimum-time solution for the optimal control of a vehicle subject to limited acceleration. Optim. Control Appl. Methods 39(2), 774–791 (2018). https://doi.org/10.1002/oca.2376

    Article  MathSciNet  MATH  Google Scholar 

  6. Browning, A.W.: A mathematical model to simulate small boat behaviour. Simulation 56(5), 329–336 (1991). https://doi.org/10.1177/003754979105600508

    Article  Google Scholar 

  7. Coles, A., Coles, A., Fox, M., Long, D.: COLIN: Planning with continuous linear numeric change. J. Artif. Intell. Res. 44, 1–96 (2012)

  8. Fernández-González, E., Williams, B., Karpas, E.: Scottyactivity: Mixed discrete-continuous planning with convex optimization. J. Artif. Intell. Res. 62, 579–664 (2018)

  9. Frego, M., Bertolazzi, E., Biral, F., Fontanelli, D., Palopoli, L.: Semi-analytical minimum time solutions with velocity constraints for trajectory following of vehicles. Automatica 86, 18–28 (2017). https://doi.org/10.1016/j.automatica.2017.08.020

    Article  MathSciNet  MATH  Google Scholar 

  10. Hartl, R.F., Sethi, S.P., Vickson, R.G.: A survey of the maximum principles for optimal control problems with state constraints. SIAM Rev. 37(2), 181–218 (1995). https://doi.org/10.1137/1037043

    Article  MathSciNet  MATH  Google Scholar 

  11. Ioslovich, I., Gutman, P.O., Berger, A., Moshenberg, S.: On energy-optimal and time-optimal precise displacement of rigid body with friction. J. Optim. Theory Appl. 172(2), 466–480 (2017). https://doi.org/10.1109/10.1007/s10957-016-0913-2

    Article  MathSciNet  MATH  Google Scholar 

  12. Ioslovich, I., Gutman, P.O.: Time-optimal control of wafer stage positioning using simplified models. Contemp. Math. 619, 99–107 (2014). https://doi.org/10.1090/conm/619/12386

    Article  MathSciNet  MATH  Google Scholar 

  13. Kirk, D.E.: Optimal Control Theory: An Introduction. Courier Corporation, New York (2012)

    Google Scholar 

  14. Krotov, V.F.: Global Methods in Optimal Control Theory. Marcel Dekker Inc, New York (1995)

    Google Scholar 

  15. Li, H.X., Williams, B.C.: Generative planning for hybrid systems based on Flow Tubes. In: Editors for proceedings of the International Conference on Automated Planning and Scheduling (ICAPS), pp. 206–213 (2008). URL https://www.aaai.org/Papers/ICAPS/2008/ICAPS08-026.pdf

  16. Pontryagin, L.S.: Mathematical Theory of Optimal Processes. CRC Press, New York (1987). https://doi.org/10.1201/9780203749319

  17. Raman-Nair, W., Gash, R., Sullivan, M.: Effect of wind and current on course control of a maneuvering vessel. In: 2014 Oceans - St. John’s, OCEANS 2014 (2014). https://doi.org/10.1109/OCEANS.2014.7003296

  18. Rieck, M., Bittner, M., Gruter, B., Diepolder, J.: Falcon.m (2016). URL http://www.fsd.mw.tum.de/software/falcon-m

  19. Taitler, A., Ioslovich, I., Gutman, P.O., Karpas, E.: Combined time and energy optimal trajectory planning with quadratic drag for mixed discrete-continuous task planning. Optimization 68(1), 125–143 (2019)

  20. Taitler, A., Ioslovich, I., Karpas, E., Gutman, P.O., Ben-Asher, J.Z.: Structural analysis of combined time and energy optimal trajectory planning with quadratic drag. In: Editors for proceedings of the IEEE Mediterranean Conference on Control and Automation (MED), pp. 153–158 (2019). https://doi.org/10.1080/10.1109/MED.2019.8798498. URL https://ieeexplore.ieee.org/document/8798498

  21. Taitler, A., Ioslovich, I., Karpas, E., Gutman, P.O.: Minimum time optimal control of second order system with quadratic drag and state constraints. In: Editors for proceedings of the IEEE Conference on Decision and Control (CDC), vol. 2019-Decem, pp. 523–528. IEEE, Nice (2019). https://doi.org/10.1109/CDC40024.2019.9029668. URL https://ieeexplore.ieee.org/document/9029668

  22. Taitler, A., Ioslovich, I., Karpas, E., Gutman, P.O.: Time optimal control for a non-linear planar vehicle subject to Disturbances. IFAC-PapersOnLine (in print) (2021). 1st IFAC Modeling, Estimation and Control Conference (MECC)

  23. Taitler, A., Ioslovich, I., Karpas, E., Gutman, P.O.: Time-Energy optimal control of a non-linear surface vehicle subject to disturbances. IFAC-PapersOnLine 54(14), 48–53 (2021). https://doi.org/10.1016/j.ifacol.2021.10.327. URL https://www.sciencedirect.com/science/article/pii/S2405896321017328. 3rd IFAC Conference on Modelling, Identification and Control of Nonlinear Systems (MICNON)

  24. Verscheure, D., Demeulenaere, B., Swevers, J., Schutter, J.D., Diehl, M.: Time-energy optimal path tracking for robots: a numerically efficient optimization approach. In: Editors for proceedings of the IEEE International Workshop on Advanced Motion Control (AMC), vol. 1, pp. 727–732. IEEE (2008). https://doi.org/10.1109/AMC.2008.4516157

  25. Yavin, Y., Frangos, C., Miloh, T.: Computation of feasible control trajectories for the navigation of a ship around an obstacle in the presence of a sea current. Math. Comput. Modell. 21(3), 99–117 (1995). https://doi.org/10.1016/0895-7177(94)00218-D

    Article  MathSciNet  MATH  Google Scholar 

  26. Zhao, Y., Tsiotras, P.: Speed profile optimization for optimal path tracking. In: Editors for proceedings of the American Control Conference (ACC), pp. 1171–1176 (2013). https://doi.org/10.1109/acc.2013.6579994

Download references

Acknowledgements

This work was supported by the PMRI—Peter Munk Research Institute—Technion.

Author information

Authors and Affiliations

  1. Technion–Institute of Technology, 3200003, Haifa, Israel

    Ayal Taitler, Ilya Ioslovich, Erez Karpas & Per-Olof Gutman

Authors
  1. Ayal Taitler
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Ilya Ioslovich
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Erez Karpas
    View author publications

    You can also search for this author inPubMed Google Scholar

  4. Per-Olof Gutman
    View author publications

    You can also search for this author inPubMed Google Scholar

Additional information

Communicated by Nikolai Osmolovskii.

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Data sharing is not applicable to this article as no datasets were generated or analyzed during the current study.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Taitler, A., Ioslovich, I., Karpas, E. et al. Minimum Mixed Time–Energy Trajectory Planning of a Nonlinear Vehicle Subject to 2D Disturbances. J Optim Theory Appl 192, 725–757 (2022). https://doi.org/10.1007/s10957-021-01990-0

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10957-021-01990-0

Keywords

Mathematics Subject Classification