Boundary Approximate Controllability under Positivity Constraints of Infinite-Dimensional Control Systems

Abstract

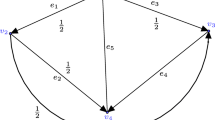
This paper focuses on boundary approximate controllability under positivity constraints of a wide range of infinite-dimensional control systems. We develop frequency-domain controllability criteria. Firstly, we derive a controllability result under positivity constraints on the control for such systems. Then, and more importantly, we provide a necessary and sufficient condition for controllability under positivity constraints on the control and the state. The obtained results are applied to the controllability of transportation and heat conduction networks. In particular, provided that the underlying graph is strongly connected, the controllability under positivity constraints on the control/state of transport network systems is fully characterized by a Kalman-type rank condition. For a system of heat equations with Robin boundary conditions on a path-like network, we establish approximate controllability under positivity state constraint with a single positive input through the starting node. However, we prove the lack of controllability under unilateral control constraint.

Appendix

Here, we provide an appendix on technical lemmas needed for the proof of Corollary 5.1.

The following result introduces a modification of the so-called Szász–Mirakjan operator, cf. [2].

Lemma .1

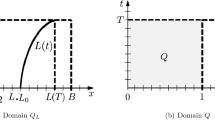
Let $f\in \mathcal {C}_b([0,+\infty )):=\{f\in {\mathcal {C}}([0,+\infty )):\;\exists \, \alpha \ge 0,\, \delta \ge 0\;\mathrm{such \, that }\; \vert f(x)\vert \le \delta e^{\alpha x} \}$ and define

$$\begin{aligned} \mathscr {M}_n(f;x):=e^{-n\varphi (x)}\sum _{k=0}^{\infty } f(\varphi ^{-1}(\tfrac{k}{n}))\frac{n^k}{k!}(\varphi (x))^k,\qquad n\ge 1,\; x\ge 0, \end{aligned}$$

(47)

where $\varphi (x)=\frac{1}{v}(1-x)$ for $x\in [0,1]$ and $\varphi (x)=0$ for $x\ge 1$. Then, the operators $\mathscr {M}_n$ are linear positive and for every $f\in \mathcal {C}_b([0,+\infty ))$ we have

$$\begin{aligned} \lim _{n\rightarrow +\infty }\mathscr {M}_n f=f,\qquad { \mathrm uniformly\, on} \;[0,1]. \end{aligned}$$

Proof

To prove our claim, we will use the Korovkin theorem, see, e.g., [2]. To this end, we have to prove that the operators $\mathscr {M}_n$ preserve the functions 1, $\varphi (x)$, $(\varphi (x))^2$. In fact, a simple computation shows

$$\begin{aligned} \mathscr {M}_n(1;x)&=1,\qquad \mathscr {M}_n(\varphi (x);x)= \varphi (x), \end{aligned}$$

and

$$\begin{aligned} \mathscr {M}_n((\varphi (x))^2;x)&= e^{-n\varphi (x)}\sum _{k=0}^{\infty } \frac{k^2}{n^2}\frac{n^k}{k!}(\varphi (x))^k\\&=(\varphi (x))^2 + \frac{1}{n}\varphi (x), \end{aligned}$$

where we have used the fact that $\varphi ^2(\varphi ^{-1}(x))=x^2$. Therefore, from [2, Theorem 4.1], we get that

$$\begin{aligned} \lim _{n\rightarrow +\infty }\mathscr {M}_n f=f \end{aligned}$$

uniformly on [0, 1]. $\square$

The last lemma at the hand, one can derive the following density result.

Lemma .2

Let $p\in [1,\infty )$ and $v>0$ be fixed. Then,

$$\begin{aligned} \overline{co}\left( e^{-\frac{n}{v}(1-.)},\qquad n\in {\mathbb {N}}\right) =L^{p}_+([0,1]. \end{aligned}$$

(48)

Proof

Let $p,q\in [1,\infty )$ with $\frac{1}{p}+\frac{1}{q}=1$ and let $g\in L^q([0,1])$ such that $\int _{0}^{1} e^{-\frac{n}{v}(1-x)}g(x)\, dx\, \ge 0$ for all $n\in {\mathbb {N}}$. Let $0\le f\in \mathcal {C}([0,1])$ and define the function

$$\begin{aligned} h(x):={\left\{ \begin{array}{ll} f(x),&{} x\in [0,1],\\ f(1),&{} t\ge 1. \end{array}\right. } \end{aligned}$$

Then, $0\le h\in \mathcal {C}_b([0,+\infty ))$ and

$$\begin{aligned}\ \int _{0}^{1} (\mathscr {M}_n h)(x)g(x)\,dx \,\ge 0. \end{aligned}$$

The continuity of f, Lemma .1 and the dominated convergence theorem further yield

$$\begin{aligned} \int _{0}^{1} f(x) g(x)\,dx\,\ge 0, \qquad \forall \, 0\le f\in \mathcal {C}([0,1]). \end{aligned}$$

Moreover, since the positive cone in $\mathcal {C}([0,1])$ is dense in $L^p_+([0,1])$, we get that

$$\begin{aligned} \int _{0}^{1} f(x) g(x)\,dx\,\ge 0, \qquad \forall f\in L^p_+([0,1]), \end{aligned}$$

and hence $g\ge 0$. $\square$