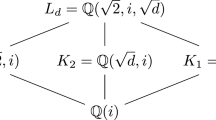Abstract
Let K be an imaginary cyclic quartic number field whose 2-class group is nontrivial, it is known that there exists at least one unramified quadratic extension F of K. In this paper, we compute the rank of the 2-class group of the field F.

Similar content being viewed by others
References
A. Azizi, I. Jerrari, M. Talbi, Unramified extensions of some cyclic quartic fields. Bol. Soc. Parana. Mat. (3s.) 36(1), 215–221 (2018)
A. Azizi, I. Jerrari, A. Zekhnini, M. Talbi, On the second 2-class group \({\rm Gal}(K_2^{(2)}/K)\) of some imaginary quartic cyclic number field \(K\). J. Number Theory 177, 562–588 (2017)
A. Azizi, M. Talbi, Capitulation des \(2\)-classes d’idéaux de certains corps biquadratiques cycliques. Acta Arith. 127, 231–248 (2007)
A. Azizi, M. Talbi, Capitulation dans certaines extensions non ramifiées de corps quartiques cycliques. Arch. Math. (BRNO) 44, 271–284 (2008)
A. Azizi, A. Zekhnini, M. Taous, Coclass of \({\rm Gal}({\rm k}^{(2)}_2/{\rm k})\) for some fields \({\rm k}=\mathbb{Q}\left(\sqrt{ p_1p_2q}, \sqrt{ -1}\right)\) with \(2\)-class groups of type \((2, 2, 2)\). J. Algebra Appl 15(2), 1650027 (2016)
A. Azizi, A. Zekhnini, M. Taous, Structure of \({\rm G}al({\rm k}^{(2)}_2/{\rm k})\) for some fields \({\rm k}=\mathbb{Q}(\sqrt{2p_1p_2}, i)\) with \(\mathbf{C}l_2({{\rm k}})\simeq (2, 2, 2)\). Abh. Math. Sem. Univ. Hambg. 84(2), 203–231 (2014)
A. Azizi, A. Zekhnini, M. Taous, Daniel C. Mayer, Principalization of \(2\)-class groups of type \((2, 2, 2)\) of biquadratic fields \({\mathbb{Q}}(\sqrt{ p_1p_2q}, i)\). Int. J. Number Theory 11(4), 1177–1215 (2015)
E. Brown, C.J. Parry, The \(2\)-class group of certain biquadratic number fields I. J. Reine Angew. Math. 295, 61–71 (1977)
P. Kaplan, Sur le \(2\)-groupe des classes d’idéaux des corps quadratiques. J. Reine Angew. Math. 283(284), 313–363 (1976)
R. Kučera, On the parity of the class number of biquadratic field. J. Number Theory 52, 43–52 (1995)
F. Lemmermeyer, Ideal class groups of cyclotomic number fields I. Acta Arith. 72, 4 (1995)
F. Lemmermeyer, On \(2\)-class field towers of some imaginary quadratic number fields. Abh. Math. Sem. Hambg. 67, 205–214 (1997)
Author information
Authors and Affiliations
Corresponding author
Additional information
This work is partially supported by CNRST (PBER) and ACSA laboratory (FSO-UMPO).
Rights and permissions
About this article
Cite this article
Azizi, A., Jerrari, I. & Talbi, M. On the rank of the 2-class group of an extension of degree 8 over \(\mathbb {Q}\). Period Math Hung 78, 128–134 (2019). https://doi.org/10.1007/s10998-018-0269-5
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10998-018-0269-5