Skip to main content
SpringerLink
Log in

S-box design based on optimize LFT parameter selection: a practical approach in recommendation system domain

  • Published:
Multimedia Tools and Applications Aims and scope Submit manuscript

Abstract

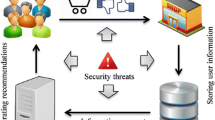
The strength of cryptography encryption is strongly depended upon the substitution box (S-box), hence highly non-linear S-box is appreciated in cryptography. In this work, we proposed a scheme to select the optimized values for control parameters (a, b, c, d) of linear fractional transform (LFT) to achieve an S-box with non-linearity of 112. For dynamic parameter values, we used location of chaotic sequences. The proposed s-box tested on different criteria such as non-linearity method (NL), probability methods (linear approximation (LP), differential approximation (DP), strictly avalanche criteria (SAC) and bit independence criteria (BIC). Our result shows that proposed s-box achieves better or equal to cryptographic strength as compared with state-of-the-art techniques. Moreover, we tested the proposed s-box based encryption method in recommendation system scenario with the idea of text-to-image conversion. The statistical analysis of this image encryption shows the bright prospects of the proposed method.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

References

  1. Abd-El-Atty B, El-Latif AAA, Venegas-Andraca SE (2019) An encryption protocol for NEQR images based on one-particle quantum walks on a circle. Quantum Inf Process 18(9):272

    Article  Google Scholar 

  2. Abdullah S, Amin NU (2015) Analysis of S-box image encryption based on generalized fuzzy soft expert set. Nonlinear Dyn 79:1679–1692

    Article  Google Scholar 

  3. Aboytes-González JA, Murguía JS, Mejía-Carlos M, González-Aguilar H, Ramírez-Torres MT (2018) Design of a strong S-box based on a matrix approach. Nonlinear Dyn 94:2003–2012

    Article  Google Scholar 

  4. Ahmad A, Mohammad T, Almimi HM (2014) Distributed text-to-image encryption algorithm. Int J Comput Appl 106:1

    Google Scholar 

  5. Al Solami E, Ahmad M, Volos C, Doja M, Beg M (2018) A new hyper chaotic system-based design for efficient bijective substitution-boxes. Entropy 20(7):525

    Article  Google Scholar 

  6. Amin M, El-Latif AAA (2010) Efficient modified RC5 based on chaos adapted to image encryption. J Electron Imaging 19(1):013012

    Article  Google Scholar 

  7. Belazi A, El-Latif AAA (2017) A simple yet efficient S-box method based on chaotic sine map. Optik 130:1438–1444

    Article  Google Scholar 

  8. Belazi A, El-Latif AAA, Rhouma R, Belghith S (2015) Selective image encryption scheme based on DWT, AES S-box and chaotic permutation. In: international wireless communications and Mobile computing conference (IWCMC) IEEE. pp. 606–610

  9. Belazi A, El-Latif AAA, Belghith S (2016) A novel image encryption scheme based on substitution-permutation network and chaos. Signal Process 128:155–170

    Article  Google Scholar 

  10. Belazi A, Khan M, El-Latif AAA, Belghith S (2017) Efficient cryptosystem approaches: S-boxes and permutation–substitution-based encryption. Nonlinear Dyn 87(1):337–361

    Article  Google Scholar 

  11. Belazi A, El-Latif AAA, Diaconu AV, Rhouma R, Belghith S (2017) Chaos-based partial image encryption scheme based on linear fractional and lifting wavelet transforms. Opt Lasers Eng 88:37–50

    Article  Google Scholar 

  12. Benrhouma O, Hermassi H, El-Latif AAA, Belghith S (2015) Cryptanalysis of a video encryption method based on mixing and permutation operations in the DCT domain. SIViP 9(6):1281–1286

    Article  Google Scholar 

  13. Bibi N, Farwa S, Muhammad N, Jahngir A, Usman M (2018) A novel encryption scheme for high-contrast image data in the Fresnelet domain. PLoS One 13

  14. Biham E, Shamir A (1991) Differential cryptanalysis of DES-like cryptosystems. J Cryptol 4:3–72

    Article  MathSciNet  MATH  Google Scholar 

  15. Çavuşoğlu Ü, Zengin A, Pehlivan I, Kaçar S (2017) A novel approach for strong S-box generation algorithm design based on chaotic scaled Zhongtang system. Nonlinear Dyn 87:1081–1094

    Article  MATH  Google Scholar 

  16. Cui L, Cao Y (2007) A new S-box structure named affine-power-affine. Int J Innov Comput Inf Control 3:751–759

    Google Scholar 

  17. Daemen J, Rijmen V (2002) The Design of Rijndael. Springer, Berlin Heidelberg. https://doi.org/10.1007/978-3-662-04722-4

    Book  MATH  Google Scholar 

  18. EL-Latif AAA, Abd-El-Atty B, Venegas-Andraca SE (2019) A novel image steganography technique based on quantum substitution boxes. Opt Laser Technol 116:92–102

    Article  Google Scholar 

  19. El-Latif AAA, Yan X, Li L, Wang N, Peng JL, Niu X (2013) A new meaningful secret sharing scheme based on random grids, error diffusion and chaotic encryption. Opt Laser Technol 54:389–400

    Article  Google Scholar 

  20. El-Latif AAA, Wang N, Peng JL, Li Q, Niu X (2013) A new encryption scheme for color images based on quantum chaotic system in transform domain. In: 5th International Conference on Digital Image Processing (ICDIP 2013). Int Soc Opt Photon 8878:88781S

    Google Scholar 

  21. El-Latif AAA, Li L, Wang N, Han Q, Niu X (2013) A new approach to chaotic image encryption based on quantum chaotic system, exploiting color spaces. Signal Process 93(11):2986–3000

    Article  Google Scholar 

  22. El-Latif AAA, Li L, Niu X (2014) A new image encryption scheme based on cyclic elliptic curve and chaotic system. Multimed Tools Appl 70(3):1559–1584

    Article  Google Scholar 

  23. El-Latif AAA, Abd-El-Atty B, Talha M (2017) Robust encryption of quantum medical images. IEEE Access 6:1073–1081

    Article  Google Scholar 

  24. Farwa S, Shah T, Idrees L (2016) A highly nonlinear S-box based on a fractional linear transformation. Springerplus 5:1658

    Article  Google Scholar 

  25. Hussain I, Shah T, Gondal MA, Mahmood H (2012) Generalized Majority Logic Criterion to Analyze the Statistical Strength of S-Boxes. Z Naturforsch A 67

  26. Hussain I, Shah T, Mahmood H, Gondal MA (2013) A projective general linear group based algorithm for the construction of substitution box for block ciphers. Neural Comput Appl 22:1085–1093

    Article  Google Scholar 

  27. Hussain I, Anees A, AlKhaldi AH, Algarni A, Aslam M (2018) Construction of chaotic quantum magnets and matrix Lorenz systems S-boxes and their applications. Chin J Phys 56:1609–1621

    Article  Google Scholar 

  28. Jhajharia S (2016) Using cell division for text to image encryption. In: 10th IEEE international conference on intelligent systems and control (ISCO), IEEE. pp. 1–7

  29. Khan M, Asghar Z (2018) A novel construction of substitution box for image encryption applications with Gingerbreadman chaotic map and S8 permutation. Neural Comput Appl 29:993–999

    Article  Google Scholar 

  30. Khan MA, Ali A, Jeoti V, Manzoor S (2018) A Chaos-based substitution box (S-box) design with improved differential approximation probability (DP). Iran J Sci Technol Trans Electr Eng 42:219–238

    Article  Google Scholar 

  31. Khan MF, Ahmed A, Saleem K (2019). A novel cryptographic substitution box design using Gaussian distribution. IEEE Access

  32. Kim J, Phan RC-W (2009) Advanced differential-style cryptanalysis of the NSA’s skipjack block cipher. Cryptologia 33:246–270

    Article  MATH  Google Scholar 

  33. Lam S, Frankowski D, Riedl J (2006) Do you trust your recommendations? An exploration of security and privacy issues in recommender systems. Emerg Trends Inf Commun Secur 2006:14–29

    Article  Google Scholar 

  34. Lambić D (2014) A novel method of S-box design based on chaotic map and composition method. Chaos, Solitons Fractals 58:16–21

    Article  MATH  Google Scholar 

  35. Lambić D (2017) A novel method of S-box design based on discrete chaotic map. Nonlinear Dyn 87(4):2407–2413

    Article  MathSciNet  Google Scholar 

  36. Liu H, Kadir A, Niu Y (2014) Chaos-based color image block encryption scheme using S-box. AEU-Int J Electron Commun 68(7):676–686

    Article  Google Scholar 

  37. Liu G, Yang W, Liu W, Dai Y (2015) Designing S-boxes based on 3-D four-wing autonomous chaotic system. Nonlinear Dyn 82(4):1867–1877

    Article  MathSciNet  Google Scholar 

  38. Matsui M (1993) Linear cryptanalysis method for DES cipher. In: Adv. Cryptol. — EUROCRYPT ‘93. Springer, Berlin Heidelberg, pp 386–397

    Google Scholar 

  39. Özkaynak F, Çelik V, Özer AB (2017) A new S-box construction method based on the fractional-order chaotic Chen system. Sig Image Video Process 11:659–664

    Article  Google Scholar 

  40. Peng J, El-Latif AAA, Belazi A, Kotulski Z (2017) Efficient chaotic nonlinear component for secure cryptosystems. In: 9th International Conference on Ubiquitous and Future Networks (ICUFN), IEEE. pp. 989–993

  41. Picek S, Batina L, Jakobović D, Ege B, Golub M (2014) S-box, SET, match: A toolbox for S-box analysis. pp. 140–149

  42. Tran MT, Bui DK, Duong AD (2008) Gray S-box for advanced encryption standard. Int. Conf. Comput. Intell. Secur., IEEE. pp. 253–258

  43. ul Islam F, Liu G (2017) Designing s-box based on 4D-4wing hyperchaotic system. 3D Res. 8:9.

  44. Ullah A, Jamal SS, Shah T (2018) A novel scheme for image encryption using substitution box and chaotic system. Nonlinear Dyn 91:359–370

    Article  MathSciNet  Google Scholar 

  45. Wang Y, Xie Q, Wu Y, Du B (2009) A software for S-box performance analysis and test. Int. Conf. Electron. Commer. Bus. Intell., IEEE. pp. 125–128

  46. Wang C, Zheng Y, Jiang J, Ren K (2018) Toward privacy-preserving personalized recommendation services. Engineering

  47. Webster AF, Tavares SE (1985) On the design of S-boxes. In: Adv. Cryptol. — CRYPTO ‘85 Proc. Springer, Berlin Heidelberg, pp 523–534

    Google Scholar 

  48. Xu K, Yan Z (2016) Privacy protection in mobile recommender systems: a survey. In: Wang G, Ray I, Alcaraz Calero J, Thampi S (eds) Security, Privacy, and Anonymity in Computation, Communication, and Storage. SpaCCS 2016. Lecture notes in computer science, vol 10066. Springer, Cham, pp 305–318

    Google Scholar 

  49. Ye T, Zhimao L (2018) Chaotic S-box: six-dimensional fractional Lorenz–Duffing chaotic system and O-shaped path scrambling. Nonlinear Dyn 94:2115–2126

    Article  Google Scholar 

  50. Yi X, Cheng SX, You XH, Lam KY (1997) A method for obtaining cryptographically strong 8×8 S-boxes. In: GLOBECOM 97. IEEE Glob. Telecommun. Conf. Conf. Rec., IEEE. pp 689–693

  51. Zaghloul A, Zhang T, Amin M, El-Latif AAA (2014l) Color encryption scheme based on adapted quantum logistic map. In 6th International Conference on Digital Image Processing (ICDIP 2014). Int Soc Opt Photon 9159:915922

    Google Scholar 

  52. Zhang TJ, El-Latif AAA, Amin M, Zaghloul A (2014) Diffusion-substitution mechanism for color image encryption based on multiple chaotic systems. Adv Mater Res Trans Tech Publ 981:327–330

    Google Scholar 

  53. Zhang X, Zhao Z, Wang J (2014) Chaotic image encryption based on circular substitution box and key stream buffer. Signal Process Image Commun 29(8):902–913

    Article  Google Scholar 

  54. Zhang T, El-Fatyany A, Li L, Amin M, El-Latif AAA (2015) Secret sharing-based chaotic image encryption. Int J Secur Appl 9(7):217–224

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Computer Science, COMSATS University Islamabad, Islamabad, Pakistan

    Saira Beg, Adeel Anjum, Mansoor Ahmad, Abid Khan & Ahmed Khan

  2. FAST-National University of Computer and Emerging Sciences, Islamabad, Pakistan

    Naveed Ahmad

  3. Federal Urdu University of Arts, Science & Technology, Islamabad, Pakistan

    Faisal Baig

Authors
  1. Saira Beg
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Naveed Ahmad
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Adeel Anjum
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Mansoor Ahmad
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Abid Khan
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. Faisal Baig
    View author publications

    You can also search for this author in PubMed Google Scholar

  7. Ahmed Khan
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Ahmed Khan.

Ethics declarations

Conflict of interest

Mentioned authors have no conflict of interest in this article.

Ethical approval

This article does not contain any studies with human participants or animals performed by any of the authors.

Additional information

Publisher’s note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Beg, S., Ahmad, N., Anjum, A. et al. S-box design based on optimize LFT parameter selection: a practical approach in recommendation system domain. Multimed Tools Appl 79, 11667–11684 (2020). https://doi.org/10.1007/s11042-019-08464-6

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11042-019-08464-6

Keywords

Search

Navigation