Abstract
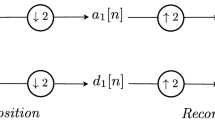
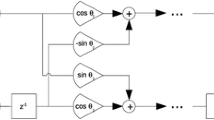
The design and analysis of two-channel two-dimensional (2D) nonseparable nearly-orthogonal symmetric wavelet filter banks with quincunx decimation is studied. The basic idea is to impose multiple zeros at the aliasing frequency to a symmetric filter and minimize the deviation of the filter satisfying the orthogonal condition to obtain a nearly-orthogonal FIR filter bank. Since multiple zeros are imposed, a scaling function may be generated from the minimized filter. With this filter, a semi-orthogonal filter bank is constructed. Methods for analyzing the correlation of the semi-orthogonal filter banks are proposed. The integer translates of the wavelet and scaling function are nearly-orthogonal. The integer translates of the wavelet at different scale are completely orthogonal. The semi-orthogonal filter bank can be efficiently implemented using the corresponding nearly-orthogonal FIR filter bank.
Similar content being viewed by others
Explore related subjects
Discover the latest articles, news and stories from top researchers in related subjects.References
Adelson, E. H., Simoncelli, E., & Hingorani, R. (1987). Orthogonal pyramid transform for image coding. In Proceedings of SPIE (Vol. 845, pp. 50–58). Cambridge, MA.
Antonini M., Barlaud M., Mathieu P., Daubechies I. (1992) Image coding using wavelet transform. IEEE Transactions on Image Processing 1(2): 205–220
Bamberger R., Smith M. (1992) A filter bank for the directional decomposition of images: Theory and design. IEEE Transactions on Signal Processing 40(4): 882–893
Basu S. (1998) Multidimensional filter banks and wavelets, a system theoretic perspective. Journal of Franklin Institute 335B(8): 1367–1409
Chen T., Vaidyanathan P. (1993) Multidimensional multirate filters and filter banks derived from one-dimensional filters. IEEE Transactions on Signal Processing 41(5): 1749–1765
Chui C. K. (1992) An introduction to wavelets. Academic Press, New York
Cohen A., Daubechies I. (1993) Non-separable bidimensional wavelet bases. Revista Matemd´ftica Iberoamericana 9(1): 51–138
Crochiere R. E., Rabiner R. L. (1983) Multirate digital signal processing. Prentice-Hall, Englewood Cliffs, NJ
da Silva E. A. B., Ghanbari M. (1996) On the performance of linear phase wavelet transforms in low bit-rate image coding. IEEE Transactions on Image Processing 5(5): 689–704
Daubechies I. (1986) Orthogonal bases of compactly supported wavelets. Communications on Pure and Applied Mathematics XLI: 909–996
Dumitrescu, B. (2010). A moulding technique for the design of 2-D nearly orthogonal filter banks. IEEE Signal Processing Letters, 17(3).
Johnston, J. D. (1980). A filter family design for use in quadrature mirror filter banks. In Proceedings of ICASSP (pp. 291–294).
Karlsson G., Vetterli M. (1990) Theory of two- dimensional multirate filter banks. IEEE Transactions on Acoustic, Speech, Signal Processing 38(6): 925–937
Kovacevic J., Vetterli M. (1992) Non-separable multidimensional filter banks and wavelet bases for R n. IEEE Transactions on Information Theory 38: 533–555
Kovacevic J., Vetterli M. (1997) Nonseparable two- and three-dimensional wavelets. IEEE Transactions on Signal Processing 43(5): 1269–1273
Mallat S. (1989) A theory for multiresolution signal decomposition: The wavelet representation. IEEE Transactions on Pattern Analysis and Machine Intelligence 11(7): 674–693
Marshall T. G. (1997) Zero-phase filter bank and wavelet code r matrices: Properties, triangular decompositions, and a fast algorithm. Multidimensional Systems and Signal Processing 8(1–2): 71–88
Shapiro J. M. (1993) Embedded image coding using zerotrees of wavelet coefficients. IEEE Transactions on Signal Processing 41(12): 3445–3462
Simoncelli E., Adelson E. (1990) Non-separable extensions of quadrature mirror filters to multiple dimensions. Proceedings of IEEE 78(4): 652–663
Sriram P., Maicellin M. W. (1995) Image coding using wavelet transforms and entropy-constrained trellis-coded quantization. IEEE Transactions on Image Processing 4(6): 725–733
Stanhill D., Zeevi Y. Y. (1998) Two-dimensional orthogonal filter banks and wavelets with linear phase. IEEE Transactions on Signal Processing 46(1): 183–190
Strang G. (1989) Wavelet and dilation equation. SIAM Journal of Mathmatics Analysis 31: 614–627
Tay D., Kingsbury N. (1993) Flexible design of multidimensional perfect reconstruction FIR 2-band filters using transformations of variables. IEEE Transactions on Image Processing 2: 466–480
Vaidyanathan P. P. (1993) Multirate systems and filter banks. Prentice Hall PTR, Englewood Cliffs, New Jersey
Venkataraman S., Levy B. (1994) State space representations of 2-D FIR lossless transfer matrices. IEEE Transactions on Circuits System II: Analog and Digital Signal Processing 41(2): 117–132
Vetterli M., Kovacevic J. (1995) Wavelets and subband coding. Prentice Hall PTR, Englewood Cliffs, New Jersey
Villasenor J. D., Belzer B., Liao J. (1995) Wavelet filter evaluation for image compression. IEEE Transactions on Image Processing 4(8): 1053–1060
Viscito E., Allebach J. (1991) The analysis and design of multidimensional FIR perfect reconstruction filter banks for arbitrary sampling lattice. IEEE Transactions on Circuits System (Video Technol.) 38: 29–42
Zhao, Y., & Swamy, M. N. S. (1999). A technique for designing new biorthogonal filters and its application to image compression. Electronics Letters, 35(18).
Zhao Y., Swamy M. N. S. (2000) New technique for designing nearly-orthogonal wavelet filter banks with linear phase. IEE Proceeding on Vision, Image and Signal Processing 147(6): 527–533
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Zhao, Y., Swamy, M.N.S. The analysis and design of two-dimensional nearly-orthogonal symmetric wavelet filter banks. Multidim Syst Sign Process 24, 199–218 (2013). https://doi.org/10.1007/s11045-011-0165-0
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11045-011-0165-0