Skip to main content
SpringerLink
Log in

Performance analysis and improvement of direct position determination based on Doppler frequency shifts in presence of model errors: case of known waveforms

  • Published:
Multidimensional Systems and Signal Processing Aims and scope Submit manuscript

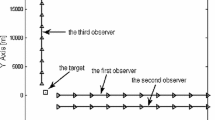
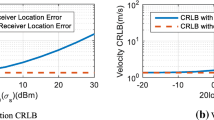
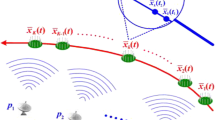
Abstract

The direct position determination (DPD) method based on Doppler frequency shifts for signals with known waveforms is first proposed by Amar and Weiss. Although this method exhibits excellent asymptotic performance, but does not account for the effects of uncertainties in the receiver positions and velocities. These uncertainties may result in a considerable reduction of localization accuracy. In this paper, the statistical performance of the DPD estimator is investigated under receiver position and velocity errors (also called model errors). We derive an analytical expression for the mean square error in the estimated source location in the case where the estimator assumes that the receiver positions and velocities are accurate, but they in fact contain errors. The main difficulty in the mathematical analysis is that the DPD cost function is not explicit with respect to the emitter position. Consequently, some algebraic manipulation is required to derive closed-form expressions for the first- and second-order partial derivatives of the cost function. The Cramér–Rao bounds (CRBs) for the target position estimation are also deduced in the presence and absence of model errors. These CRBs provide insights into the effects of model errors on the localization accuracy. Two improved DPD methods are developed based on our analysis results. The first has lower complexity than the common grid search, and the second exhibits increased robustness to model errors. Simulation results support and corroborate the theoretical developments in this paper.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13

Similar content being viewed by others

References

  • Amar, A., & Weiss, A. J. (2006). Direct position determination in the presence of model errors—Known waveforms. Digital Signal Processing, 16(1), 52–83.

    Article  Google Scholar 

  • Amar, A., & Weiss, A. J. (2007). A decoupled algorithm for geolocation of multiple emitters. Signal Processing, 87(10), 2348–2359.

    Article  MATH  Google Scholar 

  • Amar, A., & Weiss, A. J. (2008). Localization of narrowband radio emitters based on Doppler frequency shifts. IEEE Transactions on Signal Processing, 56(11), 5500–5508.

    Article  MathSciNet  MATH  Google Scholar 

  • Bao, Q. M., Ko, C. C., & Zhi, W. J. (2005). DOA estimation under unknown mutual coupling and multipath. IEEE Transactions on Aerospace and Electronic Systems, 41(2), 565–573.

    Article  Google Scholar 

  • Bar-Shalom, O., & Weiss, A. J. (2009). Efficient direct position determination of orthogonal frequency division multiplexing signals. IET Radar, Sonar and Navigation, 3(2), 101–111.

    Article  Google Scholar 

  • Beck, A., Stoica, P., & Li, J. (2008). Exact and approximate solutions of source localization problems. IEEE Transactions on Signal Processing, 56(5), 1770–1778.

    Article  MathSciNet  MATH  Google Scholar 

  • Cheung, K. W., So, H. C., Ma, W. K., & Chan, Y. T. (2004). Least squares algorithms for time-of-arrival-based mobile location. IEEE Transactions on Signal Processing, 52(4), 1121–1128.

    Article  MathSciNet  MATH  Google Scholar 

  • Ding, W., & Ying, W. (2017). Statistical performance analysis of direct position determination method based on doppler shifts in presence of model errors. Multidimensional Systems and Signal Processing, 28(1), 149–182.

    Article  MATH  Google Scholar 

  • Friedlander, B., & Weiss, A. J. (1991). Direction finding in the presence of mutual coupling. IEEE Transactions Antennas and Propagation, 39(3), 273–284.

    Article  Google Scholar 

  • Fu, Z. J., Ren, K., Shu, J. G., Sun, X. M., & Huang, F. X. (2016). Enabling personalized search over encrypted outsourced data with efficiency improvement. IEEE Transactions on Parallel and Distributed Systems, 27(9), 2546–2559.

    Article  Google Scholar 

  • Gogineni, S., & Nehorai, A. (2011). Target estimation using sparse modeling for distributed MIMO radar. IEEE Transactions on Signal Processing, 59(11), 5315–5325.

    Article  MathSciNet  MATH  Google Scholar 

  • Gu, J. F., Wei, P., & Tai, H. M. (2008). Fast direction-of-arrival estimation with known waveforms and linear operators. IET Signal Processing, 2(1), 27–36.

    Article  Google Scholar 

  • Ho, K. C., Lu, X., & Kovavisaruch, L. (2007). Source localization using TDOA and FDOA measurements in the presence of receiver location errors: Analysis and solution. IEEE Transactions on Signal Processing, 55(2), 684–696.

    Article  MathSciNet  MATH  Google Scholar 

  • Ho, K. C., & Sun, M. (2008). Passive source localization using time differences of arrival and gain ratios of arrival. IEEE Transactions on Signal Processing, 56(2), 464–477.

    Article  MathSciNet  MATH  Google Scholar 

  • Ho, K. C., & Yang, L. (2008). On the use of a calibration emitter for source localization in the presence of sensor position uncertainty. IEEE Transactions on Signal Processing, 56(12), 5758–5772.

    Article  MathSciNet  MATH  Google Scholar 

  • Kutluyil, D. (2005). Bearings-only target localization using total least squares. Signal Processing, 85(9), 1695–1710.

    Article  MATH  Google Scholar 

  • Li, J., Halder, B., Stoica, P., & Viberg, M. (1995). Computationally efficient angle estimation for signals with known waveforms. IEEE Transactions on Signal Processing, 43(9), 2154–2163.

    Article  Google Scholar 

  • Li, J. Z., Yang, L., Guo, F. C., & Jiang, W. L. (2016). Coherent summation of multiple short-time signals for direct positioning of a wideband source based on delay and Doppler. Digital Signal Processing, 48(1), 58–70.

    Article  MathSciNet  Google Scholar 

  • Mason, J. (2004). Algebraic two-satellite TOA/FOA position solution on an ellipsoidal earth. IEEE Transactions on Aerospace and Electronic Systems, 40(7), 1087–1092.

    Article  Google Scholar 

  • Nguyen, N. H., & Dogancay, K. (2015). Optimal sensor placement for Doppler shift target localization. In Proceeding of the IEEE radar conference. Arlington, USA: IEEE Press, pp. 1677–1682.

  • Nguyen, N. H., & Dogancay, K. (2015). Optimal sensor-target geometries for Doppler-shift target localization. In Proceeding of the European signal processing conference. Nice, France: IEEE Press, pp. 180–184.

  • Oispuu, M., & Nickel, U. (2010). Direct detection and position determination of multiple sources with intermittent emission. Signal Processing, 90(12), 3056–3064.

    Article  MATH  Google Scholar 

  • Pesavento, M., Gershman, A. B., & Wong, K. M. (2002). Direction finding in partly-calibrated sensor arrays composed of multiple subarrays. IEEE Transactions on Signal Processing, 55(9), 2103–2115.

    Article  Google Scholar 

  • Pourhomayoun, M., & Fowler, M. L. (2014). Distributed computation for direct position determination emitter location. IEEE Transactions on Aerospace and Electronic Systems, 50(4), 2878–2889.

    Article  Google Scholar 

  • Pulford, G. W. (2010). Analysis of a nonlinear least square procedure used in global positioning systems. IEEE Transactions on Signal Processing, 58(9), 4526–4534.

    Article  MathSciNet  MATH  Google Scholar 

  • Rahman, M. Z., & Kleeman, L. (2009). Paired measurement localization: A robust approach for wireless localization. IEEE Transactions on Mobile Computing, 8(8), 1087–1102.

    Article  Google Scholar 

  • Reuven, A. M., & Weiss, A. J. (2009). Direct position determination of cyclostationary signals. Signal Processing, 89(12), 2448–2464.

    Article  MATH  Google Scholar 

  • Roy, R., & Kailath, T. (1989). ESPRIT-estimation of signal parameters via rotational invariance techniques. IEEE Transactions on Acoustics, Speech, and Signal Processing, 37(7), 984–995.

    Article  MATH  Google Scholar 

  • Schmidt, R. O. (1986). Multiple emitter location and signal parameter estimation. IEEE Transactions on Antennas and Propagation, 34(3), 267–280.

    Google Scholar 

  • Shen, J., Shen, J., Chen, X. F., Huang, X. Y., & Susilo, W. (2017). An efficient public auditing protocol with novel dynamic structure for cloud data. IEEE Transactions on Information Forensics and Security, 12(10), 2402–2415.

    Article  Google Scholar 

  • Shen, Y., & Win, M. Z. (2010). Fundamental limits of wideband localization-Part I: A general framework. IEEE Transactions on Information Theory, 56(10), 4956–4980.

    Article  MathSciNet  MATH  Google Scholar 

  • Stein, S. (1993). Differential delay/doppler ML estimation with unknown signals. IEEE Transactions on Signal Processing, 41(8), 2717–2719.

    Article  MATH  Google Scholar 

  • Stoica, P., & Larsson, E. G. (2001). Comments on “Linearization method for finding Cramér–Rao bounds in signal processing”. IEEE Transactions on Signal Processing, 49(12), 3168–3169.

    Article  Google Scholar 

  • Sun, Y. J., & Gu, F. H. (2017). Compressive sensing of piezoelectric sensor response signal for phased array structural health monitoring. International Journal of Sensor Networks, 23(4), 258–264.

    Article  Google Scholar 

  • Sun, M., & Ho, K. C. (2011). An asymptotically efficient estimator for TDOA and FDOA positioning of multiple disjoint sources in the presence of sensor location uncertainties. IEEE Transactions on Signal Processing, 59(7), 3434–3440.

    Article  MathSciNet  MATH  Google Scholar 

  • Viberg, M., & Swindlehurst, A. L. (1994). A Bayesian approach to auto-calibration for parametric array signal processing. IEEE Transactions on Signal Processing, 42(12), 3495–3507.

    Article  Google Scholar 

  • Wang, Ding, & Ying, Wu. (2015). Sensor array calibration method in presence of gain/phase uncertainties and position perturbations using the spatial- and time-domain information of the auxiliary sources. Multidimensional Systems and Signal Processing, 26(3), 835–868.

    Article  MathSciNet  MATH  Google Scholar 

  • Wax, M., & Kailath, T. (1985). Decentralized processing in sensor arrays. IEEE Transactions on Signal Processing, 33(4), 1123–1129.

    Article  Google Scholar 

  • Weiss, A. J. (2004). Direct position determination of narrowband radio frequency transmitters. IEEE Signal Processing Letters, 11(5), 513–516.

    Article  Google Scholar 

  • Weiss, A. J. (2011). Direct geolocation of wideband emitters based on delay and Doppler. IEEE Transactions on Signal Processing, 59(6), 2513–5520.

    Article  MathSciNet  MATH  Google Scholar 

  • Weiss, A. J., & Amar, A. (2005). Direct position determination of multiple radio signals. EURASIP Journal on Applied Signal Processing, 2005(1), 37–49.

    MATH  Google Scholar 

  • Yang, L., & Ho, K. C. (2009). An approximately efficient TDOA localization algorithm in closed-form for locating multiple disjoint sources with erroneous sensor positions. IEEE Transactions on Signal Processing, 57(12), 4598–4615.

    Article  MathSciNet  MATH  Google Scholar 

  • Zhang, Y. H., Sun, X. M., & Wang, B. W. (2016). Efficient algorithm for K-barrier coverage based on integer linear programming. China Communications, 13(7), 16–23.

    Article  Google Scholar 

  • Zheng, J., & Wu, Y. C. (2010). Joint time synchronization and localization of an unknown node in wireless sensor networks. IEEE Transactions on Signal Processing, 58(3), 1309–1320.

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The authors would like to thank all the anonymous reviewers for their valuable comments and suggestions. The author also acknowledges support from National Natural Science Foundation of China (Grant Nos. 61201381, 61401513 and 61772548), China Postdoctoral Science Foundation (Grant No. 2016M592989), the Self-Topic Foundation of Information Engineering University (Grant No. 2016600701), and the Outstanding Youth Foundation of Information Engineering University (Grant No. 2016603201).

Author information

Authors and Affiliations

  1. National Digital Switching System Engineering and Technology Research Center, Zhengzhou, 450002, People’s Republic of China

    Ding Wang, Jiexin Yin, Ruirui Liu, Hongyi Yu & Yunlong Wang

  2. Zhengzhou Information Science and Technology Institute, Zhengzhou, 450002, Henan, People’s Republic of China

    Ding Wang, Jiexin Yin, Ruirui Liu, Hongyi Yu & Yunlong Wang

Authors
  1. Ding Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jiexin Yin
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Ruirui Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Hongyi Yu
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Yunlong Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Jiexin Yin.

Appendices

Appendix A: Proof of Proposition 1

A second-order Taylor-series expansion of \( \phi ({\hat{\varvec{q}}},{\varvec{\xi}}) \) around \( {\hat{\varvec{q}}} = {\varvec{q}} \) and \( {\varvec{\xi}} = {\varvec{O}}_{M \times 1} \) produces

$$ \begin{array}{l} \phi ({\hat{\varvec{q}}},{\varvec{\xi}}) = \phi ({\varvec{q}},{\varvec{O}}_{M \times 1}) + {\dot{\varvec{\varphi}}}_{1}^{\text{T}} ({\varvec{q}},{\varvec{O}}_{M \times 1}) \cdot {\varvec{\delta q}} + {\dot{\varvec{\varphi}}}_{2}^{\text{T}} ({\varvec{q}},{\varvec{O}}_{M \times 1}){\varvec{\xi}} + \frac{1}{2} \cdot ({\varvec{\delta q}})^{\text{T}} \cdot {\varvec{\ddot{\varPhi}}}_{1} ({\varvec{q}},{\varvec{O}}_{M \times 1}) \cdot {\varvec{\delta q}} \hfill \\ \,\,\,\,\,\,\,\,\,\,\,\, + ({\varvec{\delta q}})^{\text{T}} \cdot {\varvec{\ddot{\varPhi}}}_{2} ({\varvec{q}},{\varvec{O}}_{M \times 1}){\varvec{\xi}} + \frac{1}{2} \cdot {\varvec{\xi}}^{\text{T}} {\varvec{\ddot{\varPhi}}}_{3} ({\varvec{q}},{\varvec{O}}_{M \times 1}){\varvec{\xi}} + o(||{\varvec{\xi}}||_{2}^{2}) \hfill \\ \end{array} $$
(A.1)

where

$$ {\dot{\varvec{\varphi}}}_{1} ({\varvec{q}},{\varvec{O}}_{M \times 1}) = \frac{{\partial \phi ({\varvec{q}},{\varvec{O}}_{M \times 1})}}{{\partial {\varvec{q}}}}\,\,,\,\,{\dot{\varvec{\varphi}}}_{2} ({\varvec{q}},{\varvec{O}}_{M \times 1}) = \left. {\frac{{\partial \phi ({\varvec{q}},{\varvec{\xi}})}}{{\partial {\varvec{\xi}}}}} \right|_{{{\varvec{\xi}} = {\varvec{O}}_{M \times 1}}} $$
(A.2)
$$ \begin{aligned}{\varvec{\ddot{\varPhi}}}_{1} ({\varvec{q}},{\varvec{O}}_{M \times 1}) &= \frac{{\partial^{2} \phi ({\varvec{q}},{\varvec{O}}_{M \times 1})}}{{\partial {\varvec{q}}\partial {\varvec{q}}^{\text{T}}}}\,\,,\,\,{\varvec{\ddot{\varPhi}}}_{2} ({\varvec{q}},{\varvec{O}}_{M \times 1}) = \left. {\frac{{\partial^{2} \phi ({\varvec{q}},{\varvec{\xi}})}}{{\partial {\varvec{q}}\partial {\varvec{\xi}}^{\text{T}}}}} \right|_{{{\varvec{\xi}} = {\varvec{O}}_{M \times 1}}}, \\ {\varvec{\ddot{\varPhi}}}_{3} ({\varvec{q}},{\varvec{O}}_{M \times 1}) &= \left. {\frac{{\partial^{2} \phi ({\varvec{q}},{\varvec{\xi}})}}{{\partial {\varvec{\xi}}\partial {\varvec{\xi}}^{\text{T}}}}} \right|_{{{\varvec{\xi}} = {\varvec{O}}_{M \times 1}}} \end{aligned} $$
(A.3)

According to the maximum principle, we have

$$ {\dot{\varvec{\varphi}}}_{1} ({\varvec{q}},{\varvec{O}}_{M \times 1}) = \frac{{\partial \phi ({\varvec{q}},{\varvec{O}}_{M \times 1})}}{{\partial {\varvec{q}}}} = {\varvec{O}}_{D \times 1} $$
(A.4)

Then, combining (25), (A.1), and (A.4) yields

$$ {\varvec{\delta q}} \approx \arg \mathop {\max}\limits_{{{\varvec{x}} \in {\varvec{R}}^{D \times 1}}} \left\{{\frac{1}{2} \cdot {\varvec{x}}^{\text{T}} {\varvec{\ddot{\varPhi}}}_{1} ({\varvec{q}},{\varvec{O}}_{M \times 1}){\varvec{x}} + {\varvec{x}}^{\text{T}} {\varvec{\ddot{\varPhi}}}_{2} ({\varvec{q}},{\varvec{O}}_{M \times 1}){\varvec{\xi}}} \right\} $$
(A.5)

where the second- and higher-order error terms (i.e., \( o(||{\varvec{\xi}}||_{2}) \)) are neglected. It is straightforward to deduce from the second equality in (A.5) that

$$ {\varvec{\delta q}} = - {\varvec{\ddot{\varPhi}}}_{1}^{- 1} ({\varvec{q}},{\varvec{O}}_{M \times 1}){\varvec{\ddot{\varPhi}}}_{2} ({\varvec{q}},{\varvec{O}}_{M \times 1}){\varvec{\xi}} + o(||{\varvec{\xi}}||_{2}) $$
(A.6)

which proves Proposition 1.

Appendix B: Proof of Proposition 2

Define \( \varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}) \) by

$$ \varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}) = \arg \mathop {\max}\limits_{{\varDelta f_{k}}} \{\phi_{k} (\varDelta f_{k},{\varvec{q}},{\varvec{\xi}}_{k})\} $$
(B.1)

Then, it follows from the first equality in (26) that

$$ \phi ({\varvec{q}},{\varvec{\xi}}) = \sum\limits_{k = 1}^{K} {\mathop {\max}\limits_{{\varDelta f_{k}}} \{\phi_{k} (\varDelta f_{k},{\varvec{q}},{\varvec{\xi}}_{k})\}} = \sum\limits_{k = 1}^{K} {\phi_{k} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k})} $$
(B.2)

The partial derivative of \( \phi ({\varvec{q}},{\varvec{\xi}}) \) with respect to \( {\varvec{q}} \) is given by

$$ \begin{aligned} {\dot{\varvec{\varphi}}}_{1} ({\varvec{q}},{\varvec{\xi}}) &= \frac{{\partial \phi ({\varvec{q}},{\varvec{\xi}})}}{{\partial {\varvec{q}}}} \\ & = \sum\limits_{k = 1}^{K} {\left({\dot{\phi}_{k} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k}) \cdot \frac{{\partial \varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k})}}{{\partial {\varvec{q}}}} + {\dot{\varvec{\varphi}}}_{k,1} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k})} \right)} \end{aligned} $$
(B.3)

where

$$ \dot{\phi}_{k} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k}) = \left. {\frac{{\partial \phi_{k} (\varDelta f_{k},{\varvec{q}},{\varvec{\xi}}_{k})}}{{\partial \varDelta f_{k}}}} \right|_{{\varDelta f_{k} = \varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k})}} $$
(B.4)
$$ {\dot{\varvec{\varphi}}}_{k,1} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k}) = \left. {\frac{{\partial \phi_{k} (\varDelta f_{k},{\varvec{q}},{\varvec{\xi}}_{k})}}{{\partial {\varvec{q}}}}} \right|_{{\varDelta f_{k} = \varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k})}} $$
(B.5)

Further, using (B.3) we obtain

$$ {\varvec{\ddot{\Phi}}}_{ 1} ({\varvec{q}},{\varvec{\xi}}) = \sum\limits_{k = 1}^{K} {\left(\begin{array}{l} {\varvec{\ddot{\varPhi}}}_{k,1} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k}) + {\ddot{\phi}}_{k} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k}) \cdot \frac{{\partial \varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k})}}{{\partial {\varvec{q}}}} \cdot \left({\frac{{\partial \varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k})}}{{\partial {\varvec{q}}}}} \right)^{\text{T}} \\ + \frac{{\partial \varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k})}}{{\partial {\varvec{q}}}} \cdot {\varvec{\ddot{\varphi}}}_{k,1}^{\text{T}} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k}) + {\varvec{\ddot{\varphi}}}_{k,1} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k}) \cdot \left({\frac{{\partial \varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k})}}{{\partial {\varvec{q}}}}} \right)^{\text{T}} \\ + \dot{\phi}_{k} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k}) \cdot \frac{{\partial^{2} \varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k})}}{{\partial {\varvec{q}}\partial {\varvec{q}}^{\text{T}}}} \\ \end{array} \right)} $$
(B.6)
$$ {\varvec{\ddot{\Phi}}}_{ 2} ({\varvec{q}},{\varvec{\xi}}) = \sum\limits_{k = 1}^{K} {\left(\begin{array}{l} {\varvec{\ddot{\varPhi}}}_{k,2} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k}) +{\ddot{\phi}}_{k} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k}) \cdot \frac{{\partial \varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k})}}{{\partial {\varvec{q}}}} \cdot \left({\frac{{\partial \varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k})}}{{\partial {\varvec{\xi}}_{k}}}} \right)^{\text{T}} \\ + \frac{{\partial \varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k})}}{{\partial {\varvec{q}}}} \cdot {\varvec{\ddot{\varphi}}}_{k,2}^{\text{T}} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k}) + {\varvec{\ddot{\varphi}}}_{k,1} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k}) \cdot \left({\frac{{\partial \varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k})}}{{\partial {\varvec{\xi}}_{k}}}} \right)^{\text{T}} \\ + \dot{\phi}_{k,1} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k}) \cdot \frac{{\partial^{2} \varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k})}}{{\partial {\varvec{q}}\partial {\varvec{\xi}}_{k}^{\text{T}}}} \\ \end{array} \right)({\varvec{i}}_{K}^{{(k){\text{T}}}} \otimes {\varvec{I}}_{{M_{0}}})} $$
(B.7)

where

$$ \left\{\begin{array}{l} {\varvec{\ddot{\varphi}}}_{k,1} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k}) = \left. {\frac{{\partial^{2} \phi_{k} (\varDelta f_{k},{\varvec{q}},{\varvec{\xi}}_{k})}}{{\partial \varDelta f_{k} \partial {\varvec{q}}}}} \right|_{{\varDelta f_{k} = \varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k})}} \hfill \\ {\varvec{\ddot{\varphi}}}_{k,2} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k}) = \left. {\frac{{\partial^{2} \phi_{k} (\varDelta f_{k},{\varvec{q}},{\varvec{\xi}}_{k})}}{{\partial \varDelta f_{k} \partial {\varvec{\xi}}_{k}}}} \right|_{{\varDelta f_{k} = \varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k})}} \hfill \\ \end{array} \right. $$
(B.8)
$$ \left\{\begin{array}{l} {\varvec{\ddot{\varPhi}}}_{k,1} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k}) = \left. {\frac{{\partial^{2} \phi_{k} (\varDelta f_{k},{\varvec{q}},{\varvec{\xi}}_{k})}}{{\partial {\varvec{q}}\partial {\varvec{q}}^{\text{T}}}}} \right|_{{\varDelta f_{k} = \varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k})}} \hfill \\ {\varvec{\ddot{\varPhi}}}_{k,2} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k}) = \left. {\frac{{\partial^{2} \phi_{k} (\varDelta f_{k},{\varvec{q}},{\varvec{\xi}}_{k})}}{{\partial {\varvec{q}}\partial {\varvec{\xi}}_{k}^{\text{T}}}}} \right|_{{\varDelta f_{k} = \varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k})}} \hfill \\ \end{array} \right. $$
(B.9)

On the other hand, combining the maximum principle with the definition of \( \varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}) \) in (B.1) leads to

$$ {\ddot{\phi}}_{k} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k}) = \left. {\frac{{\partial \phi_{k} (\varDelta f_{k},{\varvec{q}},{\varvec{\xi}}_{k})}}{{\partial \varDelta f_{k}}}} \right|_{{\varDelta f_{k} = \varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k})}} = 0 $$
(B.10)

Taking the derivative with respect to \( {\varvec{q}} \) on both sides of (B.10) yields

$$ {\ddot{\phi}}_{k} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k}) \cdot \frac{{\partial \varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k})}}{{\partial {\varvec{q}}}} + {\varvec{\ddot{\varphi}}}_{k,1} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k}) = {\varvec{O}}_{D \times 1} $$
(B.11)

which implies

$$ \frac{{\partial \varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k})}}{{\partial {\varvec{q}}}} = - \frac{{{\varvec{\ddot{\varphi}}}_{k,1} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k})}}{{\ddot{\phi}_{k} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k})}} $$
(B.12)

Similarly, taking the derivative with respect to \( {\varvec{\xi}}_{k} \) on both sides of (B.10), we get

$$ {\phi}_{k} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k}) \cdot \frac{{\partial \varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k})}}{{\partial {\varvec{\xi}}_{k}}} + {\varvec{\ddot{\varphi}}}_{k,2} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k}) = {\varvec{O}}_{{M_{0} \times 1}} $$
(B.13)

which implies

$$ \frac{{\partial \varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k})}}{{\partial {\varvec{\xi}}_{k}}} = - \frac{{{\varvec{\ddot{\varphi}}}_{k,2} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k})}}{{\ddot{\phi}_{k} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k})}} $$
(B.14)

Inserting (B.10) and (B.12) into (B.6) produces

$$ {\varvec{\ddot{\varPhi}}}_{ 1} ({\varvec{q}},{\varvec{\xi}}) = \sum\limits_{k = 1}^{K} {\left({{\varvec{\ddot{\varPhi}}}_{k,1} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k}) - \frac{{{\varvec{\ddot{\varphi}}}_{k,1} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k}){\varvec{\ddot{\varphi}}}_{k,1}^{\text{T}} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k})}}{{\ddot{\phi}_{k} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k})^{{^{{}}}}}}} \right)} $$
(B.15)

Substituting (B.10), (B.12) and (B.14) into (B.7) yields

$$ {\varvec{\ddot{\varPhi}}}_{ 2} ({\varvec{q}},{\varvec{\xi}}) = \sum\limits_{k = 1}^{K} {\left({{\varvec{\ddot{\varPhi}}}_{k,2} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k}) - \frac{{{\varvec{\ddot{\varphi}}}_{k,1} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k}){\varvec{\ddot{\varphi}}}_{k,2}^{\text{T}} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k})}}{{\ddot{\phi}_{k} (\varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{\xi}}_{k}),{\varvec{q}},{\varvec{\xi}}_{k})^{{^{{}}}}}}} \right)({\varvec{i}}_{K}^{{(k){\text{T}}}} \otimes {\varvec{I}}_{{M_{0}}})} $$
(B.16)

Furthermore, note that \( \varDelta f_{k} = \varDelta f_{k}^{{({\text{o}})}} ({\varvec{q}},{\varvec{O}}_{{M_{0} \times 1}}) \). As a consequence, if the error vector \( {\varvec{\xi}} \) in (B.15) and (B.16) is replaced with \( {\varvec{O}}_{M \times 1} \), both (29) and (30) hold. This completes the proof of Proposition 2.

Appendix C: Detailed derivation of \( {\dot{\varvec{C}}}_{k,j}^{(1)} ({\varvec{q}},{\bar{\varvec{p}}}_{k}) \) and \( {\varvec{\ddot{C}}}_{{k,j_{1} j_{2}}}^{(1)} ({\varvec{q}},{\bar{\varvec{p}}}_{k}) \)

It can be easily seen from (16) that

$$ {\dot{\varvec{C}}}_{k,j}^{(1)} ({\varvec{q}},{\bar{\varvec{p}}}_{k}) = [({\dot{\varvec{A}}}_{j}^{(1)} ({\varvec{q}},{\bar{\varvec{p}}}_{1,k}))^{\text{H}} {\varvec{z}}_{1,k} \,\,\,({\dot{\varvec{A}}}_{j}^{(1)} ({\varvec{q}},{\bar{\varvec{p}}}_{2,k}))^{\text{H}} {\varvec{z}}_{2,k} \,\,\, \cdots \,\,\,({\dot{\varvec{A}}}_{j}^{(1)} ({\varvec{q}},{\bar{\varvec{p}}}_{L,k}))^{\text{H}} {\varvec{z}}_{L,k}] $$
(C.1)
$$ {\varvec{\ddot{C}}}_{{k,j_{1} j_{2}}}^{(1)} ({\varvec{q}},{\bar{\varvec{p}}}_{k}) = [({\varvec{\ddot{A}}}_{{j_{1} j_{2}}}^{(1)} ({\varvec{q}},{\bar{\varvec{p}}}_{1,k}))^{\text{H}} {\varvec{z}}_{1,k} \,\,\,({\varvec{\ddot{A}}}_{{j_{1} j_{2}}}^{(1)} ({\varvec{q}},{\bar{\varvec{p}}}_{2,k}))^{\text{H}} {\varvec{z}}_{2,k} \,\,\, \cdots \,\,\,({\varvec{\ddot{A}}}_{{j_{1} j_{2}}}^{(1)} ({\varvec{q}},{\bar{\varvec{p}}}_{L,k}))^{\text{H}} {\varvec{z}}_{L,k}] $$
(C.2)

where

$$ {\dot{\varvec{A}}}_{j}^{(1)} ({\varvec{q}},{\bar{\varvec{p}}}_{l,k}) = \frac{{\partial {\varvec{A}}({\varvec{q}},{\bar{\varvec{p}}}_{l,k})}}{{\partial < {\varvec{q}} >_{j}}}\,\,,\,\,{\varvec{\ddot{A}}}_{{j_{1} j_{2}}}^{(1)} ({\varvec{q}},{\bar{\varvec{p}}}_{l,k}) = \frac{{\partial^{2} {\varvec{A}}({\varvec{q}},{\bar{\varvec{p}}}_{l,k})}}{{\partial < {\varvec{q}} >_{{j_{1}}} \partial < {\varvec{q}} >_{{j_{2}}}}} $$
(C.3)

Using the first equality in (9), we have

$$ \begin{aligned} {\dot{\varvec{A}}}_{j}^{(1)} ({\varvec{q}},{\bar{\varvec{p}}}_{l,k}) & = ({\text{j2}}\uppi \,f_{c} T_{s}) \cdot \frac{{\partial \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})}}{{\partial < {\varvec{q}} >_{j}}} \\ & \quad \times {\text{diag[0}}\,\,\,{ \exp }\{{\text{j2}}{\uppi}\,f_{c} \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})T_{s} \} \,\,\,2 \cdot { \exp }\{{\text{j4}}{\uppi}\,f_{c} \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})T_{s} \} \,\,\, \cdots \,\,\,\\ & \qquad (N - 1) \cdot { \exp }\{{\text{j2}}{\uppi}\,f_{c} \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})(N - 1)T_{s} \} ]\end{aligned} $$
(C.4)

which implies

$$ \begin{aligned} \varvec{\ddot{A}}_{{j_{1} j_{2}}}^{(1)} (\varvec{q},\bar{\varvec{p}}_{l,k}) & = (\hbox{j}2\uppi \,f_{c} T_{s}) \cdot \frac{{\partial^{2} \gamma (\varvec{q},\bar{\varvec{p}}_{l,k})}}{{\partial < \varvec{q} >_{{j_{1}}} \partial < \varvec{q} >_{{j_{2}}}}} \\ & \quad \times {\text{diag}}[0\,\,\,\exp \{\hbox{j}2\uppi \,f_{c} \gamma (\varvec{q},\bar{\varvec{p}}_{l,k})T_{s} \} \,\,\,2 \cdot \exp \{\hbox{j}4\uppi \,f_{c} \gamma (\varvec{q},\bar{\varvec{p}}_{l,k})T_{s} \} \\ & \qquad \cdots (N - 1) \cdot \exp \{\hbox{j}2\uppi \,f_{c} \gamma (\varvec{q},\bar{\varvec{p}}_{l,k})(N - 1)T_{s} \}] \\ & \quad - 4\uppi^{2} f_{c}^{2} T_{s}^{2} \cdot \frac{{\partial \gamma (\varvec{q},\bar{\varvec{p}}_{l,k})}}{{\partial < \varvec{q} >_{{j_{1}}}}} \cdot \frac{{\partial \gamma (\varvec{q},\bar{\varvec{p}}_{l,k})}}{{\partial < \varvec{q} >_{{j_{2}}}}} \\ & \quad \times {\text{diag}}[0\,\,\,\exp \{\hbox{j}2\uppi \,f_{c} \gamma (\varvec{q},\bar{\varvec{p}}_{l,k})T_{s} \} \,\,\,4 \cdot \exp \{\hbox{j}4\uppi \,f_{c} \gamma (\varvec{q},\bar{\varvec{p}}_{l,k})T_{s} \} \\ & \qquad \cdots (N - 1)^{2} \cdot \exp \{\hbox{j}2\uppi \,f_{c} \gamma (\varvec{q},\bar{\varvec{p}}_{l,k})(N - 1)T_{s} \}] \\ \end{aligned} $$
(C.5)

Next, expressions for the gradient vector and Hessian matrix of \( \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k}) \) with respect to \( {\varvec{q}} \) are derived, which lead to \( \frac{{\partial \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})}}{{\partial < {\varvec{q}} >_{j}}} \) and \( \frac{{\partial^{2} \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})}}{{\partial < {\varvec{q}} >_{{j_{1}}} \partial < {\varvec{q}} >_{{j_{2}}}}} \), respectively.

First, it follows from (3) that

$$ \frac{{\partial \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})}}{{\partial {\varvec{q}}}} = \frac{1}{v} \cdot \left({{\varvec{I}}_{D} - \frac{{({\varvec{q}} - {\varvec{p}}_{l,k})({\varvec{q}} - {\varvec{p}}_{l,k})^{\text{T}}}}{{||{\varvec{q}} - {\varvec{p}}_{l,k} ||_{2}^{2}}}} \right) \cdot \frac{{{\dot{\varvec{p}}}_{l,k}}}{{||{\varvec{q}} - {\varvec{p}}_{l,k} ||_{2}}} $$
(C.6)

which implies

$$ \frac{{\partial^{2} \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})}}{{\partial {\varvec{q}}\partial {\varvec{q}}^{\text{T}}}} = \frac{1}{v} \cdot \left(\begin{array}{l} 3 \cdot \frac{{{\dot{\varvec{p}}}_{{l,{\kern 1pt} k}}^{\text{T}} ({\varvec{q}} - {\varvec{p}}_{{l,{\kern 1pt} k}})}}{{||{\varvec{q}} - {\varvec{p}}_{{l,{\kern 1pt} k}} ||_{2}^{5}}} \cdot ({\varvec{q}} - {\varvec{p}}_{{l,{\kern 1pt} k}})({\varvec{q}} - {\varvec{p}}_{{l,{\kern 1pt} k}})^{\text{T}} - \frac{{{\dot{\varvec{p}}}_{{l,{\kern 1pt} k}}^{\text{T}} ({\varvec{q}} - {\varvec{p}}_{{l,{\kern 1pt} k}})}}{{||{\varvec{q}} - {\varvec{p}}_{{l,{\kern 1pt} k}} ||_{2}^{3}}} \cdot {\varvec{I}}_{D} \\ - \frac{{({\varvec{q}} - {\varvec{p}}_{{l,{\kern 1pt} k}}){\dot{\varvec{p}}}_{{l,{\kern 1pt} k}}^{\text{T}}}}{{||{\varvec{q}} - {\varvec{p}}_{{l,{\kern 1pt} k}} ||_{2}^{3}}} - \frac{{{\dot{\varvec{p}}}_{{l,{\kern 1pt} k}} ({\varvec{q}} - {\varvec{p}}_{{l,{\kern 1pt} k}})^{\text{T}}}}{{||{\varvec{q}} - {\varvec{p}}_{{l,{\kern 1pt} k}} ||_{2}^{3}}} \\ \end{array} \right) $$
(C.7)

Obviously, \( \frac{{\partial \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})}}{{\partial < {\varvec{q}} >_{j}}} \) is the jth component of \( \frac{{\partial \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})}}{{\partial {\varvec{q}}}} \) and \( \frac{{\partial^{2} \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})}}{{\partial < {\varvec{q}} >_{{j_{1}}} \partial < {\varvec{q}} >_{{j_{2}}}}} \) is the (j1, j2)th element of \( \frac{{\partial^{2} \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})}}{{\partial {\varvec{q}}\partial {\varvec{q}}^{\text{T}}}} \).

Appendix D: Detailed derivation of \( {\dot{\varvec{C}}}_{k,j}^{(2)} ({\varvec{q}},{\bar{\varvec{p}}}_{k}) \) and \( {\varvec{\ddot{C}}}_{{k,j_{1} j_{2}}}^{(2)} ({\varvec{q}},{\bar{\varvec{p}}}_{k}) \)

Using (16) and the definition of the error vector \( {\varvec{\xi}}_{k} \) yields

$$ \left\{\begin{array}{l} {\dot{\varvec{C}}}_{k,j}^{(2)} ({\varvec{q}},{\bar{\varvec{p}}}_{k}) = {\varvec{i}}_{L}^{{(N_{1} (j)){\text{T}}}} \otimes (({\varvec{A}}({\varvec{q}},{\bar{\varvec{p}}}_{{N_{1} (j),k}}))^{\text{H}} {\varvec{i}}_{N}^{{(N_{2} (j))}})\quad \,\,\,\,\,{\kern 1pt} {\kern 1pt} (1 \le j \le 2NL\,;\,1 \le N_{2} (j) \le N) \\ {\dot{\varvec{C}}}_{k,j}^{(2)} ({\varvec{q}},{\bar{\varvec{p}}}_{k}) = {\varvec{i}}_{L}^{{(N_{1} (j)){\text{T}}}} \otimes ({\text{j(}}{\varvec{A}}({\varvec{q}},{\bar{\varvec{p}}}_{{N_{1} (j),k}}))^{\text{H}} {\varvec{i}}_{N}^{{(N_{3} (j))}})\quad (1 \le j \le 2NL\,;\,N + 1 \le N_{2} (j) \le 2N) \\ {\dot{\varvec{C}}}_{k,j}^{(2)} ({\varvec{q}},{\bar{\varvec{p}}}_{k}) = {\varvec{i}}_{L}^{{(D_{1} (j)){\text{T}}}} \otimes \left({\left({\frac{{\partial {\varvec{A}}({\varvec{q}},{\bar{\varvec{p}}}_{{D_{1} (j),k}})}}{{\partial < {\bar{\varvec{p}}}_{{D_{1} (j),k}} >_{{D_{2} (j)}}}}} \right)^{\text{H}} {\varvec{z}}_{{D_{1} (j),k}}} \right) \\ = {\varvec{i}}_{L}^{{(D_{1} (j)){\text{T}}}} \otimes (({\dot{\varvec{A}}}_{{D_{2} (j)}}^{(2)} ({\varvec{q}},{\bar{\varvec{p}}}_{{D_{1} (j),k}}))^{\text{H}} {\varvec{z}}_{{D_{1} (j),k}})\quad {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} (2NL + 1 \le j \le 2(N + D)L) \\ \end{array} \right. $$
(D.1)

where

$$ \left\{\begin{array}{l} N_{1} (j) = \left\lceil {j/2N} \right\rceil \,\,,\,\,N_{2} (j) = j - 2N(\left\lceil {j/2N} \right\rceil - 1)\,\,,\,\,N_{3} (j) = j - 2N(\left\lceil {j/2N} \right\rceil - 1) - N \hfill \\ D_{1} (j) = \left\lceil {(j - 2NL)/2D} \right\rceil \,\,,\,\,D_{2} (j) = j - 2NL - 2D(\left\lceil {(j - 2NL)/2D} \right\rceil - 1) \hfill \\ {\dot{\varvec{A}}}_{{D_{2} (j)}}^{(2)} ({\varvec{q}},{\bar{\varvec{p}}}_{{D_{1} (j),k}}) = \frac{{\partial {\varvec{A}}({\varvec{q}},{\bar{\varvec{p}}}_{{D_{1} (j),k}})}}{{\partial < {\bar{\varvec{p}}}_{{D_{1} (j),k}} >_{{D_{2} (j)}}}} \hfill \\ \end{array} \right. $$
(D.2)

Furthermore, from (D.1) and the definition of \( {\varvec{\xi}}_{k} \), we have that

$$ \left\{\begin{array}{l} {\varvec{\ddot{C}}}_{{k,j_{1} j_{2}}}^{(2)} ({\varvec{q}},{\bar{\varvec{p}}}_{k}) = {\varvec{i}}_{L}^{{(N_{1} (j_{2})){\text{T}}}} \otimes (({\dot{\varvec{A}}}_{{j_{1}}}^{(1)} ({\varvec{q}},{\bar{\varvec{p}}}_{{N_{1} (j_{2}),k}}))^{\text{H}} {\varvec{i}}_{N}^{{(N_{2} (j_{2}))}})\quad \,\,\,\,\,{\kern 1pt} {\kern 1pt} (1 \le j_{2} \le 2NL\,;\,1 \le N_{2} (j_{2}) \le N) \\ {\varvec{\ddot{C}}}_{{k,j_{1} j_{2}}}^{(2)} ({\varvec{q}},{\bar{\varvec{p}}}_{k}) = {\varvec{i}}_{L}^{{(N_{1} (j_{2})){\text{T}}}} \otimes ({\text{j(}}{\dot{\varvec{A}}}_{{j_{1}}}^{(1)} ({\varvec{q}},{\bar{\varvec{p}}}_{{N_{1} (j_{2}),k}}))^{\text{H}} {\varvec{i}}_{N}^{{(N_{3} (j_{2}))}})\quad (1 \le j_{2} \le 2NL\,;\,N + 1 \le N_{2} (j_{2}) \le 2N) \\ {\varvec{\ddot{C}}}_{{k,j_{1} j_{2}}}^{(2)} ({\varvec{q}},{\bar{\varvec{p}}}_{k}) = {\varvec{i}}_{L}^{{(D_{1} (j_{2})){\text{T}}}} \otimes \left({\left({\frac{{\partial^{2} {\varvec{A}}({\varvec{q}},{\bar{\varvec{p}}}_{{D_{1} (j_{2}),k}})}}{{\partial < {\varvec{q}} >_{{j_{1}}} \partial < {\bar{\varvec{p}}}_{{D_{1} (j_{2}),k}} >_{{D_{2} (j_{2})}}}}} \right)^{\text{H}} {\varvec{z}}_{{D_{1} (j_{2}),k}}} \right) \\ \quad \quad = {\varvec{i}}_{L}^{{(D_{1} (j_{2})){\text{T}}}} \otimes (({\varvec{\ddot{A}}}_{{j_{1},D_{2} (j_{2})}}^{(2)} ({\varvec{q}},{\bar{\varvec{p}}}_{{D_{1} (j_{2}),k}}))^{\text{H}} {\varvec{z}}_{{D_{1} (j_{2}),k}})\quad {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} (2NL + 1 \le j_{2} \le 2(N + D)L) \\ \end{array} \right. $$
(D.3)

where

$$ {\varvec{\ddot{A}}}_{{j_{1},D_{2} (j_{2})}}^{(2)} ({\varvec{q}},{\bar{\varvec{p}}}_{{D_{1} (j_{2}),k}}) = \frac{{\partial^{2} {\varvec{A}}({\varvec{q}},{\bar{\varvec{p}}}_{{D_{1} (j_{2}),k}})}}{{\partial < {\varvec{q}} >_{{j_{1}}} \partial < {\bar{\varvec{p}}}_{{D_{1} (j_{2}),k}} >_{{D_{2} (j_{2})}}}} $$
(D.4)

Similar to the derivation in (C.4) and (C.5), it can be checked that

$$ \begin{aligned} {\dot{\varvec{A}}}_{j}^{(2)} ({\varvec{q}},{\bar{\varvec{p}}}_{l,k}) & = ({\text{j2}}\uppi \,f_{c} T_{s}) \cdot \frac{{\partial \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})}}{{\partial < {\bar{\varvec{p}}}_{l,k} >_{j}}} \\ & \quad \times {\text{diag[0}}\,\,\,{ \exp }\{{\text{j2}}\uppi \,f_{c} \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})T_{s} \} \,\,\,2 \cdot { \exp }\{{\text{j4}}\uppi \,f_{c} \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})T_{s} \} \\ & \qquad \cdots (N - 1) \cdot { \exp }\{{\text{j2}}\uppi \,f_{c} \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})(N - 1)T_{s} \} ]\\ \end{aligned} $$
(D.5)
$$ \begin{aligned} {\varvec{\ddot{A}}}_{{j_{1} j_{2}}}^{(2)} ({\varvec{q}},{\bar{\varvec{p}}}_{l,k}) & = ({\text{j2}}\uppi \,f_{c} T_{s}) \cdot \frac{{\partial^{2} \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})}}{{\partial < {\varvec{q}} >_{{j_{1}}} \partial < {\bar{\varvec{p}}}_{l,k} >_{{j_{2}}}}} \\ & \quad \times {\text{diag[0}}\,\,\,{ \exp }\{{\text{j2}}\uppi \,f_{c} \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})T_{s} \} \,\,\,2 \cdot { \exp }\{{\text{j4}}\uppi \,f_{c} \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})T_{s} \} \\ & \qquad \cdots \,\,\,(N - 1) \cdot { \exp }\{{\text{j2}}\uppi \,f_{c} \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})(N - 1)T_{s} \} ]\\ & \quad - 4{\uppi}^{ 2} f_{c}^{2} T_{s}^{2} \cdot \frac{{\partial \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})}}{{\partial < {\varvec{q}} >_{{j_{1}}}}} \cdot \frac{{\partial \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})}}{{\partial < {\bar{\varvec{p}}}_{l,k} >_{{j_{2}}}}} \\ & \quad \times {\text{diag[0}}\,\,\,{ \exp }\{{\text{j2}}\uppi \,f_{c} \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})T_{s} \} \,\,\,4 \cdot { \exp }\{{\text{j4}}\uppi \,f_{c} \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})T_{s} \} \\ & \qquad \cdots (N - 1)^{2} \cdot { \exp }\{{\text{j2}}\uppi \,f_{c} \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})(N - 1)T_{s} \} ]\\ \end{aligned} $$
(D.6)

In the following, we derive expressions for the first-order partial derivative vector \( \frac{{\partial \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})}}{{\partial {\varvec{q}}}} \) and second-order partial derivative matrix \( \frac{{\partial^{2} \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})}}{{\partial {\varvec{q}}\partial {\bar{\varvec{p}}}_{l,k}^{\text{T}}}} \).

First, combining (3) and the definition of \( {\bar{\varvec{p}}}_{l,k} \) leads to

(D.7)

which implies

(D.8)

It is evident that \( \frac{{\partial \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})}}{{\partial < {\bar{\varvec{p}}}_{l,k} >_{j}}} \) is the jth component of \( \frac{{\partial \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})}}{{\partial {\bar{\varvec{p}}}_{l,k}}} \) and \( \frac{{\partial^{2} \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})}}{{\partial < {\varvec{q}} >_{{j_{1}}} \partial < {\bar{\varvec{p}}}_{l,k} >_{{j_{2}}}}} \) is the (j1, j2)th entry of \( \frac{{\partial^{2} \gamma ({\varvec{q}},{\bar{\varvec{p}}}_{l,k})}}{{\partial {\varvec{q}}\partial {\bar{\varvec{p}}}_{l,k}^{\text{T}}}} \).

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wang, D., Yin, J., Liu, R. et al. Performance analysis and improvement of direct position determination based on Doppler frequency shifts in presence of model errors: case of known waveforms. Multidim Syst Sign Process 30, 749–790 (2019). https://doi.org/10.1007/s11045-018-0579-z

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11045-018-0579-z

Keywords

Search

Navigation