Skip to main content
Springer Nature Link
Log in

\(\alpha \)-Exponential Stability of Impulsive Fractional-Order Complex-Valued Neural Networks with Time Delays

  • Published:
Neural Processing Letters Aims and scope Submit manuscript

Abstract

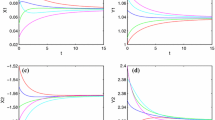
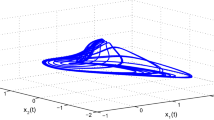
This paper investigates the global \(\alpha \)-exponential stability of impulsive fractional-order complex-valued neural networks with time delays. By constructing proper Lyapunov–Krasovskii functional and employing fractional-order complex-valued differential inequality, some sufficient conditions are obtained to ensure the existence, uniqueness and global \(\alpha \)-exponential stability of the equilibrium point for the considered neural networks. Moreover, the exponential convergence rate is estimated, which depends on the parameters and the order of differentiation of system. Finally, one numerical example with simulations is given to illustrate the effectiveness of the obtained results.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

References

  1. Ahmed E, Elgazzar AS (2007) On fractional order differential equations model for nonlocal epidemics. Physica A 379:607–614

    MathSciNet  Google Scholar 

  2. Hilfer R (2000) Applications of fractional calculus in physics. World Scientific, Singapore

    MATH  Google Scholar 

  3. Laskin N (2000) Fractional market dynamics. Physica A 287:482–492

    MathSciNet  Google Scholar 

  4. Delavari H, Baleanu D, Sadati J (2012) Stability analysis of Caputo fractional-order nonlinear systems revisited. Nonlinear Dyn 67:2433–2439

    MathSciNet  MATH  Google Scholar 

  5. Li Y, Chen YQ, Podlubny I (2009) Mittag–Leffler stability of fractional order nonlinear dynamic systems. Automatica 45:1965–1969

    MathSciNet  MATH  Google Scholar 

  6. Li Y, Chen YQ, Podlubny I (2010) Stability of fractional-order nonlinear dynamic systems: Lyapunov direct method and generalized Mittag–Leffler stability. Comput Math Appl 59:1810–1821

    MathSciNet  MATH  Google Scholar 

  7. Wu XJ, Lu HT, Shen SL (2009) Synchronization of a new fractional-order hyperchaotic system. Phys Lett A 373:2329–2337

    MathSciNet  MATH  Google Scholar 

  8. Duarte-Mermoud MA, Aguila-Camacho N, Gallegos JA, Castro-Linares R (2015) Using general quadratic Lyapunov functions to prove Lyapunov uniform stability for fractional order systems. Commun Nonlinear Sci Numer Simul 22:650–659

    MathSciNet  MATH  Google Scholar 

  9. Yang XJ, Machado JT, Cattani C, Gao F (2017) On a fractal LC-electric circuit modeled by local fractional calculus. Commun Nonlinear Sci Numer Simul 47:200–206

    Google Scholar 

  10. Yang XJ (2016) Fractional derivatives of constant and variable orders applied to anomalous relaxation models in heat-transfer problems. Therm Sci 21(3):1161–1171

    Google Scholar 

  11. Yang XJ, Li CD, Song QK, Huang TW, Chen XF (2016) Mittag–Leffler stability analysis on variable-time impulsive fractional-order neural networks. Neurocomputing 207:276–286

    Google Scholar 

  12. Yang XJ, Li CD, Huang TW, Song QK (2017) Mittag–Leffler stability analysis of nonlinear fractional-order systems with impulses. Appl Math Comput 293:416–422

    MathSciNet  MATH  Google Scholar 

  13. Wu HQ, Zhang XX, Xue SH, Wang LF, Wang Y (2016) LMI conditions to global Mittag–Leffler stability of fractional-order neural networks with impulses. Neurocomputing 193:148–154

    Google Scholar 

  14. Yu J, Hu C, Jiang HJ (2012) \(\alpha \)-stability and \(\alpha \)-synchronization for fractional-order neural networks. Neural Netw 35:82–87

    MATH  Google Scholar 

  15. Song C, Cao JD (2014) Dynamics in fractional-order neural networks. Neurocomputing 142:494–498

    Google Scholar 

  16. Chen LP, Chai Y, Wu RC, Ma TD, Zhai HZ (2013) Dynamic analysis of a class of fractional-order neural networks with delay. Neurocomputing 111:190–194

    Google Scholar 

  17. Wang H, Yu YG, Wen GG, Zhang S, Yu JZ (2015) Global stability analysis of fractional-order Hopfield neural networks with time delay. Neurocomputing 154:15–23

    Google Scholar 

  18. Wang H, Yu YG, Wen GG (2014) Stability analysis of fractional-order Hopfield neural networks with time delays. Neural Netw 55:98–109

    MATH  Google Scholar 

  19. Wang H, Yu YG, Wen GG, Zhang S (2015) Stability analysis of fractional-order neural networks with time delay. Neural Process Lett 42:479–500

    Google Scholar 

  20. Yang XJ, Song QK, Liu YR, Zhao ZJ (2015) Finite-time stability analysis of fractional-order neural networks with delay. Neurocomputing 152:19–26

    Google Scholar 

  21. Wu RC, Lu YF, Chen LP (2015) Finite-time stability of fractional delayed neural networks. Neurocomputing 149:700–707

    Google Scholar 

  22. Chen LP, Wu RC, Cao JD, Liu JB (2015) Stability and synchronization of memristor-based fractional-order delayed neural networks. Neural Netw 71:37–44

    MATH  Google Scholar 

  23. Ding ZX, Shen Y, Wang LM (2015) Global Mittag–Leffler synchronization of fractional-order neural networks with discontinuous activations. Neural Netw 73:77–85

    MATH  Google Scholar 

  24. Bao HB, Cao JD (2015) Projective synchronization of fractional-order memristor-based neural networks. Neural Netw 63:1–9

    MATH  Google Scholar 

  25. Ma WY, Li CP, Wu YJ, Wu YQ (2014) Adaptive synchronization of fractional neural networks with unknown parameters and time delays. Entropy 16:6286–6299

    MathSciNet  Google Scholar 

  26. Chen JY, Li CD, Huang TW, Yang XJ (2017) Global stabilization of memristor-based fractional-order neural networks with delay via output-feedback Control. Modern Phys Lett B 31(5):1750031

    MathSciNet  Google Scholar 

  27. Wang F, Yang YQ, Hu MF (2015) Asymptotic stability of delayed fractional-order neural networks with impulsive effects. Neurocomputing 154:239–244

    Google Scholar 

  28. Hu J, Wang J (2012) Global stability of complex-valued recurrent neural networks with time-delays. IEEE Trans Neural Netw Learn Syst 23:853–865

    Google Scholar 

  29. Zhang ZQ, Yu SH (2016) Global asymptotic stability for a class of complex-valued Cohen–Grossberg neural networks with time delays. Neurocomputing 171:1158–1166

    Google Scholar 

  30. Xu XH, Zhang JY, Shi JZ (2014) Exponential stability of complex-valued neural networks with mixed delays. Neurocomputing 128:483–490

    Google Scholar 

  31. Zhang ZY, Lin C, Chen B (2014) Global stability criterion for delayed complex-valued recurrent neural networks. IEEE Trans Neural Netw Learn Syst 25:1704–1708

    Google Scholar 

  32. Fang T, Sun JT (2014) Further investigate the stability of complex-valued recurrent neural networks with time-delays. IEEE Trans Neural Netw Learn Syst 25:1709–1713

    Google Scholar 

  33. Pan J, Liu XZ, Xie WC (2015) Exponential stability of a class of complex-valued neural networks with time-varying delays. Neurocomputing 164:293–299

    Google Scholar 

  34. Fang T, Sun JT (2014) Stability of complex-valued impulsive system with delay. Appl Math Comput 240:102–108

    MathSciNet  MATH  Google Scholar 

  35. Song QK, Zhao ZJ, Liu YR (2015) Stability analysis of complex-valued neural networks with probabilistic time-varying delays. Neurocomputing 159:96–104

    Google Scholar 

  36. Song QK, Yan H, Zhao ZJ, Liu YR (2016) Global exponential stability of complex-valued neural networks with both time-varying delays and impulsive effects. Neural Netw 79:108–116

    MATH  Google Scholar 

  37. Rakkiyappan R, Sivaranjani R, Velmurugan G, Cao JD (2016) Analysis of global \(o(t^{-\alpha })\) stability and global asymptotical periodicity for a class of fractional-order complex-valued neural networks with time varying delays. Neural Netw 77:51–69

    MATH  Google Scholar 

  38. Rakkiyappan R, Cao JD, Velmurugan G (2015) Existence and uniform stability analysis of fractional-order complex-valued neural networks with time delays. IEEE Trans Neural Netw Learn Syst 26:84–97

    MathSciNet  MATH  Google Scholar 

  39. Rakkiyappana R, Velmurugana G, Cao JD (2015) Stability analysis of fractional-order complex-valued neural networks with time delays. Chaos Solitons Fractal 78:297–316

    MathSciNet  Google Scholar 

  40. Bao HB, Park JH, Cao JD (2016) Synchronization of fractional-order complex-valued neural networks with time delay. Neural Netw 81:16–28

    MATH  Google Scholar 

  41. Xu Q, Zhuang SX, Liu SJ, Xiao J (2016) Decentralized adaptive coupling synchronization of fractional-order complex-variable dynamical networks. Neurocomputing 186:119–126

    Google Scholar 

  42. Jian JG, Wan P (2017) Lagrange \(\alpha \)-exponential stability and \(\alpha \)-exponential convergence for fractional-order complex-valued neural networks. Neural Netw 91:1–10

    Google Scholar 

Download references

Acknowledgements

The authors are grateful for the support of the National Natural Science Foundation of China (11601268).

Author information

Authors and Affiliations

  1. College of Science, China Three Gorges University, Yichang, 443002, Hubei, China

    Peng Wan & Jigui Jian

  2. Three Gorges Mathematical Research Center, China Three Gorges University, Yichang, 443002, Hubei, China

    Jigui Jian

Authors
  1. Peng Wan
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Jigui Jian
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Jigui Jian.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wan, P., Jian, J. \(\alpha \)-Exponential Stability of Impulsive Fractional-Order Complex-Valued Neural Networks with Time Delays. Neural Process Lett 50, 1627–1648 (2019). https://doi.org/10.1007/s11063-018-9938-x

Download citation

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11063-018-9938-x

Keywords