Skip to main content
Springer Nature Link
Log in

Stepanov-Like Almost Periodic Dynamics of Clifford-Valued Stochastic Fuzzy Neural Networks with Time-Varying Delays

  • Published:
Neural Processing Letters Aims and scope Submit manuscript

Abstract

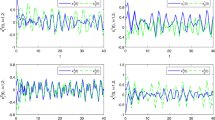
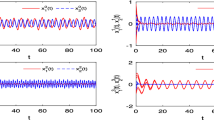
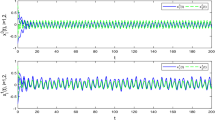
In this paper, we study the Stepanov-like almost periodic dynamics for a class of Clifford-valued stochastic fuzzy neural networks with time-varying delays. Different from the previous studies, we study p-th Stepanov-like almost periodic solutions in distribution, not in p-th mean. Firstly, we study the existence and uniqueness of p-th Stepanov-like almost periodic solutions in distribution of this kind of neural networks by using Banach fixed point theorem. Then, we investigate the global exponential stability of the unique p-th Stepanov-like almost periodic solution by using inequality techniques and counter proof method. Even if the system we consider is a real-valued one, our results are new. Finally, we give an example to illustrate the feasibility of our results.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

References

  1. Buchholz S, Sommer G (2008) On Clifford neurons and Clifford multi-layer perceptrons. Neural Netw 21(7):925–935

    Article  MATH  Google Scholar 

  2. Hitzer E, Nitta T, Kuroe Y (2013) Applications of Clifford’s geometric algebra. Adv Appl Clifford Algebras 23(2):377–404

    Article  MathSciNet  MATH  Google Scholar 

  3. Li Y, Xiang J (2019) Existence and global exponential stability of anti-periodic solution for Clifford-valued inertial Cohen–Grossberg neural networks with delays. Neurocomputing 332:259–269

    Article  Google Scholar 

  4. Li Y, Huo N, Li B (2021) On \(\mu \)-pseudo almost periodic solutions for Clifford-valued neutral type neural networks with delays in the leakage term. IEEE Trans Neural Netw Learn Syst 32(3):1365–1374

    Article  MathSciNet  Google Scholar 

  5. Chaouki A, Touati F (2020) Global dissipativity of Clifford-valued multidirectional associative memory neural networks with mixed delays. Comput Appl Math 39:310

    Article  MathSciNet  MATH  Google Scholar 

  6. Aouiti C, Dridi F (2020) Weighted pseudo almost automorphic solutions for neutral type fuzzy cellular neural networks with mixed delays and D operator in Clifford algebra. Int J Syst Sci 51(10):1759–1781

    Article  MathSciNet  MATH  Google Scholar 

  7. Aouiti C, Gharbia IB (2020) Dynamics behavior for second-order neutral Clifford differential equations: inertial neural networks with mixed delays. Comput Appl Math 39(2):1–31

    Article  MathSciNet  MATH  Google Scholar 

  8. Li Y, Wang Y, Li B (2019) The existence and global exponential stability of \(\mu \)-pseudo almost periodic solutions of Clifford-valued semi-linear delay differential equations and an application. Adv Appl Clifford Algebras 29:105

    Article  MathSciNet  MATH  Google Scholar 

  9. Li Y, Shen S (2020) Almost automorphic solutions for Clifford-valued neutral-type fuzzy cellular neural networks with leakage delays on time scales. Neurocomputing 417:23–35

    Article  Google Scholar 

  10. Rajchakit G, Sriraman R, Boonsatit N, Hammachukiattikul P, Lim CP, Agarwal P (2021) Global exponential stability of Clifford-valued neural networks with time-varying delays and impulsive effects. Adv Differ Equ 2021:208

    Article  MathSciNet  MATH  Google Scholar 

  11. Rajchakit G, Sriraman R, Vignesh P, Lim CP (2021) Impulsive effects on Clifford-valued neural networks with time-varying delays: an asymptotic stability analysis. Appl Math Comput 407:126309

    MathSciNet  MATH  Google Scholar 

  12. Rajchakit G, Sriraman R, Lim CP, Unyong B (2021) Existence, uniqueness and global stability of Clifford-valued neutral-type neural networks with time delays. Math Comput Simul. https://doi.org/10.1016/j.matcom.2021.02.023

    Article  MATH  Google Scholar 

  13. Yang T, Yang LB, Wu CW, Chua LO (1996) Fuzzy cellular neural networks: applications. In: Proceedings of the IEEE international workshop on cellular neural networks and applications, pp 225–230

  14. Chua LO, Yang L (1988) Cellular neural networks: theory. IEEE Trans Circuits Syst 35:1257–1272

    Article  MathSciNet  MATH  Google Scholar 

  15. Chua LO, Yang L (1988) Cellular neural networks: applications. IEEE Trans Circuits Syst 35:1273–1290

    Article  MathSciNet  Google Scholar 

  16. Yang T, Yang LB (1996) The global stability of fuzzy cellular neural network. IEEE Trans Circuits Syst 43(10):880–883

    Article  MathSciNet  Google Scholar 

  17. Yang T, Yang CM, Yang LB (1998) The differences between cellular neural network based and fuzzy cellular neural network based mathematical morphological operations. Int J Circuit Theory Appl 26(1):13–25

    Article  MATH  Google Scholar 

  18. Huang C, Cao J (2011) Convergence dynamics of stochastic Cohen–Grossberg neural networks with unbounded distributed delays. IEEE Trans Neural Netw 22(4):561–572

    Article  Google Scholar 

  19. Chen J, Li C, Yang X (2018) Asymptotic stability of delayed fractional-order fuzzy neural networks with impulse effects. J Frankl Inst 355(15):7595–7608

    Article  MathSciNet  MATH  Google Scholar 

  20. Sevgen S (2019) New stability results for Takagi–Sugeno fuzzy Cohen–Grossberg neural networks with multiple delays. Neural Netw 114:60–66

    Article  MATH  Google Scholar 

  21. Aouiti C, Hui Q, Jallouli H, Moulay E (2021) Fixed-time stabilization of fuzzy neutral-type inertial neural networks with time-varying delay. Fuzzy Sets Syst 411:48–67

    Article  MathSciNet  MATH  Google Scholar 

  22. Kong F, Zhu Q, Huang T (2020) New fixed-time stability lemmas and applications to the discontinuous fuzzy inertial neural networks. IEEE Trans Fuzzy Syst 29(12):3711–3722

    Article  Google Scholar 

  23. Kong F, Zhu Q, Sakthivel R, Mohammadzadeh A (2021) Fixed-time synchronization analysis for discontinuous fuzzy inertial neural networks with parameter uncertainties. Neurocomputing 422:295–313

    Article  Google Scholar 

  24. Shen S, Li Y (2020) \(S^{p}\)-almost periodic solutions of Clifford-valued fuzzy cellular neural networks with time-varying delays. Neural Process Lett 51:1749–1769

    Article  Google Scholar 

  25. Fei J, Wang Z, Liang X, Feng Z, Xue Y (2021) Fractional sliding mode control for micro gyroscope based on multilayer recurrent fuzzy neural network. IEEE Trans Fuzzy Syst. https://doi.org/10.1109/TFUZZ.2021.3064704

    Article  Google Scholar 

  26. Wan P, Sun D, Zhao M, Huang S (2021) Multistability for almost-periodic solutions of Takagi–Sugeno fuzzy neural networks with nonmonotonic discontinuous activation functions and time-varying delays. IEEE Trans Fuzzy Syst 29(12):400–414

    Article  Google Scholar 

  27. Wang J, Zhang Y, Su L, Park JH, Shen H (2021) Fuzzy-model-based \(l_{2}-l_{\infty }\) filtering for discrete-time semi-Markov jump nonlinear systems using semi-Markov kernel. IEEE Trans Fuzzy Syst. https://doi.org/10.1109/TFUZZ.2021.3078832

    Article  Google Scholar 

  28. Liu X, Xia J, Wang J, Shen H (2021) Interval type-2 fuzzy passive filtering for nonlinear singularly perturbed PDT-switched systems and its application. J Syst Sci Complex 34:2195–2218

    Article  MathSciNet  MATH  Google Scholar 

  29. Liao X, Mao X (1996) Exponential stability and instability of stochastic neural networks. Stoch Anal Appl 14(2):165–185

    Article  MathSciNet  MATH  Google Scholar 

  30. Blythe S, Mao X, Liao X (2001) Stability of stochastic delay neural networks. J Frankl Inst 338:481–495

    Article  MathSciNet  MATH  Google Scholar 

  31. Chen Y, Zheng W (2013) Stability analysis of time delay neural networks subject to stochastic perturbations. IEEE Trans Cybern 43:2122–2134

    Article  Google Scholar 

  32. Wan P, Sun D, Zhao M (2020) Finite-time and fixed-time anti-synchronization of Markovian neural networks with stochastic disturbances via switching control. Neural Netw 123:1–11

    Article  MATH  Google Scholar 

  33. Chen T, Peng S, Zhang Z (2020) Finite-time stabilization of stochastic neural networks with time-varying delay via impulsive control. In: 2020 IEEE International conference on power, intelligent computing and systems, Shenyang, China, pp 441–446

  34. Xu D, Liu Y, Liu M (2021) Finite-time synchronization of multi-coupling stochastic fuzzy neural networks with mixed delays via feedback control. Fuzzy Sets Syst 411:85–104

    Article  MathSciNet  MATH  Google Scholar 

  35. Wang P, Li S, Su H (2021) Aperiodically intermittent stabilization for complex-valued hybrid stochastic delayed systems: an average technique. Commun Nonlinear Sci Numer Simul 101:105852

    Article  MathSciNet  MATH  Google Scholar 

  36. Zhou H, Luo M, Li W (2021) Intermittent delay stabilization of complex-valued stochastic complex network. Inf Sci 577:379–397

    Article  MathSciNet  Google Scholar 

  37. Shen H, Hu X, Wang J, Cao J, Qian W (2021) Non-fragile \(H_\infty \) synchronization for Markov jump singularly perturbed coupled neural networks subject to double-layer switching regulation. IEEE Trans Neural Netw Learn Syst. https://doi.org/10.1109/TNNLS.2021.3107607

    Article  Google Scholar 

  38. Wang Y, Chen F, Zhuang G (2020) Dynamic event-based reliable dissipative asynchronous control for stochastic Markov jump systems with general conditional probabilities. Nonlinear Dyn 101:465–485

    Article  Google Scholar 

  39. Wang Y, Chen F, Zhuang G, Song G (2021) Event-based asynchronous and resilient filtering for Markov jump singularly perturbed systems against deception attacks. ISA Trans 112:56–73

    Article  Google Scholar 

  40. Wang Y, Chen F, Zhuang G, Yang G (2020) Dynamic event-based mixed \(H_\infty \) and dissipative asynchronous control for Markov jump singularly perturbed systems. Appl Math Comput 386:125443

    MathSciNet  MATH  Google Scholar 

  41. Sun L, Su L, Wang J (2021) Non-fragile dissipative state estimation for semi-Markov jump inertial neural networks with reaction–diffusion. Appl Math Comput 411:126404

    MathSciNet  MATH  Google Scholar 

  42. Wang L, Lu W, Chen T (2009) Multistability and new attraction basins of almost-periodic solutions of delayed neural networks. IEEE Trans Neural Netw 20(10):1581–1593

    Article  Google Scholar 

  43. Liu B, Huang L (2008) Positive almost periodic solutions for recurrent neural networks. Nonlinear Anal Real World Appl 9(3):830–841

    Article  MathSciNet  MATH  Google Scholar 

  44. Huang Z (2017) Almost periodic solutions for fuzzy cellular neural networks with multi-proportional delays. Int J Mach Learn Cybern 8(4):1323–1331

    Article  Google Scholar 

  45. Ammar B, Chérif F, Alimi AM (2011) Existence and uniqueness of pseudo almost-periodic solutions of recurrent neural networks with time-varying coefficients and mixed delays. IEEE Trans Neural Netw Learn Syst 23(1):109–118

    Article  Google Scholar 

  46. Kong F, Zhu Q, Wang K, Nieto JJ (2019) Stability analysis of almost periodic solutions of discontinuous BAM neural networks with hybrid time-varying delays and D operator. J Frankl Inst 356(18):11605–11637

    Article  MathSciNet  MATH  Google Scholar 

  47. Amdouni M, Chérif F (2018) The pseudo almost periodic solutions of the new class of Lotka–Volterra recurrent neural networks with mixed delays. Chaos Solitons Fractals 113:79–88

    Article  MathSciNet  MATH  Google Scholar 

  48. Abdelaziz M, Chérif F (2020) Piecewise asymptotic almost periodic solutions for impulsive fuzzy Cohen–Grossberg neural networks. Chaos Solitons Fractals 132:109575

    Article  MathSciNet  MATH  Google Scholar 

  49. Chérif F, Abdelaziz M (2020) Stepanov-like pseudo almost periodic solution of quaternion-valued for fuzzy recurrent neural networks with mixed delays. Neural Process Lett 51:2211–2243

    Article  Google Scholar 

  50. Li Y, Xiang J (2020) Existence and global exponential stability of almost periodic solution for quaternion-valued high-order Hopfield neural networks with delays via a direct method. Math Methods Appl Sci 43(10):6165–6180

    Article  MathSciNet  MATH  Google Scholar 

  51. Huang C, Yang H, Cao J (2021) Weighted pseudo almost periodicity of multi-proportional delayed shunting inhibitory cellular neural networks with D operator. Discrete Contin Dyn Syst S 14(4):1259–1272

    Article  MathSciNet  MATH  Google Scholar 

  52. Huang C, Liu B, Qian C, Cao J (2021) Stability on positive pseudo almost periodic solutions of HPDCNNs incorporating D operator. Math Comput Simul 190:1150–1163

    Article  MathSciNet  MATH  Google Scholar 

  53. Li Y, Wang X (2021) Almost periodic solutions in distribution of Clifford-valued stochastic recurrent neural networks with time-varying delays. Chaos Solitons Fractals 153:111536

    Article  MathSciNet  MATH  Google Scholar 

  54. Mellah O, Fitte PD (2013) Counterexamples to mean square almost periodicity of the solutions of some SDEs with almost periodic coefficients. Electron J Differ Equ 2013(19):1–7

    MathSciNet  MATH  Google Scholar 

  55. Brackx F, Delanghe R, Sommen F (1982) Clifford analysis. Pitman Books Limited, Boston

    MATH  Google Scholar 

  56. Dudley RM (1989) Real analysis and probability. CRC Press, New York

    MATH  Google Scholar 

  57. Corduneanu C (2009) Almost periodic oscillations and waves. Springer, New York

    Book  MATH  Google Scholar 

  58. Liu Z, Sun K (2014) Almost automorphic solutions for stochastic differential equations driven by Lévy noise. J Funct Anal 266(3):1115–1149

    Article  MathSciNet  MATH  Google Scholar 

  59. Morozan T, Tudor C (1989) Almost periodic solutions of affine Itô equations. Stoch Anal Appl 7(4):451–474

    Article  MATH  Google Scholar 

  60. Prato GD, Tudor C (1995) Periodic and almost periodic solutions for semilinear stochastic equations. Stoch Anal Appl 13(1):13–33

    Article  MathSciNet  MATH  Google Scholar 

  61. Hu S, Huang C, Wu F (2008) Stochastic differential equations. Science Press, Beijing

    Google Scholar 

  62. Kamenskii M, Mellah O, Paul R (2015) Weak averaging of semilinear stochastic differential equations with almost periodic coefficients. J Math Anal Appl 427(1):336–364

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The authors would like to thank the Editor and the anonymous referees for their helpful comments and valuable suggestions regarding this article.

Funding

This work is supported by the National Natural Science Foundation of China under Grant No. 11861072 and the Applied Basic Research Foundation of Yunnan Province under Grant No. 2019FB003.

Author information

Author notes

  1. Xiaohui Wang and Bing Li have contributed equally to this work.

Authors and Affiliations

  1. Department of Mathematics, Yunnan University, Kunming, 650091, Yunnan, China

    Yongkun Li & Xiaohui Wang

  2. School of Mathematics and Computer Science, Yunnan Minzu University, Kunming, 650500, Yunnan, China

    Bing Li

Authors
  1. Yongkun Li
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Xiaohui Wang
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Bing Li
    View author publications

    You can also search for this author inPubMed Google Scholar

Contributions

Each of the authors contributed to each part of this study equally, all authors read and approved the final manuscript.

Corresponding author

Correspondence to Yongkun Li.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interests.

Consent for Publication

All authors agree to publish this paper to Neural Processing Letters.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Li, Y., Wang, X. & Li, B. Stepanov-Like Almost Periodic Dynamics of Clifford-Valued Stochastic Fuzzy Neural Networks with Time-Varying Delays. Neural Process Lett 54, 4521–4561 (2022). https://doi.org/10.1007/s11063-022-10820-x

Download citation

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11063-022-10820-x

Keywords