Skip to main content
SpringerLink
Log in

LMI-Based Synchronization Conditions to R-L Fractional Time-Varying Delayed Neural Networks with Parametric Uncertainty

  • Published:
Neural Processing Letters Aims and scope Submit manuscript

Abstract

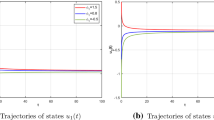
The synchronization conditions to Riemann-Liouville fractional time-varying delayed neural networks with parametric uncertainty are investigated in this article. A novel Lyapunov-Krasovskii functional including double integral terms is proposed, which greatly cutes down on the conservatism about the results. The new synchronization criteria are established and characterized as linear matrix inequalities by using convex combination technique, fractional calculus properties and delay-decomposing method. Finally, two numerical simulations illustrate the correctness of the results.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

References

  1. Anastasio TJ (1998) Nonuniformity in the linear network model of the oculomotor integrator produces approximately fractional-order dynamics and more realistic neuron behavior. Biol Cybern 79:377–391

    MATH  Google Scholar 

  2. Laskin N (2000) Fractional market dynamics. Phys A Stat Mech Appl 287:482–492

    MathSciNet  Google Scholar 

  3. Kulish VV, Lage JL (2002) Application of fractional calculus to fluid mechanics. J Fluids Eng 124:803–806

    Google Scholar 

  4. Magin RL (2010) Fractional calculus models of complex dynamics in biological tissues. Comput Math Appl 59:1586–1593

    MathSciNet  MATH  Google Scholar 

  5. Hilfer R (2000) Applications of fractional calculus in physics. Word Scientific Press, Singapore

    MATH  Google Scholar 

  6. Wang ST, Fu D, Xu M, Hu DW (2005) Applying advanced fuzzy cellular neural network AFFCN to segmentation of serial CT liver images. Int Conf Nat Comput 3612:1128–1131

    Google Scholar 

  7. Bagley RL, Torvik PJ (1983) A theoretical basis for the application of fractional calculus to viscoelasticity. J Rheol 27:201–210

    MATH  Google Scholar 

  8. Song QK, Yang XJ, Li CD, Huang TW, Chen XF (2017) Stability analysis of nonlinear fractional-order systems with variable-time impulses. J Frankl Inst 354:2959–2978

    MathSciNet  MATH  Google Scholar 

  9. Zhang H, Cheng YH, Zhang HM, Zhang WW, Cao JD (2022) Hybrid control design for Mittag-Leffler projective synchronization of FOQVNNs with multiple mixed delays and impulsive effects. Math Comput Simul 197:341–357

    MathSciNet  MATH  Google Scholar 

  10. Ding ZX, Shen Y (2016) Projective synchronization of nonidentical fractional-order neural networks based on sliding mode controlle. Neural Netw 76:97–105

    MATH  Google Scholar 

  11. Li HL, Hu C, Jiang YL, Wang ZL, Teng ZD (2016) Pinning adaptive and impulsive synchronization of fractional-order complex dynamical networks. Chaos Solitons Fractals 92:142–149

    MathSciNet  MATH  Google Scholar 

  12. Zhang H, Cheng JS, Zhang HM, Zhang WW, Cao JD (2021) Quasi-uniform synchronization of Caputo type fractional neural networks with leakage and discrete delays. Chaos Solitons Fractals 152:111432

    MathSciNet  MATH  Google Scholar 

  13. Liu S, Wu X, Zhou XF, Jiang W (2016) Asymptotical stability of Riemann-Liouville fractional nonlinear systems. Nonlinear Dyn 86:65–71

    MathSciNet  MATH  Google Scholar 

  14. Stamova I (2014) Global Mittag-Leffler stability and synchronization of impulsive fractional-order neural networks with time-varying delays. Nonlinear Dyn 77:1251–1260

    MathSciNet  MATH  Google Scholar 

  15. Zhang LZ, Yang YQ, Wang F (2018) Synchronization analysis of fractional-order neural networks with time-varying delays via discontinuous neuron activations. Neurocomputing 275:40–49

    Google Scholar 

  16. Zhang WW, Zhao HY, Sha CL, Wang Y (2021) Finite time synchronization of delayed quaternion valued neural networks with fractional order. Neural Process Lett 53:3607–3618

    Google Scholar 

  17. Wang Z, Li L, Li YX, Cheng ZS (2018) Stability and Hopf bifurcation of a three-neuron network with multiple discrete and distributed delays. Neural Process Lett 48:1481–1502

    Google Scholar 

  18. Liu YA, Xia JW, Meng B, Song XN, Shen H (2020) Extended dissipative synchronization for semi-Markov jump complex dynamic networks via memory sampled-data control scheme. J Frankl Inst 357:10900–10920

    MathSciNet  MATH  Google Scholar 

  19. Lu HT (2002) Chaotic attractors in delayed neural networks. Phys Lett A 298:109–116

    MATH  Google Scholar 

  20. Zhao Y, Gao HJ, Mou SS (2008) Asymptotic stability analysis of neural networks with successive time delay components. Neurocomputing 71:2848–2856

    Google Scholar 

  21. Gu K (2000) An integral inequality in the stability problem of time-delay systems. In: Proceedings of the 39th IEEE conference on decision and control 3:2805–2810

  22. Kharitonova VL, Zhabkob AP (2003) Lyapunov-Krasovskii approach to the robust stability analysis of time-delay systems. Automatica 39:15–20

    MathSciNet  Google Scholar 

  23. Seuret A, Gouaisbaut F (2015) Hierarchy of LMI conditions for the stability analysis of time-delay systems. Syst Control Lett 81:1–7

    MathSciNet  MATH  Google Scholar 

  24. Zhang RM, Zeng DQ, Zhong SM (2017) Novel master-slave synchronization criteria of chaotic Lure systems with time delays using sampled-data control. J Frankl Inst 354:4930–4954

    MATH  Google Scholar 

  25. Pakzad MA, Pakzad S, Nekoui MA (2013) Stability analysis of time-delayed linear fractional-order systems. Int J Control Autom Syst 11:519–525

    Google Scholar 

  26. Zhang H, Ye M, Ye R, Cao JD (2018) Synchronization stability of Riemann-Liouville fractional delay-coupled complex neural networks. Phys A Stat Mech Appl 508:155–165

    MathSciNet  MATH  Google Scholar 

  27. Ding XS, Cao JD, Zhao X, Alsaadi FE (2017) Finite-time stability of fractional order complex-valued neural networks with time delays. Neural Process Lett 46:561–580

    Google Scholar 

  28. Yu ZM, Sun Y, Dai X (2021) Stability and stabilization of the fractional-order power system with time delay. IEEE Trans Circuits Syst II Express Br 68:3446–3450

    Google Scholar 

  29. Zhang H, Ye RY, Cao JD, Alsaedi A (2018) Delay-independent stability of Riemann-Liouville fractional neutral-type delayed neural networks. Neural Process Lett 47:427–442

    Google Scholar 

  30. Liu WZ, Jiang MH, Yan M (2019) Stability analysis of memristor-based time-delay fractional-order neural networks. Neurocomputing 323:117–127

    Google Scholar 

  31. Chen SH, Song QK, Zhao ZJ, Liu YR, Alsaadi FE (2021) Global asymptotic stability of fractional-order complex-valued neural networks with probabilistic time-varying delays. Neurocomputing 450:311–318

    Google Scholar 

  32. Zhang WW, Zhang H, Cao JD, Zhang HM, Chen DY (2020) Synchronization of delayed fractional-order complex-valued neural networks with leakage delay. Phys A Stat Mech Appl 556:124710

    MathSciNet  MATH  Google Scholar 

  33. Xu HT, Zhang CK, Jiang L, Smith J (2017) Stability analysis of linear systems with two additive time-varying delays via delay-product-type Lyapunov functional. Appl Math Model 45:955–964

    MathSciNet  MATH  Google Scholar 

  34. Stamov G, Stamova I (2017) Impulsive fractional-order neural networks with time-varying delays: almost periodic solutions. Neural Comput Appl 28:3307–3316

    MATH  Google Scholar 

  35. Ozcan N, Arik S (2014) New global robust stability condition for uncertain neural networks with time delays. Neurocomputing 142:267–274

    Google Scholar 

  36. Faydasicok O, Arik S (2013) An analysis of stability of uncertain neural networks with multiple time delays. J Frankl Inst 350:1808–1826

    MathSciNet  MATH  Google Scholar 

  37. Faydasicok O, Arik S (2013) A new robust stability criterion for dynamical neural networks with multiple time delays. Neurocomputing 99:290–297

    MATH  Google Scholar 

  38. Li SK, Tang WS, Zhang JX (2012) Guaranteed cost control of synchronisation for uncertain complex delayed networks. Int J Syst Sci 43:566–575

    MathSciNet  MATH  Google Scholar 

  39. He P, Jing CG, Fan T, Chen CZ (2014) Robust decentralized adaptive synchronization of general complex networks with coupling delayed and uncertainties. Complexity 19:10–26

    MathSciNet  Google Scholar 

  40. Yu WW, Cao JD (2007) Adaptive synchronization and lag synchronization of uncertain dynamical system with time delay based on parameter identification. Phys A Stat Mech Appl 375:467–482

    Google Scholar 

  41. Kilbas A, Srivastava H, Trujillo J (2006) Theory and applications of fractional differential equations. Elsevier, New York, pp 110–115

    MATH  Google Scholar 

  42. Zhang HM, Cao JD, Xiong LL (2019) Novel synchronization conditions for time-varying delayed Lur’e system with parametric uncertainty. Appl Math Comput 350:224–236

    MathSciNet  MATH  Google Scholar 

  43. Liu J, Zhang J (2012) Note on stability of discrete-time time-varying delay systems. IET Control Theory Appl 6:335–339

    MathSciNet  Google Scholar 

  44. Ren Y, Feng ZG, Sun GH (2016) Improved stability conditions for uncertain neutral-type systems with time-varying delays. Int J Syst Sci 47:1982–1993

    MathSciNet  MATH  Google Scholar 

  45. Anbalagan P (2022) Delay-independent stability criteria for fractional order time delayed gene regulatory networks in terms of Mittag-Leffler function. Chin J Phys 77:845–860

    MathSciNet  Google Scholar 

Download references

Acknowledgements

This work was supported by the National Natural Science Foundation of China (61833005), the Natural Science Foundation of Anhui Province of China (1908085MA01), the Natural Science Foundation of the Higher Education Institutions of Anhui Province (KJ2019A0573) and the Top Young Talents Program of Higher Learning Institutions of Anhui Province of China (gxyq2019048).

Author information

Authors and Affiliations

  1. School of Mathematics and Physics, Anqing Normal University, Anqing, 246133, People’s Republic of China

    Hongmei Zhang, Hai Zhang & Weiwei Zhang

  2. School of Mathematics, Southeast University, Nanjing, 211189, People’s Republic of China

    Jinde Cao

  3. Yonsei Frontier Lab, Yonsei University, Seoul, 03722, South Korea

    Jinde Cao

Authors
  1. Hongmei Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Hai Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Weiwei Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Jinde Cao
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Hai Zhang.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhang, H., Zhang, H., Zhang, W. et al. LMI-Based Synchronization Conditions to R-L Fractional Time-Varying Delayed Neural Networks with Parametric Uncertainty. Neural Process Lett 55, 4031–4045 (2023). https://doi.org/10.1007/s11063-022-11026-x

Download citation

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11063-022-11026-x

Keywords

Search

Navigation