Skip to main content
SpringerLink
Log in

New a posteriori error estimates for singular boundary value problems

  • Published:
Numerical Algorithms Aims and scope Submit manuscript

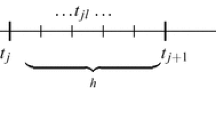
Abstract

In this paper, we discuss the asymptotic properties and efficiency of several a posteriori estimates for the global error of collocation methods. Proofs of the asymptotic correctness are given for regular problems and for problems with a singularity of the first kind. We were also strongly interested in finding out which of our error estimates can be applied for the efficient solution of boundary value problems in ordinary differential equations with an essential singularity. Particularly, we compare estimates based on the defect correction principle with a strategy based on mesh halving.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. U. Ascher, R. Mattheij and R. Russell, Numerical Solution of Boundary Value Problems for Ordinary Differential Equations (Prentice-Hall, Englewood Cliffs, NJ, 1988).

    Google Scholar 

  2. W. Auzinger, E. Karner, O. Koch, D. Praetorius and E. Weinmüller, Globale Fehlerschätzer für Randwertprobleme mit einer Singularität zweiter Art, Technical Report ANUM Preprint Nr. 6/03, Institute for Appl. Math. and Numer. Anal., Vienna University of Technology, Austria; available at http://www.math.tuwien.ac.at/~inst115/preprints.htm(2003).

  3. W. Auzinger, G. Kneisl, O. Koch and E. Weinmüller, A collocation code for boundary value problems in ordinary differential equations, Numer. Algorithms 33 (2003) 27–39.

    Article  Google Scholar 

  4. W. Auzinger, O. Koch, J. Petrickovic and E. Weinmüller, Numerical solution of boundary value problems with an essential singularity, Technical Report ANUM Preprint Nr. 3/03, Institute for Appl. Math. and Numer. Anal., Vienna University of Technology, Austria; available at http://www.math.tuwien.ac.at/~inst115/preprints.htm (2003).

  5. W. Auzinger, O. Koch and E. Weinmüller, Analysis of a new error estimate for collocation methods applied to singular boundary value problems, to appear in SIAM J. Numer. Anal; also available at http://www.math.tuwien.ac.at/~inst115/preprints.htm/.

  6. W. Auzinger, O. Koch and E. Weinmüller, Efficient mesh selection for collocation methods applied to singular BVPs, J. Comput. Appl. Math. 180 (2005) 213–227.

    Article  MathSciNet  Google Scholar 

  7. W. Auzinger, O. Koch and E. Weinmüller, Efficient collocation schemes for singular boundary value problems, Numer. Algorithms 31 (2002) 5–25.

    Article  Google Scholar 

  8. W. Auzinger, O. Koch and E. Weinmüller, New variants of defect correction for boundary value problems in ordinary differential equations, in: Current Trends in Scientific Computing, eds. Z. Chen, R. Glowinski and K. Li, AMS Series in Contemporary Mathematics, Vol. 329 (Amer. Math. Soc., Providence, RI, 2003) pp. 43–50.

    Google Scholar 

  9. W. Auzinger, O. Koch and E. Weinmüller, Collocation methods for boundary value problems with an essential singularity, in: Large-Scale Scientific Computing, eds. I. Lirkov, S. Margenov, J. Wasniewski and P. Yalamov, Lecture Notes in Computer Science, Vol. 2907 (Springer, New York, 2004) pp. 347–354.

    Google Scholar 

  10. Z. Belhachmi, B. Brighi and K. Taous, On the concave solutions of the Blasius equation, Acta Math. Univ. Comen. New. Ser. 69(2) (2000) 199–214.

    Google Scholar 

  11. C. d. Boor and B. Swartz, Collocation at Gaussian points, SIAM J. Numer. Anal. 10 (1973) 582–606.

    Article  Google Scholar 

  12. C. Budd and V. Dorodnitsyn, Symmetry-adapted moving mesh schemes for the nonlinear Schrödinger equation, J. Phys. A Meth. Gen. 34 (2001) 10387–10400.

    Article  Google Scholar 

  13. M. Drmota, R. Scheidl, H. Troger and E. Weinmüller, On the imperfection sensitivity of complete spherical shells, Comp. Mech. 2 (1987) 63–74.

    Article  Google Scholar 

  14. R. Frank and C. Überhuber, Iterated defect correction for differential equations, part I: Theoretical results, Computing 20 (1978) 207–228.

    Google Scholar 

  15. F. d. Hoog and R. Weiss, Difference methods for boundary value problems with a singularity of the first kind, SIAM J. Numer. Anal. 13 (1976) 775–813.

    Article  Google Scholar 

  16. F. d. Hoog and R. Weiss, Collocation methods for singular boundary value problems, SIAM J. Numer. Anal. 15 (1978) 198–217.

    Article  Google Scholar 

  17. F. d. Hoog and R. Weiss, The numerical solution of boundary value problems with an essential singularity, SIAM J. Numer. Anal. 16 (1979) 637–669.

    Article  Google Scholar 

  18. F. d. Hoog and R. Weiss, On the boundary value problem for systems of ordinary differential equations with a singularity of the second kind, SIAM J. Math. Anal. 11 (1980) 41–60.

    Article  Google Scholar 

  19. T. Kapitula, Existence and stability of singular heteroclinic orbits for the Ginzburg–Landau equation, Nonlinearity 9 (1996) 669–685.

    Article  Google Scholar 

  20. O. Koch, Asymptotically correct error estimation for collocation methods applied to singular boundary value problems, Numer. Math. 101(1) (2005) 143–164.

    Article  Google Scholar 

  21. O. Koch and E. Weinmüller, Analytical and numerical treatment of a singular initial value problem in avalanche modeling, Appl. Math. Comput. 148(2) (2003) 561–570.

    Article  Google Scholar 

  22. M. Lentini and H. Keller, Boundary value problems on semi-infinite intervals and their numerical solution, SIAM J. Numer. Anal. 17(3) (1980) 577–604.

    Article  Google Scholar 

  23. P. Markowich, Asymptotic analysis of von Karman flows, SIAM J. Appl. Math. 42(3) (1982) 549–557.

    Article  Google Scholar 

  24. P. Markowich and C. Ringhofer, Collocation methods for boundary value problems on “long” intervals Math. Comp. 40 (1983) 123–150.

    Google Scholar 

  25. H.J. Stetter, The defect correction principle and discretization methods, Numer. Math. 29 (1978) 425–443.

    Article  Google Scholar 

  26. E. Weinmüller, Collocation for singular boundary value problems of second order, SIAM J. Numer. Anal. 23 (1986) 1062–1095.

    Article  Google Scholar 

  27. P. Zadunaisky, On the estimation of errors propagated in the numerical integration of ODEs, Numer. Math. 27 (1976) 21–39.

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Vienna University of Technology, Vienna, Austria

    Winfried Auzinger, Othmar Koch, Dirk Praetorius & Ewa Weinmüller

Authors
  1. Winfried Auzinger
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Othmar Koch
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Dirk Praetorius
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Ewa Weinmüller
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Winfried Auzinger.

Additional information

Communicated by C. Brezinski

AMS subject classification

65L05

Supported in part by the Austrian Research Fund (FWF) grant P-15072-MAT and SFB Aurora.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Auzinger, W., Koch, O., Praetorius, D. et al. New a posteriori error estimates for singular boundary value problems. Numer Algor 40, 79–100 (2005). https://doi.org/10.1007/s11075-005-3791-5

Download citation

  • Received:

  • Accepted:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11075-005-3791-5

Keywords

Search

Navigation