Abstract
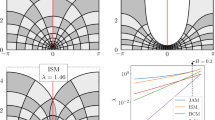
Tensor-product formulae based on one-dimensional Gaussian quadratures are developed for evaluating double integrals of the type indicated in the title. If the singularities occur only along the diagonal and the regular part of the integrand is a polynomial of total degree d, the formulae can be made exact by choosing the number of quadrature points larger than, or equal to, 1 + d/2. Numerical examples are given as well as an application to a problem in aerodynamics.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Ashley, H., Landahl, M.: Aerodynamics of Wings and Bodies. Addison-Wesley, Reading, MA (1965)
Gautschi, W.: Orthogonal Polynomials: Computation and Approximation. Numerical Mathematics and Scientific Computation, Oxford University Press, New York (2004)
Gautschi, W.: Orthogonal polynomials (in MATLAB). J. Comput. Appl. Math. 178, 215–234 (2005)
Liepmann, H.W., Roshko, A.: Elements of Gasdynamics. Aeronautical Ser. Guggenheim Aeronautical Laboratory, California Institute of Technology, Wiley, London (1957)
Author information
Authors and Affiliations
Corresponding author
Additional information
Dedicated to Claude Brezinski and Sebastiano Seatzu on the occasion of their 70th birthdays.
An erratum to this article is available at http://dx.doi.org/10.1007/s11075-013-9787-7.
Rights and permissions
About this article
Cite this article
Gautschi, W. Numerical integration over the square in the presence of algebraic/logarithmic singularities with an application to aerodynamics. Numer Algor 61, 275–290 (2012). https://doi.org/10.1007/s11075-012-9611-9
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11075-012-9611-9
Keywords
- Numerical integration over the square
- Algebraic/logarithmic singular lines along the diagonal and the sides of the square
- Gaussian quadrature
- Aerodynamical drag coefficient