Skip to main content
SpringerLink
Log in

Integrable discretization of nonlinear Schrödinger equation and its application with Fourier pseudo-spectral method

  • Original Paper
  • Published:
Numerical Algorithms Aims and scope Submit manuscript

Abstract

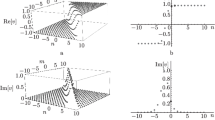
A new integrable discretization of the nonlinear Schr¨odinger (NLS) equation is presented. Different from the one given by Ablowitz and Ladik, we discretize the time variable in this paper. The new discrete system converges to the NLS equation when we take a standard limit and has the same scattering operator as the original NLS equation. This indicates that both the new system and the NLS equation possess the same set of infinite conservation quantities. By applying the Fourier pseudo-spectral method to the space variable, we calculate the first five conservation quantities at different times. The numerical results indeed verify the conservation properties.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Zabusky, N.J., Kruskal, M.D.: Interaction of “Solitons” in a collisionless plasma and the recurrence of initial states. Phys. Rev. Lett. 15, 240–243 (1965)

    Article  MATH  Google Scholar 

  2. Ablowitz, M.J., Prinari, B., Trubatch, A.D.: Discrete and continuous nonlinear Schrdinger systems. Cambridge University Press (2004)

  3. Taha, T.R., Ablowitz, M.J.: Analytical and numerical aspects of certain nonlinear evolution equations. I. Analytical. J. Comput. Phys. 55(2), 192–02 (1984)

    Article  MathSciNet  MATH  Google Scholar 

  4. Taha, T.R., Ablowitz, M.J.: Analytical and numerical aspects of certain nonlinear evolution equations. II. Numerical, nonlinear Schrödinger equation. J. Comput. Phys. 55(2), 203–30 (1984)

    Article  MathSciNet  MATH  Google Scholar 

  5. Ablowitz, M.J., Herbst, B.M.: On homoclinic structure and numerically induced chaos for the nonlinear Schrödinger equation. SIAM J. Appl. Math. 50(2), 339–351 (1990)

    Article  MathSciNet  MATH  Google Scholar 

  6. Herbst, B.M., Ablowitz, M.J.: Numerically induced chaos in the nonlinear Schrödinger equation. Phys. Rev. Lett. 62(18), 2065 (1989)

    Article  MathSciNet  Google Scholar 

  7. Hirota, R., Nagai, J.N., Gilson, C.: Direct Method in Soliton Theory. Cambridge University Press), Cambridge (2004)

    Book  MATH  Google Scholar 

  8. Ablowitz, M.J., Segur, H.: Solitons and the inverse scattering transform. SIAM, Philadelphia (1981)

    Book  MATH  Google Scholar 

  9. Yang, J.: Nonlinear waves in integrable and non-integrable systems. SIAM (2010)

  10. Fornberg, B.: A practical guide to pseudospectral methods. Cambridge University Press, Cambridge (1998)

    MATH  Google Scholar 

  11. Zakharov, V.E., Shabat, A.B.: Exact theory of two-dimensional self-focusing and one-dimensional self-modulation of waves in nonlinear media. Sov. Phys. JETP 34, 62–9 (1972)

    MathSciNet  Google Scholar 

  12. Zakharov, V.E., Shabat, A.B.: Interaction between solitons in a stable medium. Sov. Phys. JETP 37, 823–28 (1973)

    Google Scholar 

  13. Anderson, M.H., Ensher, J.R., Matthews, M.R., Wieman, C.E., Cornell, E.A.: Observation of Bose-Einstein condensation in a dilute atomic vapor. Science 269, 198–201 (1995)

    Article  Google Scholar 

  14. Hasegawa, A., Tappert, F.: Transmission of stationary nonlinear optical pulses in dispersive dielectric fibers: I. Anomalous dispersion. Appl. Phys. Lett. 23, 142–44 (1973)

    Article  Google Scholar 

  15. HASEGAWA, A., TAPPERT, F.: Transmission of stationary nonlinear optical pulses in dispersive dielectric fibers: II. Normal dispersion. Appl. Phys. Lett. 23, 171–72 (1973)

    Article  Google Scholar 

  16. Kivshar, Y.S., Luther-Davies, B.: Dark optical solitons: physics and applications. Phys. Rep. 298, 81–97 (1998)

    Article  Google Scholar 

  17. Carr, L.D., Kutz, J.N., Reinhardt, W.P.: Stability of stationary states in the cubic nonlinear Schrödinger equation: applications to the Bose-Einstein condensate. Phys. Rev. E 63, 066604 (2001)

    Article  Google Scholar 

  18. Carretero-González, R., Frantzeskakis, D.J., Kevrekidis, P.G.: Nonlinear waves in Bose-Einstein condensates: physical relevance and mathematical techniques. Nonlinearity 21, 139–202 (2008)

    Article  MathSciNet  Google Scholar 

  19. Frantzeskakis, D.J.: Dark solitons in atomic Bose-Einstein condensates: from theory to experiments. J. Phys. A: Math. Theor. 43(21), 213001 (2010)

    Article  MathSciNet  Google Scholar 

  20. Akrivis, G.D.: Finite difference discretization of the cubic Schrödinger equation. IMA J. Numer. Anal. 13, 115–24 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  21. Chang, Q.S., Jia, E., Sun, W.: Difference schemes for solving the generalized nonlinear Schrödinger equation. J. Comput. Phys. 148, 397–215 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  22. Delfour, M., Fortin, M., Payre, G.: Finite-difference solutions of a nonlinear Schrödinger equation. J. Comput. Phys. 44, 277–88 (1981)

    Article  MathSciNet  MATH  Google Scholar 

  23. Bao, W.: Numerical methods for the nonlinear Schrödinger equation with nonzero far-field conditions. Methods Appl. Anal. 367–87, 11 (2004)

    Google Scholar 

  24. Shen, J., Tang, T.: Spectral and High-Order Methods with Applications. Science, Beijing (2006)

    MATH  Google Scholar 

  25. Zouraris, G.: On the convergence of a linear two-step finite element method for the nonlinear Schrödinger equation. ESAIM Math. Model. Numer. Anal. 35(03), 389–405 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  26. Bao, W., Jin, S., Markowich, P.A.: On time-splitting spectral approximations for the Schrödinger equation in the semiclassical regime. J. Comput. Phys. 487–524, 175 (2002)

    MathSciNet  Google Scholar 

  27. Besse, C., Bidegaray, B., Descombes, S.: Order estimates in time of splitting methods for the nonlinear Schrödinger equation. SIAM J. Numer. Anal. 26–30, 40 (2002)

    MathSciNet  Google Scholar 

  28. Weideman, J.A.C., Herbst, B.M.: Split-step methods for the solution of the nonlinear Schrödinger equation. SIAM J. Numer. Anal. 485–507, 23 (1986)

    MathSciNet  Google Scholar 

  29. Karakashian, O., Akrivis, G.D., Dougalis, V.A.: On optimal order error estimates for the nonlinear Schrödinger equation. SIAM J. Numer. Anal. 377–400, 30 (1993)

    MathSciNet  Google Scholar 

  30. Islas, A.L., Karpeev, D.A., Schober, C.M.: Geometric integrators for the nonlinear Schrödinger equation. J. Comput. Phys. 173(1), 116–148 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  31. Islas, A.L., Schober, C.M.: On the preservation of phase space structure under multisymplectic discretization. J. Comput. Phys. 197(2), 585–609 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  32. Chen, J.B., Qin, M.Z., Tang, Y.F.: Symplectic and multi-symplectic methods for the nonlinear Schrödinger equation. Comput. Math. Appl. 43(8), 1095–1106 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  33. Matsuo, T., Furihata, D.: Dissipative or conservative finite-difference schemes for complex-valued nonlinear partial differential equations. J. Math. Phys. 171(2), 425–447 (2001)

    MathSciNet  MATH  Google Scholar 

  34. Chiu, S.C., Ladik, J.F.: Generating exactly soluble nonlinear discrete evolution equations by a generalized Wronskian technique. J Math Phys 18, 690 (1977)

    Article  MathSciNet  MATH  Google Scholar 

  35. Levi, D.: Nonlinear differential difference equations as Bäcklund transformations. J. Phys. A Math. Theor. 14, 1083–1098 (1981)

    Article  MATH  Google Scholar 

  36. Ablowitz, M.J., Ladik, J.F.: Nonlinear differential-difference equations and Fourier analysis. J. Math. Phys. 16, 598–603 (1975)

  37. Hirota, R.: Nonlinear partial difference equations I. A difference analogue of the Korteweg-de Vries equation. J. Phys. Soc. Jpn. 43, 1424–1433 (1977)

    Article  MathSciNet  Google Scholar 

  38. Hirota, R.: Nonlinear partial difference equations II. Discrete-time Toda equation. J. Phys. Soc. Jpn. 43, 2074–2078 (1977)

    Article  MathSciNet  Google Scholar 

  39. Hirota, R.: Nonlinear partial difference equations III. Discrete Sine-Gordon equation. J. Phys. Soc. Jpn. 43, 2079–2086 (1977)

    Article  MathSciNet  MATH  Google Scholar 

  40. Date, E., Jimbo, M., Miwa, T.: J. Phys. Soc. Jpn. 51, 4116–4124 (1982)

    Article  Google Scholar 

  41. Date, E., Jimbo, M., Miwa, T.: Methods for generating discrete soliton equations II. J. Phys. Soc. Jpn. 51, 4125–4131 (1982)

    Article  Google Scholar 

  42. Date, E., Jimbo, M., Miwa, T.: Methods for generating discrete soliton equations III. J. Phys. Soc. Jpn. 52, 388–393 (1983)

    Article  MathSciNet  MATH  Google Scholar 

  43. Date, E., Jimbo, M., Miwa, T.: Methods for generating discrete soliton equations IV. J. Phys. Soc. Jpn. 52, 761–765 (1983)

    Article  MathSciNet  MATH  Google Scholar 

  44. Date, E., Jimbo, M., Miwa, T.: Methods for generating discrete soliton equations V. J. Phys. Soc. Jpn. 52, 766–771 (1983)

    Article  Google Scholar 

  45. Levi, D., Pilloni, L., Santini, P.M.: Integrable three-dimensional lattices. J. Phys. A. Math. Gen. 14, 1567–1575 (1981)

    Article  MathSciNet  MATH  Google Scholar 

  46. Levi, D., Benguria, R.: Bäcklund transformations nonlinear differential difference equations. Proc. Natl. Acad. Sci. (USA) 77, 5025–5027 (1980)

    Article  MathSciNet  MATH  Google Scholar 

  47. Nijhoff, F.W., Capel, H.W., Wiersma, G.L., Quispel, G.R.W.: Linearizing integral transform and partial difference equations. Phys. Lett. A 103, 293 (1984)

    Article  MathSciNet  Google Scholar 

  48. Quispel, G.R.W., Nijhoff, F.W., Capel, H.W., Van-der-Linden, J.: Linear integral equations and nonlinear differrence-difference equations. Physica A 125, 344–380 (1984)

    Article  MathSciNet  MATH  Google Scholar 

  49. Faddeev, L., Volkov, A.Y.: Hirota equation as an example of integrable symplectic map. Lett. Math. Phys. 32, 125–135 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  50. Suris, Y.B.: The problem of integrable discretization: Hamiltonian approach. Birkhäuser (2003)

  51. Schiff, J.: Loop groups and discrete KdV equations. Nonlinearity 16, 257–275 (2003)

    Article  MathSciNet  Google Scholar 

  52. Rogers, C., Shadwick, W.F.: Bäcklund transformations and their applications (Volume 161 Mathematics in Science and Engineering)

  53. Nimmo, J.J.C.: A bilinear Bäcklund transformation for the nonlinear Schrödinger equation. Phys. Lett. A 99(6), 279–280 (1983)

    Article  MathSciNet  Google Scholar 

  54. Zhang, Y., Tam, H.W., Hu, X.B.: Integrable discretization of ’time’ and its application on the Fourier pseudospectral method to the Korteweg-de Vries equation. J. Phys. A. Math. Theor. 47, 045202 (2014)

    Article  MathSciNet  Google Scholar 

  55. Miles, J.W.: An envelope soliton problem. SIAM J. Appl. Math. 41(2), 227–230 (1981)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institute of Computational Mathematics and Scientific Engineering Computing, Academy of Mathematics and Systems Science, Chinese Academy of Sciences, Beijing, China

    Y. Zhang & X. B. Hu

  2. Department of Computer Science, Hong Kong Baptist University, Hongkong, China

    H. W. Tam

Authors
  1. Y. Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. X. B. Hu
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. H. W. Tam
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Y. Zhang.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhang, Y., Hu, X.B. & Tam, H.W. Integrable discretization of nonlinear Schrödinger equation and its application with Fourier pseudo-spectral method. Numer Algor 69, 839–862 (2015). https://doi.org/10.1007/s11075-014-9928-7

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11075-014-9928-7

Keywords

Search

Navigation