Skip to main content
Springer Nature Link
Log in

Parameter uniform numerical scheme for time dependent singularly perturbed convection-diffusion-reaction problems with general shift arguments

  • Original Paper
  • Published:
Numerical Algorithms Aims and scope Submit manuscript

Abstract

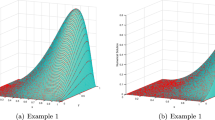
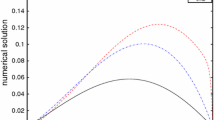
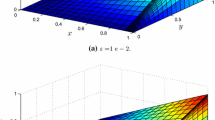
In this work, a parameter uniform numerical method is developed to find the approximate solution of time-dependent singularly perturbed convection-diffusion-reaction problems with general shift arguments in the space variable. The earlier work on such type of initial-interval boundary value problems is restricted to the case of small delay and advance arguments while in practical situations the shift arguments can be of arbitrary size (i.e. it may be bigger or small enough in size). The fitted mesh technique to establish parameter uniform error estimates is not extendable for the class of singularly perturbed parabolic partial differential-difference equations (SPPPDDEs) with general shift arguments in the space variable. To observe the dispersive behaviour of the solution of the problem considered in this paper, we use systematically constructed suitable denominator function for the discrete second order derivative. The motivation behind the construction of the scheme is modelling rules for non-standard finite difference methods (NSFDMs), developed by Mickens. The proposed numerical scheme is analysed for consistency and stability. It is proved that the scheme is unconditionally stable and parameter uniform convergent. The scheme is convergent for bigger shift arguments as well as for small shift arguments. The performance of the method is corroborated by numerical examples.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Ansari, A.R., Bakr, S., Shishkin, G.I.: A parameter-robust finite difference method for singularly perturbed delay parabolic partial differential equations. J. Comput. Appl. Math. 205(1), 552–566 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  2. Bansal, K., Rai, P., Sharma, K.K.: Numerical treatment for the class of time dependent singularly perturbed parabolic problems with general shift arguments. Differ. Equ. Dyn. Syst. 1–20 (2015)

  3. Bashier, E., Patidar, K.C.: A second-order fitted operator finite difference method for a singularly perturbed delay parabolic partial differential equation. J. Differ. Equ. Appl. 17(5), 779–794 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  4. Clavero, C., Gracia, J.L.: On the uniform convergence of a finite difference scheme for time dependent singularly perturbed reaction-diffusion problems. Appl. Math. Comput. 216(5), 1478–1488 (2010)

    MathSciNet  MATH  Google Scholar 

  5. Clavero, C., Gracia, J.L., Jorge, J.C.: High-order numerical methods for one-dimensional parabolic singularly perturbed problems with regular layers. Numer. Methods Partial Differ. Equ. 21(1), 149–169 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  6. Clavero, C., Jorge, J.C., Lisbona, F.: A uniformly convergent scheme on a nonuniform mesh for convection–diffusion parabolic problems. J. Comput. Appl. Math. 154(2), 415–429 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  7. Cope, D.K., Tuckwell, H.C.: Firing rates of neurons with random excitation and inhibition. J. Theor. Biol. 80(1), 1–14 (1979)

    Article  MathSciNet  Google Scholar 

  8. Farrell, P.A., Hegarty, A.F.: On the determination of the order of uniform convergence. In: Proceedings of 13th IMACS World Congress on Computational and Applied Mathematics, pp 501–502 (1991)

  9. Gowrisankar, S., Natesan, S.: A robust numerical scheme for singularly perturbed delay parabolic initial-boundary-value problems on equidistributed grids. Electron. Trans. Numer. Anal. 41, 376–395 (2014)

    MathSciNet  MATH  Google Scholar 

  10. Jorge, J.C., Bujanda, B.: Numerical methods for evolutionary reaction–diffusion problems with nonlinear reaction terms. J. Comput. Appl. Math. 166(1), 167–180 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  11. Kadalbajoo, M.K., Patidar, K.C., Sharma, K.K.: ε-uniformly convergent fitted methods for the numerical solution of the problems arising from singularly perturbed general DDEs. Appl. Math. Comput. 182(1), 119–139 (2006)

    MathSciNet  MATH  Google Scholar 

  12. Kaushik, A.: Error estimates for a class of partial functional differential equation with small dissipation. Appl. Math. Comput. 226, 250–257 (2014)

    MathSciNet  MATH  Google Scholar 

  13. Kaushik, A., Sharma, M.: A robust numerical approach for singularly perturbed time delayed parabolic partial differential equations. Comput. Math. Model. 23(1), 96–106 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  14. Kumar, D., Kadalbajoo, M.K.: A parameter-uniform numerical method for time-dependent singularly perturbed differential-difference equations. Appl. Math. Model. 35(6), 2805–2819 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  15. Kumar, M., Rao, S.C.S.: High order parameter-robust numerical method for time dependent singularly perturbed reaction–diffusion problems. Computing 90(1), 15–38 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  16. Ladyz̆enskaja, O. A., Solonnikov, V.A., Ural’ceva, N.N.: Linear and Quasi-Linear Equations of Parabolic Type, vol. 23. American Mathematical Society, Providence (1988)

    Google Scholar 

  17. Lánskỳ, P.: On approximations of Stein’s neuronal model. J. Theor. Biol. 107(4), 631–647 (1984)

    Article  Google Scholar 

  18. Lánskỳ, P., Musila, M.: Variable initial depolarization in Stein’s neuronal model with synaptic reversal potentials. Biol. Cybern. 64(4), 285–291 (1991)

    Article  Google Scholar 

  19. Lánskỳ, P., Smith, C.E.: The effect of a random initial value in neural first-passage-time models. Math. Biosci. 93(2), 191–215 (1989)

    Article  MathSciNet  Google Scholar 

  20. Linss, T., Madden, N.: Analysis of an alternating direction method applied to singularly perturbed reaction-diffusion problems. Int. J. Numer. Anal. Model. 7(3), 507–519 (2010)

    MathSciNet  MATH  Google Scholar 

  21. Mickens, R.E.: Nonstandard Finite Difference Models of Differential Equations. World Scientific (1994)

  22. Munyakazi, J.B., Patidar, K.C.: A fitted numerical method for singularly perturbed parabolic reaction-diffusion problems. Comput. Appl. Math. 32(3), 509–519 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  23. Murray, J.D.: Mathematical Biology. Biomathematics Series, vol. 19, 2nd edn. Springer, Berlin (1993)

    Google Scholar 

  24. Murray, J.D.: Mathematical Biology I: An Introduction, vol. 17. Springer, New York (2002)

    Google Scholar 

  25. Musila, M., Lánskỳ, P.: Generalized Stein’s model for anatomically complex neurons. BioSystems 25(3), 179–191 (1991)

    Article  Google Scholar 

  26. Ng-Stynes, M.J., O’Riordan, E., Stynes, M.: Numerical methods for time-dependent convection-diffusion equations. J. Comput. Appl. Math. 21(3), 289–310 (1988)

    Article  MathSciNet  MATH  Google Scholar 

  27. Ramesh, V.P., Kadalbajoo, M.K.: Upwind and midpoint upwind difference methods for time-dependent differential-difference equations with layer behavior. Appl. Math. Comput. 202(2), 453–471 (2008)

    MathSciNet  MATH  Google Scholar 

  28. Rathish Kumar, B.V., Kumar, S.: Convergence of three-step Taylor galerkin finite element scheme based monotone schwarz iterative method for singularly perturbed differential-difference equation. Numer. Funct. Anal. Optim. 36(8), 1029–1045 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  29. Roos, H.G., Stynes, M., Tobiska, L.: Robust Numerical Methods for Singularly Perturbed Differential Equations: Convection-Diffusion-Reaction and Flow Problems, vol. 24. Springer Science & Business Media (2008)

  30. Smith, C.E., Smith, M.V.: Moments of voltage trajectories for Stein’s model with synaptic reversal potentials. J. Theor. Neurobiol. 3, 67–77 (1984)

    Google Scholar 

  31. Stein, R.B.: A theoretical analysis of neuronal variability. Biophys. J. 5(2), 173–194 (1965)

    Article  Google Scholar 

  32. Stein, R.B.: Some models of neuronal variability. Biophys. J. 7(1), 37–68 (1967)

    Article  Google Scholar 

  33. Tuckwell, H.: Firing rates of motoneurons with strong random synaptic excitation. Biol. Cybern. 24(3), 147–152 (1976)

    Article  MATH  Google Scholar 

  34. Tuckwell, H.C., Richter, W.: Neuronal interspike time distributions and the estimation of neurophysiological and neuroanatomical parameters. J. Theor. Biol. 71 (2), 167–183 (1978)

    Article  Google Scholar 

  35. Wilbur, W.J., Rinzel, J.: An analysis of Stein’s model for stochastic neuronal excitation. Biol. Cybern. 45(2), 107–114 (1982)

    Article  MathSciNet  MATH  Google Scholar 

  36. Wilbur, W.J., Rinzel, J.: A theoretical basis for large coefficient of variation and bimodality in neuronal interspike interval distributions. J. Theor. Biol. 105(2), 345–368 (1983)

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, (Center for Advance Study in Mathematics), Panjab University, Chandigarh, 160014, India

    Komal Bansal & Kapil K. Sharma

  2. South Asian University, New Delhi, 110021, India

    Kapil K. Sharma

Authors
  1. Komal Bansal
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Kapil K. Sharma
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Kapil K. Sharma.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Bansal, K., Sharma, K.K. Parameter uniform numerical scheme for time dependent singularly perturbed convection-diffusion-reaction problems with general shift arguments. Numer Algor 75, 113–145 (2017). https://doi.org/10.1007/s11075-016-0199-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11075-016-0199-3

Keywords

Mathematics Subject Classification (2010)