Abstract
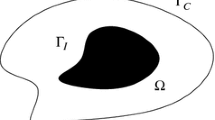
A data completion method is proposed for solving Cauchy problems for which the number of data is less than the number of unknowns. This method is presented on the Cauchy problem for the Laplace equation in 2D situations. The idea is to search the solution in a space of discrete harmonic functions for which the existence and the uniqueness of a discrete harmonic continuation are guaranteed. Many numerical simulations using the finite element method highlight the efficiency, accuracy, and stability of this method even when data are noisy.
Similar content being viewed by others
References
Andrieux, S., Baranger, T.N., Ben Abda, A.: Solving Cauchy problems by minimizing an energy-like functional. Inverse Prob. 22, 115–133 (2006)
Bourgeois, L.: A mixed formulation of quasi-reversibility to solve the Cauchy problem for the Laplace equation. Inverse Prob. 21, 1087–1104 (2005)
CASTEM 2000: Code de calcul pour l’analyse de structures par la méthode des éléments finis. Guide d’utilisation. Commissariat l’Energie Atomique, DEN/DM2S/SEMT/LM2S, F-91191 Gif-sur-Yvette, France (1998)
Chen, K.H., Kao, J.H., Chen, J.T., Wu, K.L.: Desingularized meshless method for solving Laplace equation with over-specified boundary conditions using regularization techniques. Comput. Mech. 43, 827–837 (2009)
Cimetière, A., Delvare, F., Pons, F.: Une méthode inverse avec régularisation évanescente. Comptes Rendus de l’Académie des Sciences - Série IIb: Mécanique 328, 639–644 (2000)
Cimetière, A., Delvare, F., Jaoua, M., Pons, F.: Solution of the Cauchy problem using iterated Tikhonov regularisation. Inverse Prob. 17, 553–570 (2001)
Cimetière, A., Delvare, F., Jaoua, M., Pons, F.: An inversion method for harmonic functions reconstruction. Int. J. Therm. Sci. 41, 509–516 (2002)
Cimetière, A., Delvare, F., Pons, F.: Une méthode inverse d’ordre un pour les problèmes de complétion de données. C. R. Mec. 333, 123–126 (2005)
Delvare, F., Cimetière, A., Pons, F.: An iterative boundary element method for Cauchy inverse problems. Comput. Mech. 28, 291–302 (2002)
Delvare, F., Cimetière, A.: A first order method for the Cauchy problem for the Laplace equation using BEM. Comput. Mech. 41, 789–796 (2008)
Delvare, F., Cimetière, A., Hanus, J.L., Bailly, P.: An iterative method for the Cauchy problem in linear elasticity with fading regularization effect. Comput. Methods Appl. Mech. Eng. 199, 3336–3344 (2010)
Delvare, F., Cimetière, A.: A robust data completion method for two dimensional Cauchy problems associated with the Laplace equation. European Journal of Computational Mechanics 20, 309–340 (2011)
Durand, B., Delvare, F., Bailly, P.: Numerical solution of Cauchy problems in linear elasticity in axisymmetric situations. Int. J. Solids Struct. 48, 3041–3053 (2011)
Engl, H.W., Hanke, M., Neubauer, A.: Regularization of Inverse Problems. Kluwer Academic Publishers, Boston (1996)
Hadamard, J.: Lectures on Cauchy’s Problem in Linear Partial Differential Equations. Yale University Press, New Haven (1923)
Hansen, P.C.: Analysis of discrete ill-posed problems by means of the L-curve. SIAM Rev. 34, 561–580 (1992)
Hayashi, K., Ohura, Y., Onishi, K.: Direct method of solution for general boundary value problem of the Laplace equation. Engineering Analysis with Boundary Elements 26, 763–771 (2002)
Hayashi, K., Onishi, K., Ohura, Y.: Direct numerical identification of boundary values in the Laplace equation. J. Comput. Appl. Math. 152, 161–174 (2003)
Jourhmane, M., Lesnic, D., Mera, N.S.: Relaxation procedures for an iterative algorithm for solving the Cauchy problem for the Laplace equation. Eng. Anal. Bound. Elem. 28, 655–665 (2004)
Klibanov, M.V., Santosa, F.: A computational quasi-reversibility method for Cauchy problems for Laplace’s equation. SIAM J. Appl. Math. 51, 1653–1675 (1991)
Kozlov, V.A., Maz’ya, V.G., Fomin, A.V.: An iterative method for solving the Cauchy problem for elliptic equations. Comput. Math. Math. Phys. 31, 45–52 (1991)
Jin, B., Zou, J.: A Bayesian inference approach to the ill-posed Cauchy problem of steady-state heat conduction. Int. J. Numer. Methods Eng. 76, 521–544 (2008)
Lattès, R., Lions, J.L.: Méthode de quasi-réversibilité et applications. Dunod, Paris (1967)
Lesnic, D., Elliot, L., Ingham, D.B.: An iterative boundary element method for solving numerically the Cauchy problem for the Laplace equation. Eng. Anal. Bound. Elem. 20, 123–133 (1997)
Marin, L.: Numerical solution of the Cauchy problem for steady-state heat transfer in two-dimensional functionally graded materials. Int. J. Solids Struct. 42, 4338–4351 (2005)
Marin, L.: An iterative MFS algorithm for the Cauchy problem associated with the Laplace equation. Comput. Model. Eng. Sci. 48, 121–152 (2009)
Marin, L.: An alternating iterative M.F.S. Algorithm for the Cauchy problem in two-dimensional anisotropic heat conduction. Computers, Materials & Continua 12, 71–99 (2009)
Marin, L.: Relaxation procedures for an iterative MFS algorithm for two-dimensional steady-state isotropic heat conduction Cauchy problems. Eng. Anal. Bound. Elem. 35, 415–429 (2011)
Rischette, R., Baranger, T.N., Debit, N.: Numerical analysis of an energy-like minimization method to solve the Cauchy problem with noisy data. J. Comput. Appl. Math. 235, 3257–3269 (2011)
Tikhonov, A.N., Arsenin, V.Y.: Solution of Ill-Posed Problems. Wiley, New York (1977)
Wahba, G.: Practical approximate solutions to linear operator equations when the data are noisy. SIAM J. Numer. Anal. 14, 651–667 (1977)
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Delvare, F., Cimetière, A. Unique discrete harmonic continuation and data completion problems using the fading regularization method. Numer Algor 75, 731–751 (2017). https://doi.org/10.1007/s11075-016-0218-4
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11075-016-0218-4