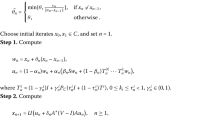Abstract
In this paper, we suggest and analyze a Krasnoselski-Mann type iterative method to approximate a common element of solution sets of a hierarchical fixed point problem for nonexpansive mappings and a split mixed equilibrium problem. We prove that sequences generated by the proposed iterative method converge weakly to a common element of solution sets of these problems. Further, we derive some consequences from our main result. Furthermore, we extend the considered iterative method to a split monotone variational inclusion problem and deduce some consequences. Finally, we give a numerical example to justify the main result. The method and results presented in this paper generalize and unify the corresponding known results in this area.
Similar content being viewed by others
References
Bnouhachem, A.: Algorithms of common solution for a variational inequality, a split equilibrium problem and a hierarchical fixed point problem. Fixed Point Theory Appl. 2013, Article ID 278 (2013)
Bnouhachem, A.: Strong convergence algorithm for split equilibrium problem and hierarchical fixed point problems. Sci. World J. Article ID 390956 (2014)
Blum, E., Oettli, W.: From optimization and variational inequalities to equilibrium problems. Math. Stud. 63, 123–145 (1994)
Bre~zis, H.: Operateurs maximaux monotones et semi-groupes de contractions dans les espaces de Hilbert. Math. Stud. (Amsterdam: North-Holand) 5, 759–775 (1973)
Byrne, C.: Iterative oblique projection onto convex sets and the split feasibility problem. Inverse Probl. 18, 441–453 (2002)
Byrne, C.: A unified treatment of some iterative algorithms in signal processing and image reconstruction. Inverse Probl. 20, 103–120 (2004)
Byrne, C., Censor, Y., Gibali, A., Reich, S.: The split common null point problem. J. Nonlinear Convex Anal. 13(4), 759–775 (2012)
Cabot, A.: Proximal point algorithm controlled by a slowly vanishing term: application to hierarchical minimization. SIAM J. Optim. 15, 555–572 (2005)
Censor, Y., Elfving, T.: A multiprojection algorithm using Bregman projections in product space. Numer. Algorithms 8, 221–239 (1994)
Censor, Y., Bortfeld, T., Martin, B., Trofimov, A.: A unified approach for inversion problems in intensity modulated radiation therapy. Phys. Med. Biol. 51, 2353–2365 (2006)
Censor, Y., Gibali, A., Reich, S.: Algorithms for the split variational inequality problem. Numer. Algorithms 59(2), 301–323 (2012)
Combettes, P. L.: The convex feasibility problem in image recovery. Adv. Imag. Electron Phys. 95, 155–453 (1996)
Combettes, P.L.: Quasi-Fejerian analysis of some optimization algorithms. In: Butnariu, D., Censor, Y., Riech, S. (eds.) Inherently Parallel Algorithms in Feasibility and Optimization and Their Applications, pp 115–152. Elsevier, New York (2001)
Combettes, P.L., Hirstoaga, S.A.: Equilibrium programming in Hilbert spaces. J. Nonlinear Convex Anal. 6, 117–136 (2005)
Geobel, K., Kirk, W. A.: Topics in metric fixed point theory Cambridge Studies in Advanced Mathematics, vol. 28. Cambridge University Press, Cambridge (1990)
Kazmi, K. R., Rizvi, S.H.: Iterative approximation of a common solution of a split equilibrium problem, a variational inequality problem and a fixed point problem. J. Egyptian Math. Soc. 21, 44–51 (2013)
Kazmi, K. R., Rizvi, S. H.: An iterative method for split variational inclusion problem and fixed point problem for a nonexpansive mapping. Optim. Lett. 8, 1113–1124 (2014)
Luo, Z. Q., Pang, J. S., Ralph, D.: Mathematical programs with equilibrium constraints. Cambridge University Press, Cambridge (1996)
Marino, G., Xu, H. K.: Weak and strong convergence theorems for strict pseudocontractions in Hilbert space. J. Math. Anal. Appl. 329, 336–346 (2007)
Marino, G., Colao, V., Muglia, L., Yao, Y.: Krasnoselski-Mann iteration for hierarchical fixed-points and equilibrium problem. Bull. Aust. Math. Soc. 79, 187–200 (2009)
Moudafi, A., Théra, M.: Proximal and dynamical approaches to equilibrium problems, Lecture Notes in Economics and Mathematical Systems, vol. 477, pp 187–201. Springer-Verlag, New York (1999)
Moudafi, A., Mainge, P. -E.: Towards viscosity approximations of hierarchical fixed-point problems. Fixed Point Theory Appl. 2006, Article ID 95453 (2006)
Moudafi, A.: Krasnoselski-Mann iteration for hierarchical fixed-point problems. Inverse Probl. 23, 1635–1640 (2007)
Moudafi, A., Mainge, P. -E.: Strong convergence of an iterative method for hierarchical fixed-point problems. Pac. J. Optim. 3, 529–538 (2007)
Moudafi, A.: Split monotone variational inclusions. J. Optim. Theory Appl. 150, 275–283 (2011)
Yao, Y., Liou, Y. C.: Weak and strong convergence of Krasnoselski-Mann iteration for hierarchical fixed-point problems. Inverse Probl. 24, 501–508 (2008)
Yamada, I., Ogura, N.: Hybrid steepest descent method for the variational inequality problem over the fixed point set of certain quasi-nonexpansive mappings. Numer. Funct. Anal. Optim. 25, 619–655 (2004)
Yang, Q., Zhao, J.: Generalized KM theorem and their applications. Inverse Probl. 22, 833–844 (2006)
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Kazmi, K.R., Ali, R. & Furkan, M. Krasnoselski-Mann type iterative method for hierarchical fixed point problem and split mixed equilibrium problem. Numer Algor 77, 289–308 (2018). https://doi.org/10.1007/s11075-017-0316-y
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11075-017-0316-y
Keywords
- Hierarchical fixed point problem
- Split mixed equilibrium problem
- Split monotone variational inclusion problem
- Krasnoselski-Mann type iterative method
- Nonexpansive mapping
- Weak convergence