Skip to main content
SpringerLink
Log in

Iterative method with inertial terms for nonexpansive mappings: applications to compressed sensing

  • Original Paper
  • Published:
Numerical Algorithms Aims and scope Submit manuscript

Abstract

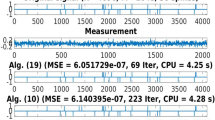
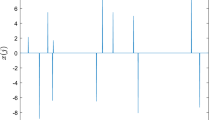
Our interest in this paper is to introduce a Halpern-type algorithm with both inertial terms and errors for approximating fixed point of a nonexpansive mapping. We obtain strong convergence of the sequence generated by our proposed method in real Hilbert spaces under some reasonable assumptions on the sequence of parameters. As applications, we present some strong convergence results for monotone inclusion, variational inequality problem, linear inverse problem, and LASSO problem in Compressed Sensing. Our result improves the rate of convergence of existing Halpern method for monotone inclusion, variational inequality problem, linear inverse problem and LASSO problem in compressed sensing as illustrated in our numerical examples both in finite and infinite dimensional Hilbert spaces.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

References

  1. Alvarez, F.: Weak convergence of a relaxed and inertial hybrid projection-proximal point algorithm for maximal monotone operators in Hilbert space. SIAM J. Optim. 14, 773–782 (2004)

    MathSciNet  MATH  Google Scholar 

  2. Alvarez, F., Attouch, H.: An inertial proximal method for maximal monotone operators via discretization of a nonlinear oscillator with damping. Set-Valued Anal. 9, 3–11 (2001)

    MathSciNet  MATH  Google Scholar 

  3. Ansari, Q.H., Köbis, E., Yao, J.-C.: Vector Variational Inequalities and Vector Optimization, Theory and Applications, Vector Optimization. Springer, Cham (2018). xiii+ 509 pp

    MATH  Google Scholar 

  4. Attouch, H., Peypouquet, J.: The rate of convergence of Nesterov’s accelerated forward-backward method is actually faster than \(\frac {1}{k^{2}}\). SIAM J. Optim. 26, 1824–1834 (2016)

    MathSciNet  MATH  Google Scholar 

  5. Aubin, J.-P., Ekeland, I.: Applied Nonlinear Analysis. Wiley, New York (1984)

    MATH  Google Scholar 

  6. Baiocchi, C., Capelo, A.: Variational and Quasivariational Inequalities; Applications to Free Boundary Problems. Wiley, New York (1984)

    MATH  Google Scholar 

  7. Bauschke, H.H., Combettes, P.L.: Convex Analysis and Monotone Operator Theory in Hilbert Spaces, CMS Books in Mathematics. Springer, New York (2011)

    MATH  Google Scholar 

  8. Beck, A., Teboulle, M.: A fast iterative shrinkage-thresholding algorithm for linear inverse problems. SIAM J. Imaging Sci. 2(1), 183–202 (2009)

    MathSciNet  MATH  Google Scholar 

  9. Ben-Tal, A., Nemirovski, A.: Lectures on Modern Convex Optimization: Analysis, Algorithms and Engineering Applications, MPS-SIAM Series on Optimization. SIAM, Philadelphia (2001)

    MATH  Google Scholar 

  10. van den Berg, E., Friedlander, M.P.: SPGL1: a Solver for Large-scale Sparse Reconstruction, http://www.cs.ubc.ca/labs/scl/spgl1 (2007)

  11. Boikanyo, O.A., Morosanu, G.: Four parameter proximal point algorithms. Nonlinear Anal. 74, 544–555 (2011)

    MathSciNet  MATH  Google Scholar 

  12. Bot, R.I., Csetnek, E.R., Meier, D.: Inducing strong convergence into the asymptotic behaviour of proximal splitting algorithms in Hilbert spaces. Optim. Methods Software, https://doi.org/10.1080/10556788.2018.1457151

  13. Bréziz, H.: Operateur maximaux monotones. In: Mathematics Studies, vol. 5, North-Holland (1973)

  14. Chambolle, A., Dossal, C.h.: On the convergence of the iterates of the “fast iterative shrinkage/thresholding algorithm”. J. Optim. Theory. Appl. 166, 968–982 (2015)

    MathSciNet  MATH  Google Scholar 

  15. Chen, P., Huang, J., Zhang, X.: A primal-dual fixed point algorithm for convex separable minimization with applications to image restoration. Inverse Probl. 29, 025011 (2013)

    MathSciNet  MATH  Google Scholar 

  16. Chen, S., Donoho, D.L., Saunders, M.: Atomic decomposition by basis pursuit. SIAM J. Sci. Comput. 20, 33–61 (1998)

    MathSciNet  MATH  Google Scholar 

  17. Chidume, C.E., Chidume, C.O.: Iterative approximation of fixed points of nonexpansive mappings. J. Math. Anal. Appl. 318, 288–295 (2006)

    MathSciNet  MATH  Google Scholar 

  18. Cholamjiak, W., Cholamjiak, P., Suantai, S.: An inertial forward-backward splitting method for solving inclusion problems in Hilbert spaces. J. Fixed Point Theory Appl. 20(2), Art. 68, 21 pp (2018)

    MathSciNet  MATH  Google Scholar 

  19. Combettes, P.L., Wajs, V.: Signal recovery by proximal forward-backward splitting. SIAM Multiscale Model Simul. 4, 1168–1200 (2005)

    MathSciNet  MATH  Google Scholar 

  20. Daubechies, I., Defrise, M., De Mol, C.: An iterative thresholding algorithm for linear inverse problems with a sparsity constraint. Commun. Pure Appl. Math. 57, 1413–1457 (2004)

    MathSciNet  MATH  Google Scholar 

  21. Dong, Q.L., Jiang, D., Cholamjiak, P., Shehu, Y.: A strong convergence result involving an inertial forward-backward algorithm for monotone inclusions. J. Fixed Point Theory Appl. 19, 3097–3118 (2017)

    MathSciNet  MATH  Google Scholar 

  22. Dong, Q.L., Yuan, H.B., Cho, Y.J., Rassias, Th.M.: Modified inertial Mann algorithm and inertial CQ-algorithm for nonexpansive mappings. Optim. Lett. 12(1), 87–102 (2018)

    MathSciNet  MATH  Google Scholar 

  23. Duchi, J., Singer, Y.: Efficient online and batch learning using forward backward splitting. J. Mach. Learn. Res. 10, 2899–2934 (2009)

    MathSciNet  MATH  Google Scholar 

  24. Facchinei, F., Pang, J. -S.: Finite-Dimensional Variational Inequalities and Complementarity Problems, vol. II. Springer Series in Operations Research, Springer, New York (2003)

    MATH  Google Scholar 

  25. Glowinski, R., Lions, J.-L., Trémolières, R.: Numerical Analysis of Variational Inequalities. North-Holland, Amsterdam (1981)

    MATH  Google Scholar 

  26. Goebel, K., Kirk, W.A.: On Metric Fixed Point Theory. Cambridge University Press, Cambridge (1990)

    MATH  Google Scholar 

  27. Halpern, B.: Fixed points of nonexpansive maps. Bull. Amer. Math. Soc. 73, 957–961 (1967)

    MathSciNet  MATH  Google Scholar 

  28. Iiduka, H.: Iterative algorithm for triple-hierarchical constrained nonconvex optimization problem and its application to network bandwidth allocation. SIAM J. Optim. 22, 862–878 (2012)

    MathSciNet  MATH  Google Scholar 

  29. Iiduka, H.: Fixed point optimization algorithms for distributed optimization in networked systems. SIAM J. Optim. 23, 1–26 (2013)

    MathSciNet  MATH  Google Scholar 

  30. Kanzow, C., Shehu, Y.: Generalized Krasnoselskii–Mann-type iterations for nonexpansive mappings in Hilbert spaces. Comput. Optim. Appl. 67, 595–620 (2017)

    MathSciNet  MATH  Google Scholar 

  31. Kanzow, C., Shehu, Y.: Strong convergence of a double projection-type method for monotone variational inequalities in Hilbert spaces. J. Fixed Point Theory Appl. 20(1), Art. 51, 24 pp (2018)

    MathSciNet  MATH  Google Scholar 

  32. Khatibzadeh, H., Ranjbar, S.: Halpern type iterations for strongly quasi-nonexpansive sequences and its applications. Taiwanese J. math. 19, 1561–1576 (2015)

    MathSciNet  MATH  Google Scholar 

  33. Kinderlehrer, D., Stampacchia, G.: An Introduction to Variational Inequalities and Their Applications. Academic Press, New York (1980)

    MATH  Google Scholar 

  34. Konnov, I.V.: Combined Relaxation Methods for Variational Inequalities. Springer-Verlag, Berlin (2001)

    MATH  Google Scholar 

  35. Korpelevich, G.M.: An extragradient method for finding saddle points and for other problems. Ékonom. i Mat. Metody 12, 747–756 (1976)

    MathSciNet  MATH  Google Scholar 

  36. Kraikaew, R., Saejung, S.: Strong convergence of the Halpern subgradient extragradient method for solving variational inequalities in Hilbert spaces. J. Optim. Theory Appl. 163, 399–412 (2014)

    MathSciNet  MATH  Google Scholar 

  37. Kraikaew, R., Saejung, S.: On split common fixed point problems. J. Math. Anal. Appl. 415, 513–524 (2014)

    MathSciNet  MATH  Google Scholar 

  38. Lemaire, B.: Which fixed point does the iteration method select?. In: Recent Advances in Optimization, vol. 452, pp 154–157. Springer, Berlin (1997)

  39. Lions, P.-L.: Approximation de points fixes de contractions. C. R. Acad. Sci. Paris Sér. A-B 284, A1357–A1359 (1977)

    MathSciNet  Google Scholar 

  40. Lorenz, D.A., Pock, T.: An inertial forward-backward algorithm for monotone inclusions. J. Math. Imaging Vis. 51, 311–325 (2015)

    MathSciNet  MATH  Google Scholar 

  41. Lorenz, D.A.: Constructing test instances for basis pursuit denoising. IEEE Trans. Signal Process. 61, 1210–1214 (2013)

    MathSciNet  MATH  Google Scholar 

  42. Maingé, P.-E.: Approximation methods for common fixed points of nonexpansive mappings in Hilbert spaces. J. Math. Anal. Appl. 325, 469–479 (2007)

    MathSciNet  MATH  Google Scholar 

  43. Maingé, P.-E.: Strong convergence of projected subgradient methods for nonsmooth and nonstrictly convex minimization. Set-Valued Anal. 16, 899–912 (2008)

    MathSciNet  MATH  Google Scholar 

  44. Maingé, P. -E., Gobinddass, M.L.: Convergence of one-step projected gradient methods for variational inequalities. J. Optim. Theory Appl. 171, 146–168 (2016)

    MathSciNet  MATH  Google Scholar 

  45. Malitsky, Y.u.V.: Projected reflected gradient methods for monotone variational inequalities. SIAM J. Optim. 25, 502–520 (2015)

    MathSciNet  MATH  Google Scholar 

  46. Marino, G., Xu, H.K.: Convergence of generalized proximal point algorithm. Comm. Pure Appl. Anal. 3, 791–808 (2004)

    MathSciNet  MATH  Google Scholar 

  47. Micchelli, C.A., Shen, L., Xu, Y.: Proximity algorithms for image models: denoising. Inverse Probl. 27, 045009 (2011)

    MathSciNet  MATH  Google Scholar 

  48. Moudafi, A., Oliny, M.: Convergence of a splitting inertial proximal method for monotone operators. J. Comput. Appl. Math. 155, 447–454 (2003)

    MathSciNet  MATH  Google Scholar 

  49. Nagurney, A.: Network Economics: a Variational Inequality Approach. Kluwer Academic Publishers, Dordrecht (1999)

    MATH  Google Scholar 

  50. Nakajo, K., Takahashi, W.: Strong convergence theorems for nonexpansive mappings and nonexpansive semigroups. J. Math. Anal. Appl. 279, 372–379 (2003)

    MathSciNet  MATH  Google Scholar 

  51. Nesterov, Y.: A method for solving the convex programming problem with convergence rate \(O(\frac {1}{k^{2}})\). Dokl. Akad. Nauk SSSR 269, 543–547 (1983)

    MathSciNet  Google Scholar 

  52. Parikh, N., Boyd, S.: Proximal algorithms. Found. Trends Optim. 1, 123–231 (2013)

    Google Scholar 

  53. Polyak, B.T.: Some methods of speeding up the convergence of iteration methods. Zh. Vychisl. Mat. Mat. Fiz. 4, 1–17 (1964)

    Google Scholar 

  54. Qin, X., Petrusel, A., Yao, J.-C.: CQ iterative algorithms for fixed points of nonexpansive mappings and split feasibility problems in Hilbert spaces. J. Nonlinear Convex Anal. 19, 157–165 (2018)

    MathSciNet  MATH  Google Scholar 

  55. Raguet, H., Fadili, J., Peyré, G.: A generalized forward-backward splitting. SIAM J. Imaging Sci. 6, 1199–1226 (2013)

    MathSciNet  MATH  Google Scholar 

  56. Reich, S.: Strong convergence theorems for resolvents of accretive operators in Banach spaces. J. Math. Anal. Appl. 75, 287–292 (1980)

    MathSciNet  MATH  Google Scholar 

  57. Reich, S.: Some problems and results in fixed point theory. Contemp. Math. 21, 179–187 (1983)

    MathSciNet  MATH  Google Scholar 

  58. Saejung, S.: Halpern’s iteration in Banach spaces. Nonlinear Anal. 73, 3431–3439 (2010)

    MathSciNet  MATH  Google Scholar 

  59. Shehu, Y.: Iterative approximations for zeros of sum of accretive operators in Bannach spaces. J. Funct. Spaces 5973468, 9 (2016)

    MATH  Google Scholar 

  60. Shehu, Y., Cai, G.: Strong convergence result of forward-backward splitting methods for accretive operators in Banach spaces with application. RACSAM 11, 71–87 (2018)

    MathSciNet  MATH  Google Scholar 

  61. Shioji, N., Takahashi, W.: Strong convergence of approximated sequences for nonexpansive mapping in Banach spaces. Proc. Amer. Math. Soc. 125, 3641–3645 (1997)

    MathSciNet  MATH  Google Scholar 

  62. Suzuki, T.: Strong convergence of Krasnoselskii and Mann’s type sequences for one-parameter nonexpansive semigroups without Bochner integrals. J. Math. Anal. Appl. 305, 227–239 (2005)

    MathSciNet  MATH  Google Scholar 

  63. Tibshirami, R.: Strong convergence of approximated sequences for nonexpansive mapping in Banach spaces. Roy. Statist. Soc. Ser. B 58, 267–288 (1996)

    MathSciNet  Google Scholar 

  64. Wang, F., Cui, H.: On the contraction-proximal point algorithms with multi-parameters. J. Global Optim. 54, 485–491 (2012)

    MathSciNet  MATH  Google Scholar 

  65. Wang, Y., Wang, F., Xu, H.K.: Error sensitivity for strongly convergent modifications of the proximal point algorithm. J. Optim. Theory Appl. 168, 901–916 (2016)

    MathSciNet  MATH  Google Scholar 

  66. Wang, Y., Xu, H.-K.: Strong convergence for the proximal-gradient method. J. Nonlinear Convex Anal. 15, 581–593 (2014)

    MathSciNet  MATH  Google Scholar 

  67. Wittmann, R.: Approximation of fixed points of nonexpansive mappings. Arch. Math. 58, 486–491 (1992)

    MathSciNet  MATH  Google Scholar 

  68. Xu, H.K.: Iterative algorithm for nonlinear operators. J. London Math. Soc. 66, 240–256 (2002)

    MathSciNet  MATH  Google Scholar 

  69. Xu, H.K.: Another control condition in an iterative method for nonexpansive mappings. Bull. Astral. Math. Soc. 65, 109–113 (2002)

    MathSciNet  MATH  Google Scholar 

Download references

Acknowledgments

The research of Y. Shehu is supported by the Alexander von Humboldt-Foundation and Postdoctoral Fellowship from the Institute of Science and Technology (IST), Klosterneuburg, Vienna, Austria.

The research of F. U. Ogbuisi is wholly supported by the National Research Foundation (NRF) of South Africa (S& F-DST/NRF Innovation Postdoctoral Fellowship; Grant Number: 111992). Opinions expressed and conclusions arrived are those of the authors and are not necessarily to be attributed to the NRF.

Author information

Authors and Affiliations

  1. Department of Mathematics, Zhejiang Normal University, Jinhua, 321004, People’s Republic of China

    Yekini Shehu

  2. Department of Mathematics, Computer Science and Information System, California University of Pennsylvania, California, PA, USA

    Olaniyi S. Iyiola

  3. Department of Mathematics, University of Nigeria, Nsukka, Nigeria

    Ferdinard U. Ogbuisi

  4. School of Mathematics, Statistics and Computer Science, University of KwaZulu-Natal, Durban, South Africa

    Ferdinard U. Ogbuisi

Authors
  1. Yekini Shehu
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Olaniyi S. Iyiola
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Ferdinard U. Ogbuisi
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Yekini Shehu.

Additional information

Publisher’s note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Shehu, Y., Iyiola, O.S. & Ogbuisi, F.U. Iterative method with inertial terms for nonexpansive mappings: applications to compressed sensing. Numer Algor 83, 1321–1347 (2020). https://doi.org/10.1007/s11075-019-00727-5

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11075-019-00727-5

Keywords

Mathematics Subject Classification (2010)

Search

Navigation