Skip to main content

Advertisement

Springer Nature Link
Log in

Using Gauss-Jacobi quadrature rule to improve the accuracy of FEM for spatial fractional problems

  • Original Paper
  • Published:
Numerical Algorithms Aims and scope Submit manuscript

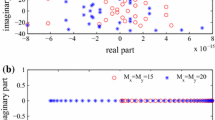
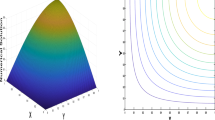
Abstract

Though the finite element method has been widely used in solving fractional differential equations, the effects of the Gaussian quadrature rule on the numerical results have rarely been considered. Since the fractional derivatives of the basis functions are not polynomials with integer power and always have weak singularities on some elements, the Gaussian quadrature rule (Gauss-Legendre quadrature rule) may not be suitable in assembling the fractional stiffness matrix. By splitting the integrand of the inner products into a weak singularity part and a smooth part and utilizing the Gauss-Jacobi quadrature rule for the weak singularity part, we present a modified algorithm to assemble the fractional stiffness matrix. The numerical results, conducted on 1D and 2D domains, show that our method can significantly improve the accuracy of the stiffness matrix as well as the accuracy of the numerical solution with much fewer Gaussian points.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12

Similar content being viewed by others

References

  1. Kulish, V.V., Lage, J.L.: Application of fractional calculus to fluid mechanics. ASME J. Fluids Eng. 124(3), 803–806 (2002). https://doi.org/10.1115/1.1478062

    Article  Google Scholar 

  2. Laskin, N.: Fractional quantum mechanics. Phys. Rev. E 62, 3135–3145 (2000). https://doi.org/10.1103/PhysRevE.62.3135

    Article  Google Scholar 

  3. Bagley, R.L., Torvik, P.J.: A theoretical basis for the application of fractional calculus to viscoelasticity. J. Rheol. 27(3), 201–210 (1983)

    Article  Google Scholar 

  4. Meral, F.C., Royston, T.J., Magin, R.: Fractional calculus in viscoelasticity: an experimental study. Commun. Nonlinear Sci. Numer. Simul. 15(4), 939–945 (2010). https://doi.org/10.1016/j.cnsns.2009.05.004

    Article  MathSciNet  Google Scholar 

  5. Magin, R.L., Abdullah, O., Baleanu, D., Zhou, X.J.: Anomalous diffusion expressed through fractional order differential operators in the Bloch-Torrey equation. J. Magn. Reson. 190(2), 255–270 (2008). https://doi.org/10.1016/j.jmr.2007.11.007

    Article  Google Scholar 

  6. Yu, Q., Reutens, D., Vegh, V.: Can anomalous diffusion models in magnetic resonance imaging be used to characterise white matter tissue microstructure?. Neuroimage 175, 122–137 (2018). https://doi.org/10.1016/j.neuroimage.2018.03.052

    Article  Google Scholar 

  7. Samko, S.G., Kilbas, A.A., Marichev, O.I., et al.: Fractional Integrals and Derivatives. Gordon and Breach Science Publishers, Yverdon Yverdon-les-Bains, Switzerland (1993)

    MATH  Google Scholar 

  8. Benson, D.A., Wheatcraft, S.W., Meerschaert, M.M.: Application of a fractional advection-dispersion equation. Water Resour. Res. 36(6), 1403–1412 (2000)

    Article  Google Scholar 

  9. Kilbas, A., Srivastava, H.M., Trujillo, J.: Theory and applications of fractional differential equations. Elsevier Science (2006)

  10. Bishehniasar, M., Salahshour, S., Ahmadian, A., Ismail, F., Baleanu, D.: An accurate approximate-analytical technique for solving time-fractional partial differential equations. Complexity, 8718209. https://doi.org/10.1155/2017/8718209 (2017)

  11. Yusuf, A., Qureshi, S., Inc, M., Aliyu, A.I., Baleanu, D., Shaikh, A.A.: Two-strain epidemic model involving fractional derivative with Mittag-Leffler kernel. Chaos: Interdiscip. J. Nonlinear Sci. 28(12), 123121. https://doi.org/10.1063/1.5074084(2018)

  12. Sene, N.: SIR epidemic model with Mittag–Leffler fractional derivative. Chaos Solitons Fractals 137, 109833. https://doi.org/10.1016/j.chaos.2020.109833 (2020)

  13. Meerschaert, M.M., Tadjeran, C.: Finite difference approximations for fractional advection–dispersion flow equations. J. Comput. Appl. Math. 172(1), 65–77 (2004). https://doi.org/10.1016/j.cam.2004.01.033

    Article  MathSciNet  Google Scholar 

  14. Zhuang, P., Liu, F., Anh, V., Turner, I.: New solution and analytical techniques of the implicit numerical method for the anomalous subdiffusion equation. SIAM J. Numer Anal. 46(2), 1079–1095 (2008). https://doi.org/10.1137/060673114

    Article  MathSciNet  Google Scholar 

  15. Wang, H., Basu, T.S.: A fast finite difference method for two-dimensional space-fractional diffusion equations. SIAM J. Sci. Comput. 34(5), A2444–A2458 (2012). https://doi.org/10.1137/12086491X

    Article  MathSciNet  Google Scholar 

  16. Ervin, V.J., Roop, J.P.: Variational formulation for the stationary fractional advection dispersion equation. Numer. Methods Partial Differ. Equ. 22 (3), 558–576 (2006). https://doi.org/10.1002/num.20112

    Article  MathSciNet  Google Scholar 

  17. Deng, W.: Finite element method for the space and time fractional Fokker-Planck equation. SIAM. J. Numer. Anal. 47(1), 204–226 (2008). https://doi.org/10.1137/080714130

    Article  MathSciNet  Google Scholar 

  18. Li, X., Xu, C.: A space-time spectral method for the time fractional diffusion equation. SIAM J. Numer. Anal. 47(3), 2108–2131 (2009). https://doi.org/10.1137/080718942

    Article  MathSciNet  Google Scholar 

  19. Li, C., Zeng, F., Liu, F.: Spectral approximations to the fractional integral and derivative. Fract. Calc. Appl. Anal. 15(3), 383–406 (2012). https://doi.org/10.2478/s13540-012-0028-x

    Article  MathSciNet  Google Scholar 

  20. Ervin, V.J., Roop, J.P.: Variational solution of fractional advection dispersion equations on bounded domains in \(\mathbb {R}^{d}\). Numer. Methods Partial Differ. Equ. 23 (2), 256–281 (2007). https://doi.org/10.1002/num.20169

    Article  MathSciNet  Google Scholar 

  21. Jin, B., Lazarov, R., Pasciak, J., Zhou, Z.: Error analysis of a finite element method for the space-fractional parabolic equation. SIAM J. Numer. Anal. 52(5), 2272–2294 (2014). https://doi.org/10.1137/13093933x

    Article  MathSciNet  Google Scholar 

  22. Bu, W., Tang, Y., Wu, Y., Yang, J.: CRank-Nicolson ADI Galerkin finite element method for two-dimensional fractional FitzHugh-Nagumo monodomain model. Appl. Math. Comput. 257, 355–364 (2015). https://doi.org/10.1016/j.amc.2014.09.034

    MathSciNet  MATH  Google Scholar 

  23. Feng, L.B., Zhuang, P., Liu, F., Turner, I., Gu, Y.T.: Finite element method for space-time fractional diffusion equation. Numer. Algorithm. 72(3), 749–767 (2016). https://doi.org/10.1007/s11075-015-0065-8

    Article  MathSciNet  Google Scholar 

  24. Yang, Z., Yuan, Z., Nie, Y., Wang, J., Zhu, X., Liu, F.: Finite element method for nonlinear Riesz space fractional diffusion equations on irregular domains. J. Comput. Phys. 330, 863–883 (2017). https://doi.org/10.1016/j.jcp.2016.10.053

    Article  MathSciNet  Google Scholar 

  25. Fan, W., Liu, F., Jiang, X., Turner, I.: A novel unstructured mesh finite element method for solving the time-space fractional wave equation on a two-dimensional irregular convex domain. Fract. Calc. Appl. Anal. 20(2). https://doi.org/10.1515/fca-2017-0019 (2017)

  26. Yang, Z., Nie, Y., Yuan, Z., Wang, J.: Finite element methods for fractional PDEs in three dimensions. Appl. Math. Lett. 100, 106041. https://doi.org/10.1016/j.aml.2019.106041 (2020)

  27. Acosta, G., Bersetche, F.M., Borthagaray, J.P., short, A: FE Implementation for a 2d homogeneous dirichlet problem of a fractional laplacian. Comput. Math. Appl. 74(4), 784–816 (2017). https://doi.org/10.1016/j.camwa.2017.05.026

    Article  MathSciNet  Google Scholar 

  28. Acosta, G., Borthagaray, J.P.: A fractional laplace equation: Regularity of solutions and finite element approximations. SIAM. J. Numer. Anal. 55 (2), 472–495 (2017). https://doi.org/10.1137/15m1033952

    Article  MathSciNet  Google Scholar 

  29. Lai, J., Liu, F., Anh, V.V., Liu, Q.: A space-time finite element method for solving linear Riesz space fractional partial differential equations. Numer. Algorithm. https://doi.org/10.1007/s11075-020-01047-9 (2021)

  30. Shen, J., Tang, T., Wang, L. -L.: Spectral Mehtods: algorithms, Analysis and Applications, 1st Edition, no. 41 in Springer Series in Computational Mathematics Springer, Berlin. https://doi.org/10.1007/978-3-540-71041-7 (2011)

Download references

Funding

This research was supported by the National Natural Science Foundation of China (11971386), the National Key R&D Program of China (2020YFA0713603), the Natural Science Foundation of Shaanxi Province (2020JM-132), and the Fundamental Research Funds for the Central Universities (310201911cx025).

Author information

Authors and Affiliations

  1. Research Center for Computational Science, Northwestern Polytechnical University, Xi’an, Shaanxi, 710129, China

    Zongze Yang, Jungang Wang, Zhanbin Yuan & Yufeng Nie

Authors
  1. Zongze Yang
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Jungang Wang
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Zhanbin Yuan
    View author publications

    You can also search for this author inPubMed Google Scholar

  4. Yufeng Nie
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Yufeng Nie.

Additional information

Publisher’s note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Yang, Z., Wang, J., Yuan, Z. et al. Using Gauss-Jacobi quadrature rule to improve the accuracy of FEM for spatial fractional problems. Numer Algor 89, 1389–1411 (2022). https://doi.org/10.1007/s11075-021-01158-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11075-021-01158-x

Keywords

Mathematics Subject Classification (2010)