Skip to main content
SpringerLink
Log in

Nitsche’s method for elliptic Dirichlet boundary control problems on curved domains

  • Original Paper
  • Published:
Numerical Algorithms Aims and scope Submit manuscript

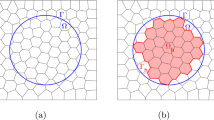
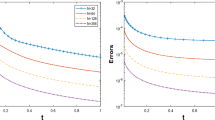
Abstract

We consider Nitsche’s method for solving elliptic Dirichlet boundary control problems on curved domains with control constraints. By using Nitsche’s method for the treatment of inhomogeneous Dirichlet boundary conditions, the L2 boundary control enters in the variational formulation in a natural sense. The idea was first used in Chang, et al. (Math. Anal. Appl. 453, 529–557 2017) where the curved boundary was approximated by a broken line and a locally defined mapping was needed to obtain the numerical control on the curved boundary. In this paper, we develop a method defined on curved domains directly. We derive a priori estimates of quasi-optimal order for the control in the L2 norm, and quasi-optimal order for the state and adjoint state in energy norms. Numerical examples are provided to show the performance of the proposed method.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

Data availability

Data will be made available on request.

References

  1. Apel, T., Mateos, M., Pfefferer, J., Rösch, A.: On the regularity of the solutions of Dirichlet optimal control problems in polygonal domains. SIAM. J. Control Optim. 53, 3620–3641 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  2. Apel, T., Mateos, M., Pfefferer, J., Rösch, A.: Error estimates for Dirichlet control problems in polygonal domains: quasi-uniform meshes. Math. Control Relat. Fields 8, 217–245 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  3. Apel, T., Rösch, A., Winkler, G.: Discretization error estimates for an optimal control problem in a nonconvex domain. In: Numerical mathematics and advanced applications, pages 299–307. Springer (2006)

  4. Becker, R.: Mesh adaptation for Dirichlet flow control via Nitsche’s method. Comm. Numer. Methods Engrg. 18, 669–680 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  5. Bernardi, C.: Optimal finite-element interpolation on curved domains. SIAM J.Numer Anal. 26, 1212–1240 (1989)

    Article  MathSciNet  MATH  Google Scholar 

  6. Bramble, J. H., King, J. T.: A robust finite element method for nonhomogeneous Dirichlet problems in domains with curved boundaries. Math. Comput. 63, 1–17 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  7. Brenner, S. C., Scott, L. R.: The Mathematical Theory of Finite Element Methods, Third edition. Springer, New York (2008)

    Book  MATH  Google Scholar 

  8. Cai, Z., He, C., Zhang, S.: Discontinuous finite element methods for interface problems Robust a priori and a posteriori error estimates. SIAM J. Numer. Anal. 55, 400–418 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  9. Cai, Z., Ye, X., Zhang, S.: Discontinuous GAlerkin finite element methods for interface problems: a priori and a posteriori error estimations. SIAM J. Numer. Anal. 49, 1761–1787 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  10. Casas, E., Raymond, J.: Error estimates for the numerical approximation of D,irichlet boundary control for semilinear elliptic equations. SIAM J. Control Optim. 45(5), 1586–1611 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  11. Chang, L., Gong, W., Yan, N.: Weak boundary penalization for Dirichlet boundary control problems governed by elliptic equations. J. Math. Anal Appl. 453, 529–557 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  12. Chowdhury, S., Gudi, T., Nandakumaran, A. K.: Error bounds for a Dirichlet boundary control problem based on energy spaces. Math. Comp. 86, 1103–1126 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  13. Deckelnick, K., Günther, A., Hinze, M.: Finite element approximation of Dirichlet boundary control for elliptic PDEs on two- and three-dimensional curved domains. SIAM J. Control Optim. 48(4), 2798–2819 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  14. Geveci, T.: On the approximation of the solution of an optimal control problem governed by an elliptic equation. RAIRO Anal. Numer. 13, 313–328 (1979)

    Article  MathSciNet  MATH  Google Scholar 

  15. Gong, W., Hu, W., Mateos, M., Singler, J. R., Zhang, X., Zhang, Y.: A new HDG method for Dirichlet boundary control of convection diffusion PDEs II Low regularity. SIAM J. Numer. Anal. 56, 2262–2287 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  16. Gong, W., Yan, N.: Mixed finite element method for Dirichlet boundary control problem governed by elliptic PDEs. SIAM J. Control Optim 49 (3), 984–1014 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  17. Grisvard, P.: Elliptic Problems in Nonsmooth Domains. Monogr. Stud. Math. 24, Pitman, Boston (1985)

  18. Gunzburger, M. D., Hou, L. S.: Finite-dimensional approximation of a class of constrained nonlinear optimal control problems. SIAM J. Control Optim. 34, 1001–10043 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  19. Guzmán, J., Sánchez, M. A., Sarkis, M.: Higher-order finite element methods for elliptic problems with interfaces. ESIAM Math. Model. Numer Anal. 50, 1561–1583 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  20. Hinze, M.: A variational discretization concept in control constrained optimization: the linear-quadratic case. Comput. Optim. Appl. 30, 45–61 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  21. Hinze, M., Pinnau, R., Ulbrich, M., Ulbrich, S.: Optimization with PDE Constraints, Volume 23 of Mathematial Modelling : Theory and Applications. Springer, New York (2009)

    MATH  Google Scholar 

  22. Hu, W., Shen, J., Singler, J. R., Zhang, Y., Zheng, X.: A superconvergent hybridizable discontinuous Galerkin method for Dirichlet boundary control of elliptic PDEs. Numer. Math. 144, 375–411 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  23. Ito, K., Kunisch, K., Peichl, G. H.: Variational approach to shape derivatives for a class of Bernoulli problems. J. Math. Anal Appl. 314, 126–149 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  24. John, L., Swierczynski, P., Wohlmuth, B.: Energy corrected FEM for optimal Dirichlet boundary control problems. Numer. Math. 139, 913–938 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  25. Kunisch, K., Vexler, B.: Optimal vortex reduction for instationary flows based on translation invariant cost functionals. SIAM J. Control Optim. 46 (4), 1368–1397 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  26. May, S., Rannacher, R., Vexler, B.: Error analysis for a finite element approximation of elliptic dirichlet boundary control problems. SIAM J. Control Optim. 51, 2585–2611 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  27. Meyer, C., Rösch, A.: Superconvergence properties of optimal control problems. SIAM J. Control Optim. 43, 970–985 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  28. Neilan, M.: Localized pointwise error estimates and global Lp error estimates for Nitsche’s method. Int. J. Numer. Anal. Model. Ser. B 2, 338–354 (2011)

    MathSciNet  MATH  Google Scholar 

  29. Nitsche, J. A.: Über ein Variationsprinzip zur Lösung Dirichlet-Problemen bei Verwendung von Teilräumen, die keinen. Abh. Math. Sem. Univ. Hamburg 36, 9–15 (1971)

    Article  MathSciNet  MATH  Google Scholar 

  30. Vexler, B.: Finite element approximation of elliptic Dirichlet optimal control problems. Numer. Funct. Anal. Optim. 28, 957–973 (2007)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

The authors would like to thank the anonymous referees for beneficial comments and suggestions.

Funding

This work was partially supported by the Natural Science Foundation of Jiangsu Province, China (Grant No. BK20200848), the National Natural Science Foundation of China (Grant Nos.12101327 and 11971241), and the Supporting Project of National Natural Science Youth Fund of Nanjing University of Chinese Medicine (Grant No. XPT12101327).

Author information

Authors and Affiliations

  1. School of Artificial Intelligence and Information Technology, Nanjing University of Chinese Medicine, Nanjing, 210023, China

    Qian Zhang

  2. Jiangsu Key Laboratory for NSLSCS, School of Mathematical Sciences, Nanjing Normal University, Nanjing, 210023, China

    Zhiyue Zhang

Authors
  1. Qian Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Zhiyue Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Qian Zhang.

Ethics declarations

Competing interests

The authors declare no competing interests.

Additional information

Publisher’s note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhang, Q., Zhang, Z. Nitsche’s method for elliptic Dirichlet boundary control problems on curved domains. Numer Algor 94, 511–545 (2023). https://doi.org/10.1007/s11075-023-01510-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11075-023-01510-3

Keywords

Mathematics Subject Classification 2010

Search

Navigation