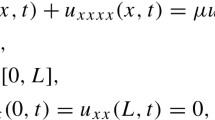Abstract
The aim of this study is to develop compact difference method to approximate parabolic PDEs of fourth order equipped with Dirichlet and Neumann boundary conditions involving half-step points. The proposed method converges quaternary and quadratically in space and time, respectively. The imbedding technique has been applied to approximate derivative terms of lower order by means of the governing differential equation to deduce the high-order method. The primary utility of this new discretization is that it can be straightaway applied to problems with singularities without necessitating fictitious nodes or special approach which has consequently lowered computational complicacy. We have examined linear stability of the proposed three-level implicit difference scheme using matrix stability analysis. In addition, we also obtained the solution of the first-order spatial derivative which is of significance in several physical problems. The efficacy of the proposed approximation is confirmed through numerical tests performed on a collection of physically relevant problems comprising the Euler Bernoulli beam equation and the highly nonlinear good Boussinesq equation. Numerical experiments evidently exhibit that the method provides more accurate results in contrast with the existing numerical techniques. The present method is able to simulate well the complex and intriguing long time dynamics of the good Boussinesq equation.





Similar content being viewed by others
Data availibility
Because no datasets were collected or analyzed during the present investigation, data sharing is not applicable to this publication.
References
Mohebbi, A., Asgari, Z.: Efficient numerical algorithms for the solution of “good” Boussinesq equation in water wave propagation. Comput. Phys. Comm. 182, 2464–2470 (2011)
Almatrafi, M.B., Alharbi, A.R.: Tunç, Cemil: Constructions of the soliton solutions to the good Boussinesq equation. Adv. Difference Equ. Paper No. 629 (2020)
Boussinesq, M.J.: Theory of waves and vortices propagating along a horizontal rectangular channel, communicating to the liquid in the channel generally similar velocities of the bottom surface. J. Math. Pures Appl. 17, 55–108 (1872)
Bratsos, A.G.: The solution of the Boussinesq equation using the method of lines. Comput. Methods Appl. Mech. Eng. 157, 33–44 (1998)
Bratsos, A.G.: A second order numerical scheme for the solution of the one-dimensional Boussinesq equation. Numer. Algor. 46, 45–58 (1872)
Brugnano, L., Gurioli, G., Zhang, C.: Spectrally accurate energy-preserving methods for the numerical solution of the “good’’ Boussinesq equation. Numer. Methods Partial. Differ. Equ. 35, 1343–1362 (2019)
Cai, J., Wang, Y.: Local structure-preserving algorithms for the “good’’ Boussinesq equation. J. Comput. Phys. 239, 72–89 (2013)
Chawla, M.M., Shivakumar, P.N.: An efficient finite difference method for two-point boundary value problems. Neural Parallel Sci. Comput. 4, 387–395 (1996)
Chen, M., Kong, L., Hong, Y.: Efficient structure-preserving schemes for good Boussinesq equation. Math. Methods Appl. Sci. 41, 1743–1752 (2018)
Dehghan, M., Salehi, R.: A meshless based numerical technique for traveling solitary wave solution of Boussinesq equation. Appl. Math. Model. 36, 1939–1956 (2012)
El-Gamel, M.: A note on solving the fourth-order parabolic equation by the sinc-Galerkin method. Calcolo 52, 327–342 (2015)
Gorman, D.G.: Free vibrations analysis of beams and shafts. Wiley, New York (1975)
Hageman, L.A., Young, D.M.: Applied iterative methods. Dover Publication, New York (2004)
Ismail, M.S., Mosally, F.: A fourth order finite difference method for the good Boussinesq equation. Abstr. Appl. Anal. Art. ID 323260, 10 (2014)
Jiang, C., Sun, J., He, X., Zhou, L.: High order energy-preserving method of the “good’’ Boussinesq equation. Numer. Math. Theory Methods Appl. 9, 111–122 (2016)
Kaur, D., Mohanty, R.K.: Highly accurate compact difference scheme for fourth order parabolic equation with Dirichlet and Neumann boundary conditions: Application to good Boussinesq equation. Appl. Math. Comput. 378(125202) (2020)
Kelly, C.Y.: Iterative methods for linear and non-linear equations. SIAM Publications, Philadelphia (1995)
Manoranjan, V.S., Mitchell, A.R., Morris, JLl.: Numerical solutions of the good Boussinesq equation. SIAM J. Sci. Statist. Comput. 5, 946–957 (1984)
Maruno, K., Biondini, G.: Resonance and web structure in discrete soliton systems: the two-dimensional Toda lattice and its fully discrete and ultradiscrete analogues. J. Phys. A: Math. Gen. 37, 11819–11839 (2004)
Meirovitch, L.: Principles and techniques of vibrations. Prentice Hall, New Jersey (1997)
Mohammadi, R.: Sextic B-spline collocation method for solving Euler-Bernoulli beam models. Appl. Math. Comput. 241, 151–166 (2014)
Mohanty, R.K., Kaur, D.: Unconditionally stable high accuracy compact difference schemes for multi-space dimensional vibration problems with simply supported boundary conditions Appl. Math. Model. 55, 281–298 (2018)
Ostermann, A., Su, C.: Two exponential-type integrators for the “good’’ Boussinesq equation. Numer. Math. 143, 683–712 (2019)
Saad, Y.: Iterative methods for sparse linear systems. SIAM Publisher (2003)
Smith, R.C., Bowers, K.L., Lund, J.: A fully sinc-Galerkin method for Euler-Bernoulli beam models. Numer. Methods Part. Differ. Equ. 8, 171–202 (1992)
Soh, C.W.: Euler-Bernoulli beams from a symmetry standpoint-characterization of equivalent equations. J. Math. Anal. Appl. 45, 387–395 (2008)
Su, C., Yao, W.: A Deuflhard-type exponential integrator Fourier pseudo-spectral method for the “good” Boussinesq equation. J. Sci. Comput. Paper No. 4, 83, 19 (2020)
Ucar, Y., Esen, A., Karaagac, B.: Numerical solutions of Boussinesq equation using Galerkin finite element method. Numer. Methods Part. Differ. Equ. 37, 1612–1630 (2021)
Uddin, M., Haq, S., Ishaq, M.: RBF-pseudospectral method for the numerical solution of good Boussinesq equation. Appl. Math. Sci. (Ruse) 6, 2403–2410 (2012)
Yan, J., Zhang, Z.: New energy-preserving schemes using Hamiltonian boundary value and Fourier pseudospectral methods for the numerical solution of the “good’’ Boussinesq equation Comput. Phys. Commun. 201, 33–42 (2016)
Zhang, C., Huang, J., Wang, C., Yue, X.: On the operator splitting and integral equation preconditioned deferred correction methods for the “good’’ Boussinesq equation. J. Sci. Comput. 75, 687–712 (2018)
Acknowledgements
We are thankful to Science and Engineering Research Board (SERB) (Sanction Order No.: CRG/2018/004608) for providing support during this research work.
Author information
Authors and Affiliations
Contributions
In this work, all authors contributed equally.
Corresponding author
Ethics declarations
Ethical approval
There are no studies involving human participants or animals done by any of the authors in this article.
Conflict of interest
The authors declare no competing interests.
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Kaur, D., Mohanty, R.K. High-order half-step compact numerical approximation for fourth-order parabolic PDEs. Numer Algor 95, 1127–1153 (2024). https://doi.org/10.1007/s11075-023-01602-0
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11075-023-01602-0
Keywords
- Fourth-order parabolic equations
- Compact difference method
- Euler-Bernoulli beam equation
- Good Boussinesq equation
- Solitary wave
- Block tridiagonal