Skip to main content
Springer Nature Link
Log in

Improved parallel finite element methods for the stationary Navier–Stokes problem

  • Original Paper
  • Published:
Numerical Algorithms Aims and scope Submit manuscript

Abstract

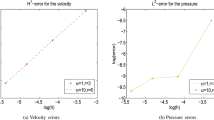
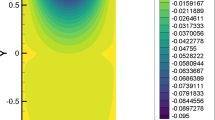
In this study, two improved parallel finite element algorithms based on two-grid strategies are developed to approximate the stationary Navier–Stokes equations. Algorithms are devised to improve the existing local and parallel finite element methods to arrive at \(L^2\) optimal velocity approximation by considering one further coarse grid correction. Rigorously numerical analysis is established, and numerical experiments are reported to support the theoretical findings.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3

Similar content being viewed by others

Data availability

Data sharing is not applicable to this article as no datasets were generated or analyzed during the current study.

References

  1. Adams, R.: Sobolev spaces. Academaic Press Inc, New York (1975)

    Google Scholar 

  2. Du, G., Zuo, L.: Local and parallel partition of unity scheme for the mixed Navier-Stokes-Darcy problem. Numer. Algor. 91(2), 635–650 (2020)

    Article  MathSciNet  Google Scholar 

  3. Du, G., Zuo, l.: A parallel partition of unity scheme based on two-grid discretizations for the Navier-Stokes problem. J. Sci. Comput. 75(3), 1445–1462 (2018)

  4. Du, G., Zuo, L.: Local and parallel finite element post-processing scheme for the Stokes problem. Comput. Math. Appl. 73(1), 129–140 (2017)

    Article  MathSciNet  Google Scholar 

  5. Du, G., Zuo, L.: Local and parallel finite element method for the mixed Navier-Stokes/Darcy model with Beavers-Joseph interface conditions. Acta. Math. Sci. 37(5), 1331–1347 (2017)

    Article  MathSciNet  Google Scholar 

  6. Girault, V., Raviart, P.: Finite element methods for Navier-Stokes equations: theory and algorithms. Springer-Verlag, Berlin Heidelberg (1986)

    Book  Google Scholar 

  7. He, Y., Xu, J., Zhou, A., Li, J.: Local and parallel finite element algorithms for the Stokes problem. Numer. Math. 109(3), 415–434 (2008)

    Article  MathSciNet  Google Scholar 

  8. He, Y., Xu, J., Zhou, A.: Local and parallel finite element algorithms for the Navier-Stokes problem. J. Comput. Math. 24(3), 227–238 (2006)

    MathSciNet  Google Scholar 

  9. Hecht, F.: New development in FreeFem++. J. Numer. Math. 20(3–4), 251–265 (2012)

    MathSciNet  Google Scholar 

  10. Layton, W., L. Tobiska, L.: A two-level method with backtracking for the Navier-Stokes equations. SIAM J. Numer. Anal. 35(5), 2035–2054 (1998)

  11. Li, Q., Du, G.: Local and parallel finite element methods based on two-grid discretizations for the nonstationary Navier-Stokes equations. Numer. Algor. 88(4), 1915–1936 (2021)

    Article  MathSciNet  Google Scholar 

  12. Shang, Y., He, Y.: Parallel iterative finite element algorithms based on full domain partition for the stationary Navier-Stokes equations. Appl. Numer. Math. 60(7), 719–737 (2010)

    Article  MathSciNet  Google Scholar 

  13. Ran, H., Zheng, B., Shang, Y.: A parallel finite element variational multiscale method for the Navier-Stokes equations with nonlinear slip boundary conditions. Appl. Numer. Math. 168, 274–292 (2021)

    Article  MathSciNet  Google Scholar 

  14. Shang, Y., Wang, K.: Local and parallel finite element algorithms based on two-grid discretizations for the transient Stokes equations. Numer. Algor. 54(2), 195–218 (2010)

    Article  MathSciNet  Google Scholar 

  15. Wang, X., Du, G.: Local and parallel stabilized finite element methods based on two-grid discretizations for the Stokes equations. Numer. Algor. 93(1), 67–83 (2023)

    Article  MathSciNet  Google Scholar 

  16. Xu, J., Zhou, A.: Local and parallel finite element algorithms based on two-grid discretizations. Math. Comput. 69(231), 881–909 (2000)

    Article  MathSciNet  Google Scholar 

  17. Yu, J., Shi, F., Zheng, H.: Local and parallel finite element algorithms based on the partition of unity for the Stokes problem. SIAM J. Sci. Comput. 36(5), C547–C567 (2014)

    Article  MathSciNet  Google Scholar 

  18. Zheng, B., Qin, J., Shang, Y.: Stability and convergence of some parallel iterative subgrid stabilized algorithms for the steady Navier-Stokes equations. Adv. Comput. Math. 48(3), 35 (2022)

    Article  MathSciNet  Google Scholar 

  19. Zheng, H., Yu, J., Shi, F.: Local and parallel finite element method based on the partition of unity for incompressible flow. J. Sci. Comput. 65(2), 512–532 (2015)

    Article  MathSciNet  Google Scholar 

  20. Zuo, L., Du, G.: A parallel two-grid linearized method for the coupled Navier-Stokes-Darcy problem. Numer. Algor. 77(1), 151–165 (2018)

    Article  MathSciNet  Google Scholar 

Download references

Acknowledgements

The authors would like to thank the reviewers for their constructive comments, which allowed for the improvement of the presentation of the results.

Funding

This work is subsidized by the National Natural Science Foundation of China (Nos. 12172202, 12001234), the Support Plan for Outstanding Youth Innovation Team in Shandong Higher Education Institutions (No. 2022KJ249), and the Natural Science Foundation of Shandong Province (No. ZR2021MA063).

Author information

Authors and Affiliations

  1. School of Mathematics and Statistics, Shandong Normal University, Jinan, 250014, China

    Guangzhi Du

  2. School of Mathematical Sciences, University of Jinan, Jinan, 250022, China

    Liyun Zuo

Authors
  1. Guangzhi Du
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Liyun Zuo
    View author publications

    You can also search for this author inPubMed Google Scholar

Contributions

GD: formal analysis, visualization, writing, review. LZ: conceptualization, methodology, validation, review. All authors reviewed the manuscript.

Corresponding author

Correspondence to Liyun Zuo.

Ethics declarations

Ethical approval and consent to participate

Not applicable

Consent for publication

Not applicable

Competing interests

The authors declare no competing interests.

Human and animal ethics

Not applicable

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Du, G., Zuo, L. Improved parallel finite element methods for the stationary Navier–Stokes problem. Numer Algor 97, 1383–1396 (2024). https://doi.org/10.1007/s11075-024-01754-7

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11075-024-01754-7

Keywords

Mathematics Subject Classification (2010)