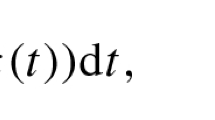Abstract
The paper deals with a number of variational dynamic problems with parameters subject to unknown smooth drift in time. Solution schemes are considered using both the classical variational method and reduction of the original problem to a conditional nonholonomic adaptive optimal control problem. In the second case, a solution is found with the help of the dynamic programming method and a specially chosen adjustment algorithm for unknown parameters.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
REFERENCES
Grodzovskii, G.L., Ivanov, Yu.N., and Tokarev, V.V., Mekhanika kosmicheskogo poleta. Problemy optimizatsii (Spaceflight Mechanics. Optimization Problems), Moscow: Nauka, 1975.
Il’in, V.A. and Kuzmak, G.E., Optimal’nye perelety kosmicheskikh apparatov s dvigatelyami bol’shoi tyagi (Optimal Flights of Spacecrafts with High-Thrust Engines), Moscow: Nauka, 1976.
Okhotsimskii, D.E. and Sikharulidze, Yu.G., Osnovy mekhaniki kosmicheskogo poleta (Foundations of Spaceflight Mechanics), Moscow: Nauka, 1990.
Tertychnyi-Dauri, V.Yu., Adaptivnaya mekhanika (Adaptive Mechanics), Moscow: Nauka, Fizmatlit, 1998.
Tertychnyi-Dauri, V.Yu., Stokhasticheskaya mekhanika (Stochastic Mechanics), Moscow: Faktorial, 2001.
Young, L.C., Lectures on the Calculus of Variations and Optimal Control Theory, Philadelphia: Saunders, 1969. Translated under the title Lektsii po variatsionnomu ischisleniyu i teorii optimal’nogo upravleniya, Moscow: Mir, 1974.
El’sgol’ts, L.E., Differentsial’nye uravneniya i variatsionnoe ischislenie, Moscow: Nauka, 1969, 2nd ed. Translated under the title Differential Equations and the Calculus of Variations, Moscow: Mir, 1970.
Korn, G.A. and Korn, T.M., Mathematical Handbook for Scientists and Engineers, New York: McGraw-Hill, 1968. Translated under the title Spravochnik po matematike dlya nauchnykh rabotnikov i inzhenerov, Moscow: Nauka, 1974.
Gabasov, R. and Kirillova, F.M., Kachestvennaya teoriya optimal’nykh protsessov, Moscow: Nauka, 1971. Translated under the title The Qualitative Theory of Optimal Processes, New York: Dekker, 1976.
Boltyanskii, V.G., Matematicheskie metody optimal’nogo upravleniya, Moscow: Nauka, 1969, 2nd ed. Translated under the title Mathematical Methods of Optimal Control, New York: Holt, Rinehart, Winston, 1971.
Chernous’ko, F.L. and Kolmanovskii, V.B., Computational and Approximate Methods of Optimal Control, in Itogi Nauki i Tekhn.,Ser. Mat. Analiz, vol. 17, Moscow: VINITI, 1977, pp. 101–166.
Tertychnyi-Dauri, V.Yu., Adaptive Optimal Nonlinear Filtering and Some Related Questions. I, II, Avtomat. Telemekh., 2001, vol. 62, no.9, pp. 125–137; 2002, vol. 63, no. 1, pp. 86–101 [Automat. Remote Control (Engl. Transl.), 2001, vol. 62, no. 9, pp. 1511–1522; 2002, vol. 63, no. 1, pp. 76–89].
Tertychnyi-Dauri, V.Yu., Parametric Filtering: Optimal Synthesis on a Nonlinear Dynamic Variety, Probl. Peredachi Inf., 2001, vol. 37, no.4, pp. 97–111 [Probl. Inf. Trans. (Engl. Transl.), 2001, vol. 37, no. 4, pp. 365–379].
Beletskii, V.V. and Egorov, V.A., Interplanetary Flights with Constant-Power Engines, Kosmich. Issled., 1964, vol. 2, no.3, pp. 360–372.
Author information
Authors and Affiliations
Additional information
Translated from Problemy Peredachi Informatsii, No. 1, 2005, pp. 53–67.
Original Russian Text Copyright © 2005 by Tertychnyi-Dauri.
Rights and permissions
About this article
Cite this article
Tertychnyi-Dauri, V.Y. Solution of variational dynamic problems under parametric uncertainty. Probl Inf Transm 41, 45–58 (2005). https://doi.org/10.1007/s11122-005-0009-3
Received:
Issue Date:
DOI: https://doi.org/10.1007/s11122-005-0009-3