Abstract
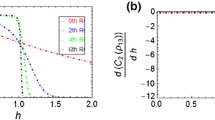
The effect of Dzialoshinski–Moriya (DM) interaction on the tripartite thermal entanglement of a spin-star model with four spins has been analyzed by an entanglement measure of the tripartite negativity. Our results imply that the tripartite thermal entanglement can be established among the three surrounding parties which do not interact with each other but interact with the central party independently. From the results, we find that the strong DM interaction can enhance the tripartite thermal entanglement while the high temperature can shrink it. The effect of the inhomogeneous coupling on the tripartite thermal entanglement is also discussed.
Similar content being viewed by others
Explore related subjects
Discover the latest articles, news and stories from top researchers in related subjects.References
Bennett C.H.: Quantum cryptography using any two nonorthogonal states. Phys. Rev. Lett. 68(21), 3121–3124 (1992)
Bennett C.H., Brassard G., Crepeau C., Jozsa R., Peres A., Wootters W.K.: Teleporting an unknown quantum state via dual classical and Einstein-Podolsky-Rosen channels. Phys. Rev. Lett. 70(13), 1895–1899 (1993)
Bouwmeester D., Pan J.W., Daniell M., Weinfurter H., Zeilinger A.: Observation of three-photon Greenberger-Horne-Zeilinger entanglement. Phys. Rev. Lett. 82(7), 1345–1349 (1999)
Nielsen M.A., Chuang I.L.: Quantum Computation and Quantum Information, pp. 571–590. Cambridge University Press, Cambridge (2000)
Horodecki R., Horodecki P., Horodecki M., Horodecki K.: Quantum entanglement. Rev. Mod. Phys. 81(2), 865–942 (2009)
Wootters W.K.: Entanglement of formation of an arbitrary state of two qubits. Phys. Rev. Lett. 80(10), 2245–2248 (1998)
Zyczkowski K., Horodecki P., Sanpera A., Lewenstein M.: Volume of the set of separable states. Phys. Rev. A 58(2), 883–892 (1998)
Vidal G., Werner R.F.: Computable measure of entanglement. Phys. Rev. A 65(3), 032314 (2002)
Peres A.: Separability criterion for density matrices. Phys. Rev. Lett. 77(8), 1413–1415 (1996)
Coffman V., Kundu J., Wootters W.K.: Distributed entanglement. Phys. Rev. A 61(5), 0532306 (2000)
Yu C.S., Song H.S.: Free entanglement measure of multiparticle quantum states. Phys. Lett. A 330(5), 377–383 (2004)
Meyer D., Wallach N.R.: Global entanglement in multiparticle systems. J. Math. Phys. 43(9), 4273–4278 (2002)
Brennen G.K.: An observable measure of entanglement for pure states of multi-qubit systems. Quantum Inf. Comput. 3(6), 619–626 (2003)
Pan F., Liu D., Lu G., Draayer J.P.: Simple entanglement measure for multipartite pure states. Int. J. Theor. Phys. 43(5), 1241–1247 (2004)
Facchi P., Florio G., Pascazio S.: Probability-density-function characterization of multipartite entanglement. Phys. Rev. A 74(4), 042331 (2006)
Sabin C., Garcia-Alcaine G.: A classification of entanglement in three-qubit systems. Eur. Phys. J. D 48(3), 435–442 (2008)
Breuer H.P., Burgarth D., Petruccione F.: Non-Markovian dynamics in a spin star system: exact solution and approximation techniques. Phys. Rev. B 70(4), 045323 (2004)
Krovi H., Oreshkov O., Ryazanov M., Lidar D.A.: Non-Markovian dynamics of a qubit coupled to an Ising spin bath. Phys. Rev. A 76(5), 052117 (2007)
Ferraro E., Breuer H.P., Napoli A., Jivulescu M.A., Messina A.: Non-Markovian dynamics of a single electron spin coupled to a nuclear spin bath. Phys. Rev. B 78(6), 064309 (2008)
Rossini D., Facchi P., Fazio R., Florio G., Lidar D.A., Pascazio S., Plastina F., Zanardi P.: Bang-bang control of a qubit coupled to a quantum critical spin bath. Phys. Rev. A 77(5), 052112 (2008)
Arshed N., Toor A.H., Lidar D.A.: Channel capacities of an exactly solvable spin-star system. Phys. Rev. A 81(6), 062353 (2010)
Chen Y., Shao X.Q., Zhu A., Yeon K.H., Yu S.C.: Improving fidelity of quantum cloning via the Dzyaloshinskii–Moriya interaction in a spin network. Phys. Rev. A 81(3), 032338 (2010)
Yang W.-L., Wei H., Feng M., An J.-H.: Tunable thermal entanglement in an effective spin-star system using coupled microcavities. Chinese Phys. B 18(9), 3677–3686 (2009)
Anza F., Militello B., Messina A.: Tripartite thermal correlations in an inhomogeneous spinCstar system. J. Phys. B 43(20), 205501 (2010)
Militello B., Messina A.: Genuine tripartite entanglement in a spin-star network at thermal equilibrium. Phys. Rev. A 83(4), 042305 (2011)
Huber M., Mintert F., Gabriel A., Hiesmayr B.C.: Detection of high-dimensional genuine multipartite entanglement of mixed states. Phys. Rev. Lett. 104(21), 210501 (2010)
Dzialoshinski I.: A thermodynamic theory of weak ferromagnetism of antiferromagnetics. J. Phys. Chem. Solid 4(4), 241–255 (1958)
Moriya T.: New mechanism of anisotropic superexchange interaction. Phys. Rev. Lett. 4(5), 228–230 (1960)
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Ma, X.S., Zhao, G.X., Zhang, J.Y. et al. Tripartite entanglement of a spin star model with Dzialoshinski–Moriya interaction. Quantum Inf Process 12, 321–329 (2013). https://doi.org/10.1007/s11128-012-0378-7
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11128-012-0378-7