The general theory of three-party quantum secret sharing protocols over phase-damping channels

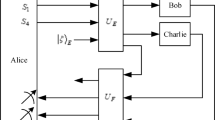
Abstract

The general theory of three-party QSS protocols with the noisy quantum channels is discussed. When the particles are transmitted through the noisy quantum channels, the initial pure three-qubit tripartite entangled states would be changed into mixed states. We analyze the security of QSS protocols with the different kinds of three-qubit tripartite entangled states under phase-damping channels and figure out, for different kinds of initial states, the successful probabilities that Alice’s secret can be recovered by legal agents are different. Comparing with one recent QSS protocol based on GHZ states, our scheme is secure, and has a little smaller key rate than that of the recent protocol.

Appendix

By the coincidence between the state of second particle and that of the third particle, the states shown in Eq. (2) can be easily divided into four groups, $\{\rho ^{\prime }_{II}(\rho ^{\prime }_{ZZ}), \rho ^{\prime }_{IZ}(\rho ^{\prime }_{ZI})\}, \{\rho ^{\prime }_{IX}(\rho ^{\prime }_{ZY}), \rho ^{\prime }_{IY}(\rho ^{\prime }_{ZX})\}, \{\rho ^{\prime }_{XI}(\rho ^{\prime }_{YZ}), \rho ^{\prime }_{XZ}(\rho ^{\prime }_{YI})\}$, and $\{\rho ^{\prime }_{XX}(\rho ^{\prime }_{YY}), \rho ^{\prime }_{XY}(\rho ^{\prime }_{YX})\}$. The two states in each group can be discriminated in same way. Now we give the discrimination between

$$\begin{aligned} \rho ^{\prime }_{II}=\frac{1}{2}(|000\rangle \langle 000|+|111\rangle \langle 111|)+\frac{(1-p)^4}{2}(|000\rangle \langle 111|+|111\rangle \langle 000|) \end{aligned}$$

and

$$\begin{aligned} \rho ^{\prime }_{IZ}=\frac{1}{2}(|000\rangle \langle 000|+|111\rangle \langle 111|)-\frac{(1-p)^4}{2}(|000\rangle \langle 111|+|111\rangle \langle 000|), \end{aligned}$$

which are appeared with the prior probabilities $1/2$ and $1/2$, respectively. From the forms of $\rho ^{\prime }_{II}$ and $\rho ^{\prime }_{IZ}$, we know that the support set of $\rho ^{\prime }_{II}$ and that of $\rho ^{\prime }_{IZ}$ are same, so there is no unambiguous discrimination between these two mixed states. Thus the maximum confidence discrimination is considered.

Suppose the positive detection operators are $\Pi _{II}, \Pi _{IZ}$ and $\Pi _2=I-\Pi _{II}-\Pi _{IZ}$, where they satisfy that $\Pi _{II}, \Pi _{IZ}, \Pi _2\ge 0$. The failure probability is $Q=1-tr(\rho \Pi _{II})-tr(\rho \Pi _{IZ})$, where the density operator $\rho$ is equal to $1/2\rho ^{\prime }_{II}+1/2\rho ^{\prime }_{IZ}$. The confidences are also introduced by $C_j=\frac{tr(\rho ^{\prime }_j\Pi _j)}{2\times tr(\rho \Pi _j)}$ with $j=II,IZ$. We define the positive operators ${\tilde{\rho }}_j=\frac{1}{2}\rho ^{-1/2}\rho ^{\prime }_j\rho ^{-1/2}$, so there exist

$$\begin{aligned} {\tilde{\rho }}_{II}=\frac{1}{2}(|000\rangle \langle 000|+|111\rangle \langle 111|)+\frac{(1-p)^4}{2}(|000\rangle \langle 111|+|111\rangle \langle 000|),\\ {\tilde{\rho }}_{IZ}=\frac{1}{2}(|000\rangle \langle 000|+|111\rangle \langle 111|)-\frac{(1-p)^4}{2}(|000\rangle \langle 111|+|111\rangle \langle 000|), \end{aligned}$$

and the spectral decompositions are

$$\begin{aligned} {\tilde{\rho }}_{II}&= \frac{1+\left( 1-p\right) ^4}{2}\left( \frac{1}{\sqrt{2}}|000\rangle +\frac{1}{\sqrt{2}}|111\rangle \right) \left( \frac{1}{\sqrt{2}}\langle 000|+\frac{1}{\sqrt{2}}\langle 111|\right) \\&\quad +\frac{1-\left( 1-p\right) ^4}{2}\left( \frac{1}{\sqrt{2}}|000\rangle +\frac{1}{\sqrt{2}}|111\rangle \right) \left( \frac{1}{\sqrt{2}}\langle 000|+\frac{1}{\sqrt{2}}\langle 111|\right) ,\\ {\tilde{\rho }}_{IZ}&= \frac{1-\left( 1-p\right) ^4}{2}\left( \frac{1}{\sqrt{2}}|000\rangle +\frac{1}{\sqrt{2}}|111\rangle \right) \left( \frac{1}{\sqrt{2}}\langle 000|+\frac{1}{\sqrt{2}}\langle 111|\right) \\&\quad +\frac{1+\left( 1-p\right) ^4}{2}\left( \frac{1}{\sqrt{2}}|000\rangle +\frac{1}{\sqrt{2}}|111\rangle \right) \left( \frac{1}{\sqrt{2}}\langle 000|+\frac{1}{\sqrt{2}}\langle 111|\right) . \end{aligned}$$

When $\tilde{\Pi }_j=\frac{\rho ^{1/2}\Pi _j\rho ^{1/2}}{tr(\rho \Pi _j)}$ for $j=II, IZ$, we have $C_j=tr({\tilde{\rho }}_j\tilde{\Pi }_j)$. In order to maximizing $C_j$, the operators $\tilde{\Pi }_j$ should be diagonalized under the basis $\{|v_0\rangle \langle v_0|=(|000\rangle +|111\rangle )(\langle 000|+\langle 111|)/2, |v_1\rangle \langle v_1|=(|000\rangle +|111\rangle )(\langle 000|+\langle 111|)/2\}$. Because of $tr(\tilde{\Pi }_j)=1$, the maximum confidences take the form $C_{II}^{max}=C_{IZ}^{max}=[1+(1-p)^4]/2$, which are the largest eigenvalues of the operators ${\tilde{\rho }}_{II}$ and ${\tilde{\rho }}_{IZ}$. According to the values of $\tilde{\Pi }_j$, there have $\Pi _{II}=c_{II}\rho ^{-1/2}|v_0\rangle \langle v_0|\rho ^{-1/2}=a_{II}|w_0\rangle \langle w_0|$ and $\Pi _{IZ}=c_{IZ}\rho ^{-1/2}|v_1\rangle \langle v_1|\rho ^{-1/2}=a_{IZ}|w_1\rangle \langle w_1|$, where the normalized states $|w_j\rangle =\frac{\rho ^{-1/2}|v_j\rangle }{\sqrt{\langle v_j|\rho ^{-1}|v_j\rangle }}$ for $j=0, 1$ are

$$\begin{aligned} |w_0\rangle =\frac{1}{\sqrt{2}}(|000\rangle +|111\rangle ),\ |w_1\rangle =\frac{1}{\sqrt{2}}(|000\rangle -|111\rangle ). \end{aligned}$$

Thus the minimum failure probability $Q=tr(\rho \Pi _2)=0$ is reached, when $a_{II}=a_{IZ}=1$. So the space spanned by the measurement operators $\Pi _{II}$ and $\Pi _{IZ}$ is same with the space spanned by $\rho ^{\prime }_{II}$ and $\rho ^{\prime }_{IZ}$. At the same time, the optimal measurement operators are $\Pi _{II}=(\frac{1}{\sqrt{2}}|000\rangle +\frac{1}{\sqrt{2}}|111\rangle )(\frac{1}{\sqrt{2}}\langle 000|+\frac{1}{\sqrt{2}}\langle 111|)$ and $\Pi _{IZ}=(\frac{1}{\sqrt{2}}|000\rangle -\frac{1}{\sqrt{2}}|111\rangle )(\frac{1}{\sqrt{2}}\langle 000|-\frac{1}{\sqrt{2}}\langle 111|)$. The error rate $P=[tr(\rho ^{\prime }_{II}\Pi _{IZ})+tr(\rho ^{\prime }_{IZ}\Pi _{II})]/2=[1-(1-p)^4]/2$ is related with the parameter of channel $p$. Until now, we give the discrimination of the $\rho ^{\prime }_{II}$ and $\rho ^{\prime }_{IZ}$ with maximum confidence and the minimum failure probability. The discrimination of other group states in Eq. (2) is in the same way.