Skip to main content

Advertisement

Springer Nature Link
Log in

Implementing a one-bit reversible full adder using quantum-dot cellular automata

  • Published:
Quantum Information Processing Aims and scope Submit manuscript

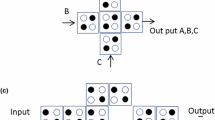
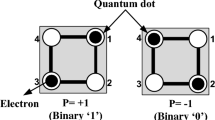
Abstract

Quantum-dot cellular automata (QCA) technique is one of the emerging and promising nanotechnologies. It has considerable advantages versus CMOS technology in various aspects such as extremely low power dissipation, high operating frequency and small size. In this paper, designing of a one-bit full adder is investigated using a QCA implementation of Toffoli and Fredkin gates. Then, a full adder design with reversible QCA1 gates is proposed regarding to overhead and power savings. Our proposed full adder design is more preferable when considering both circuit area and speed. The proposed design uses only two QCA1 gates and maximizes the circuit density and focuses on a layout of the circuit which is minimal in using QCA cells.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

References

  1. Landauer, R.: Irreversibility and heat generation in the computing processes. IBM J. Res. Dev. 5, 183–191 (1961)

    Article  MathSciNet  MATH  Google Scholar 

  2. Huang, J., Metra, Ma, X., Metra, C., Lombardi, F.: Testing reversible 1D arrays for molecular QCA. In: Proceedings of the 21st IEEE International Symposium on Defect and Fault-Tolerance in VLSI Systems, pp. 71–79 (2006)

  3. Bennett, C.: Logic reversibility of computation. IBM J. Res. Dev. 17, 525–532 (1973)

    Article  MATH  Google Scholar 

  4. Gupta, P., Jha, N.K., Lingappan, L.: test generation framework for combinational quantum cellular automata circuits. IEEE Trans. VLSI Syst. 15(1), 24–36 (2007)

    Article  Google Scholar 

  5. Askari, M., Taghizadeh, M.: Logic circuit design in nano-scale using quantum-dot cellular automata. Eur. J. Sci. Res. 48(3), 516–526 (2011). ISSN 1450–216X

  6. Hennessy, K., Lent, C.S.: Clocking of molecular quantum-dot cellular automata. J. Vac. Sci. Technol. 19(5), 1752–1755 (2001)

    Article  Google Scholar 

  7. Tahoori, M.B., Huang, J., Momenzadeh, M., Lombardi, F.: Testing of quantum cellular automata. IEEE Trans. Nanotechnol. 3(4), 432–442 (2004)

    Article  ADS  Google Scholar 

  8. Ma, X., Huang, J., Metra, C., Lombardi, F.: Reversible and Testable Circuits for Molecular QCA Design. Northeastern University, ECE Department, Internal report (2007)

  9. Amlani, I., Orlov, A.O., Snider, G.L., Lent, C.S.: Demonstration of a six-dot quantum cellular automata system. Appl. Phys. Lett. 72(17), 2179–2181 (1998)

    Article  ADS  Google Scholar 

  10. Orlov, A.O., Amlani, I., Bernstein, G.H., Lent, C.S., Snider, G.L.: Realization of a functional cell for quantum-dot cellular automata. Science 277, 928–930 (1997)

    Article  Google Scholar 

  11. Walus, K., Jullien, G.A., Dimitrov, V.S.: Computer arithmetic structures for quantum cellular automata. In: Proceedings of Asimolar Conference (2003)

  12. Ma, X.: Physical/biochemical inspired computing models for reliability Nano-technology systems. Computer Engineering Dissertations (2008). Paper 2. http://hdl.handle.net/2047/d10017859

  13. Kyosun, K., Kaijie, W., Ramesh, K.: Quantum-dot cellular automata design guideline. IEICE Trans. Fundam. Electron. Commun. Comput. Sci. E89–A(6), 1607–1614 (2006)

    ADS  Google Scholar 

  14. Toffoli, T.: Reversible Computing. MIT Laboratory for Computer Science, Technical Report MIT/LCS/TM-151, Feb (1980)

  15. Maslov, D., Dueck, G.W.: Synthesis of Fredkin-Toffoli reversible networks. IEEE Trans. VLSI (2004)

  16. Mohammadi, M., Eshghi, M.: Heuristic Methods to use don’t cares in automated design of reversible and quantum logic circuits. Quantum Inf. Process. 7(4), 175–192 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  17. Maslov, D., Dueck, G.W.: Garbage in reversible design of multiple output functions. In: 6th International Symposium on Representations and Methodology of Future Computing Technologies, Trier (1980)

  18. Mohammadi, M., Eshghi, M.: On figures of merit in reversible and quantum logic designs. Quantum Inf. Process. 8(4), 297–318 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  19. Wang, W., Walus, K., Jullien, G.A.: Quantum-dot cellular automata adders. IEEE-Nano Third IEEE Conf. Nanotechnol. 1, 461–464 (2003)

    Article  Google Scholar 

  20. Wang, W., Zhang, R., Walus, K., Jullien, G.A.: A method of majority logic reduction for quantum cellular automata. IEEE Trans. Nanotechnol. 3(4), 443–450 (2004)

    Article  ADS  Google Scholar 

  21. Walus, K., Schulhof, G., Jullien, G.A.: High level exploration of quantum-dot cellular automata. In: Proc. Conf. Signals, Systems, and Computers, pp. 7–10, Nov (2004)

  22. QCA Designer [Online]. Available: http://www.mina.ubc.ca

Download references

Author information

Authors and Affiliations

  1. Department of Computer Engineering, Kerman Branch, Islamic Azad University, Kerman, Iran

    Zahra Mohammadi

  2. Department of Computer Engineering, Shahid Bahonar University of Kerman, Kerman, Iran

    Majid Mohammadi

Authors
  1. Zahra Mohammadi
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Majid Mohammadi
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Majid Mohammadi.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Mohammadi, Z., Mohammadi, M. Implementing a one-bit reversible full adder using quantum-dot cellular automata. Quantum Inf Process 13, 2127–2147 (2014). https://doi.org/10.1007/s11128-014-0782-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11128-014-0782-2

Keywords