Abstract
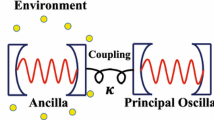
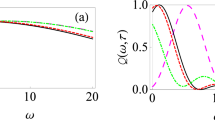
This paper presents a Green’s function-based root locus method to investigate the boundary between Markovian and non-Markovian open quantum systems in the frequency domain. A Langevin equation for the boson-boson coupling system is derived, where we show that the structure of the Green’s function dominates the system dynamics. In addition, by increasing the coupling between the system and its environment, the system dynamics are driven from Markovian to non-Markovian dynamics, which results from the redistribution in the modes of the Green’s function in the frequency domain. Both a critical transition and a critical point condition under Lorentzian noise are graphically presented using a root locus method. Related results are verified using an example of a boson-boson coupling system.




Similar content being viewed by others
Explore related subjects
Discover the latest articles, news and stories from top researchers in related subjects.References
Breuer, H.P., Petruccione, F.: The Theory of Open Quantum Systems. Oxford University Press, Oxford (2002)
Divincenzo, D.P.: Quantum computation. Science 270(5234), 255–261 (1995)
Chirolli, L., Burkard, G.: Decoherence in solid-state qubits. Adv. Phys. 57(3), 225–285 (2008)
Zhou, D., Lang, A., Joynt, R.: Disentanglement and decoherence from classical non-Markovian noise: random telegraph noise. Quantum Inf. Process. 9(6), 727–747 (2010)
Gardiner, C., Zoller, P.: Quantum Noise. Springer, Berlin (2000)
Petersen, I.R.: Cascade cavity realization for a class of complex transfer functions arising in coherent quantum feedback control. Automatica 47(8), 1757–1763 (2011)
Hassen, S.Z.S., Heurs, M., Huntington, E.H., Petersen, I.R., James, M.R.: Frequency locking of an optical cavity using linear-quadratic Gaussian integral control. J. Phys. B At. Mol. Phys. 42(17), 175501 (2009)
Xue, S., Wu, R.B., Zhang, W.M., Zhang, J., Li, C.W., Tarn, T.J.: Decoherence suppression via non-Markovian coherent feedback control. Phys. Rev. A 86, 052304 (2012)
Tu, M.W.Y., Zhang, W.M.: Non-Markovian decoherence theory for a double-dot charge qubit. Phys. Rev. B 78(23), 235311 (2008)
Tu, M.W.Y., Lee, M.T., Zhang, W.M.: Exact master equation and non-Markovian decoherence for quantum dot quantum computing. Quantum Inf. Process. 8(6), 631–646 (2009)
Wu, R.-B., Li, T.-F., Kofman, A., Zhang, J., Liu, Yx, Pashkin, Y., Tsai, J.-S., Nori, F.: Spectral analysis and identification of noises in quantum systems. Phys. Rev. A 87, 022324 (2013)
Longhi, S.: Non-Markovian decay and lasing condition in an optical microcavity coupled to a structured reservoir. Phys. Rev. A 74, 063826 (2006)
Tan, H.T., Zhang, W.M.: Non-Markovian dynamics of an open quantum system with initial system-reservoir correlations: A nanocavity coupled to a coupled-resonator optical waveguide. Phys. Rev. A 83(3), 032102 (2011)
Burkard, G.: Non-Markovian qubit dynamics in the presence of \(1/f\) noise. Phys. Rev. B 79(12), 125317 (2009)
Anastopoulos, C., Shresta, S., Hu, B.L.: Non-Markovian entanglement dynamics of two qubits interacting with a common electromagnetic field. Quantum Inf. Process. 8(6), 549–563 (2009)
Xue, S., Zhang, J., Wu, R.B., Li, C.W., Tarn, T.J.: Quantum operation for a one-qubit system under a non-Markovian environment. J. Phys. B At. Mol. Phys. 44(15), 154016 (2011)
Orieux, A., DArrigo, A., Ferranti, G., Franco, R.L., Benenti, G., Paladino, E., Falci, G., Sciarrino, F., Mataloni, P.: Experimental on-demand recovery of entanglement by local operations within non-Markovian dynamics. Sci. Rep. 5, 8575 (2015)
Bellomo, B., Lo Franco, R., Maniscalco, S., Compagno, G.: Two-qubit entanglement dynamics for two different non-Markovian environments. Phys. Scr. T140, 014014 (2010)
D’Arrigo, A., Lo Franco, R., Benenti, G., Paladino, E., Falci, G.: Recovering entanglement by local operations. Ann. Phys. 350, 211–224 (2014)
Bellomo, B., Lo Franco, R., Maniscalco, S., Compagno, G.: Entanglement trapping in structured environments. Phys. Rev. A 78, 060302 (2008)
Xu, J.-S., Sun, K., Li, C.-F., Xu, X.-Y., Guo, G.-C.: Experimental recovery of quantum correlations in absence of system-environment back-action. Nat. Commun. 4, 2851 (2013)
Franco, R.L., Bellomo, B., Maniscalco, S., Compagno, G.: Dynamics of quantum correlations in two-qubit systems within non-Markovian environments. Int. J. Mod. Phys. B 27, 1345053 (2013)
Chruściński, D., Maniscalco, S.: Degree of non-Markovianity of quantum evolution. Phys. Rev. Lett. 112, 120404 (2014)
Bylicka, B., Chruściński, D., Maniscalco, S.: Non-Markovianity and reservoir memory of quantum channels: a quantum information theory perspective. Sci. Rep. 4, 5720 (2014)
Zhang, W.M., Lo, P.Y., Xiong, H.N., Tu, M.W.Y., Franco, N.: General non-Markovian dynamics of open quantum systems. Phys. Rev. Lett. 109, 170402 (2012)
Lei, C.U., Zhang, W.M.: Decoherence suppression of open quantum systems through a strong coupling to non-Markovian reservoirs. Phys. Rev. A 84, 052116 (2011)
Xiong, H.N., Zhang, W.M., Tu, M.W.Y., Braun, D.: Dynamically stabilized decoherence-free states in non-Markovian open fermionic systems. Phys. Rev. A 86, 032107 (2012)
Liu, B.H., Li, L., Huang, Y.F., Li, C.F., Guo, G.C., Laine, E.M., Breuer, H.P., Piilo, J.: Experimental control of the transition from Markovian to non-Markovian dynamics of open quantum systems. Nat. Phys. 7(12), 931–934 (2011)
Breuer, H.P., Laine, E.M., Piilo, J.: Measure for the degree of non-Markovian behavior of quantum processes in open systems. Phys. Rev. Lett. 103, 210401 (2009)
Ogata, K.: Modern Control Engineering. PrenticeHall, Englewood Cliffs (1996)
Zhao, X.Y., Hedemann, S.R., Yu, T.: Restoration of a quantum state in a dephasing channel via environment-assisted error correction. Phys. Rev. A 88, 022321 (2013)
Benedetti, C., Paris, M.G.A., Maniscalco, S.: Non-Markovianity of colored noisy channels. Phys. Rev. A 89, 012114 (2014)
Xue, S., Wu, R.B., Tarn, T.J.: Modeling and analysis of non-Markovian open quantum systems for coherent feedback. In 3rd IFAC International Conference on Intelligent Control and Automation Science, 3, pp. 365–370, (2013)
Acknowledgments
This research was supported by the Australian Research Council under grant FL110100020. Re-Bing Wu acknowledges support from TNlist and Natural Science Foundation of China (Grant Nos. 61374091 and 61134008).
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Xue, S., Wu, R., Tarn, TJ. et al. Witnessing the boundary between Markovian and non-Markovian quantum dynamics: a Green’s function approach. Quantum Inf Process 14, 2657–2672 (2015). https://doi.org/10.1007/s11128-015-1000-6
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11128-015-1000-6