Skip to main content
Springer Nature Link
Log in

Geometric phase, quantum Fisher information, geometric quantum correlation and quantum phase transition in the cavity-Bose–Einstein-condensate system

  • Published:
Quantum Information Processing Aims and scope Submit manuscript

Abstract

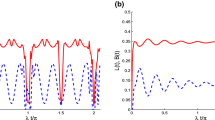
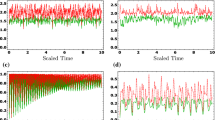
We investigate the quantum phase transition of an atomic ensemble trapped in a single-mode optical cavity via the geometric phase and quantum Fisher information of an extra probe atom which is injected into the optical cavity and interacts with the cavity field. We also find that the geometric quantum correlation between two probe atoms exhibits a double sudden transition phenomenon and show this double sudden transition phenomenon is closely associated with the quantum phase transition of the atomic ensemble. Furthermore, we propose a theoretical scheme to prolong the frozen time during which the geometric quantum correlation remains constant by applying time-dependent electromagnetic field.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

References

  1. Sachdev, S.: Quantum Phase Transition. Cambridge University Press, New York (1999)

    Google Scholar 

  2. Osterloh, A., Amico, L., Falci, G., Fazio, R.: Scaling of entanglement close to a quantum phase transition. Nature (London) 416, 608 (2002)

    Article  ADS  Google Scholar 

  3. Gu, S.-J.: Fidelity approach to quantum phase transitions. Int. J. Mod. Phys. B 24, 4371 (2010)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  4. Luo, D.-W., Xu, J.-B.: Trace distance and scaling behavior of a coupled cavity lattice at finite temperature. Phys. Rev. A 87, 013801 (2013)

    Article  ADS  Google Scholar 

  5. Dicke, R.H.: Coherence in spontaneous radiation processes. Phys. Rev. 93, 99 (1954)

    Article  ADS  MATH  Google Scholar 

  6. Baumann, K., Guerlin, C., Brennecke, F., Esslinger, T.: Dicke quantum phase transition with a superfluid gas in an optical cavity. Nature (London) 464, 1301 (2010)

    Article  ADS  Google Scholar 

  7. Sabin, C., White, A., Hackermuller, L., Fuentes, I.: Impurities as a quantum thermometer for a Bose-Einstein condensate. Sci. Rep. 4, 6436 (2014)

    Article  ADS  Google Scholar 

  8. Berry, M.V.: Quantal phase factors accompanying adiabatic changes. Proc. R. Soc. Lond. A 392, 45 (1984)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  9. Tong, D.M., Sjoqvist, E., Kwek, L.C., Oh, C.H.: Kinematic approach to the mixed state geometric phase in nonunitary evolution. Phys. Rev. Lett. 93, 080405 (2004)

    Article  ADS  Google Scholar 

  10. Yi, X.X., Wang, W.: Geometric phases induced in auxiliary qubits by many-body systems near their critical points. Phys. Rev. A 75, 032103 (2007)

    Article  ADS  MathSciNet  Google Scholar 

  11. Zhang, X., Zhang, A., Li, L.: Detecting the multi-spin interaction of an XY spin chain by the geometric phase of a coupled qubit. Phys. Lett. A 376, 2090 (2012)

    Article  ADS  MATH  Google Scholar 

  12. Zhang, A., and Li, F.: Geometric phase of a central qubit coupled to a spin chain in a thermal equilibrium state. Phys. Lett. A, 377, 528 (2103)

  13. Braunstein, S.L., Caves, C.M.: Statistical distance and the geometry of quantum states. Phys. Rev. Lett. 72, 3439 (1994)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  14. Helstrom, C.W.: Quantum Detection and Estimation Theory. AcademicPress, New York (1976)

    MATH  Google Scholar 

  15. Holevo, A.S.: Probabilistic and Statistical Aspect of Quantum Theory. North-Holland, Amsterdam (1982)

    MATH  Google Scholar 

  16. Wootters, W.K.: Statistical distance and Hilbert space. Phys. Rev. D 23, 357 (1981)

    Article  ADS  MathSciNet  Google Scholar 

  17. Liu, W.-F., Ma, J., Wang, X.: Quantum Fisher information and spin squeezing in the ground state of the XY model. J. Phys. A: Math. Theor. 46, 045302 (2013)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  18. Ma, J., Wang, X.: Fisher information and spin squeezing in the Lipkin-Meshkov-Glick model. Phys. Rev. A 80, 012318 (2009)

    Article  ADS  Google Scholar 

  19. Wang, T.-L., Wu, L.-N., Yang, W., Jin, G.-R., Lambert, N., Nori, F.: Quantum Fisher information as a signature of the superradiant quantum phase transition. New J. Phys. 16, 063039 (2014)

    Article  ADS  MathSciNet  Google Scholar 

  20. Nielsen, M.A., Chuang, I.L.: Quantum Computation and QuantumInformation. Cambridge University Press, Cambridge (2000)

    Google Scholar 

  21. Modi, K., Brodutch, A., Cable, H., Paterek, T., Vedral, V.: The classical-quantum boundary for correlations: discord and related measures. Rev. Mod. Phys. 84, 1655 (2012)

    Article  ADS  Google Scholar 

  22. Dakic, B., Vedral, V., Brukner, C.: Necessary and sufficient condition for nonzero quantum discord. Phys. Rev. Lett. 105, 190502 (2010)

    Article  ADS  MATH  Google Scholar 

  23. Paula, F.M., de Oliveira, T.R., Sarandy, M.S.: Geometric quantum discord through the Schatten 1-norm. Phys. Rev. A 87, 064101 (2013)

    Article  ADS  Google Scholar 

  24. Paula, F.M., Silva, I.A., Montealegre, J.D., Souza, A.M., deAzevedo, E.R., Sarthour, R.S., Saguia, A., Oliveira, I.S., Soares-Pinto, D.O., Adesso, G., Sarandy, M.S.: Observation of environment-induced double sudden transitions in geometric quantum correlations. Phys. Rev. Lett. 111, 250401 (2013)

    Article  ADS  Google Scholar 

  25. Paula, F.M., Montealegre, J.D., Saguia, A., deAzevedo, E.R., Sarandy, M.S.: Geometric classical and total correlations via trace distance. Europhys. Lett. 103, 50008 (2013)

    Article  ADS  Google Scholar 

  26. Montealegre, J.D., Paula, F.M., Saguia, A., Sarandy, M.S.: One-norm geometric quantum discord under decoherence. Phys. Rev. A 87, 042115 (2013)

    Article  ADS  Google Scholar 

  27. Spehner, D., Orszag, M.: Geometric quantum discord with Bures distance. New J. Phys. 15, 103001 (2013)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  28. Aaronson, B., Franco, R.L., Compagno, G., Adesso, G.: Hierarchy and dynamics of trace distance correlations. New J. Phys. 15, 093022 (2013)

    Article  ADS  Google Scholar 

  29. Ciccarello, F., Tufarelli, T., Giovannetti, V.: Toward computability of trace distance discord. New J. Phys. 16, 013038 (2014)

    Article  ADS  Google Scholar 

  30. Aaronson, B., Franco, R.L., Adesso, G.: Comparative investigation of the freezing phenomena for quantum correlations under nondissipative decoherence. Phys. Rev. A 88, 012120 (2013)

    Article  ADS  Google Scholar 

  31. Villar, P.I., Lombardo, F.C.: Geometric phases in the presence of a composite environment. Phys. Rev. A 83, 052121 (2011)

    Article  ADS  Google Scholar 

  32. Pezze, L., Smerzi, A.: Entanglement, Nonlinear Dynamics, and the Heisenberg Limit. Phys. Rev. Lett. 102, 100401 (2009)

    Article  ADS  MathSciNet  Google Scholar 

  33. Nagy, D., Konya, G., Szirmai, G., Domokos, P.: Dicke-Model Phase Transition in the Quantum Motion of a Bose-Einstein Condensate in an Optical Cavity. Phys. Rev. Lett. 104, 130401 (2010)

    Article  ADS  Google Scholar 

  34. Brennecke, F., Ritter, S., Donner, T., Esslinger, T.: Cavity optomechanics with a Bose-Einstein condensate. Science 322, 235 (2008)

    Article  ADS  Google Scholar 

  35. Brahms, N., Botter, T., Schreppler, S., Brooks, D.W.C., Stamper-Kurni, D.M.: Optical detection of the quantization of collective atomic motion. Phys. Rev. Lett. 108, 133601 (2012)

    Article  ADS  Google Scholar 

  36. de Faria, J.G.P., Nemes, M.C.: Dissipative dynamics of the Jaynes-Cummings model in the dispersive approximation: Analytical results. Phys. Rev. A 59, 3918 (1999)

    Article  ADS  Google Scholar 

  37. Yuan, J.-B., Kuang, L.-M.: Quantum-discord amplification induced by a quantum phase transition via a cavity-Bose-Einstein-condensate system. Phys. Rev. A 87, 024101 (2013)

    Article  ADS  Google Scholar 

  38. Huang, J.-F., Li, Y., Liao, J.-Q., Kuang, L.-M., Sun, C.P.: Dynamic sensitivity of photon-dressed atomic ensemble with quantum criticality. Phys. Rev. A 80, 063829 (2009)

    Article  ADS  Google Scholar 

  39. Sun, Z., Ma, J., Lu, X.-M., Wang, X.: Fisher information in a quantum-critical environment. Phys. Rev. A 82, 022306 (2010)

    Article  ADS  Google Scholar 

  40. Solano, E., Agarwal, G.S., Walther, H.: Strong-Driving-Assisted Multipartite Entanglement in Cavity QED. Phys. Rev. Lett. 90, 027903 (2003)

    Article  ADS  Google Scholar 

  41. Zhang, J.-S., Xu, J.-B.: Control of the entanglement of a two-level atom in a dissipative cavity via a classical field. Opt. Commun. 282, 2543 (2009)

    Article  ADS  Google Scholar 

Download references

Acknowledgments

This project was supported by the National Natural Science Foundation of China (Grant No. 11274274) and the fundamental Research Funds for the Central Universities (Grant No. 2016FZA3004).

Author information

Authors and Affiliations

  1. Zhejiang Institute of Modern Physics and Physics Department, Zhejiang University, Hangzhou, 310027, China

    Wei Wu & Jing-Bo Xu

Authors
  1. Wei Wu
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Jing-Bo Xu
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Jing-Bo Xu.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wu, W., Xu, JB. Geometric phase, quantum Fisher information, geometric quantum correlation and quantum phase transition in the cavity-Bose–Einstein-condensate system. Quantum Inf Process 15, 3695–3709 (2016). https://doi.org/10.1007/s11128-015-1186-7

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11128-015-1186-7

Keywords