Abstract
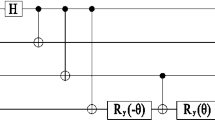
In this paper, we propose a novel scheme for deterministic controlled remote state preparation (CRSP) of arbitrary two-qubit states. Suitably chosen partially entangled state is used as the quantum channel. With proper projective measurements carried out by the sender and controller, the receiver can reconstruct the target state by means of appropriate unitary operation. Unit success probability can be achieved for arbitrary two-qubit states. Different from some previous CRSP schemes utilizing partially entangled channels, auxiliary qubit is not required in our scheme. We also show that the success probability is independent of the parameters of the partially entangled quantum channel.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Nielsen, M.A., Chuang, I.L.: Quantum Computation and Quantum Information. Cambridge University Press, Cambridge (2000)
Bennett, C.H., Brassard, G., Crépeau, C., Jozsa, R., Peres, A., Wootters, W.K.: Teleporting an unknown quantum state via dual classical and Einstein–Podolsky–Rosen channels. Phys. Rev. Lett. 70, 1895 (1993)
Lo, H.K.: Classical-communication cost in distributed quantum-information processing: a generalization of quantum-communication complexity. Phys. Rev. A 62, 012313 (2000)
Pati, A.K.: Minimum classical bit for remote preparation and measurement of a qubit. Phys. Rev. A 63, 014302 (2000)
Bennett, C.H., DiVincenzo, D.P., Shor, P.W., Smolin, J.A., Terhal, B.M., Wootters, W.K.: Remote state preparation. Phys. Rev. Lett. 87, 077902 (2001)
Devetak, I., Berger, T.: Low-entanglement remote state preparation. Phys. Rev. Lett. 87, 197901 (2001)
Berry, D.W., Sanders, B.C.: Optimal remote state preparation. Phys. Rev. Lett. 90, 057901 (2003)
Abeyesinghe, A., Hayden, P.: Generalized remote state preparation: trading cbits, qubits, and ebits in quantum communication. Phys. Rev. A 68, 062319 (2003)
Leung, D.W., Shor, P.W.: Oblivious remote state preparation. Phys. Rev. Lett. 90, 127905 (2003)
Ye, M.Y., Zhang, Y.S., Guo, G.C.: Faithful remote state preparation using finite classical bits and a nonmaximally entangled state. Phys. Rev. A 69, 022310 (2004)
Peng, X.H., Zhu, X.W., Fang, X.M., Feng, M., Liu, M.L., Gao, K.L.: Experimental implementation of remote state preparation by nuclear magnetic resonance. Phys. Lett. A 306, 271 (2003)
Xiang, G.Y., Li, J., Yu, B., Guo, G.C.: Remote preparation of mixed states via noisy entanglement. Phys. Rev. A 72, 012315 (2005)
Peters, N.A., Barreiro, J.T., Goggin, M.E., Wei, T.C., Kwiat, P.G.: Remote state preparation: arbitrary remote control of photon polarization. Phys. Rev. Lett. 94, 150502 (2005)
Liu, W.T., Wu, W., Ou, B.Q., Chen, P.X., Li, C.Z., Yuan, J.M.: Experimental remote preparation of arbitrary photon polarization states. Phys. Rev. A 76, 022308 (2007)
Rosenfeld, W., Berner, S., Volz, J., Weber, M., Weinfurter, H.: Remote preparation of an atomic quantum memory. Phys. Rev. Lett. 98, 050504 (2007)
Barreiro, J.T., Wei, T.C., Kwiat, P.G.: Remote preparation of single-photon “hybrid” entangled and vector-polarization states. Phys. Rev. Lett. 105, 030407 (2010)
Wang, Z.Y., Liu, Y.M., Zuo, X.Q., Zhang, Z.J.: Controlled remote state preparation. Commun. Theor. Phys. 52, 235 (2009)
Hou, K., Wang, J., Yuan, H., Shi, S.H.: Multiparty-controlled remote preparation of two-particle state. Commun. Theor. Phys. 52, 848 (2009)
Luo, M.X., Chen, X.B., Ma, S.Y., Yang, Y.X., Hu, Z.M.: Remote preparation of an arbitrary two-qubit state with three-party. Int. J. Theor. Phys. 49, 1262 (2010)
Wang, Z.Y.: Controlled remote preparation of a two-qubit state via an asymmetric quantum channel. Commun. Theor. Phys. 55, 244 (2011)
Song, J.F., Wang, Z.Y.: Controlled remote preparation of a two-qubit state via positive operator-valued measure and two three-qubit entanglements. Int. J. Theor. Phys. 50, 2410 (2011)
Li, Z., Zhou, P.: Probabilistic multiparty-controlled remote preparation of an arbitrary m-qubit state via positive operator-valued measurement. Int. J. Quantum Inf. 10, 1250062 (2012)
Guan, X.W., Chen, X.B., Yang, Y.X.: Controlled-joint remote preparation of an arbitrary two-qubit state via non-maximally entangled channel. Int. J. Theor. Phys. 51, 3575 (2012)
Wang, D., Ye, L.: Multiparty-controlled joint remote state preparation. Quantum Inf. Process 12, 3223 (2013)
Liu, L.L., Hwang, T.: Controlled remote state preparation protocols via AKLT states. Quantum Inf. Process. 13, 1639 (2014)
Wang, C., Zeng, Z., Li, X.H.: Controlled remote state preparation via partially entangled quantum channel. Quantum Inf. Process. 14, 1077 (2015)
An, N.B., Bich, C.T.: Perfect controlled joint remote state preparation independent of entanglement degree of the quantum channel. Phys. Lett. A 378, 3582 (2014)
Li, X.H., Ghose, S.: Control power in perfect controlled teleportation via partially entangled channels. Phys. Rev. A 90, 052305 (2014)
Wootters, W.K.: Entanglement of formation of an arbitrary state of two qubits. Phys. Rev. Lett. 80, 2245 (1998)
Acknowledgments
We are grateful to the patient and professional guidance of the anonymous reviewers. This work is supported by the National Natural Science Foundation of China (Grant Nos. 61372076, 61301171) and the 111 Project (Grant No. B08038).
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Chen, N., Quan, D.X., Yang, H. et al. Deterministic controlled remote state preparation using partially entangled quantum channel. Quantum Inf Process 15, 1719–1729 (2016). https://doi.org/10.1007/s11128-015-1226-3
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11128-015-1226-3