Skip to main content

Advertisement

Springer Nature Link
Log in

A revisit to non-maximally entangled mixed states: teleportation witness, noisy channel and discord

  • Published:
Quantum Information Processing Aims and scope Submit manuscript

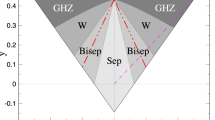
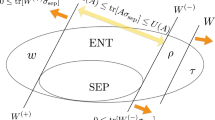
Abstract

We constructed a class of non-maximally entangled mixed states (Adhikari et al. in Quantum Inf Comput 10:0398, 2010) and extensively studied their entanglement properties and also their usefulness as teleportation channels. In this article, we have revisited our constructed state and have studied it from three different perspectives. Since every entangled state is associated with a witness operator, we have found a suitable entanglement as well as teleportation witness operator for our non-maximally entangled mixed states. We considered the noisy channel’s effects on our constructed states to see how much it affects the states’ capacities as teleportation channels. For this purpose, we have mainly focussed on amplitude damping channel. A comparative study on concurrence and quantum discord of our constructed state of Adhikari et al. (2010) has also been carried out here.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

References

  1. Adhikari, S., Majumdar, A.S., Roy, S., Ghosh, B., Nayak, N.: Teleportation via maximally and non-maximally entangled mixed states. Quantum Inf. Comput. 10, 0398 (2010)

    MathSciNet  MATH  Google Scholar 

  2. Bennett, C.H., Brassard, G., Crepeau, C., Jozsa, R., Peres, A., Wootters, W.K.: Teleporting an unknown quantum state via dual classical and Einstein–Podolsky–Rosen channels. Phys. Rev. Lett. 70, 1895 (1993)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  3. Bennett, C.H., Wiesner, S.J.: Communication via one- and two-particle operators on Einstein–Podolsky–Rosen states. Phys. Rev. Lett. 69, 2881 (1992)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  4. Hillery, M., Buzek, V., Berthiaume, A.: Quantum secret sharing. Phys. Rev. A 59, 1829 (1999)

    Article  ADS  MathSciNet  Google Scholar 

  5. Bennett, C.H., Brassard, G.: Proceedings of IEEE International Conference on Computers, Systems and Signal Processing Bangalore, India. IEEE, New York, p. 175 (1984)

  6. Bennett, C.H., Brassard, G., Mermin, N.: Quantum cryptography without Bells theorem. Phys. Rev. Lett. 68, 557 (1992)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  7. Werner, R.F.: Quantum states with Einstein–Podolsky–Rosen correlations admitting a hidden variable model. Phys. Rev. A 40, 4277 (1989)

    Article  ADS  Google Scholar 

  8. Lee, J., Kim, M.S.: Entanglement teleportation via Werner states. Phys. Rev. Lett. 84, 4236 (2000)

    Article  ADS  Google Scholar 

  9. Munro, W.J., James, D.F.V., White, A.G., Kwiat, P.G.: Maximizing the entanglement of two mixed qubits. Phys. Rev. A. 64, 030302 (2001)

    Article  ADS  Google Scholar 

  10. Hiroshima, T., Ishizaka, S.: Local and nonlocal properties of Werner states. Phys. Rev. A 62, 044302 (2000)

    Article  ADS  MathSciNet  Google Scholar 

  11. Greenberger, D.M., Horne, M.A., Zeilinger, A.: Bell’s Theorem, Quantum Theory and Conceptions of the Universe. In: Kafatos, M. (ed.), Kluwer, Dordrecht, p. 69 (1989)

  12. Greenberger, D.M., Horne, M.A., Zeilinger, A.: Bells theorem without inequalities. Am. J. Phys. 58, 1131 (1990)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  13. Zeilinger, A., Horne, M.A., Greenberger, D.M.: NASA Conference Publication Code NTT Washington, p. 3135 (1997)

  14. Adhikari, S., Majumdar, A.S., Home, D., Pan, A.K., Joshi, P.: Quantum teleportation using non-orthogonal entangled channels. Phys. Scr. 85, 045001 (2012)

    Article  ADS  MATH  Google Scholar 

  15. Eibl, M., Kiesel, N., Bourennane, M., Kurtsiefer, C., Weinfurter, H.: Experimental realization of a three-qubit entangled W state. Phys. Rev. Lett. 92, 077901 (2004)

    Article  ADS  Google Scholar 

  16. Lu, H., Chen, L.-K., Liu, C., Xu, P., Yao, X.-C., Li, L., Liu, N.-L., Zhao, B., Chen, Y.-A., Pan, J.-W.: Experimental realization of a concatenated Greenberger–Horne–Zeilinger state for macroscopic quantum superpositions. Nat. Photonics 8, 364 (2014)

    Article  ADS  Google Scholar 

  17. Yu, T., Eberly, J.H.: Finite-time disentanglement via spontaneous emission. Phys. Rev. Lett. 93, 140404 (2004)

    Article  ADS  Google Scholar 

  18. Yu, T., Eberly, J.H.: Quantum open system theory: bipartite aspects. Phys. Rev. Lett. 97, 140403 (2006)

    Article  ADS  Google Scholar 

  19. Bose, S., Knight, P.L., Plenio, M.B., Vedral, V.: Proposal for teleportation of an atomic state via cavity decay. Phys. Rev. Lett. 83, 5158 (1999)

    Article  ADS  Google Scholar 

  20. Cirelson, B.S.: Quantum generalizations of Bells inequality. Lett. Math. Phys. 4, 93 (1980)

    Article  ADS  MathSciNet  Google Scholar 

  21. Poh, H.S., Joshi, S.K., Cere, A., Cabello, A., Kurtsiefer, C.: Approaching Tsirelson’s bound in a photon pair experiment. Phys. Rev. Lett. 115, 180408 (2015)

    Article  ADS  Google Scholar 

  22. Ishizaka, S., Hiroshima, T.: Maximally entangled mixed states under nonlocal unitary operations in two qubits. Phys. Rev. A 62, 022310 (2000)

    Article  ADS  Google Scholar 

  23. Guhne, O., Toth, G.: Entanglement detection. Phys. Rep. 1, 474 (2009)

    MathSciNet  Google Scholar 

  24. Ganguly, N., Adhikari, S., Majumdar, A.S., Chatterjee, J.: Entanglement witness operator for quantum teleportation. Phys. Rev. Lett. 107, 270501 (2011)

    Article  Google Scholar 

  25. Nielsen, M.A., Chuang, I.L.: Quantum Computation and Quantum Inforation. Cambridge University Press, 10th anniversary edition published, ISBN:978-1-107-00217-3 (2010)

  26. Kraus, K.: States, effects and operations. In: Bonn, A. et al. (eds.) Lectures in Mathematical Physics. Springer, Berlin, ISBN: 3-540-12732-1 (1983)

  27. Bru\(\beta \), B., Machhiavello, C.: On the entanglement structure in quantum cloning. Found. Phys. 33, 1617 (2003)

  28. Ollivier, H., Zurek, W.H.: Quantum discord: a measure of the quantumness of correlations. Phys. Rev. Lett. 88, 017901 (2001)

    Article  ADS  MATH  Google Scholar 

  29. Sarandy, M.S.: Classical correlation and quantum discord in critical systems. Phy. Rev. A. 80, 022108 (2009)

    Article  ADS  Google Scholar 

  30. Wang, C.-Z., Li, C.-X., Nie, L.-Y., Li, J.-F.: Classical correlation and quantum discord mediated by cavity in two coupled qubits. J. Phys. B At. Mol. Opt. Phys. 44, 015503 (2011)

    Article  ADS  Google Scholar 

  31. Guo, Y.-n, Fang, M.-f, Wang, G.-y, Zeng, K.: Generation and Protection of Steady-State Quantum Correlations Due to Quantum Channels with Memory. arXiv:1603.06676 [quant-ph] (2016)

  32. Ali, M., Rau, A.R.P., Alber, G.: Quantum discord for two-qubit X states. Phys. Rev. A. 81, 042105 (2010)

    Article  ADS  Google Scholar 

  33. Huang, Y.: Quantum discord for two-qubit X states: analytical formula with very small worst-case error. Phys. Rev. A. 88, 014302 (2013); Private communication with Dr. Yiechen Huang

  34. Modi, K., Brodutch, A., Cable, H., Paterek, T., Vedral, V.: The classical-quantum boundary for correlations: discord and related measures. Rev. Mod. Phys. 84, 1655 (2012)

    Article  ADS  Google Scholar 

  35. Ghosh, B., Majumdar, A.S., Nayak, N.: Environment assisted entanglement enhancement. Phys. Rev. A. 74, 052315 (2006)

    Article  ADS  Google Scholar 

  36. Ghosh, B., Majumdar, A.S., Nayak, N.: Effects of cavity-field statistics in atomic entanglement in the Jaynes–Cumming model. Int. J. Quantum Inf. 5, 169 (2007)

    Article  MATH  Google Scholar 

  37. Datta, A., Ghosh, B., Majumdar, A.S., Nayak, N.: Information transfer through a one-atom micromaser. Euro. Phys. Lett. 67, 934 (2004)

    Article  ADS  Google Scholar 

Download references

Acknowledgements

The authors S. Roy and B. Ghosh acknowledge co-authors from their earlier paper of Ref. [1]. The authors are also grateful to Dr. Y. Huang of Institute for Quantum Information and Matter, California Institute of Technology for his valuable comments, through private communication, on quantum discord. The authors are also acknowledge Dr. Md. Manirul Ali of Physics division of National Center for Theoretical Sciences, Hsinchu, Taiwan.

Author information

Authors and Affiliations

  1. Department of Mathematics, Techno India, EM 4/1, Salt Lake, Kolkata, 91, India

    Sovik Roy

  2. S. N. Bose National Centre for Basic Sciences, Salt Lake, Kolkata, 98, India

    Sovik Roy

  3. Department of Physics, Vivekananda College for Women, Barisha, Kolkata, 8, India

    Biplab Ghosh

Authors
  1. Sovik Roy
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Biplab Ghosh
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Sovik Roy.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Roy, S., Ghosh, B. A revisit to non-maximally entangled mixed states: teleportation witness, noisy channel and discord. Quantum Inf Process 16, 108 (2017). https://doi.org/10.1007/s11128-017-1557-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11128-017-1557-3

Keywords