Abstract
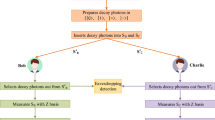
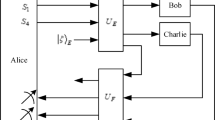
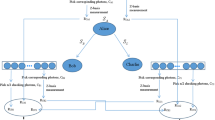
This paper proposes a multi-party semi-quantum secret sharing (MSQSS) protocol which allows a quantum party (manager) to share a secret among several classical parties (agents) based on GHZ-like states. By utilizing the special properties of GHZ-like states, the proposed scheme can easily detect outside eavesdropping attacks and has the highest qubit efficiency among the existing MSQSS protocols. Then, we illustrate an efficient way to convert the proposed MSQSS protocol into a multi-party semi-quantum key distribution (MSQKD) protocol. The proposed approach is even useful to convert all the existing measure–resend type of semi-quantum secret sharing protocols into semi-quantum key distribution protocols.






Similar content being viewed by others
Explore related subjects
Discover the latest articles, news and stories from top researchers in related subjects.References
Hillery, M., Bužek, V., Berthiaume, A.: Quantum secret sharing. Phys. Rev. A 59(3), 1829–1834 (1999)
Guo, G.P., Guo, G.C.: Quantum secret sharing without entanglement. Phys. Lett. A 310(4), 247–251 (2003)
Li, Y., Zhang, K., Peng, K.: Multiparty secret sharing of quantum information based on entanglement swapping. Phys. Lett. A 324(5–6), 420–424 (2004)
Deng, F.G., Long, G.L., Zhou, H.Y.: An efficient quantum secret sharing scheme with Einstein–Podolsky–Rosen pairs. Phys. Lett. A 340(1–4), 43–50 (2005)
Hsu, L.Y., Li, C.M.: Quantum secret sharing using product states. Phys. Rev. A 71(2), 022321 (2005)
Zhang, Z.J., Man, Z.X.: Multiparty quantum secret sharing of classical messages based on entanglement swapping. Phys. Rev. A 72(2), 022303 (2005)
Deng, F.G., Li, X.H., Zhou, H.Y.: Efficient high-capacity quantum secret sharing with two-photon entanglement. Phys. Lett. A 372(12), 1957–1962 (2008)
Gu, B., Li, C.Q., Xu, F., Chen, Y.L.: High-capacity three-party quantum secret sharing with superdense coding. Chin. Phys. B 18(11), 4690–4694 (2009)
Sun, Y., Wen, Q.Y., Gao, F., Chen, X.B., Zhu, F.C.: Multiparty quantum secret sharing based on Bell measurement. Opt. Commun. 282(17), 3647–3651 (2009)
Gu, B., Mu, L.L., Ding, L.G., Zhang, C.Y., Li, C.Q.: Fault tolerant three-party quantum secret sharing against collective noise. Opt. Commun. 283(15), 3099–3103 (2010)
Hsieh, C.R., Tasi, C.W., Hwang, T.: Quantum secret sharing using GHZ-like state. Commun. Theor. Phys. 54(6), 1019–1022 (2010)
Shi, R.H., Huang, L.S., Yang, W., Zhong, H.: Multiparty quantum secret sharing with Bell states and Bell measurements. Opt. Commun. 283(11), 2476–2480 (2010)
Hwang, T., Hwang, C.C., Li, C.M.: Multiparty quantum secret sharing based on GHZ states. Phys. Scr. 83(4), 045004 (2011)
Lin, J., Hwang, T.: An enhancement on Shi et al.’s multiparty quantum secret sharing protocol. Opt. Commun. 284(5), 1468–1471 (2011)
Boyer, M., Kenigsberg, D., Mor, T.: Quantum key distribution with classical Bob. Phys. Rev. Lett. 99(14), 140501 (2007)
Bennett, C.H., Brassard, G.: Quantum cryptography: public key distribution and coin tossing. In: Proceedings of the IEEE International Conference on Computers, Systems and Signal Processing, pp. 175–179. IEEE Press, Bangalore (1984)
Boyer, M., Gelles, R., Kenigsberg, D., Mor, T.: Semiquantum key distribution. Phys. Rev. A 79(3), 032341 (2009)
Zou, X., Qiu, D., Li, L., Wu, L., Li, L.: Semiquantum-key distribution using less than four quantum states. Phys. Rev. A 79(5), 052312 (2009)
Xian-Zhou, Z., Wei-Gui, G., Yong-Gang, T., Zhen-Zhong, R., Xiao-Tian, G.: Quantum key distribution series network protocol with M-classical Bobs. Chin. Phys. B 18(6), 2143 (2009)
Jian, W., Sheng, Z., Quan, Z., Chao-Jing, T.: Semiquantum key distribution using entangled states. Chin. Phys. Lett. 28(10), 100301 (2011)
Yu, K.F., Yang, C.W., Liao, C.H., Hwang, T.: Authenticated semi-quantum key distribution protocol using Bell states. Quantum Inf. Process. 13(6), 1457–1465 (2014)
Wang, J., Zhang, S., Zhang, Q., Tang, C.-J.: Semiquantum secret sharing using two-particle entangled state. Int. J. Quantum Inf. 10(05), 1250050 (2012)
Li, L., Qiu, D., Mateus, P.: Quantum secret sharing with classical Bobs. J. Phys. A Math. Theor. 46(4), 045304 (2013)
Li, Q., Chan, W., Long, D.-Y.: Semiquantum secret sharing using entangled states. Phys. Rev. A 82(2), 022303 (2010)
Yang, C.-W., Hwang, T.: Efficient key construction on semi-quantum secret sharing protocols. Int. J. Quantum Inf. 11(05), 1350052 (2013)
Yang, K., Huang, L.S., Yang, W., Song, F.: Quantum teleportation via GHZ-like state. Int. J. Theor. Phys. 48(2), 516–521 (2009)
Tsai, C.W., Hwang, T.: Teleportation of a pure EPR state via GHZ-like state. Int. J. Theor. Phys. 49(8), 1969–1975 (2010)
Tsai, C.W., Hwang, T.: Entanglement swapping of a GHZ state via a GHZ-like state. Phys. Scr. 84(4), 045006 (2011)
Hung, S.-M., Hwang, S.-L., Hwang, T., Kao, S.-H.: Multiparty quantum private comparison with almost dishonest third parties for strangers. Quantum Inf. Process. 16(2), 36 (2017)
Deutsch, D., Ekert, A., Jozsa, R., Macchiavello, C., Popescu, S., Sanpera, A.: Quantum privacy amplification and the security of quantum cryptography over noisy channels. Phys. Rev. Lett. 77(13), 2818 (1996)
Yang, C.-W., Hwang, T., Luo, Y.-P.: Enhancement on “quantum blind signature based on two-state vector formalism”. Quantum Inf. Process. 12(1), 109–117 (2013)
Yang, C.-W., Hwang, T.: Quantum dialogue protocols immune to collective noise. Quantum Inf. Process. 12(6), 2131–2142 (2013)
Yang, C.-W., Hwang, T.: Improved QSDC protocol over a collective-dephasing noise channel. Int. J. Theor. Phys. 51(12), 3941–3950 (2012)
Xie, C., Li, L., Qiu, D.: A novel semi-quantum secret sharing scheme of specific bits. Int. J. Theor. Phys. 54(10), 3819–3824 (2015)
Deng, F.G., Zhou, P., Li, X.H., Li, C.Y., Zhou, H.Y.: Robustness of two-way quantum communication protocols against Trojan horse attack. Quantum Phys. (2005). arXiv:quant-ph/0508168
Cai, Q.Y.: Eavesdropping on the two-way quantum communication protocols with invisible photons. Phys. Lett. A 351(1–2), 23–25 (2006)
Yang, C.W., Hwang, T., Luo, Y.P.: Enhancement on quantum blind signature based on two-state vector formalism. Quantum Inf. Process. 12(1), 109–117 (2013)
Deng, F.G., Li, X.H., Zhou, H.Y., Zhang, Z.J.: Improving the security of multiparty quantum secret sharing against Trojan horse attack. Phys. Rev. A 72(4), 044302 (2005)
Li, X.H., Deng, F.G., Zhou, H.Y.: Improving the security of secure direct communication based on the secret transmitting order of particles. Phys. Rev. A 74(5), 054302 (2006)
Acknowledgements
We would like to thank the Ministry of Science and Technology of the Republic of China, Taiwan, for partially supporting this research in finance under the Contract No. MOST 105-2221-E-006 -162 -MY2 .
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Yu, KF., Gu, J., Hwang, T. et al. Multi-party semi-quantum key distribution-convertible multi-party semi-quantum secret sharing. Quantum Inf Process 16, 194 (2017). https://doi.org/10.1007/s11128-017-1631-x
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s11128-017-1631-x