Skip to main content

Advertisement

Springer Nature Link
Log in

Spin coherent states phenomena probed by quantum state tomography in Zeeman perturbed nuclear quadrupole resonance

  • Published:
Quantum Information Processing Aims and scope Submit manuscript

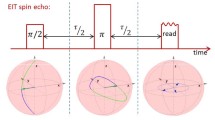
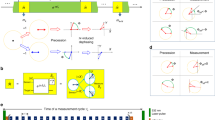
Abstract

Recently, we reported an experimental implementation of quantum information processing (QIP) by nuclear quadrupole resonance (NQR). In this work, we present the first quantum state tomography (QST) experimental implementation in the NQR QIP context. Two approaches are proposed, employing coherence selection by temporal and spatial averaging. Conditions for reduction in the number of cycling steps are analyzed, which can be helpful for larger spin systems. The QST method was applied to the study of spin coherent states, where the alignment-to-orientation phenomenon and the evolution of squeezed spin states show the effect of the nonlinear quadrupole interaction intrinsic to the NQR system. The quantum operations were implemented using a single-crystal sample of KClO\(_{3}\) and observing \(^{35}\)Cl nuclei, which posses spin 3/2.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

Notes

  1. In this paper we use spin angular momentum notation I, since their atomic counterpart satisfy the same mathematical properties.

References

  1. Agarwal, G.S.: Relation between atomic coherent-state representation, state multipoles, and generalized phase-space distributions. Phys. Rev. A 24, 2889–2896 (1981). https://doi.org/10.1103/PhysRevA.24.2889

    Article  ADS  MathSciNet  Google Scholar 

  2. Alnis, J., Auzinsh, M.: Angular-momentum spatial distribution symmetry breaking in Rb by an external magnetic field. Phys. Rev. A 63, 023,407 (2001). https://doi.org/10.1103/PhysRevA.63.023407

    Article  Google Scholar 

  3. Amiet, J.P., Weigert, S.: Reconstructing a pure state of a spin \(s\) through three Stern–Gerlach measurements. J. Phys. A Math. Gen. 32(15), 2777–2784 (1999). https://doi.org/10.1088/0305-4470/32/15/006

    Article  ADS  MathSciNet  MATH  Google Scholar 

  4. Araujo-Ferreira, A.G., Brasil, C.A., Soares-Pinto, D.O., Deazevedo, E.R., Bonagamba, T.J., Teles, J.: Quantum state tomography and quantum logical operations in a three qubits NMR quadrupolar system. Int. J. Quantum Inf. 10(02), 1250,016 (2012). https://doi.org/10.1142/s0219749912500165

    Article  MATH  Google Scholar 

  5. Auccaise, R., Teles, J., Sarthour, R., Bonagamba, T., Oliveira, I., deAzevedo, E.: A study of the relaxation dynamics in a quadrupolar NMR system using quantum state tomography. J. Magn. Reson. 192(1), 17–26 (2008). https://doi.org/10.1016/j.jmr.2008.01.009. http://www.sciencedirect.com/science/article/B6WJX-4RPTJ8M-1/2/a2ff51926c5e3feb296e0b09c8f3bbfe

  6. Auccaise, R., Araujo-Ferreira, A.G., Sarthour, R.S., Oliveira, I.S., Bonagamba, T.J., Roditi, I.: Spin squeezing in a quadrupolar nuclei NMR system. Phys. Rev. Lett. 114, 043,604 (2015). https://doi.org/10.1103/PhysRevLett.114.043604

    Article  Google Scholar 

  7. Auzinsh, M.P., Ferber, R.S.: J-selective stark orientation of molecular rotation in a beam. Phys. Rev. Lett. 69, 3463–3466 (1992). https://doi.org/10.1103/PhysRevLett.69.3463

    Article  ADS  Google Scholar 

  8. Auzinsh, M., Blushs, K., Ferber, R., Gahbauer, F., Jarmola, A., Tamanis, M.: Electric-field-induced symmetry breaking of angular momentum distribution in atoms. Phys. Rev. Lett. 97, 043,002 (2006). https://doi.org/10.1103/PhysRevLett.97.043002

    Article  Google Scholar 

  9. Auzinsh, M., Budker, D., Rochester, S.M.: Optically Polarized Atoms, 1st edn. Oxford University Press, Oxford (2010)

    MATH  Google Scholar 

  10. Auzinsh, M., Berzins, A., Ferber, R., Gahbauer, F., Kalvans, L., Mozers, A., Spiss, A.: Alignment-to-orientation conversion in a magnetic field at nonlinear excitation of the \({D}_{2}\) line of rubidium: experiment and theory. Phys. Rev. A 91, 053,418 (2015). https://doi.org/10.1103/PhysRevA.91.053418

    Article  Google Scholar 

  11. Bain, A.D.: Coherence levels and coherence pathways in NMR. A simple way to design phase cycling procedures. J. Mag. Reson. (1969) 56(3), 418–427 (1984). https://doi.org/10.1016/0022-2364(84)90305-6

    Article  Google Scholar 

  12. Barends, R., Kelly, J., Megrant, A., Veitia, A., Sank, D., Jeffrey, E., White, T.C., Mutus, J., Fowler, A.G., Campbell, B., Chen, Y., Chen, Z., Chiaro, B., Dunsworth, A., Neill, C., O’Malley, P., Roushan, P., Vainsencher, A., Wenner, J., Korotkov, A.N., Cleland, A.N., Martinis, J.M.: Superconducting quantum circuits at the surface code threshold for fault tolerance. Nature 508(7497), 500–503 (2014). https://doi.org/10.1038/nature13171

    Article  ADS  Google Scholar 

  13. Bax, A., Jong, P.D., Mehlkopf, A., Smidt, J.: Separation of the different orders of NMR multiple-quantum transitions by the use of pulsed field gradients. Chem. Phys. Lett. 69(3), 567–570 (1980)

    Article  ADS  Google Scholar 

  14. Benedict, M.G., Czirják, A.: Wigner functions, squeezing properties, and slow decoherence of a mesoscopic superposition of two-level atoms. Phys. Rev. A 60, 4034–4044 (1999). https://doi.org/10.1103/PhysRevA.60.4034

    Article  ADS  Google Scholar 

  15. Bonk, F.A., Sarthour, R.S., deAzevedo, E.R., Bulnes, J.D., Mantovani, G.L., Freitas, J.C.C., Bonagamba, T.J., Guimarães, A.P., Oliveira, I.S.: Quantum-state tomography for quadrupole nuclei and its application on a two-qubit system. Phys. Rev. A 69(4), 042,322 (2004). https://doi.org/10.1103/PhysRevA.69.042322

    Article  Google Scholar 

  16. Budker, D., Kimball, D., Rochester, S., Urban, J.: Alignment-to-orientation conversion and nuclear quadrupole resonance. Chem. Phys. Lett. 378(34), 440–448 (2003). https://doi.org/10.1016/S0009-2614(03)01327-7. http://www.sciencedirect.com/science/article/pii/S0009261403013277

  17. Budker, D., Kimball, D.F., Rochester, S.M., Yashchuk, V.V.: Nonlinear magneto-optical rotation via alignment-to-orientation conversion. Phys. Rev. Lett. 85, 2088–2091 (2000). https://doi.org/10.1103/PhysRevLett.85.2088

    Article  ADS  Google Scholar 

  18. Bulutay, C.: Cat-state generation and stabilization for a nuclear spin through electric quadrupole interaction. Phys. Rev. A (2017). https://doi.org/10.1103/physreva.96.012312

    Google Scholar 

  19. Childs, A.M., Chuang, I.L., Leung, D.W.: Realization of quantum process tomography in NMR. Phys. Rev. A (2001). https://doi.org/10.1103/physreva.64.012314

    Google Scholar 

  20. Chuang, I.L., Gershenfeld, N., Kubinec, M.G., Leung, D.W.: Bulk quantum computation with nuclear magnetic resonance: theory and experiment. Proc. R. Soc. A Math. Phys. Eng. Sci. 454(1969), 447–467 (1998). https://doi.org/10.1098/rspa.1998.0170

    Article  ADS  MATH  Google Scholar 

  21. Cory, D.G., Fahmy, A.F., Havel, T.F.: Ensemble quantum computing by NMR spectroscopy. Proceedings of the National Academy of Sciences of the United States of America 94(5), 1634–1639 (1997). https://doi.org/10.1073/pnas.94.5.1634. http://www.pnas.org/cgi/content/abstract/94/5/1634

  22. Cory, D.G., Price, M.D., Havel, T.F.: Nuclear magnetic resonance spectroscopy: an experimentally accessible paradigm for quantum computing. Phys. D Nonlinear Phenom. 120(1–2), 82–101 (1998). https://doi.org/10.1016/s0167-2789(98)00046-3

    Article  ADS  Google Scholar 

  23. da Silva, M.P., Landon-Cardinal, O., Poulin, D.: Practical characterization of quantum devices without tomography. Phys. Rev. Lett (2011). https://doi.org/10.1103/physrevlett.107.210404

    Google Scholar 

  24. Das, R., Kumar, A.: Use of quadrupolar nuclei for quantum-information processing by nuclear magnetic resonance: implementation of a quantum algorithm. Phys. Rev. A 68(3), 032,304 (2003). https://doi.org/10.1103/PhysRevA.68.032304

    Article  Google Scholar 

  25. Estrada, R.A., deAzevedo, E.R., Duzzioni, E.I., Bonagamba, T.J., Moussa, M.H.Y.: Spin coherent states in NMR quadrupolar system: experimental and theoretical applications. Eur. Phys. J. D 67(6), 127 (2013). https://doi.org/10.1140/epjd/e2013-30689-1

    Article  ADS  Google Scholar 

  26. Fano, U., Macek, J.H.: Impact excitation and polarization of the emitted light. Rev. Mod. Phys. 45, 553–573 (1973). https://doi.org/10.1103/RevModPhys.45.553

    Article  ADS  Google Scholar 

  27. Fortunato, E.M., Pravia, M.A., Boulant, N., Teklemariam, G., Havel, T.F., Cory, D.G.: Design of strongly modulating pulses to implement precise effective Hamiltonians for quantum information processing. J. Chem. Phys. 116(17), 7599–7606 (2002)

    Article  ADS  Google Scholar 

  28. Fraval, E., Sellars, M.J., Longdell, J.J.: Dynamic decoherence control of a solid-state nuclear-quadrupole qubit. Phys. Rev. Lett. (2005). https://doi.org/10.1103/physrevlett.95.030506

    Google Scholar 

  29. Furman, G.B., Goren, S.D., Meerovich, V.M., Sokolovsky, V.L.: Two qubits in pure nuclear quadrupole resonance. J. Phys. Condens. Matter 14(37), 8715 (2002). http://stacks.iop.org/0953-8984/14/i=37/a=308

  30. Furman, G.B., Meerovich, V.M., Sokolovsky, V.L.: Single-spin entanglement. Quantum Inf. Process. 16(9), 206 (2017). https://doi.org/10.1007/s11128-017-1655-2

    Article  ADS  MathSciNet  MATH  Google Scholar 

  31. Gross, D., Liu, Y.K., Flammia, S.T., Becker, S., Eisert, J.: Quantum state tomography via compressed sensing. Phys. Rev. Lett. (2010). https://doi.org/10.1103/physrevlett.105.150401

    Google Scholar 

  32. Hilborn, R.C., Hunter, L.R., Johnson, K., Peck, S.K., Spencer, A., Watson, J.: Atomic barium and cesium alignment-to-orientation conversion in external electric and magnetic fields. Phys. Rev. A 50, 2467–2474 (1994). https://doi.org/10.1103/PhysRevA.50.2467

    Article  ADS  Google Scholar 

  33. Home, J.P., Hanneke, D., Jost, J.D., Amini, J.M., Leibfried, D., Wineland, D.J.: Complete methods set for scalable ion trap quantum information processing. Science 325(5945), 1227–1230 (2009). https://doi.org/10.1126/science.1177077

    Article  ADS  MathSciNet  MATH  Google Scholar 

  34. Howard, M., Twamley, J., Wittmann, C., Gaebel, T., Jelezko, F., Wrachtrup, J.: Quantum process tomography and Linblad estimation of a solid-state qubit. New J. Phys. 8(3), 33–33 (2006). https://doi.org/10.1088/1367-2630/8/3/033

    Article  ADS  Google Scholar 

  35. Jin, G.R., Kim, S.W.: Spin squeezing and maximal-squeezing time. Phys. Rev. A 76(4), 043,621 (2007). https://doi.org/10.1103/PhysRevA.76.043621

    Article  Google Scholar 

  36. Jin, G.R., Kim, S.W.: Storage of spin squeezing in a two-component Bose–Einstein condensate. Phys. Rev. Lett. 99(17), 170,405 (2007). https://doi.org/10.1103/PhysRevLett.99.170405

    Article  Google Scholar 

  37. Kampermann, H., Veeman, W.S.: Quantum computing using quadrupolar spins in solid state N M R. Quantum Inf. Proces. 1(5), 327–344 (2002)

    Article  MathSciNet  Google Scholar 

  38. Knill, E., Laflamme, R., Martinez, R., Negrevergne, C.: Benchmarking quantum computers: the five-qubit error correcting code. Phys. Rev. Lett. 86(25), 5811–5814 (2001). https://doi.org/10.1103/physrevlett.86.5811

    Article  ADS  Google Scholar 

  39. Kuntz, M.C., Hilborn, R.C., Spencer, A.M.: Dynamic stark shift and alignment-to-orientation conversion. Phys. Rev. A 65, 023,411 (2002). https://doi.org/10.1103/PhysRevA.65.023411

    Article  Google Scholar 

  40. Lauterbur, P.C.: Image formation by induced local interactions: examples employing nuclear magnetic resonance. Nature 242(5394), 190–191 (1973)

    Article  ADS  Google Scholar 

  41. Law, C.K., Ng, H.T., Leung, P.T.: Coherent control of spin squeezing. Phys. Rev. A 63(5), 055,601 (2001). https://doi.org/10.1103/PhysRevA.63.055601

    Article  Google Scholar 

  42. Leonhardt, U.: Quantum-state tomography and discrete Wigner function. Phys. Rev. Lett. 76(22), 4293–4293 (1996). https://doi.org/10.1103/physrevlett.76.4293

    Article  ADS  MathSciNet  Google Scholar 

  43. Leskowitz, G.M., Ghaderi, N., Olsen, R.A., Mueller, L.J.: Three-qubit nuclear magnetic resonance quantum information processing with a single-crystal solid. J. Chem. Phys. 119(3), 1643–1649 (2003). https://doi.org/10.1063/1.1582171

    Article  ADS  Google Scholar 

  44. Li, K., Zhang, J., Cong, S.: Fast reconstruction of high-qubit-number quantum states via low-rate measurements. Phys. Rev. A (2017). https://doi.org/10.1103/physreva.96.012334

    MathSciNet  Google Scholar 

  45. Liu, Y.X, Wei, L.F., Nori, F.: Tomographic measurements on superconducting qubit states. Phys. Rev. B (2005). https://doi.org/10.1103/physrevb.72.014547

  46. Mallet, F., Castellanos-Beltran, M.A., Ku, H.S., Glancy, S., Knill, E., Irwin, K.D., Hilton, G.C., Vale, L.R., Lehnert, K.W.: Quantum state tomography of an itinerant squeezed microwave field. Phys. Rev. Lett. (2011). https://doi.org/10.1103/physrevlett.106.220502

    Google Scholar 

  47. Mansfield, P., Grannell, P.K.: NMR ’diffraction’ in solids? J. Phys. C Solid State Phys. 6(22), L422–L426 (1973)

    Article  ADS  Google Scholar 

  48. Miranowicz, A., zdemir, Ş.K., Bajer, J., Yusa, G., Imoto, N., Hirayama, Y., Nori, F.: Quantum state tomography of large nuclear spins in a semiconductor quantum well: Optimal robustness against errors as quantified by condition numbers. Phys. Rev. B (2015). https://doi.org/10.1103/physrevb.92.075312

  49. Myrskog, S.H., Fox, J.K., Mitchell, M.W., Steinberg, A.M.: Quantum process tomography on vibrational states of atoms in an optical lattice. Phys. Rev. A (2005). https://doi.org/10.1103/physreva.72.013615

    Google Scholar 

  50. Ofek, N., Petrenko, A., Heeres, R., Reinhold, P., Leghtas, Z., Vlastakis, B., Liu, Y., Frunzio, L., Girvin, S.M., Jiang, L., Mirrahimi, M., Devoret, M.H., Schoelkopf, R.J.: Extending the lifetime of a quantum bit with error correction in superconducting circuits. Nature 536(7617), 441–445 (2016). https://doi.org/10.1038/nature18949

    Article  ADS  Google Scholar 

  51. Oren, D., Mutzafi, M., Eldar, Y.C., Segev, M.: Quantum state tomography with a single measurement setup. Optica 4(8), 993 (2017). https://doi.org/10.1364/optica.4.000993

    Article  Google Scholar 

  52. Possa, D., Gaudio, A.C., Freitas, J.C.: Numerical simulation of NQR/NMR: Applications in quantum computing. J. Mag. Reson. 209(2), 250–260 (2011). https://doi.org/10.1016/j.jmr.2011.01.020. http://www.sciencedirect.com/science/article/pii/S109078071100036X

  53. Price, W.S.: Pulsed-field gradient nuclear magnetic resonance as a tool for studying translational diffusion: part 1. Basic theory. Concepts Mag. Reson. 9(5), 299–336 (1997)

    Article  Google Scholar 

  54. Raymer, M.G.: Measuring the quantum mechanical wave function. Contemp. Phys. 38(5), 343–355 (1997). https://doi.org/10.1080/001075197182315

    Article  ADS  Google Scholar 

  55. Redfield, A.G.: On the theory of relaxation processes. IBM J. Res. Dev. 1(1), 19–31 (1957). https://doi.org/10.1147/rd.11.0019

    Article  Google Scholar 

  56. Riebe, M., Kim, K., Schindler, P., Monz, T., Schmidt, P.O., Krber, T.K., Hnsel, W., Hffner, H., Roos, C.F., Blatt, R.: Process tomography of ion trap quantum gates. Phys. Rev. Lett. (2006). https://doi.org/10.1103/physrevlett.97.220407

    Google Scholar 

  57. Rochester, S.M., Ledbetter, M.P., Zigdon, T., Wilson-Gordon, A.D., Budker, D.: Orientation-to-alignment conversion and spin squeezing. Phys. Rev. A 85, 022,125 (2012). https://doi.org/10.1103/PhysRevA.85.022125

    Article  Google Scholar 

  58. Sánchez-Soto, L.L., Klimov, A.B., de la Hoz, P., Leuchs, G.: Quantum versus classical polarization states: when multipoles count. J. Phys. B Atomic, Mol. Opt. Phys. 46(10), 104,011 (2013). http://stacks.iop.org/0953-4075/46/i=10/a=104011

  59. Santagati, R., Silverstone, J.W., Strain, M., Sorel, M., Miki, S., Yamashita, T., Fujiwara, M., Sasaki, M., Terai, H., Tanner, M., Natarajan, C., Hadfield, R.H., Brien, J.O., Thompson, M.: Silicon photonic processor of two-qubit entangling quantum logic. J. Opt. (2017). https://doi.org/10.1088/2040-8986/aa8d56

    Google Scholar 

  60. Sarthour, R.S., deAzevedo, E.R., Bonk, F.A., Vidoto, E.L.G., Bonagamba, T.J., Guimarães, A.P., Freitas, J.C.C., Oliveira, I.S.: Relaxation of coherent states in a two-qubit NMR quadrupole system. Phys. Rev. A 68(2), 022,311 (2003). https://doi.org/10.1103/PhysRevA.68.022311

    Article  Google Scholar 

  61. Schindler, P., Barreiro, J.T., Monz, T., Nebendahl, V., Nigg, D., Chwalla, M., Hennrich, M., Blatt, R.: Experimental repetitive quantum error correction. Science 332(6033), 1059–1061 (2011). https://doi.org/10.1126/science.1203329

    Article  ADS  Google Scholar 

  62. Teles, J., deAzevedo, E.R., Auccaise, R., Sarthour, R.S., Oliveira, I.S., Bonagamba, T.J.: Quantum state tomography for quadrupolar nuclei using global rotations of the spin system. J. Chem. Phys. 126(15), 154506 (2007). https://doi.org/10.1063/1.2717179. http://link.aip.org/link/?JCP/126/154506/1

  63. Teles, J., Rivera-Ascona, C., Polli, R.S., Oliveira-Silva, R., Vidoto, E.L.G., Andreeta, J.P., Bonagamba, T.J.: Experimental implementation of quantum information processing by Zeeman-perturbed nuclear quadrupole resonance. Quantum Inf. Proces. 14(6), 1889–1906 (2015). https://doi.org/10.1007/s11128-015-0967-3

    Article  ADS  MATH  Google Scholar 

  64. Varshalovich, D.: Quantum Theory Of Angular Momemtum. World Scientific, Singapore (1988)

    Book  Google Scholar 

  65. Weber, M.J.: Nuclear quadrupole spin-lattice relaxation in solids. J. Phys. Chem. Solids 17(3–4), 267–277 (2015). https://doi.org/10.1016/0022-3697(61)90192-5

    ADS  Google Scholar 

  66. Yamamoto, T., Neeley, M., Lucero, E., Bialczak, R.C., Kelly, J., Lenander, M., Mariantoni, M., O’Connell, A.D., Sank, D., Wang, H., Weides, M., Wenner, J., Yin, Y., Cleland, A.N., Martinis, J.M.: Quantum process tomography of two-qubit controlled-z and controlled-NOT gates using superconducting phase qubits. Phys. Rev. B 82(18), (2010). https://doi.org/10.1103/physrevb.82.184515

  67. Zhang, J., Laflamme, R., Suter, D.: Experimental implementation of encoded logical qubit operations in a perfect quantum error correcting code. Phys. Rev. Lett. (2012). https://doi.org/10.1103/physrevlett.109.100503

    Google Scholar 

Download references

Acknowledgements

This work was supported by the Brazilian agency FAPESP (2012/02208-5) and by the Brazilian National Institute of Science and Technology for Quantum Information (INCT-IQ). The authors also acknowledge Edson Luiz Gea Vidoto and Aparecido Donizeti Fernandes de Amorim by the technical support.

Author information

Authors and Affiliations

  1. Departamento de Ciências da Natureza, Matemática e Educação, Universidade Federal de São Carlos, Caixa Postal 153, 13600-970, Araras, SP, Brasil

    João Teles

  2. Departamento de Física, Universidade Estadual de Ponta Grossa, Av. Carlos Cavalcanti, 4748, Ponta Grossa, PR, 84030-900, Brasil

    Ruben Auccaise

  3. Instituto de Física de São Carlos, Universidade de São Paulo, CP 369, São Carlos, SP, 13560-970, Brasil

    Christian Rivera-Ascona, Arthur G. Araujo-Ferreira, José P. Andreeta & Tito J. Bonagamba

Authors
  1. João Teles
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Ruben Auccaise
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Christian Rivera-Ascona
    View author publications

    You can also search for this author inPubMed Google Scholar

  4. Arthur G. Araujo-Ferreira
    View author publications

    You can also search for this author inPubMed Google Scholar

  5. José P. Andreeta
    View author publications

    You can also search for this author inPubMed Google Scholar

  6. Tito J. Bonagamba
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to João Teles.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Teles, J., Auccaise, R., Rivera-Ascona, C. et al. Spin coherent states phenomena probed by quantum state tomography in Zeeman perturbed nuclear quadrupole resonance. Quantum Inf Process 17, 177 (2018). https://doi.org/10.1007/s11128-018-1947-1

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11128-018-1947-1

Keywords