Thermal quantum correlation and entanglement in the Bose–Hubbard Hamiltonian

Abstract

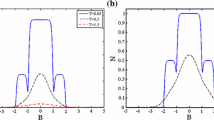
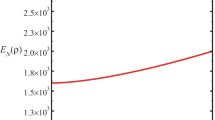
We study a two-qutrit system which is described by the Bose–Hubbard Hamiltonian with two external magnetic fields. The entanglement (through the negativity) and quantum correlation (through the geometric discord) between the qutrits are calculated as functions of the magnetic field (B), the temperature (T), the linear and nonlinear coupling constants among two spins (J and K). Then, we compare the effect of these parameters on entanglement and quantum correlation of this system. For some values of system parameters, we show that the negativity is zero while, the geometric discord is nonzero. Moreover, we investigate the effect of finite external magnetic fields direction on these measures. This study leads to some new and interesting results as well.

Appendix

Unlike the case of parallel magnetic fields, for antiparallel case we cannot write eigenstates and eigenvalues in explicit forms. Hence we derive them in two special cases as $K<<J$ and $K>>J$.

The eigenvalues in the case of $K<<J$ are

$$\begin{aligned} E_1= & {} - \frac{1}{6}\eta +\frac{3}{2}\delta -\frac{2}{3}J -\frac{1}{2}i\sqrt{3}\left( \frac{1}{3}\eta +3\delta \right) ,\nonumber \\ E_2= & {} - \frac{1}{6}\eta +\frac{3}{2}\delta -\frac{2}{3}J +\frac{1}{2}i\sqrt{3}\left( \frac{1}{3}\eta +3\delta \right) ,\nonumber \\ E_3= & {} \frac{1}{3}\eta -3\delta -\frac{2}{3}J,\nonumber \\ E_{4,5}= & {} - \sqrt{B^2+J^2},\nonumber \\ E_{6,7}= & {} \sqrt{B^2+J^2}\,\, \hbox { and}\nonumber \\ E_{8,9}= & {} J, \end{aligned}$$

(23)

where we have defined $\eta \equiv \bigg (-36\,{B}^{2}J+10\,{J}^{3}+ 3\,\sqrt{-192\,{B}^{6}-192\,{J}^{2}{B}^{4}- 276\,{J}^{4}{B}^{2}-27\,{J}^{6}}\bigg )^\frac{1}{3}$, $\delta \equiv \frac{-\frac{4}{3}B^2-\frac{7}{9}J^2}{\eta }$, while corresponding eigenstates are

$$\begin{aligned} {\vert }{\varphi _1}{\rangle }= & {} \frac{E_1^2+2BE_1+JE_1-J^2}{J^2}{\vert }{1,-1}{\rangle }\nonumber \\&\quad +\,\frac{JE_1+2B}{J}{\vert }{0,0}{\rangle }+{\vert }{-1,1}{\rangle },\nonumber \\ {\vert }{\varphi _2}{\rangle }= & {} \frac{E_2^2+2BE_2+JE_2-J^2}{J^2}{\vert }{1,-1}{\rangle }\nonumber \\&\quad +\,\frac{E_2+J+2B}{J}{\vert }{0,0}{\rangle }+{\vert }{-1,1}{\rangle },\nonumber \\ {\vert }{\varphi _3}{\rangle }= & {} \frac{E_3^2+2BE_3+JE_3-J^2}{J^2}{\vert }{1,-1}{\rangle }\nonumber \\&\quad +\,\frac{E_3+J+2B}{J}{\vert }{0,0}{\rangle }+{\vert }{-1,1}{\rangle },\nonumber \\ {\vert }{\varphi _4}{\rangle }= & {} \frac{E_{4,5}+B}{J}{\vert }{0,-1}{\rangle }+{\vert }{-1,0}{\rangle },\nonumber \\ {\vert }{\varphi _5}{\rangle }= & {} \frac{E_{4,5}+B}{J}{\vert }{1,0}{\rangle }+{\vert }{0,1}{\rangle },\nonumber \\ {\vert }{\varphi _6}{\rangle }= & {} \frac{E_{6,7}+B}{J}{\vert }{0,-1}{\rangle }+{\vert }{-1,0}{\rangle },\nonumber \\ {\vert }{\varphi _7}{\rangle }= & {} \frac{E_{6,7}+B}{J}{\vert }{1,0}{\rangle }+{\vert }{0,1}{\rangle },\nonumber \\ {\vert }{\varphi _8}{\rangle }= & {} {\vert }{-1,-1}{\rangle }\quad \hbox {and} \nonumber \\ {\vert }{\varphi _9}{\rangle }= & {} {\vert }{1,1}{\rangle }. \end{aligned}$$

(24)

The eigenvalues in the case of $K>>J$ are

$$\begin{aligned} E_{1,2}= & {} K+B,\nonumber \\ E_{3,4}= & {} -B+K,\nonumber \\ E_5= & {} \frac{1}{3}\mu -3 \nu +2 K,\nonumber \\ E_{6}= & {} -\,\frac{1}{6}\mu +\frac{3}{2}\nu +2K +\frac{1}{2}i\sqrt{3}\left( \frac{1}{3}\mu +3\nu \right) ,\nonumber \\ E_{7}= & {} -\,\frac{1}{6}\mu +\frac{3}{2}\nu +2K-\frac{1}{2}i\sqrt{3}\left( \frac{1}{3}\mu +3\nu \right) \,\,\hbox {and} \nonumber \\ E_{8,9}= & {} K. \end{aligned}$$

(25)

where $\mu \equiv (27K^3+6\sqrt{-48B^6-108K^2B^4-81K^4B^2})^\frac{1}{3}$, $\nu \equiv -\frac{\frac{4}{3}B^2+K^2}{\mu }$ and the corresponding eigenstates are

$$\begin{aligned} {\vert }{\varphi _1}{\rangle }= & {} {\vert }{1,0}{\rangle },\nonumber \\ {\vert }{\varphi _2}{\rangle }= & {} {\vert }{0,-1}{\rangle },\nonumber \\ {\vert }{\varphi _3}{\rangle }= & {} {\vert }{0,1}{\rangle },\nonumber \\ {\vert }{\varphi _4}{\rangle }= & {} {\vert }{-1,0}{\rangle },\nonumber \\ {\vert }{\varphi _5}{\rangle }= & {} -\,\frac{-2BE_5-E_5^2+5E_5K-4K^2+6BK}{2BK}{\vert }{1,-1}{\rangle }\nonumber \\&\quad -\,\frac{2BK-E_5^2+5E_5K+4B^2-4K^2}{2BK}{\vert }{0,0}{\rangle }+{\vert }{-1,1}{\rangle }, \nonumber \\ {\vert }{\varphi _6}{\rangle }= & {} -\,\frac{-2BE_6-E_6^2+5E_6K-4K^2+6BK}{2BK}{\vert }{1,-1}{\rangle }\nonumber \\&\quad -\,\frac{2BK-E_6^2+5E_6K+4B^2-4K^2}{2BK}{\vert }{0,0}{\rangle }+{\vert }{-1,1}{\rangle },\nonumber \\ {\vert }{\varphi _7}{\rangle }= & {} -\frac{-2BE_7-E_7^2+5E_7K-4K^2+6BK}{2BK}{\vert }{1,-1}{\rangle }\nonumber \\&\quad -\,\frac{2BK-E_7^2+5E_7K+4B^2-4K^2}{2BK}{\vert }{0,0}{\rangle }+{\vert }{-1,1}{\rangle }, \nonumber \\ {\vert }{\varphi _8}{\rangle }= & {} {\vert }{-1,-1}{\rangle }\,\,\hbox {and}\nonumber \\ {\vert }{\varphi _9}{\rangle }= & {} {\vert }{1,1}{\rangle }. \end{aligned}$$

(26)