Skip to main content

Advertisement

Springer Nature Link
Log in

Simulating Markovian quantum decoherence processes through an all-optical setup

  • Published:
Quantum Information Processing Aims and scope Submit manuscript

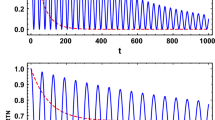
Abstract

We investigate through both theory and experiment quantum decoherence processes. By considering a qubit under the effect of Markovian channels, we analytically obtain expressions for the \(l_1\)-norm of coherence and for the corresponding maximal coherence measure in terms of the initial state and decoherence parameters. In particular, we examine under which conditions such quantum coherence quantifiers exhibit the eternal freezing phenomenon, and we find that this property is more common in maximal quantum coherence compared to quantum coherence. We implement an all-optical setup with an intense laser to perform the experimental simulation of the quantum channels, where the qubit was encoded in polarization degree of freedom and the environment was encoded in the propagation path.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

References

  1. Bagan, E., Bergou, J.A., Cottrell, S.S., Hillery, M.: Relations between coherence and path information. Phys. Rev. Lett. 116, 160406 (2016)

    ADS  Google Scholar 

  2. Jha, P.K., Mrejen, M., Kim, J., Wu, C., Wang, Y., Rostovtsev, Y.V., Zhang, X.: Coherence-driven topological transition in quantum metamaterials. Phys. Rev. Lett. 116, 165502 (2016)

    ADS  Google Scholar 

  3. Giovannetti, V., Lloyd, S., Maccone, L.: Quantum-enhanced measurements: beating the standard quantum limit. Science 306, 1330 (2004)

    ADS  Google Scholar 

  4. Demkowicz-Dobrzanski, R., Maccone, L.: Using entanglement against noise in quantum metrology. Phys. Rev. Lett. 113, 250801 (2014)

    ADS  Google Scholar 

  5. Giovannetti, V., Lloyd, S., Maccone, L.: Advances in quantum metrology. Nat. Photonics 5, 222 (2011)

    ADS  Google Scholar 

  6. Glauber, R.J.: Coherent and incoherent states of the radiation field. Phys. Rev. 131, 2766 (1963)

    ADS  MathSciNet  MATH  Google Scholar 

  7. Sudarshan, E.C.G.: Equivalence of semiclassical and quantum mechanical descriptions of statistical light beams. Phys. Rev. Lett. 10, 277 (1963)

    ADS  MathSciNet  MATH  Google Scholar 

  8. Mandel, L., Wolf, E.: Optical Coherence and Quantum Optics. Cambridge University Press, Cambridge (1995)

    Google Scholar 

  9. Streltsov, A., Adesso, G., Plenio, M.B.: Colloquium: Quantum coherence as a resource. Rev. Mod. Phys. 89, 041003 (2017)

    ADS  MathSciNet  Google Scholar 

  10. Huelga, S.F., Plenio, M.B.: Vibrations, quanta and biology. Contemp. Phys. 54, 181 (2013)

    ADS  Google Scholar 

  11. Baumgratz, T., Cramer, M., Plenio, M.: Quantifying coherence. Phys. Rev. Lett. 113, 140401 (2014)

    ADS  Google Scholar 

  12. Levi, F., Mintert, F.: A quantitative theory of coherent delocalization. New J. Phys. 16, 033007 (2014)

    ADS  Google Scholar 

  13. Girolami, D.: Observable measure of quantum coherence in finite dimensional systems. Phys. Rev. Lett. 113, 170401 (2014)

    ADS  Google Scholar 

  14. Smyth, C., Scholes, G.D.: Method of developing analytical multipartite delocalization measures for mixed-like states. Phys. Rev. A 90, 032312 (2014)

    ADS  Google Scholar 

  15. Streltsov, A., Kampermann, H., Wölk, S., Gessner, M., Bruß, D.: Maximal coherence and the resource theory of purity. New J. Phys. 20, 053058 (2018)

    ADS  MathSciNet  Google Scholar 

  16. Yu, C., Yang, S., Guo, B.: Total quantum coherence and its applications. Quantum Inf. Process. 15, 3773 (2016)

    ADS  MathSciNet  MATH  Google Scholar 

  17. Streltsov, A., Singh, U., Dhar, H.S., Bera, M.N., Adesso, G.: Measuring quantum coherence with entanglement. Phys. Rev. Lett. 115, 020403 (2015)

    ADS  MathSciNet  Google Scholar 

  18. Yao, Y., Xiao, X., Ge, L., Sun, C.P.: Quantum coherence in multipartite systems. Phys. Rev. A 92, 022112 (2015)

    ADS  Google Scholar 

  19. Bromley, T.R., Cianciaruso, M., Adesso, G.: Frozen quantum coherence. Phys. Rev. Lett. 114, 210401 (2015)

    ADS  Google Scholar 

  20. Silva, I.A., Souza, A.M., Bromley, T.R., Cianciaruso, M., Marx, R., Sarthour, R.S., Oliveira, I.S., Lo Franco, R., Glaser, S.J., de Azevedo, E.R., Soares-Pinto, D.O., Adesso, G.: Time-invariant coherence in a nuclear magnetic resonance quantum simulator. Phys. Rev. Lett. 117, 160402 (2016)

    ADS  Google Scholar 

  21. Yu, X.D., Zhang, D.J., Liu, C.L., Tong, D.M.: Measure-independent freezing of quantum coherence. Phys. Rev. A 93, 060303 (2016)

    ADS  Google Scholar 

  22. Yang, L.W., Han, W., Xia, Y.J.: Freezing quantum coherence with weak measurement. Chin. Opt. Lett. 15, 052701 (2017)

    ADS  Google Scholar 

  23. Yang, L.W., Man, Z.X., Zhang, Y.J., Han, F., Du, S.J., Xia, Y.J.: Preparation of freezing quantum state for quantum coherence. Quantum Inf. Process. 17, 127 (2018)

    ADS  MathSciNet  MATH  Google Scholar 

  24. Ming-Liang, H., Fan, H.: Evolution equation for quantum coherence. Sci. Rep. 6, 29260 (2016)

    ADS  Google Scholar 

  25. Passos, M.H.M., Obando, P.C., Balthazar, W.F., Paula, F.M., Huguenin, J.A.O., Sarandy, M.S.: Non-Markovianity through quantum coherence in an all-optical setup. Opt. Lett. 44, 2478–2481 (2019)

    ADS  Google Scholar 

  26. Maioli, A.C., Passos, M.H.M., Balthazar, W.F., Souza, C.E.R., Huguenin, J.A.O., Schmidt, A.G.M.: Quantization and experimental realization of the Colonel Blotto game. Quantum Inf. Process. 18, 1–20 (2018)

    MathSciNet  MATH  Google Scholar 

  27. Song, X., Sun, Y., Li, P., Qin, H., Zhang, X.: Bell’s measure and implementing quantum Fourier transform with orbital angular momentum of classical light. Sci. Rep. 5, 14113 (2015)

    ADS  Google Scholar 

  28. Dudley, A., Nock, M., Konrad, T., Filippus, R.S., Forbes, A.: Amplitude damping of Laguerre–Gaussian modes. Opt. Express 18, 22789–22795 (2010)

    ADS  Google Scholar 

  29. Walborn, S.P., de Oliveira, A.N., Monken, C.H.: Implementing the Deutsch algorithm with polarization and transverse spatial modes. J. Opt. B 7, 288 (2005)

    ADS  Google Scholar 

  30. Hor-Meyll, M., Tasca, D.S., Walborn, S.P., Ribeiro, P.H.S., Santos, M.M., Duzzioni, E.I.: Deterministic quantum computation with one photonic qubit. Phys. Rev. A 92, 012337 (2015)

    ADS  Google Scholar 

  31. Qing, L., Bing, H.: Single-photon logic gates using minimal resources. Phys. Rev. A 80, 042310 (2009)

    ADS  Google Scholar 

  32. Babazadeh, A., Erhard, M., Wang, F., Malik, M., Nouroozi, R., Krenn, M., Zeilinger, A.: High-dimensional single-photon quantum gates: concepts and experiments. Phys. Rev. Lett. 119, 180510 (2017)

    ADS  Google Scholar 

  33. D’Ambrosio, V., Nagali, E., Walborn, S.P., Aolita, L., Slussarenko, S., Marrucci, L., Sciarrino, F.: Complete experimental toolbox for alignment-free quantum communication. Nat. Commun. 3, 961 (2012)

    ADS  Google Scholar 

  34. Barreiro, J.T., Wei, T.C., Kwiat, P.G.: Remote preparation of single-photon “hybrid” entangled and vector-polarization states. Phys. Rev. Lett. 105, 030407 (2010)

    ADS  Google Scholar 

  35. Khoury, A.Z., Milman, P.: Quantum teleportation in the spin–orbit variables of photon pairs. Phys. Rev. A 83, 060301 (2011)

    ADS  Google Scholar 

  36. Salles, A., de Melo, F., Almeida, M.P., Hor-Meyll, M., Walborn, S.P., Souto Ribeiro, P.H., Davidovich, L.: Experimental investigation of the dynamics of entanglement: sudden death, complementarity, and continuous monitoring of the environment. Phys. Rev. A 78(2), 022322 (2008)

    ADS  Google Scholar 

  37. Souza, C.E.R., Huguenin, J.A.O., Milman, P., Khoury, A.Z.: Topological phase for spin–orbit transformations on a laser beam. Phys. Rev. Lett. 99, 160401 (2007)

    ADS  Google Scholar 

  38. Souza, C.E.R., Huguenin, J.A.O., Khoury, A.Z.: Topological phase structure of vector vortex beams. J. Opt. Soc. Am. A 31, 1007 (2014)

    ADS  Google Scholar 

  39. Borges, C.V.S., Hor-Meyll, M., Huguenin, J.A.O., Khoury, A.Z.: Bell-like inequality for the spin–orbit separability of a laser beam. Phys. Rev. A 82, 033833 (2010)

    ADS  Google Scholar 

  40. Qian, X.-F., Little, B., Howell, J.C., Eberly, J.H.: Shifting the quantum-classical boundary: theory and experiment for statistically classical optical fields. Optica 2, 611 (2015)

    ADS  Google Scholar 

  41. Balthazar, W.F., Souza, C.E.R., Caetano, D.P., Galvão, E.F., Huguenin, J.A.O., Khoury, A.Z.: Tripartite nonseparability in classical optics. Opt. Lett. 41, 5797 (2016)

    ADS  Google Scholar 

  42. Souza, C.E.R., Borges, C.V.S., Khoury, A.Z., Huguenin, J.A.O., Aolita, L., Walborn, S.P.: Quantum key distribution without a shared reference frame. Phys. Rev. A 77, 032345 (2008)

    ADS  MathSciNet  MATH  Google Scholar 

  43. da Silva, B.P., Leal, M.A., Souza, C.E.R., Galvão, E.F., Khoury, A.Z.: Spin–orbit laser mode transfer via a classical analogue of quantum teleportation. J. Phys. B 49, 055501 (2016)

    ADS  Google Scholar 

  44. Passos, M.H.M., Balthazar, W.F., Khoury, A.Z., Hor-Meyll, M., Davidovich, L., Huguenin, J.A.O.: Experimental investigation of environment-induced entanglement using an all-optical setup. Phys. Rev. A 97, 022321 (2018)

    ADS  Google Scholar 

  45. Souza, C.E.R., Khoury, A.Z.: A Michelson controlled-not gate with a single-lens astigmatic mode converter. Opt. Express 1, 9207 (2010)

    ADS  Google Scholar 

  46. Balthazar, W.F., Huguenin, J.A.O.: Conditional operation using three degrees of freedom of a laser beam for application in quantum information. J. Opt. Soc. Am. B 33, 1649 (2016)

    ADS  Google Scholar 

  47. Nielsen, M.A., Chuang, I.: Quantum Computation and Quantum Information. Cambridge University Press, Cambridge (2000)

    MATH  Google Scholar 

  48. Rana, S., Parashar, P., Lewenstein, M.: Trace-distance measure of coherence. Phys. Rev. A 93, 012110 (2016)

    ADS  MathSciNet  Google Scholar 

  49. Adesso, G., Bromley, T.R., Cianciaruso, M.: Measures and applications of quantum correlations. J. Phys. A Math. Theor. 49, 473001 (2016)

    ADS  MathSciNet  MATH  Google Scholar 

  50. Raimond, J.M., Haroche, S.: Monitoring the decoherence of mesoscopic quantum superpositions in a cavity. Prog. Math. Phys. 48, 33 (2006)

    MathSciNet  Google Scholar 

  51. Spreeuw, R.J.: a classical analogy of entanglement. Found. Phys. 28, 361 (1998)

    MathSciNet  Google Scholar 

  52. Xiao-Feng, Qian, Eberly, J.H.: Entanglement and classical polarization states. Opt. Lett. 36, 4110–4112 (2011)

    ADS  Google Scholar 

  53. Pereira, L.J., Khoury, A.Z., Dechoum, K.: Quantum and classical separability of spin–orbit laser modes. Phys. Rev. A. 90, 053842 (2014)

    ADS  Google Scholar 

  54. Altepeter, J.B., Jeffrey, E.R., Kwiat, P.G.: Photonic state tomography. Adv. At. Mol. Opt. Phys. 52, 105 (2005)

    ADS  Google Scholar 

  55. Lidar, D.A., Chuang, I.L., Whaley, K.B.: Decoherence-free subspaces for quantum computation. Phys. Rev. Lett. 81, 2594 (1998)

    ADS  Google Scholar 

  56. Kwiat, P.G., Berglund, A.J., Altepeter, J.B., White, A.G.: Experimental verification of decoherence-free subspaces. Science 290, 498 (2000)

    ADS  Google Scholar 

  57. Lidar, D.A., Bacon, D., Kempe, J., Whaley, K.B.: Decoherence-free subspaces for multiple-qubit errors. II. Universal, fault-tolerant quantum computation. Phys. Rev. A 63, 022307 (2001)

    ADS  MathSciNet  Google Scholar 

  58. Shabani, A., Lidar, D.A.: Theory of initialization-free decoherence-free subspaces and subsystems. Phys. Rev. A 72, 042303 (2005)

    ADS  Google Scholar 

  59. Lidar, D.A.: Review of decoherence free subspaces, noiseless subsystems, and dynamical decoupling. Adv. Chem. Phys. 154, 295 (2014)

    MATH  Google Scholar 

  60. Blume-Kohout, R., Khoon Ng, H., Poulin, D., Viola, L.: Characterizing the structure of preserved information in quantum processes. Phys. Rev. Lett. 100, 030501 (2008)

    ADS  Google Scholar 

  61. Blume-Kohout, R., Khoon Ng, H., Poulin, D., Viola, L.: Information-preserving structures: a general framework for quantum zero-error information. Phys. Rev. A 82, 062306 (2010)

    ADS  Google Scholar 

  62. Alber, G., Beth, Th, Charnes, Ch., Delgado, A., Grassl, M., Mussinger, M.: Stabilizing distinguishable qubits against spontaneous decay by detected-jump correcting quantum codes. Phys. Rev. Lett. 86, 4402 (2001)

    ADS  Google Scholar 

  63. Alber, G., Delgado, A., Mussinger, M.: Quantum error correction and quan-tum computation with detected-jump correcting quantum codes. Fortschr. Phys. 49, 901 (2001)

    MathSciNet  MATH  Google Scholar 

  64. Khodjasteh, K., Lidar, D.A.: Universal fault-tolerant quantum computationin the presence of spontaneous emission and collective dephasing. Phys. Rev. Lett. 89, 197904 (2002)

    ADS  Google Scholar 

  65. Knill, E., Laflamme, R., Viola, L.: Theory of quantum error correction for general noise. Phys. Rev. Lett. 84, 2525 (2000)

    ADS  MathSciNet  MATH  Google Scholar 

  66. Ollerenshaw, J.E., Lidar, D.A., Kay, L.E.: Magnetic resonance realization of decoherence-free quantum computation. Phys. Rev. Lett. 91, 217904 (2003)

    ADS  Google Scholar 

  67. Zanardi, P., Rossi, F.: Quantum information in semiconductors: noiseless encoding in a quantum-dot array. Phys. Rev. Lett. 81, 4752 (1998)

    ADS  Google Scholar 

  68. Zanardi, P., Rossi, F.: Subdecoherent information encoding in a quantum-dot array. Phys. Rev. B 59, 8170 (1999)

    ADS  Google Scholar 

  69. Kielpinski, D., Monroe, C., Wineland, D.J.: Architecture for a large-scale ion-trap quantum computer. Nature 417, 709 (2002)

    ADS  Google Scholar 

  70. Lidar, D.A., Whaley, K.B.: Decoherence-free subspaces and subsystems. In: Benatti, F., Floreanini, R. (eds.) Irreversible Quantum Dynamics. Springer, Berlin (2003)

    Google Scholar 

  71. Farhi, E., Gutmann, S.: Analog analogue of a digital quantum computation. Phys. Rev. A 57, 2403 (1998)

    ADS  Google Scholar 

  72. Anand, N., Pati, A.K.: Coherence and entanglement monogamy in the discrete analogue of analog Grover search. arXiv: 1611.04542 [quant-ph] (2016)

  73. Kagalwala, K.H., Di Giuseppe, G., Abouraddy, A.F., Saleh, B.E.A.: Bell’s measure in classical optical coherence. Nat. Photonics 7, 72 (2013)

    ADS  Google Scholar 

Download references

Acknowledgements

We would like to thank Professor M.S. Sarandy by fruitful discussions. This work is supported by the Brazilian agencies CNPq, CAPES, FAPERJ and the Brazilian National Institute for Science and Technology of Quantum Information (INCT-IQ).

Author information

Authors and Affiliations

  1. Instituto de Física, Universidade Federal Fluminense, Av. Gal. Milton Tavares de Souza s/n, Gragoatá, Niterói, RJ, 24210-346, Brazil

    P. C. Obando, M. H. M. Passos & J. A. O. Huguenin

  2. Brazilian Center for Research in Physics (CBPF), Rua Dr. Xavier Sigaud, 150, Rio de Janeiro, RJ, Brazil

    P. C. Obando

  3. Instituto de Ciências Exatas, Universidade Federal Fluminense, Volta Redonda, RJ, Brazil

    M. H. M. Passos & J. A. O. Huguenin

  4. Núcleo de Ciências Físicas, Universidade Tecnológica Federal do Paraná, Rua Cristo rei 19, Vila Becker, Toledo, PR, 85902-490, Brazil

    F. M. Paula

  5. Centro de Ciências Naturais e Humanas, Universidade Federal do ABC, Avenida dos Estados 5001, Santo André, SP, 09210-580, Brazil

    F. M. Paula

Authors
  1. P. C. Obando
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. M. H. M. Passos
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. F. M. Paula
    View author publications

    You can also search for this author inPubMed Google Scholar

  4. J. A. O. Huguenin
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to J. A. O. Huguenin.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Obando, P.C., Passos, M.H.M., Paula, F.M. et al. Simulating Markovian quantum decoherence processes through an all-optical setup. Quantum Inf Process 19, 7 (2020). https://doi.org/10.1007/s11128-019-2499-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11128-019-2499-8

Keywords