Quantum witness of a damped and driven qubit by sequential intermediate measurements with uniform and nonuniform time intervals

Abstract

Measurement can reveal the difference between quantum physics and classical one. Recently, a quantum witness $W_q$ was proposed to characterize quantumness (Li et al. in Sci Rep 2:885, 2012; Kofler and Brukner in Phys Rev A 87:052115, 2013). It is built upon the no-signaling-in-time condition, and there is only one-time intermediate measurement. As an extension, we consider here multiple intermediate measurements at different moments of time. And we discuss the quantumness of a damped and driven qubit. Uniform, quasiperiodic and random time-interval sequences (TISs) of measurements are considered, respectively. Numerical results show that $W_q$ depends on the kind of TISs when the number of measurements N is less than 10, while it is almost independent of the kind of TISs when N is larger. Further, $W_q\le W_q^{max}(N)=(1-\frac{1}{2N}) e^{-\gamma \tau }$ for all cases, where $\tau$ is the evolution time, $\gamma$ is the dephasing intensity, and $W_q^{max}(N)$ is the maximum violation of the quantum witness equality.

Appendices

Appendix A

For uniform TISs, based on Eq. (17) and at the driving amplitude $\Delta =0.0$, quantum witness is reduced to

$$\begin{aligned} W_q=\frac{1}{2}e^{-\gamma \tau } \left| \cos (\omega _0\tau )-\cos ^{N+1}\left( \frac{\omega _0\tau }{N+1}\right) \right| . {(A1)} \end{aligned}$$

As the number of intermediate measurement times $N\rightarrow \infty$ and $\omega _0\tau$ is finite, $\frac{\omega _0\tau }{N+1}\rightarrow 0$. Using Taylor expansion,

$$\begin{aligned} \cos \left( \frac{\omega _0\tau }{N+1}\right) \approx 1-\frac{1}{2}\left( \frac{\omega _0\tau }{N+1}\right) ^2 {(A2)} \end{aligned}$$

and

$$\begin{aligned} \cos ^{N+1}\left( \frac{\omega _0\tau }{N+1}\right) \approx \left[ 1-\frac{1}{2}\left( \frac{\omega _0\tau }{N+1}\right) ^2\right] ^{N+1}\approx 1-\frac{\omega _0^2\tau ^2}{2(N+1)}. {(A3)} \end{aligned}$$

Therefore,

$$\begin{aligned} W_q\approx \frac{1}{2}e^{-\gamma \tau } \left| \cos (\omega _0\tau )-1+\frac{\omega _0^2\tau ^2}{2(N+1)}\right| . {(A4)} \end{aligned}$$

At $\omega _0\tau =\pi$, $W_q\approx [1-\frac{\pi ^2}{4(N+1)}]e^{-\gamma \tau }<W_q^{max}(N)$.

Appendix B

Fig. 6

(Color online) a Probability $p''_+$ and $p_+$ as functions of time $\tau$; Inset in a: Partial enlarger for $\tau$ in the range [10, 12.5]. b Factor $\chi$ as functions of time $\tau$. For uniform, quasiperiodic and random TISs, the probability $p''_+$ are denoted by $p''^u_+, p''^f_+$ and $p''^r_+$, and the factor $\chi$ are denoted by $\chi _u, \chi _f$ and $\chi _r$, respectively. Intermediate measurement times $N=10^4$. The driving amplitude $\Delta =0.95$, the dephasing intensity $\gamma =0.2$ and the original transition frequency $\omega _0=1.0$

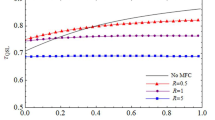
For uniform, quasiperiodic and random TISs, Fig. 4d shows that quantum witness $W_q$ as function of time $\tau$ at intermediate measurement times $N=10^4$. As given in Eq. (17),

$$\begin{aligned} W_q(\tau )=\Big |p_+(\tau )-p''_+(\tau )\Big |, {(B1)} \end{aligned}$$

where $p_+(\tau )$ and $p''_+(\tau )$ are the probabilities of the system in the $|+\rangle$ eigenstate at last moment $\tau$ in the presence and in the absence of multiple intermediate measurements. As given in Eq. (11),

$$\begin{aligned} p_+(\tau )=\frac{1}{2}\left[ 1+e^{-\gamma \tau } \cos \left( \frac{\Delta \tau ^{2}}{2}+\omega _0\tau \right) \right] ,{(B2)} \end{aligned}$$

so the probability $p_+(\tau )$ exhibits underdamped behavior, which is shown in Fig. 6a. Based on Eqs. (15) and (16), we define a factor

$$\begin{aligned} \chi =\prod _{n=1}^{N+1}\widetilde{V_{11}}(\tau _n,\tau _{n-1})=\prod _{n=1}^{N+1}\cos \left\{ -\frac{1}{2}(\tau _n-\tau _{n-1})\left[ \Delta (\tau _n+\tau _{n-1})+2\omega _0\right] \right\} ,{(B3)} \end{aligned}$$

then

$$\begin{aligned} p''_+(\tau )=\frac{1}{2}[1+\chi \exp (-\gamma \tau )].{(B4)} \end{aligned}$$

Figure 6a shows that $p''_+$ exhibits critically damped or overdamped behavior. The inset in Fig. 6a shows that there exist differences among $p''^u_+, p''^f_+$ and $p''^r_+$. On the whole, the differences are very small. In Eq.(B4), two factors, i.e., $\exp (-\gamma \tau )$ and $\chi$, make the decay of $p''_+$. Fig. 6b shows that $\chi$ smoothly decreases with time $\tau$. In fact, at relative small $\tau$, the differences among $\chi _u, \chi _f$ and $\chi _r$ are small. On the other hand, at relative large $\tau$, there exist obvious differences among $\chi _u, \chi _f$ and $\chi _r$; at the same time, the factor $\exp (-\gamma \tau )$ is smaller. Therefore, combined the two aspects, there are no obviously differences in $p''^u_+, p''^f_+$ and $p''^r_+$.

Appendix C

The system evolves within the range $[0, \tau ]$. The time interval is defined by $\mu _n=\tau _n-\tau _{n+1}$, so the measurement moment $\tau _n=\sum _{k=1}^{n}\mu _{k}$. The time interval $\mu _n$ is generated from the two-point distribution, exponential distribution, normal distribution, respectively.

The two-point distribution is

$$\begin{aligned} \mu = \left\{ \begin{array}{lcl} {\mu (C)} &{}\text {if probability}&{}p<p_c, \\ {\mu (D)} &{}\text {if probability}&{}p\ge p_c. \end{array} \right. {(C1)} \end{aligned}$$

We set $\mu (C):\mu (D)=1:2$ and $p_c=0.5$.

The exponential distribution function is

$$\begin{aligned} f(\mu ;\lambda ) = \left\{ \begin{array}{lcl} {\lambda e^{-\lambda \mu }} &{}\text {if}&{}\mu \ge 0, \\ {0} &{}\text {if}&{}\mu <0. \end{array} \right. {(C2)} \end{aligned}$$

We set $\lambda =1.0$.

The normal distribution function is

$$\begin{aligned} f(\mu ;\nu ,\sigma ) =\frac{1}{\sqrt{2\pi }\sigma }e^{-\frac{(\mu -\nu )^2}{2\sigma ^2}}, {(C3)} \end{aligned}$$

where $\nu$ is the mean value and $\sigma$ is the standard deviation. In calculation, we set $\nu =0.0$ and $\sigma =1$. At the same time, $\mu >0$ is used.

Fig. 7

(Color online) Quantum witness $W_q$ as functions of time $\tau$ with intermediate measurement times a $N=1$, b $N=2$, c $N=10$ and d $N=10^4$, respectively. For the two-point distribution, exponential distribution, normal distribution, intermediate measurement times N are denoted by $N_t, N_e$ and $N_n$, respectively. Here, $W_q^{max}(N)=(1-\frac{1}{2N})e^{-\gamma \tau }$, the driving amplitude $\Delta =0.95$, the dephasing intensity $\gamma =0.2$ and the original transition frequency $\omega _0=1.0$

In calculations, the time interval $\mu _n$ is generated from the above three different distributions. We also set $\tau _0=0$ and $\tau _{N+1}=\tau$. At last, $\tau _n$ is rescaled by the restriction that $\tau _{N+1}=\tau$. Quantum witness $W_q$ as functions of time $\tau$ is shown in Fig. 7a–d, respectively, where $N=1,2,10$ and $10^4$. It shows that for all cases, $W_q(\tau )\le W_q^{max}(N)=(1-\frac{1}{2N}) e^{-\gamma \tau }$. When intermediate measurement times N are tens [Fig. 7a–c], $W_q$ depends on the kind of TISs, while when N are larger [Fig. 7d], it is almost independent of the kind of TIS.