Decoherence of a two-level system in a coherent superposition of two dephasing environments

Abstract

Decoherence of a two-level system is studied when it interacts with one of two environments via a dephasing coupling, where the environments are treated as classical and quantum systems. The situation is considered in which the environment that actually interacts with the two-level system is determined by a state of an ancillary two-level system. When information conveyed by the ancillary two-level system is discarded, the reduced dynamics of the relevant two-level system is described by a statistical mixture of two dephasing channels, which shows mixing-induced non-Markovianity. When projective measurement is performed on the ancillary two-level system to extract some information, the diagonal elements of the reduced state of the two-level system depend on time in spite of the dephasing process. Furthermore, it is found that the coherence of the two-level system can exceed its initial value. The parameter region is clarified in which the measurement on the ancillary two-level system can improve the coherence of the relevant two-level system. In particular, when the two environments are equivalent and the ancillary two-level system is in the maximally coherent state, the coherence of the two-level system can be always enhanced by measurement.

The relaxation and phase parameters in the Ohmic-like dissipation

We derive explicit expressions for the relaxation and phase parameters, $\gamma _{j}(t)$ and $\theta _{j}(t)$, when the environment $E_{j}$ has the Ohmic-like dissipation. Taking a continuum limit as $4\sum _{k}\vert g_{j,k}\vert ^{2}F(\omega _{k}) \rightarrow \int _{0}^{\infty }\text {d}w\,J_{j}(w)F(w)$ with a spectral function $J_{j}(w)$ of the jth environment $E_{j}$, we obtain the relaxation and phase parameters from Eqs. (75) and (76)

$$\begin{aligned} \gamma _{j}(t)&=\int _{0}^{\infty }\text {d}w\,J_{j}(w) \frac{1-\cos w t}{w^{2}} \coth \left( \frac{\hbar w}{2k_{\mathrm {B}}T_{j}}\right) , \end{aligned}$$

(95)

$$\begin{aligned} \theta _{j}(t)&=\int _{0}^{\infty }\text {d}w\,J_{j}(w) \frac{w t-\sin w t}{w^{2}}. \end{aligned}$$

(96)

The spectral function of the Ohmic-like environment [20, 72, 73] is given by

$$\begin{aligned} J_{j}(w)=\lambda _{j}\varOmega _{j} \left( \frac{w}{\varOmega _{j}}\right) ^{s_{j}} \text {e}^{-w/\varOmega _{j}}, \end{aligned}$$

(97)

where $\lambda _{j}$ stands for a coupling strength between the two-level system Q and the environment $E_{j}$, $\varOmega _{j}$ is a cutoff frequency and $s_{j}$ represents an Ohmicity parameter of the environment $E_{j}$ which is referred to as the sub-Ohmic, Ohmic and super-Ohmic environment, according to $0<s_{j}<1$, $s_{j}=1$ and $1<s_{j}$. Then, the phase parameter $\theta _{j}(t)$ and the relaxation parameter $\gamma _{j}(t)$ [73, 74] are calculated to be

$$\begin{aligned} \theta _{j}(t)&=\lambda _{j}\varOmega _{j}\varGamma (s_{j})t +\lambda _{j}\tan ^{-1}(\varOmega _{j}t), \end{aligned}$$

(98)

$$\begin{aligned} \gamma _{j}(t)&=\frac{1}{2}\lambda _{j}\ln [1+(\varOmega _{j}t)^{2}] +2\lambda _{j}\nonumber \\&\quad \times \left\{ \ln \varGamma \left( 1+\frac{k_{\mathrm {B}}T_{j}}{\hbar \varOmega _{j}}\right) -\ln \left| \varGamma \left( 1+\frac{k_{\mathrm {B}}T_{j}}{\hbar \varOmega _{j}} +i\frac{k_{\mathrm {B}}T_{j}}{\hbar }t\right) \right| \right\} , \end{aligned}$$

(99)

for $s_{j}=1$ and

$$\begin{aligned} \theta _{j}(t)&=\lambda _{j}\varOmega _{j}\varGamma (s_{j})t +\lambda _{j}\varGamma (s_{j}-1) \frac{\sin [(s_{j}-1)\tan ^{-1}(\varOmega _{j}t)]}{[1+(\varOmega _{j}t)^{2}]^{(s_{j}-1)/2}}, \end{aligned}$$

(100)

$$\begin{aligned} \gamma _{j}(t)&=\lambda _{j}\varGamma (s_{j}-1)g_{s_{j}}(\varOmega _{j}t) +2\lambda _{j}\left( \frac{k_{\mathrm {B}}T_{j}}{\hbar \varOmega _{j}}\right) ^{s_{j}-1} \varGamma (s_{j}-1)\nonumber \\&\quad \times \sum _{n=1}^{\infty } \frac{\displaystyle { g_{s_{j}}\left( \frac{\frac{k_{\mathrm {B}}T_{j}}{\hbar }t}{n+\frac{k_{\mathrm {B}}T_{j}}{\hbar \varOmega _{j}}}\right) }}{\displaystyle { \left( n+\frac{k_{\mathrm {B}}T_{j}}{\hbar \varOmega _{j}}\right) ^{(s_{j}-1)/2}} }, \end{aligned}$$

(101)

for $s_{j}\ne 1$, where $g_{s}(z)$ is given by

$$\begin{aligned} g_{s}(z)=1-\frac{\cos [(s-1)\tan ^{-1}z]}{(1+z^{2})^{(s-1)/2}}. \end{aligned}$$

(102)

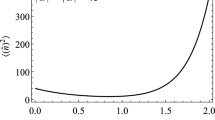
The asymptotic value of the relaxation parameter is

$$\begin{aligned} \gamma _{j}(\infty )={\left\{ \begin{array}{ll} \lambda _{j}\varGamma (s_{j}-1) &{} (T_{j}=0 \text { and }s_{j}>1) \\ \displaystyle {\lambda _{j}\varGamma (s_{j}-1)\left[ 1+2\left( \frac{k_{\mathrm {B}}T}{\hbar \varOmega }\right) ^{s_{j}-1} \zeta (s_{j}-1,1+k_{\mathrm {B}}T/\hbar \varOmega )\right] } &{} (T_{j}>0 \text { and }s_{j}>2) \\ \infty &{} \text {(otherwise)} \end{array}\right. } \qquad \end{aligned}$$

(103)

with $\zeta (s,a)=\sum _{n=0}^{\infty }(n+a)^{-s}$. We note that the coherence of the two-level system Q remains finite in the asymptotic limit unless $\lim _{t\rightarrow \infty }\gamma _{j}(t)=\infty$ [73]. It is also known that the reduced time-evolution of the two-level system Q which is caused by the environment $E_{j}$ is Markovian (non-Markovian) if $s_{j}\le 2$ ($s_{j}>2$).