Skip to main content

Advertisement

Springer Nature Link
Log in

Entanglement in a cavity magnomechanical system

  • Published:
Quantum Information Processing Aims and scope Submit manuscript

Abstract

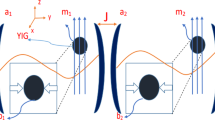
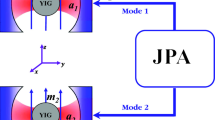
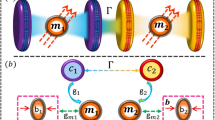
We study steady-state three bipartite entanglements in a cavity magnomechanical system which is composed of magnon, two cavities, and mechanical resonator. The two cavities are connected by an optical fiber, one of cavities couples to the magnon via the magnetic dipole interaction, at the same time, the magnon couples to the mechanical resonator via magnetostrictive interaction. Making use of logarithmic negativity, magnon, cavity, and mechanical resonator are entangled with each other by selecting appropriately the parameter. We find that the steady-state entanglements in the magnon–mechanical resonator subsystem and in the magnon–cavity subsystem are very sensitive to the effective magnomechanical coupling strength. Moreover, the entanglement is robust with respect to the certain environment temperature.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

Data Availability Statement

All data generated or analysed during this study are included in this published article (and its supplementary information files).

References

  1. Vitali, D., Gigan, S., Ferreira, A., Böm, H.R., Tombesi, P., Guerreiro, A., Vedral, V., Zeilinger, A., Aspelmeyer, M.: Optomechanical entanglement between a movable mirror and a cavity field. Phys. Rev. Lett. 98, 030405 (2007)

    Article  ADS  Google Scholar 

  2. Genes, C., Vitali, D., Tombesi, P.: Emergence of atom-light-mirror entanglement inside an optical cavity. Phys. Rev. A 77, 050307 (2008)

    Article  ADS  Google Scholar 

  3. Villanueva, L.G., Schmid, S.: Evidence of surface loss as ubiquitous limiting damping mechanism in SiN micro- and nanomechanical resonators. Phys. Rev. Lett. 113, 227201 (2014)

    Article  ADS  Google Scholar 

  4. Zhang, X.F., Zou, C.L., Jiang, L., Tang, H.X.: Strongly coupled magnons and cavity microwave photons. Phys. Rev. Lett. 113, 156401 (2014)

    Article  ADS  Google Scholar 

  5. Huebl, H., Zollitsch, C.W., Lotze, J., Hocke, F., Greifenstein, M., Marx, A., Gross, R., Goennenwein, S.T.B.: High cooperativity in coupled microwave resonator ferrimagnetic insulator hybrids. Phys. Rev. Lett. 111, 127003 (2013)

    Article  ADS  Google Scholar 

  6. Bennett, C.H., Brassard, G., Crépeau, C., Jozsa, R., Peres, A., Wootters, W.K.: Teleporting an unknown quantum state via dual classical and Einstein-Podolsky-Rosen channels. Phys. Rev. Lett. 70, 1895 (1993)

    Article  ADS  MathSciNet  Google Scholar 

  7. Pellizzari, T., Gardiner, S.A., Cirac, J.I., Zoller, P.: Decoherence, continuous observation, and quantum computing: a cavity QED model. Phys. Rev. Lett. 75, 3788 (1995)

    Article  ADS  Google Scholar 

  8. Jones, J.A., Jaksch, D.D.: Quantum Information. Cambridge University Press, Cambridge (2012)

    MATH  Google Scholar 

  9. Jennewein, T., Simon, C., Weihs, G., Weinfurter, H., Zeilinger, A.: Quantum cryptography with entangled photons. Phys. Rev. Lett. 84, 4729 (2000)

    Article  ADS  Google Scholar 

  10. Wehner, S., Elkouss, D., Hanson, R.: Quantum internet: a vision for the road ahead. Science 362, 6412 (2018)

    Article  MathSciNet  Google Scholar 

  11. Julsgaard, B., Kozhekin, A., Polzik, E.S.: Experimental long-lived entanglement of two macroscopic objects. Nature (London) 413, 400 (2001)

    Article  ADS  Google Scholar 

  12. Krauter, H., Muschik, C.A., Jensen, K., Wasilewski, W., Petersen, J.M., Cirac, J.I., Polzik, E.S.: Entanglement generated by dissipation and steady state entanglement of two macroscopic objects. Phys. Rev. Lett. 107, 080503 (2011)

    Article  ADS  Google Scholar 

  13. Neeley, M., Bialczak, R.C., Lenander, M., Lucero, E., Mariantoni, M., O’Connell, A.D., Sank, D., Wang, H., Weides, M., Wenner, J., Yin, Y., Yamamoto, T., Cleland, A.N., Martinis, J.M.: Generation of three-qubit entangled sates using superconducting phase qubits. Nature (London) 467, 570 (2010)

  14. DiCarlo, L., Reed, M.D., Sun, L., Johnson, R.L., Chow, J.M., Gambetta, J.M., Frunzio, L., Girvin, S.M., Devoret, M.H., Schoelkopf, R.J.: Preparation and measurement of three-qubit entanglement in a superconducting circuit. Nature (London) 467, 574 (2010)

    Article  ADS  Google Scholar 

  15. Flurin, E., Roch, N., Mallet, F., Devoret, M.H., Huard, B.: Generating entangled microwave radiation over two transmission lines. Phys. Rev. Lett. 109, 183901 (2012)

    Article  ADS  Google Scholar 

  16. Yan, X.B., Deng, Z.J., Tian, X.D., Wu, J.H.: Entanglement optimization of filtered output fields in cavity optomechanics. Opt. Express 27, 24393–24402 (2019)

    Article  ADS  Google Scholar 

  17. Yan, X.B.: Enhanced output entanglement with reservoir engineering. Phys. Rev. A 96, 053831 (2017)

    Article  ADS  Google Scholar 

  18. Li, J., Zhu, S.Y., Agarwal, G.S.: Magnon-photon-phonon entanglement in cavity magnomechanics. Phys. Rev. Lett. 121, 203601 (2018)

    Article  ADS  Google Scholar 

  19. Li, J., Li, G., Zippilli, S., Vitali, D., Zhang, T.C.: Enhanced entanglement of two different mechanical resonators via coherent feedback. Phys. Rev. A 95, 043819 (2017)

    Article  ADS  Google Scholar 

  20. Kong, C., Xiong, H., Wu, Y.: Magnon-induced nonreciprocity based on the Magnon Kerr effect. Phys. Rev. Appl. 12(3), 034001 (2019)

    Article  ADS  Google Scholar 

  21. Zhang, G.Q., You, J.Q.: Higher-order exceptional point in a cavity magnonics system. Phys. Rev. B 99, 054404 (2019)

    Article  ADS  Google Scholar 

  22. Wang, B., Liu, Z.X., Kong, C., Xiong, H., Wu, Y.: Magnon-induced transparency and amplification in PT-symmetric cavity-magnon system. Opt. Express 26, 20248–20257 (2018)

    Article  ADS  Google Scholar 

  23. Kong, C., Wang, B., Liu, Z.X., Xiong, H., Wu, Y.: Magnetically controllable slow light based on magnetostrictive forces. Opt. Express 27, 5544 (2019)

    Article  ADS  Google Scholar 

  24. Liu, Z.X., Xiong, H., Wu, Y.: Magnon blockade in a hybrid ferromagnet-superconductor quantum system. Phys. Rev. B 100, 134421 (2019)

    Article  ADS  Google Scholar 

  25. Wang, L., Yang, Z., Liu, Y., Bai, C.H., Wang, D.Y., Zhang, S., Wang, H.F.: Magnon blockade in a PT-symmetric-like cavity magnomechanical system. Ann. Phys. 532, 2000028 (2020)

    Article  MathSciNet  Google Scholar 

  26. Yuan, H.Y., Zheng, Shasha, Ficek, Zbigniew, He, Q.Y., Yung, M.H.: Enhancement of magnon-magnon entanglement inside a cavity. Phys. Rev. B 101, 014419 (2020)

    Article  ADS  Google Scholar 

  27. Yang, Z.B., Yang, R.C., Liu, H.Y.: Generation of optical-photon-and-magnon entanglement in an optomagnonics-mechanical system. Quantum Inf. Process. 19, 264 (2020)

    Article  ADS  MathSciNet  Google Scholar 

  28. Xu, W.L., Gao, Y.P., Wang, T.J., Wang, C.: Magnon-induced optical high-order sideband generation in hybrid atom-cavity optomagnonical system. Opt. Express 28, 22334–22344 (2020)

    Article  ADS  Google Scholar 

  29. Gao, Y.P., Cao, C., Duan, Y.W., Liu, X.F., Pang, T.T., Wang, T.J., Wang, C.: Magnons scattering induced photonic chaos in the optomagnonic resonators. Nanophotonics 9, 1953–1961 (2019)

    Article  Google Scholar 

  30. Graf, J., Pfeifer, H., Marquardt, F., Kusminskiy, S.V.: Cavity optomagnonics with magnetic textures: coupling a magnetic vortex to light. Phys. Rev. B 98, 241406 (R) (2018)

  31. Li, J., Zhu, S.Y.: Entangling two magnon modes via magnetostrictive interaction. New J. Phys. 21, 085001 (2019)

    Article  ADS  Google Scholar 

  32. Zhang, X.F., Zou, C.L., Jiang, L., Tang, H.X.: Cavity magnomechanics. Sci. Adv. 2, e1501286 (2016)

    Article  ADS  Google Scholar 

  33. DeJesus, E.X., Kaufman, C.: Routh–Hurwitz criterion in the examination of eigenvalues of a system of nonlinear ordinary differential equations. Phys. Rev. A 35, 5288–90 (1987)

    Article  ADS  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. College of Physics, Tonghua Normal University, Tonghua, 134000, Jilin, People’s Republic of China

    Jing Wang

Authors
  1. Jing Wang
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Jing Wang.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wang, J. Entanglement in a cavity magnomechanical system. Quantum Inf Process 21, 105 (2022). https://doi.org/10.1007/s11128-022-03438-4

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11128-022-03438-4

Keywords