Skip to main content

Advertisement

Springer Nature Link
Log in

Low-rank approximation to entangled multipartite quantum systems

  • Published:
Quantum Information Processing Aims and scope Submit manuscript

Abstract

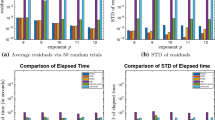
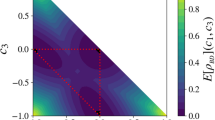
Qualifying the entanglement of a mixed multipartite state by gauging its distance to the nearest separable state of a fixed rank is a challenging but critically important task in quantum technologies. Such a task is computationally demanding partly because of the necessity of optimization over the complex field in order to characterize the underlying quantum properties correctly and partly because of the high nonlinearity due to the multipartite interactions. Representing the quantum states as complex density matrices with respect to some suitably selected bases, this work offers two avenues to tackle this problem numerically. For the rank-1 approximation, an iterative scheme solving a nonlinear singular value problem is investigated. For the general low-rank approximation with probabilistic combination coefficients, a projected gradient dynamics is proposed. Both techniques are shown to converge globally to a local solution. Numerical experiments are carried out to demonstrate the effectiveness and the efficiency of these methods.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

Data Availability Statements

The datasets generated during and/or analyzed during the current study are available from the corresponding author on reasonable request.

Notes

  1. The very same notation \(\otimes \) has been used for many different meanings in the literature. The distinction between a tensor product and the Kronecker product is necessary for computation and will be explained in Footnote 2. For a general composite system \({\mathscr {H}}_{1} \otimes {\mathscr {H}}_{2}\), we emphasize that \(\otimes \) is merely a bilinear map.

  2. The tensor product of tensors leads to a multi-indexed array. While the way to enumerate its elements is often immaterial in theory, it is essential to enumerate them consistently for numerical calculation. One general rule adopted is that the indices of the leftmost tensor are counted first, e.g., the indices in the tensor product \({\mathbf {a}} \circ {\mathbf {b}}\) of two vectors are enumerated in the same way as the matrix \({\mathbf {a}}{\mathbf {b}}^{\top }\). The relationship (2.3) therefore follows.

References

  1. Einstein, A., Podolsky, B., Rosen, N.: Can quantum-mechanical description of physical reality be considered complete? Phys. Rev. 47, 777–780 (1935). https://doi.org/10.1103/PhysRev.47.777

    Article  ADS  MATH  Google Scholar 

  2. Friis, N., Vitagliano, G., Malik, M., Huber, M.: Entanglement certification from theory to experiment. Nat. Rev. Phys. 1, 72–87 (2019). https://doi.org/10.1038/s42254-018-0003-5

    Article  Google Scholar 

  3. Gühne, O., Tóth, G.: Entanglement detection. Phys. Rep. 474, 1–75 (2009). https://doi.org/10.1016/j.physrep.2009.02.004

    Article  ADS  MathSciNet  Google Scholar 

  4. Horodecki, R., Horodecki, P., Horodecki, M., Horodecki, K.: Quantum entanglement. Rev. Mod. Phys. 81, 865–942 (2009). https://doi.org/10.1103/RevModPhys.81.865

    Article  ADS  MathSciNet  MATH  Google Scholar 

  5. Dahl, G., Leinaas, J.M., Myrheim, J., Ovrum, E.: A tensor product matrix approximation problem in quantum physics. Linear Algebra Appl. 420, 711–725 (2007). https://doi.org/10.1016/j.laa.2006.08.026

    Article  MathSciNet  MATH  Google Scholar 

  6. Kye, S.-H.: Necessary conditions for optimality of decomposable entanglement witnesses. Rep. Math. Phys. 69, 419–426 (2012). https://doi.org/10.1016/S0034-4877(13)60007-5

    Article  ADS  MathSciNet  MATH  Google Scholar 

  7. Thirring, W., Bertlmann, R.A., Köhler, P., Narnhofer, H.: Entanglement or separability: the choice of how to factorize the algebra of a density matrix. Eur. Phys. J. D 64, 181–196 (2011). https://doi.org/10.1140/epjd/e2011-20452-1

    Article  ADS  Google Scholar 

  8. Aaronson, S.: Quantum Computing Since Democritus. Cambridge University Press, Cambridge (2013). https://doi.org/10.1017/CBO9780511979309

    Book  MATH  Google Scholar 

  9. Hiai, F., Petz, D.: Introduction to matrix analysis and applications, Universitext, Springer, Cham; Hindustan Book Agency. New Delhi (2014). https://doi.org/10.1007/978-3-319-04150-6

  10. Nakahara, M., Ohmi, T.: Quantum Computing: From Linear Algebra to Physical Realizations. CRC Press, Boca Raton (2008). https://doi.org/10.1201/9781420012293

    Book  MATH  Google Scholar 

  11. Nielsen, M.A., Chuang, I.L.: Quantum Computation and Quantum Information. Cambridge University Press, Cambridge (2010). https://doi.org/10.1017/CBO9780511976667

    Book  MATH  Google Scholar 

  12. Karam, R.: Why are complex numbers needed in quantum mechanics? some answers for the introductory level. Am. J. Phys. 88, 39–45 (2020). https://doi.org/10.1119/10.0000258

    Article  ADS  Google Scholar 

  13. Renou, M.-O., Trillo, D., Weilenmann, M., Le, T. P., Tavakoli, A., Gisin, N., Acín, A., Navascués, M.: Quantum theory based on real numbers can be experimentally falsified, Nature, 1–5 (2021). https://doi.org/10.1038/s41586-021-04160-4

  14. Chen, K., Wu, L.-A.: A matrix realignment method for recognizing entanglement. Quantum Inf. Comput. 3, 193–202 (2003). https://doi.org/10.26421/QIC3.3-1

    Article  MathSciNet  MATH  Google Scholar 

  15. Werner, R.F.: Quantum states with Einstein–Podolsky–Rosen correlations admitting a hidden-variable model. Phys. Rev. A 40, 4277–4281 (1989). https://doi.org/10.1103/PhysRevA.40.4277

    Article  ADS  MATH  Google Scholar 

  16. Horodecki, M., Horodecki, P., Horodecki, R.: Mixed-State Entanglement and Quantum Communication, pp. 151–195. Springer, Berlin, Heidelberg (2001). https://doi.org/10.1007/3-540-44678-8_5

    Book  MATH  Google Scholar 

  17. Chen, L., Aulbach, M., Hajdušek, M.: Comparison of different definitions of the geometric measure of entanglement. Phys. Rev. A 89, 042305 (2014). https://doi.org/10.1103/PhysRevA.89.042305

    Article  ADS  Google Scholar 

  18. Leinaas, J.M., Myrheim, J., Ovrum, E.: Geometrical aspects of entanglement. Phys. Rev. A (3) 74, 012313, 13 (2006). https://doi.org/10.1103/PhysRevA.74.012313

    Article  ADS  MathSciNet  Google Scholar 

  19. Golub, G.H., Van Loan, C.F.: Matrix computations. In: Johns Hopkins Studies in the Mathematical Sciences, 4 edn. Johns Hopkins University Press, Baltimore (2013)

  20. Webster, R.: Convexity. Oxford Science Publications, The Clarendon Press, Oxford University Press, New York (1994)

    MATH  Google Scholar 

  21. Jia, Z.-A., Zhai, R., Yu, S., Wu, Y.-C., Guo, G.-C.: Hierarchy of genuine multipartite quantum correlations, Quantum Inf. Process., 19 (2020), pp. Paper No. 419, 13. https://doi.org/10.1007/s11128-020-02922-z

  22. Kolda, T.G., Bader, B.W.: Tensor decompositions and applications. SIAM Rev. 51, 455–500 (2009). https://doi.org/10.1137/07070111X

    Article  ADS  MathSciNet  MATH  Google Scholar 

  23. Vervliet, N., Debals, O., Sorber, L., Van Barel, M., De Lathauwer, L.: Tensorlab 3.0 (2016). https://www.tensorlab.net

  24. Chu, M.T., Lin, M.M.: Nonlinear power-like and SVD-like iterative schemes with applications to entangled bipartite rank-1 approximation. SIAM J. Sci. Comput. 43, S448–S474 (2021). https://doi.org/10.1137/20M1336059

    Article  MathSciNet  MATH  Google Scholar 

  25. Van Loan, C.F.: Structured matrix problems from tensors, in Exploiting hidden structure in matrix computations: algorithms and applications, vol. 2173 of Lecture Notes in Math. Springer, Cham, pp. 1–63 (2016)

  26. Guan, Y., Chu, M.T., Chu, D.: SVD-based algorithms for the best rank-1 approximation of a symmetric tensor. SIAM J. Matrix Anal. Appl. 39, 1095–1115 (2018). https://doi.org/10.1137/17M1136699

    Article  MathSciNet  MATH  Google Scholar 

  27. Wirtinger, W.: Zur formalen Theorie der Funktionen von mehr komplexen Veränderlichen. Math. Ann. 97, 357–375 (1927). https://doi.org/10.1007/BF01447872

    Article  MathSciNet  MATH  Google Scholar 

  28. Guan, Y., Chu, M.T., Chu, D.: Convergence analysis of an SVD-based algorithm for the best rank-1 tensor approximation. Linear Algebra Appl. 555, 53–69 (2018). https://doi.org/10.1016/j.laa.2018.06.006

    Article  MathSciNet  MATH  Google Scholar 

  29. García, C.B., Li, T.-Y.: On the number of solutions to polynomial systems of equations. SIAM J. Numer. Anal. 17, 540–546 (1980). https://doi.org/10.1137/0717046

    Article  ADS  MathSciNet  MATH  Google Scholar 

  30. Sommese, A.J., Wampler, C.W.: The Numerical Solution of Systems of Polynomials Arising in Engineering and Science. World Scientific, Singapore (2005). https://doi.org/10.1142/5763

    Book  MATH  Google Scholar 

  31. Moré, J.J., Sorensen, D.C.: Computing a trust region step. SIAM J. Sci. Statist. Comput. 4, 553–572 (1983). https://doi.org/10.1137/0904038

    Article  MathSciNet  MATH  Google Scholar 

  32. Shampine, L.F., Thompson, S., Kierzenka, J.A., Byrne, G.D.: Non-negative solutions of ODEs. Appl. Math. Comput. 170, 556–569 (2005). https://doi.org/10.1016/j.amc.2004.12.011

    Article  MathSciNet  MATH  Google Scholar 

  33. Chill, R.: On the łojasiewicz–Simon gradient inequality. J. Funct. Anal. 201, 572–601 (2003). https://doi.org/10.1016/S0022-1236(02)00102-7

    Article  MathSciNet  MATH  Google Scholar 

  34. S. Łojasiewicz, Une propriété topologique des sous-ensembles analytiques réels, in Les Équations aux Dérivées Partielles (Paris, 1962), Éditions du Centre National de la Recherche Scientifique, Paris, pp. 87–89 (1963)

  35. Absil, P.-A., Mahony, R., Andrews, B.: Convergence of the iterates of descent methods for analytic cost functions. SIAM J. Optim. 16, 531–547 (2005). https://doi.org/10.1137/040605266

    Article  MathSciNet  MATH  Google Scholar 

  36. Pierre, M.: Quelques applications de l’inégalit’e de Lojasiewicz à des discrétisations d’EDP. SMAI. http://smai.emath.fr/smai2011/slides/mpierre/Slides.pdf (2011)

  37. Gharibian, S.: Strong NP-hardness of the quantum separability problem. Quantum Inf. Comput. 10, 343–360 (2010)

    MathSciNet  MATH  Google Scholar 

  38. Gurvits, L.: Classical complexity and quantum entanglement. J. Comput. System Sci. 69, 448–484 (2004). https://doi.org/10.1016/j.jcss.2004.06.003

    Article  MathSciNet  MATH  Google Scholar 

  39. Ekert, A., Knight, P.L.: Entangled quantum systems and the Schmidt decomposition. Am. J. Phys. 63, 415–423 (1995). https://doi.org/10.1119/1.17904

    Article  ADS  MathSciNet  MATH  Google Scholar 

  40. Eltschka, C., Siewert, J.: Entanglement of three-qubit Greenberger–Horne–Zeilinger-symmetric states. Phys. Rev. Lett. 108, 020502 (2012). https://doi.org/10.1103/PhysRevLett.108.020502

    Article  ADS  Google Scholar 

  41. Greenberger, D.M., Horne, M.A., Zeilinger, A.: Going Beyond Bell’s Theorem, pp. 69–72. Springer, Dordrecht (1989). https://doi.org/10.1007/978-94-017-0849-4_10

  42. Vidal, G., Tarrach, R.: Robustness of entanglement. Phys. Rev. A 59, 141–155 (1999). https://doi.org/10.1103/PhysRevA.59.141

    Article  ADS  MathSciNet  Google Scholar 

  43. Braunstein, S.L., Caves, C.M., Jozsa, R., Linden, N., Popescu, S., Schack, R.: Separability of very noisy mixed states and implications for nmr quantum computing. Phys. Rev. Lett. 83, 1054–1057 (1999). https://doi.org/10.1103/PhysRevLett.83.1054

    Article  ADS  Google Scholar 

  44. Murao, M., Plenio, M.B., Popescu, S., Vedral, V., Knight, P.L.: Multiparticle entanglement purification protocols. Phys. Rev. A 57, R4075–R4078 (1998). https://doi.org/10.1103/PhysRevA.57.R4075

    Article  ADS  Google Scholar 

  45. Dür, W., Cirac, J.I.: Classification of multiqubit mixed states: separability and distillability properties. Phys. Rev. A 61, 042314 (2000). https://doi.org/10.1103/PhysRevA.61.042314

    Article  ADS  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, National Cheng Kung University, Tainan, 701, Taiwan

    Matthew M. Lin

  2. Department of Mathematics, North Carolina State University, Raleigh, NC, 27695-8205, USA

    Moody T. Chu

Authors
  1. Matthew M. Lin
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Moody T. Chu
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Matthew M. Lin.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

M. M. Lin: This research was supported in part by the National Center for Theoretical Sciences of Taiwan and by the Ministry of Science and Technology of Taiwan under Grant 111-2636-M-006-018. M. T. Chu: This research was supported in part by the National Science Foundation under Grant DMS-1912816.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Lin, M.M., Chu, M.T. Low-rank approximation to entangled multipartite quantum systems. Quantum Inf Process 21, 120 (2022). https://doi.org/10.1007/s11128-022-03467-z

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11128-022-03467-z

Keywords

Mathematics Subject Classification