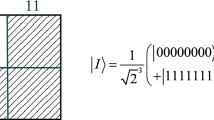
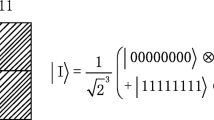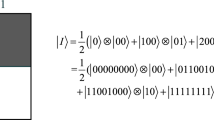Abstract
As a branch of quantum image processing, the quantum image scaling has been widely studied in the recent years. In this paper, an asymmetric scaling for quantum image with arbitrary scaling ratio is proposed. Firstly, the generalized quantum image representation is employed to represent a quantum image of arbitrary size \(H \times W\), and the bilinear interpolation is utilized to obtain the interpolated image. Then, the quantum circuit of the quantum image scaling algorithm with different scaling ratios in two dimensions is designed. Finally, the network complexity and simulation results of the two scaling methods are analyzed. The final result shows that the proposed scheme is a quadratic function, which is much lower than the cubic function and exponential function of other bilinear interpolation schemes.



















Similar content being viewed by others
Explore related subjects
Discover the latest articles and news from researchers in related subjects, suggested using machine learning.Data availability
The data that support the findings of this study are available from the corresponding author upon reasonable request.
References
Feynman, R.P.: Simulating physics with computers. Int. J. Theor. Phys. 21(6/7), 467–488 (1982)
Deutsch, D.: Quantum theory, the Church–Turing principle and the universal quantum computer. In: Proceedings of the royal society of London. A. mathematical and physical sciences 400.1818, 97–117 (1985)
Shor, P.: Algorithms for quantum computation: discrete logarithms and factoring. In: Proceedings of the 35th Annual Symposium on Foundations of Computer Science, pp. 124–134 (1994)
Grover, L.: A fast quantum mechanical algorithm for database search. In: Proceedings of the 28th Annual ACM Symposium on Theory of Computing, pp. 212–219 (1996)
Vlasov, A.Y.: Quantum computations and images recognition. arXiv:quant-ph/9703010. (1997)
Venegas-Andraca, S.E., Bose, S.: Storing, processing and retrieving an image using quantum mechanics. In: Proceedings of the SPIE Conference on Quantum Information and Computation, pp. 137–147 (2003)
Venegas-Andraca, S.E., Ball, J.L.: Processing images in entangled quantum systems. Quantum Inf. Process. 9(1), 1–11 (2010)
Le, P.Q., Dong, F.Y., Hirota, K.: A flexible representation of quantum images for polynomial preparation, image compression and processing operations. Quantum Inf. Process. 10(1), 63–84 (2011)
Zhang, Y., Lu, K., Gao, Y., Wang, M.: NEQR: a novel enhanced quantum representation of digital images. Quantum Inf. Process. 12, 2833–2860 (2013)
Jiang, N., Luo, W.: Quantum image scaling using nearest neighbor interpolation. Quantum Inf. Process. 14(1559), 1571 (2015)
Jiang, N., Wang, J., Mu, Y.: Quantum image scaling up based on nearest-neighbor interpolation with integer scaling ratio. Quantum Inf Process 14, 4001–4026 (2015)
Sang, J., Wang, S., Niu, X.: Quantum realization of the nearest-neighbor interpolation method for FRQI and NEQR. Quantum Inf. Process. 15, 37–64 (2016)
Li, P., Liu, X.: Bilinear interpolation method for quantum images based on quantum Fourier transform. Int. J. Quantum Inf. 16, 1850031 (2018)
Zhou, R.-G., Cheng, Y., Liu, D.: Quantum image scaling based on bilinear interpolation with arbitrary scaling ratio. Quantum Inf. Process. 18(9), 267 (2019)
Zhou, R.G., Cheng, Y., Qi, X., et al.: Asymmetric scaling scheme over the two dimensions of a quantum image. Quantum Inf Process 19, 343 (2020)
Yan, F., et al.: Implementing bilinear interpolation with quantum images. Digital Signal Process. 117, 103149 (2021)
Le, P.Q., Iliyasu, A.M., Dong, F., Hirota, K.: Fast geometric transformations on quantum images. IAENG Int. J. Appl. Math. 40, 3 (2010)
Le, P.Q., et al.: Efficient color transformations on quantum images. J. Adv. Comput. Intell. Intell. Inf. 15, 698–706 (2011)
Zhang, Y., et al.: Local feature point extraction for quantum images. Quantum Inf. Process. 14, 1573–1588 (2015)
Caraiman, S., Vasile, I.M.: Image segmentation on a quantum computer. Quantum Inf. Process. 14, 1693–1715 (2015)
Zhou, R.G., Hu, W., Fan, P.: Quantum watermarking scheme through Arnold scrambling and LSB steganography. Quantum Inf. Process. 16, 1–21 (2017)
Gonzalez, R.C., Woods, R.E.: Digital image processing. Prentice Hall, New Jersey (2007)
Le, P.Q., Iliyasu, A.M., Dong, F., Hirota, K.: Strategies for designing geometric transformations on quantum images. Theor. Comput. Sci. 412, 1406–1418 (2011)
Thapliyal, H., Ranganathan, N.: Design of efficient reversible binary subtractors based on a new reversible gate. In: Proceedings of the IEEE computer society annual symposium on VLSI, Tampa, pp. 229–234 (2009)
Thapliyal, H., Ranganathan, N.: A new design of the reversible subtractor circuit. In: 2011 11th IEEE Conference on Nanotechnology (IEEE-NANO), IEEE, pp. 1430–1435 (2011)
Khosropour, A., Aghababa, H., Forouzandeh, B.: Quantum division circuit based on restoring division algorithm. In: Eighth International Conference on Information Technology: New Generations, Itng 2011, Las Vegas, 11–13 April, DBLP, pp. 1037–1040 (2011)
Thapliyal, H., et al.: Quantum circuit designs of integer division optimizing T-count and T-depth. IEEE Trans. Emerg. Topics Comput. 9, 1045 (2019)
Muñoz-Coreas, E., Himanshu, T.: Quantum circuit design of a t-count optimized integer multiplier. IEEE Trans. Comput. 68, 729–739 (2018)
Kotiyal, S., Thapliyal, H., Ranganathan, N.: Circuit for reversible quantum multiplier based on binary tree optimizing Ancilla and garbage bits. In: 2014 27th International Conference on VLSI Design and 2014 13th International Conference on Embedded Systems, IEEE, pp. 545–550 (2014)
Islam, M.S., Rahman, M.M., Begum, Z., Hafiz, M.Z.: Low cost quantum realization of reversible multiplier circuit. Inf. Technol. J. 8(2), 208–213 (2009)
Ruiz-Perez, L., Garcia-Escartin, J.C.: Quantum arithmetic with the quantum Fourier transform. Quantum Inf. Process. 16(6), 152 (2017)
Nielson, M.A., Chuang, I.L.: Quantum computation and quantum information. Cambridge University Press, Cambridge (2000)
Acknowledgements
This work is supported by the National Natural Science Foundation of China under Grant No. 6217070290, Shanghai Science and Technology Project under Grant No. 21JC1402800 and 20040501500, and Top-notch Innovative Talent Program for Postgraduate Students of Shanghai Maritime University under Grant No. 2021YBR009.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
Springer Nature or its licensor holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Gao, C., Zhou, RG., Li, X. et al. Asymmetric scaling of a quantum image based on bilinear interpolation with arbitrary scaling ratio. Quantum Inf Process 21, 270 (2022). https://doi.org/10.1007/s11128-022-03612-8
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s11128-022-03612-8