Skip to main content
Springer Nature Link
Log in

Controllable magnon–magnon entanglement and one-way EPR steering with two cascaded cavities

  • Published:
Quantum Information Processing Aims and scope Submit manuscript

Abstract

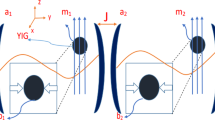
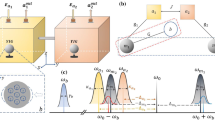
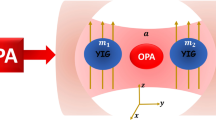
We propose an alternative scheme for achieving magnon–magnon entanglement and one-way Einstein–Podolsky–Rosen (EPR) steering via the cavity dissipation processes, where two cascaded cavities are respectively coupled to two separated magnon modes of the yttrium iron garnet (YIG) spheres. Based on the quantum theory, the reduced master equation of magnon modes is derived by adiabatically eliminating the cavity variables. The results show that the entanglement and one-way EPR steering of two distant magnons could be obtained and controlled by adequately selecting the physical parameters such as magneto-optical coupling strength, the coupling efficiency of cascaded cavities, and magnon dissipation rate. The remarkable feature of our scheme is that the generated bipartite correlations are insensitive to the dynamical parameter fluctuations. Furthermore, the simplified operation and actual parameters make the scheme more feasible, which may provide a new platform for the study of the macroscopic quantum phenomenon and quantum information processing.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

Data availability

Data sharing not applicable to this article as no datasets were generated or analysed during the current study.

References

  1. Zheng, S.-B., Guo, G.-C.: Efficient scheme for two-atom entanglement and quantum information processing in cavity QED. Phys. Rev. Lett. 85(11), 2392–2395 (2000). https://doi.org/10.1103/PhysRevLett.85.2392

    Article  ADS  Google Scholar 

  2. Wang, X.-L., Chen, L.-K., Li, W., Huang, H.-L., Liu, C., Chen, C., Luo, Y.-H., Su, Z.-E., Wu, D., Li, Z.-D., et al.: Experimental ten-photon entanglement. Phys. Rev. Lett. 117(21), 210502 (2016). https://doi.org/10.1103/PhysRevLett.117.210502

    Article  ADS  Google Scholar 

  3. Häffner, H., Hänsel, W., Roos, C., Benhelm, J., Chek-al-Kar, D., Chwalla, M., Körber, T., Rapol, U., Riebe, M., Schmidt, P., et al.: Scalable multiparticle entanglement of trapped ions. Nature 438(7068), 643–646 (2005). https://doi.org/10.1038/nature04279

    Article  ADS  Google Scholar 

  4. Riste, D., Dukalski, M., Watson, C., De Lange, G., Tiggelman, M., Blanter, Y.M., Lehnert, K.W., Schouten, R., DiCarlo, L.: Deterministic entanglement of superconducting qubits by parity measurement and feedback. Nature 502(7471), 350–354 (2013). https://doi.org/10.1038/nature12513

    Article  ADS  Google Scholar 

  5. Laurat, J., Choi, K., Deng, H., Chou, C., Kimble, H.: Heralded entanglement between atomic ensembles: preparation, decoherence, and scaling. Phys. Rev. Lett. 99(18), 180504 (2007). https://doi.org/10.1103/PhysRevLett.99.180504

    Article  ADS  Google Scholar 

  6. Schrödinger, E.: Discussion of probability relations between separated systems. In: Mathematical Proceedings of the Cambridge Philosophical Society, vol. 31, pp. 555–563. Cambridge University Press, Cambridge (1935). https://doi.org/10.1017/S0305004100013554

  7. Wiseman, H.M., Jones, S.J., Doherty, A.C.: Steering, entanglement, nonlocality, and the Einstein–Podolsky–Rosen paradox. Phys. Rev. Lett. 98(14), 140402 (2007). https://doi.org/10.1103/PhysRevLett.98.140402

    Article  MathSciNet  MATH  ADS  Google Scholar 

  8. Einstein, A., Podolsky, B., Rosen, N.: Can quantum-mechanical description of physical reality be considered complete? Phys. Rev. 47(10), 777 (1935). https://doi.org/10.1103/PhysRev.47.777

    Article  MATH  ADS  Google Scholar 

  9. Kogias, I., Lee, A.R., Ragy, S., Adesso, G.: Quantification of gaussian quantum steering. Phys. Rev. Lett. 114(6), 060403 (2015). https://doi.org/10.1103/PhysRevLett.114.060403

    Article  ADS  Google Scholar 

  10. Bartkiewicz, K., Černoch, A., Lemr, K., Miranowicz, A., Nori, F.: Temporal steering and security of quantum key distribution with mutually unbiased bases against individual attacks. Phys. Rev. A 93(6), 062345 (2016). https://doi.org/10.1103/PhysRevA.93.062345

    Article  ADS  Google Scholar 

  11. Barnum, H., Crépeau, C., Gottesman, D., Smith, A., Tapp, A.: Authentication of quantum messages. In: The 43rd Annual IEEE Symposium on Foundations of Computer Science, 2002. Proceedings., pp. 449–458. IEEE (2002). https://doi.org/10.1109/SFCS.2002.1181969

  12. Piani, M., Watrous, J.: Necessary and sufficient quantum information characterization of Einstein–Podolsky–Rosen steering. Phys. Rev. Lett. 114(6), 060404 (2015). https://doi.org/10.1103/PhysRevLett.114.060404

    Article  MathSciNet  ADS  Google Scholar 

  13. Sun, K., Ye, X.-J., Xiao, Y., Xu, X.-Y., Wu, Y.-C., Xu, J.-S., Chen, J.-L., Li, C.-F., Guo, G.-C.: Demonstration of Einstein–Podolsky–Rosen steering with enhanced subchannel discrimination. npj Quantum Inf. 4(1), 1–7 (2018). https://doi.org/10.1038/s41534-018-0067-1

    Article  ADS  Google Scholar 

  14. Zheng, S.-S., Sun, F.-X., Yuan, H.-Y., Ficek, Z., Gong, Q.-H., He, Q.-Y.: Enhanced entanglement and asymmetric EPR steering between magnons. Sci. China Phys. Mech. Astron. 64(1), 1–9 (2021). https://doi.org/10.1007/s11433-020-1587-5

    Article  ADS  Google Scholar 

  15. Wang, M., Xiang, Y., Kang, H., Han, D., Liu, Y., He, Q., Gong, Q., Su, X., Peng, K.: Deterministic distribution of multipartite entanglement and steering in a quantum network by separable states. Phys. Rev. Lett. 125(26), 260506 (2020). https://doi.org/10.1103/PhysRevLett.125.260506

    Article  ADS  Google Scholar 

  16. Zhong, W., Zhao, D., Cheng, G., Chen, A.: One-way Einstein–Podolsky–Rosen steering of macroscopic magnons with squeezed light. Opt. Commun. 497, 127138 (2021). https://doi.org/10.1016/j.optcom.2021.127138

    Article  Google Scholar 

  17. Xiang, Y., Su, X., Mišta, L., Jr., Adesso, G., He, Q.: Multipartite Einstein–Podolsky–Rosen steering sharing with separable states. Phys. Rev. A 99(1), 010104 (2019). https://doi.org/10.1103/PhysRevA.99.010104

    Article  ADS  Google Scholar 

  18. Armstrong, S., Wang, M., Teh, R.Y., Gong, Q., He, Q., Janousek, J., Bachor, H.-A., Reid, M.D., Lam, P.K.: Multipartite Einstein–Podolsky–Rosen steering and genuine tripartite entanglement with optical networks. Nat. Phys. 11(2), 167–172 (2015). https://doi.org/10.1038/nphys3202

    Article  Google Scholar 

  19. Li, C.-M., Chen, Y.-N., Lambert, N., Chiu, C.-Y., Nori, F.: Certifying single-system steering for quantum-information processing. Phys. Rev. A 92(6), 062310 (2015). https://doi.org/10.1103/PhysRevA.92.062310

    Article  ADS  Google Scholar 

  20. Händchen, V., Eberle, T., Steinlechner, S., Samblowski, A., Franz, T., Werner, R.F., Schnabel, R.: Observation of one-way Einstein–Podolsky–Rosen steering. Nat. Photonics 6(9), 596–599 (2012). https://doi.org/10.1038/nphoton.2012.202

    Article  ADS  Google Scholar 

  21. Wollmann, S., Walk, N., Bennet, A.J., Wiseman, H.M., Pryde, G.J.: Observation of genuine one-way Einstein–Podolsky–Rosen steering. Phys. Rev. Lett. 116(16), 160403 (2016). https://doi.org/10.1103/PhysRevLett.116.160403

    Article  ADS  Google Scholar 

  22. Tischler, N., Ghafari, F., Baker, T.J., Slussarenko, S., Patel, R.B., Weston, M.M., Wollmann, S., Shalm, L.K., Verma, V.B., Nam, S.W., et al.: Conclusive experimental demonstration of one-way Einstein–Podolsky–Rosen steering. Phys. Rev. Lett. 121(10), 100401 (2018). https://doi.org/10.1103/PhysRevLett.121.100401

    Article  ADS  Google Scholar 

  23. Zeng, Q., Shang, J., Nguyen, H.C., Zhang, X.: Reliable experimental certification of one-way Einstein–Podolsky–Rosen steering. Phys. Rev. Res. 4(1), 013151 (2022). https://doi.org/10.1103/PhysRevResearch.4.013151

    Article  Google Scholar 

  24. Chumak, A.V., Vasyuchka, V.I., Serga, A.A., Hillebrands, B.: Magnon spintronics. Nat. Phys. 11(6), 453–461 (2015). https://doi.org/10.1038/nphys3347

    Article  Google Scholar 

  25. Lachance-Quirion, D., Tabuchi, Y., Gloppe, A., Usami, K., Nakamura, Y.: Hybrid quantum systems based on magnonics. Appl. Phys. Express 12(7), 070101 (2019). https://doi.org/10.7567/1882-0786/ab248d

    Article  ADS  Google Scholar 

  26. Wang, X., Wang, J., Ren, Z., Wen, R., Zou, C.-L., Siviloglou, G.A., Chen, J.: Quantum interference between photons and single quanta of stored atomic coherence. Phys. Rev. Lett. 128(8), 083605 (2022). https://doi.org/10.1103/PhysRevLett.128.083605

    Article  ADS  Google Scholar 

  27. Zhang, X., Zou, C.-L., Jiang, L., Tang, H.X.: Strongly coupled magnons and cavity microwave photons. Phys. Rev. Lett. 113(15), 156401 (2014). https://doi.org/10.1103/PhysRevLett.113.156401

    Article  ADS  Google Scholar 

  28. Tabuchi, Y., Ishino, S., Ishikawa, T., Yamazaki, R., Usami, K., Nakamura, Y.: Hybridizing ferromagnetic magnons and microwave photons in the quantum limit. Phys. Rev. Lett. 113(8), 083603 (2014). https://doi.org/10.1103/PhysRevLett.113.083603

    Article  ADS  Google Scholar 

  29. Goryachev, M., Farr, W.G., Creedon, D.L., Fan, Y., Kostylev, M., Tobar, M.E.: High-cooperativity cavity QED with magnons at microwave frequencies. Phys. Rev. Appl. 2(5), 054002 (2014). https://doi.org/10.1103/PhysRevApplied.2.054002

    Article  ADS  Google Scholar 

  30. Bourhill, J., Kostylev, N., Goryachev, M., Creedon, D., Tobar, M.: Ultrahigh cooperativity interactions between magnons and resonant photons in a YIG sphere. Phys. Rev. B 93(14), 144420 (2016). https://doi.org/10.1103/PhysRevB.93.144420

    Article  ADS  Google Scholar 

  31. Kostylev, N., Goryachev, M., Tobar, M.E.: Superstrong coupling of a microwave cavity to yttrium iron garnet magnons. Appl. Phys. Lett. 108(6), 062402 (2016). https://doi.org/10.1063/1.4941730

    Article  ADS  Google Scholar 

  32. Julsgaard, B., Grezes, C., Bertet, P., Mølmer, K.: Quantum memory for microwave photons in an inhomogeneously broadened spin ensemble. Phys. Rev. Lett. 110(25), 250503 (2013). https://doi.org/10.1103/PhysRevLett.110.250503

    Article  ADS  Google Scholar 

  33. Grèzes, C., Kubo, Y., Julsgaard, B., Umeda, T., Isoya, J., Sumiya, H., Abe, H., Onoda, S., Ohshima, T., Nakamura, K., et al.: Towards a spin-ensemble quantum memory for superconducting qubits. C R Phys. 17(7), 693–704 (2016). https://doi.org/10.1016/j.crhy.2016.07.006

    Article  ADS  Google Scholar 

  34. Williamson, L.A., Chen, Y.-H., Longdell, J.J.: Magneto-optic modulator with unit quantum efficiency. Phys. Rev. Lett. 113(20), 203601 (2014). https://doi.org/10.1103/PhysRevLett.113.203601

    Article  ADS  Google Scholar 

  35. O’Brien, C., Lauk, N., Blum, S., Morigi, G., Fleischhauer, M.: Interfacing superconducting qubits and telecom photons via a rare-earth-doped crystal. Phys. Rev. Lett. 113(6), 063603 (2014). https://doi.org/10.1103/PhysRevLett.113.063603

    Article  ADS  Google Scholar 

  36. Hisatomi, R., Osada, A., Tabuchi, Y., Ishikawa, T., Noguchi, A., Yamazaki, R., Usami, K., Nakamura, Y.: Bidirectional conversion between microwave and light via ferromagnetic magnons. Phys. Rev. B 93(17), 174427 (2016). https://doi.org/10.1103/PhysRevB.93.174427

    Article  ADS  Google Scholar 

  37. Bienfait, A., Pla, J., Kubo, Y., Stern, M., Zhou, X., Lo, C., Weis, C., Schenkel, T., Thewalt, M., Vion, D., et al.: Reaching the quantum limit of sensitivity in electron spin resonance. Nat. Nanotechnol. 11(3), 253–257 (2016). https://doi.org/10.1038/nnano.2015.282

    Article  ADS  Google Scholar 

  38. Degen, C.L., Reinhard, F., Cappellaro, P.: Quantum sensing. Rev. Mod. Phys. 89(3), 035002 (2017). https://doi.org/10.1103/RevModPhys.89.035002

    Article  MathSciNet  ADS  Google Scholar 

  39. Tabuchi, Y., Ishino, S., Noguchi, A., Ishikawa, T., Yamazaki, R., Usami, K., Nakamura, Y.: Coherent coupling between a ferromagnetic magnon and a superconducting qubit. Science 349(6246), 405–408 (2015). https://doi.org/10.1126/science.aaa3693

    Article  MathSciNet  MATH  ADS  Google Scholar 

  40. Lachance-Quirion, D., Tabuchi, Y., Ishino, S., Noguchi, A., Ishikawa, T., Yamazaki, R., Nakamura, Y.: Resolving quanta of collective spin excitations in a millimeter-sized ferromagnet. Sci. Adv. 3(7), 1603150 (2017). https://doi.org/10.1126/sciadv.1603150

    Article  ADS  Google Scholar 

  41. Zhang, X., Zou, C.-L., Jiang, L., Tang, H.X.: Cavity magnomechanics. Sci. Adv. 2(3), 1501286 (2016). https://doi.org/10.1126/sciadv.1501286

    Article  ADS  Google Scholar 

  42. Li, J., Zhu, S.-Y., Agarwal, G.: Magnon–photon–phonon entanglement in cavity magnomechanics. Phys. Rev. Lett. 121(20), 203601 (2018). https://doi.org/10.1103/PhysRevLett.121.203601

    Article  ADS  Google Scholar 

  43. Li, J., Zhu, S.-Y., Agarwal, G.: Squeezed states of magnons and phonons in cavity magnomechanics. Phys. Rev. A 99(2), 021801 (2019). https://doi.org/10.1103/PhysRevA.99.021801

    Article  ADS  Google Scholar 

  44. Sun, F.-X., Zheng, S.-S., Xiao, Y., Gong, Q., He, Q., Xia, K.: Remote generation of magnon Schrödinger cat state via magnon–photon entanglement. Phys. Rev. Lett. 127(8), 087203 (2021). https://doi.org/10.1103/PhysRevLett.127.087203

    Article  ADS  Google Scholar 

  45. Wang, Y.-D., Clerk, A.A.: Reservoir-engineered entanglement in optomechanical systems. Phys. Rev. Lett. 110(25), 253601 (2013). https://doi.org/10.1103/PhysRevLett.110.253601

    Article  ADS  Google Scholar 

  46. Lü, X.-Y., Wu, Y., Johansson, J., Jing, H., Zhang, J., Nori, F.: Squeezed optomechanics with phase-matched amplification and dissipation. Phys. Rev. Lett. 114(9), 093602 (2015). https://doi.org/10.1103/PhysRevLett.114.093602

    Article  ADS  Google Scholar 

  47. Holstein, T., Primakoff, H.: Field dependence of the intrinsic domain magnetization of a ferromagnet. Phys. Rev. 58(12), 1098 (1940). https://doi.org/10.1103/PhysRev.58.1098

    Article  MATH  ADS  Google Scholar 

  48. Yang, W., Yin, Z., Chen, Q., Chen, C., Feng, M.: Two-mode squeezing of distant nitrogen-vacancy-center ensembles by manipulating the reservoir. Phys. Rev. A 85(2), 022324 (2012). https://doi.org/10.1103/PhysRevA.85.022324

    Article  ADS  Google Scholar 

  49. Gardiner, C.W., Zoller, P.: Quantum Noise. Springer, Berlin (2000)

    Book  MATH  Google Scholar 

  50. Duan, L.-M., Giedke, G., Cirac, J.I., Zoller, P.: Inseparability criterion for continuous variable systems. Phys. Rev. Lett. 84(12), 2722 (2000). https://doi.org/10.1103/PhysRevLett.84.2722

    Article  ADS  Google Scholar 

  51. Tan, H.-T., Zhu, S.-Y., Zubairy, M.S.: Continuous-variable entanglement in a correlated spontaneous emission laser. Phys. Rev. A 72(2), 022305 (2005). https://doi.org/10.1103/PhysRevA.72.022305

    Article  ADS  Google Scholar 

  52. Li, G.-X., Tan, H.-T., Macovei, M.: Enhancement of entanglement for two-mode fields generated from four-wave mixing with the help of the auxiliary atomic transition. Phys. Rev. A 76(5), 053827 (2007). https://doi.org/10.1103/PhysRevA.76.053827

    Article  ADS  Google Scholar 

  53. Reid, M.D.: Demonstration of the Einstein–Podolsky–Rosen paradox using nondegenerate parametric amplification. Phys. Rev. A 40(2), 913 (1989). https://doi.org/10.1103/PhysRevA.40.913

    Article  ADS  Google Scholar 

  54. Jones, S.J., Wiseman, H.M., Doherty, A.C.: Entanglement, Einstein–Podolsky–Rosen correlations, bell nonlocality, and steering. Phys. Rev. A 76(5), 052116 (2007). https://doi.org/10.1103/PhysRevA.76.052116

    Article  MathSciNet  MATH  ADS  Google Scholar 

Download references

Acknowledgements

This work is supported by the National Natural Science Foundation of China (Grants Nos. 12165007, 11905064, and 11775190) and the Scientific Research Foundation of Jiangxi Provincial Department of Education, China (Grant No. GJJ200624).

Author information

Authors and Affiliations

  1. Department of Applied Physics, East China Jiaotong University, Nanchang, 330013, China

    Dingwei Zhao, Wenxue Zhong & Guangling Cheng

  2. Department of Physics, Zhejiang Sci-Tech University, Hangzhou, 310018, China

    Aixi Chen

Authors
  1. Dingwei Zhao
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Wenxue Zhong
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Guangling Cheng
    View author publications

    You can also search for this author inPubMed Google Scholar

  4. Aixi Chen
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding authors

Correspondence to Guangling Cheng or Aixi Chen.

Ethics declarations

Conflict of interest

The authors declare no conflicts of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhao, D., Zhong, W., Cheng, G. et al. Controllable magnon–magnon entanglement and one-way EPR steering with two cascaded cavities. Quantum Inf Process 21, 384 (2022). https://doi.org/10.1007/s11128-022-03731-2

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11128-022-03731-2

Keywords