Generalized quantum teleportation of shared quantum secret: a coined quantum-walk approach

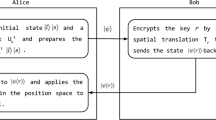
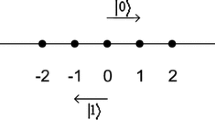
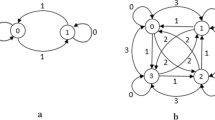
Abstract

Very recently, many tasks in quantum information processing have been achieved with coined quantum walks. In this paper, an efficient and secure quantum teleportation of shared quantum secret is presented in a quantum-walk architecture. To achieve the transfer of the quantum secret from a group of n senders to another group of m receivers, quantum walk with n walkers on the line is utilized and we adopt two different approaches: the homogeneous coin operator and the position-dependent coin operator. Furthermore, we show that none of the participants can fully access the information. This work not only opens a route to the realization of secure distributed quantum communications and computations in quantum networks, but also opens the wider application purpose of quantum walks.

Appendices

Appendices

1.1 Appendix A: The derivation process of the formula (18)

Firstly, we give the quantum state

$$\begin{aligned} \left| \varPhi ^{\prime }\right\rangle =\sum _{k=0}^{m}(\alpha \left| m_{k}\right\rangle \left| 1\right\rangle ^{\otimes (n-1)}\left| 0\right\rangle ^{\otimes n}+\beta \left| m_{k+1}\right\rangle \left| -1\right\rangle ^{\otimes (n-1)}\left| 1\right\rangle ^{\otimes n}){\textbf{P}}[|1\rangle ^{\otimes k}|0\rangle ^{\otimes (m\!-\!k)}],\nonumber \\ \end{aligned}$$

(A.1)

according to the parity of k, which can be written as: $\left| \varPhi ^{\prime }\right\rangle = \left| \varPhi ^{\prime }\right\rangle _{\text {even}} +\left| \varPhi ^{\prime }\right\rangle _{\text {odd}}$ defined by

$$\begin{aligned} \left| \varPhi ^{\prime }\right\rangle _{\text {even}}&=\sum _{k=0}^{m^{\prime }}\alpha \left| m_{2k}\right\rangle \left| 1\right\rangle ^{\otimes (n-1)}\left| 0\right\rangle ^{\otimes n}{\textbf{P}}\left[ |1\rangle ^{\otimes 2k}|0\rangle ^{\otimes (m\!-\!2k)}\right] \nonumber \\&\quad +\sum _{k=0}^{m^{\prime \prime }}\beta \left| m_{2k\!+\!2}\right\rangle \left| -1\right\rangle ^{\otimes (n-1)}\left| 1\right\rangle ^{\otimes n}{\textbf{P}}\left[ |1\rangle ^{\otimes (2k\!+\!1)}|0\rangle ^{\otimes (m\!-\!2k\!-\!1)}\right] , \end{aligned}$$

(A.2a)

$$\begin{aligned} \left| \varPhi ^{\prime \prime }\right\rangle _{\text {odd}}&=\sum _{k=0}^{m^{\prime \prime }}\alpha \left| m_{2k\!+\!1}\right\rangle \left| 1\right\rangle ^{\otimes (n-1)}\left| 0\right\rangle ^{\otimes n}{\textbf{P}}\left[ |1\rangle ^{\otimes (2k\!+\!1)}|0\rangle ^{\otimes (m\!-\!2k\!-\!1)}\right] \nonumber \\&\quad +\sum _{k=0}^{m^{\prime }}\beta \left| m_{2k+1}\right\rangle \left| -1\right\rangle ^{\otimes (n-1)}\left| 1\right\rangle ^{\otimes n}{\textbf{P}}\left[ |1\rangle ^{\otimes 2k}|0\rangle ^{\otimes (m\!-\!2k)}\right] , \end{aligned}$$

(A.2b)

where $m^{\prime }=[\frac{m}{2}]$, $m^{\prime \prime }=[\frac{m-1}{2}]$. Next, by utilizing the following equations:

$$\begin{aligned} {\textbf{P}}\left[ |1\rangle ^{\otimes 2k}|0\rangle ^{\otimes (m\!-\!2k)}\right]&={\textbf{P}}\left[ |1\rangle ^{\otimes 2k}|0\rangle ^{\otimes (m\!-\!2k\!-\!1)}\right] |0\rangle + {\textbf{P}}\left[ |1\rangle ^{\otimes 2k\!-\!1}|0\rangle ^{\otimes (m\!-\!2k)}\right] |1\rangle , \end{aligned}$$

(A.3a)

$$\begin{aligned} {\textbf{P}}\left[ |1\rangle ^{\otimes 2k\!+\!1}|0\rangle ^{\otimes (m\!-\!2k\!-\!1)}\right]&={\textbf{P}}\left[ |1\rangle ^{\otimes 2k\!+\!1}|0\rangle ^{\otimes (m\!-\!2k\!-\!2)}\right] |0\rangle + {\textbf{P}}\left[ |1\rangle ^{\otimes 2k}|0\rangle ^{\otimes (m\!-\!2k\!-\!1)}\right] |1\rangle , \end{aligned}$$

(A.3b)

the initial state $\left| \varPhi ^{\prime }\right\rangle$ can be expressed as:

$$\begin{aligned} \left| \varPhi ^{\prime }\right\rangle{} & {} =\sum _{k=0}^{m^{\prime \prime }}\alpha \left| m_{2k}\right\rangle \left| 1\right\rangle ^{\otimes (n-1)}\left| 0\right\rangle ^{\otimes n}{\textbf{P}}\left[ |1\rangle ^{\otimes 2k}|0\rangle ^{\otimes (m\!-\!2k\!-\!1)}\right] |0\rangle \nonumber \\{} & {} \qquad +\sum _{k=1}^{m^{\prime }}\alpha \left| m_{2k}\right\rangle \left| 1\right\rangle ^{\otimes (n-1)}\left| 0\right\rangle ^{\otimes n} \nonumber \\{} & {} \qquad {\textbf{P}}\left[ |1\rangle ^{\otimes (2k\!-\!1)}|0\rangle ^{\otimes (m\!-\!2k)}\right] |1\rangle +\sum _{k=0}^{m^{\prime }-1}\beta \left| m_{2k\!+\!2}\right\rangle \left| -1\right\rangle ^{\otimes (n-1)}\left| 1\right\rangle ^{\otimes n}\nonumber \\{} & {} \qquad {\textbf{P}}\left[ |1\rangle ^{\otimes (2k+1)}|0\rangle ^{\otimes (m\!-\!2k-2)}\right] |0\rangle \nonumber \\{} & {} \quad +\sum _{k=0}^{m^{\prime \prime }}\beta \left| m_{2k\!+\!2}\right\rangle \left| -1\right\rangle ^{\otimes (n-1)}\left| 1\right\rangle ^{\otimes n}{\textbf{P}}\left[ |1\rangle ^{\otimes (2k)}|0\rangle ^{\otimes (m\!-\!2k-1)}\right] |1\rangle \nonumber \\{} & {} \quad +\sum _{k=0}^{m^{\prime }\!-\!1}\alpha \left| m_{2k\!+\!1}\right\rangle \left| 1\right\rangle ^{\otimes (n-1)}\left| 0\right\rangle ^{\otimes n} \nonumber \\{} & {} \qquad {\textbf{P}}\left[ |1\rangle ^{\otimes (2k\!+\!1)}|0\rangle ^{\otimes (m\!-\!2k\!-\!2)}\right] |0\rangle +\sum _{k=0}^{m^{\prime \prime }}\alpha \left| m_{2k\!+\!1}\right\rangle \left| 1\right\rangle ^{\otimes (n-1)}\left| 0\right\rangle ^{\otimes n}\nonumber \\{} & {} \qquad {\textbf{P}}\left[ |1\rangle ^{\otimes (2k)}|0\rangle ^{\otimes (m\!-\!2k\!-\!1)}\right] |1\rangle \nonumber \\{} & {} \quad +\sum _{k=0}^{m^{\prime \prime }}\beta \left| m_{2k+1}\right\rangle \left| -1\right\rangle ^{\otimes (n-1)}\left| 1\right\rangle ^{\otimes n}{\textbf{P}}\left[ |1\rangle ^{\otimes 2k}|0\rangle ^{\otimes (m\!-\!2k\!-\!1)}\right] |0\rangle \nonumber \\{} & {} \quad +\sum _{k=1}^{m^{\prime }}\beta \left| m_{2k+1}\right\rangle \left| -1\right\rangle ^{\otimes (n-1)}\left| 1\right\rangle ^{\otimes n} \nonumber \\{} & {} \qquad {\textbf{P}}\left[ |1\rangle ^{\otimes (2k\!-\!1)}|0\rangle ^{\otimes (m\!-\!2k)}\right] |1\rangle . \end{aligned}$$

(A.4)

Then, by adjusting the order of the terms, it will become the same as the formula (18).

1.2 Appendix B

Next, we prove that any two quantum states at the receivers $\{{\varvec{{r}}}_{1}, {\varvec{{r}}}_{2},\ldots ,{\varvec{{r}}}_{m}\}$ are symmetric, that is

$$\begin{aligned} \begin{aligned} |\varPhi _{h,s}\rangle _{r}^{m}&=(-1)^{s}|1\rangle ^{\otimes ^{m\!-\!1}}_{\text {odd}}\sigma _{x}\sigma _{z}^{\omega _{h,m}}|\phi \rangle +|1\rangle ^{\otimes ^{m\!-\!1}}_{\text {even}}\sigma _{z}^{\omega _{h,m}}R_{z}(\theta _{s})|\phi \rangle \\&=(-1)^{s}\left( |1\rangle ^{\otimes ^{j\!-\!1}}_{\text {even}}\sigma _{x}\sigma _{z}^{\omega _{h,m}}|\phi \rangle _{j}|1\rangle ^{\otimes ^{m\!-\!j\!-\!1}}_{\text {odd}} +|1\rangle ^{\otimes ^{j\!-\!1}}_{\text {odd}}\sigma _{x}\sigma _{z}^{\omega _{h,m}}|\phi \rangle _{j}|1\rangle ^{\otimes ^{m\!-\!j\!-\!1}}_{\text {even}}\right) \\&\quad +|1\rangle ^{\otimes ^{j\!-\!1}}_{\text {even}}\sigma _{z}^{\omega _{h,m}}R_{z}(\theta _{s})|\phi \rangle _{j}|1\rangle ^{\otimes ^{m\!-\!j\!-\!1}}_{\text {even}} +|1\rangle ^{\otimes ^{j\!-\!1}}_{\text {odd}}\sigma _{z}^{\omega _{h,m}}R_{z}(\theta _{s})|\phi \rangle _{j}|1\rangle ^{\otimes ^{m\!-\!j\!-\!1}}_{\text {odd}}. \end{aligned}\nonumber \\ \end{aligned}$$

(B.1)

First of all, it can be derived that

$$\begin{aligned} \begin{aligned} |\varPhi _{h,s}\rangle _{r}^{m}&=|1\rangle ^{\otimes ^{m\!-\!2}}_{\text {odd}}\left[ (-1)^{s}\sigma _{x}\sigma _{z}^{\omega _{h,m}}|\phi \rangle |0\rangle +\sigma _{z}^{\omega _{h,m}}R_{z}(\theta _{s})|\phi \rangle )\right] |1\rangle \\&\quad +|1\rangle ^{\otimes ^{m\!-\!2}}_{\text {even}}\left[ (-1)^{s}(\sigma _{x}\sigma _{z}^{\omega _{h,m}}|\phi \rangle |1\rangle +\sigma _{z}^{\omega _{h,m}}R_{z}(\theta _{s})|\phi \rangle |0\rangle )\right] , \end{aligned} \end{aligned}$$

(B.2)

with the equation

$$\begin{aligned} \begin{aligned}&(-1)^{s}|a\rangle \sigma _{x}\sigma _{z}^{\omega _{h,m}}|\phi \rangle +|(a\!+\!1)\,\text {mod}\, 2\rangle \sigma _{z}^{\omega _{h,m}}R_{z}(\theta _{s})|\phi \rangle \\&=(-1)^{s}\sigma _{x}\sigma _{z}^{\omega _{h,m}}|\phi \rangle |a\rangle +\sigma _{z}^{\omega _{h,m}}R_{z}(\theta _{s})|\phi \rangle |(a\!+\!1)\,\text {mod}\, 2\rangle ,\,a=\{0,1\}, \end{aligned} \end{aligned}$$

(B.3)

which implies that the quantum states of $\{{\varvec{{r}}}_{m-1},{\varvec{{r}}}_{m}\}$ are symmetric. It is obvious that the quantum states of any two receivers of $\{{\varvec{{r}}}_{1},\ldots ,{\varvec{{r}}}_{m-1}\}$ are symmetric. Therefore, it can be concluded that any two quantum states at the receivers $\{{\varvec{{r}}}_{1}, {\varvec{{r}}}_{2},\ldots ,{\varvec{{r}}}_{m}\}$ are symmetric.

Next, after any receiver ${\varvec{{r}}}_{j}$ and the other $m\!-\!1$ receivers, respectively, performing the Pauli operator $\sigma _{z}^{\omega _{h,m}}\sigma _{x}$ and $\sigma _{z}^{\omega _{h,m}}$, it will yield

$$\begin{aligned} \begin{aligned}&|1\rangle ^{\otimes ^{j\!-\!1}}_{\text {even}}|\phi \rangle _{j}|1\rangle ^{\otimes ^{m\!-\!j\!-\!1}}_{\text {odd}} +|1\rangle ^{\otimes ^{j\!-\!1}}_{\text {odd}}|\phi \rangle _{j}|1\rangle ^{\otimes ^{m\!-\!j\!-\!1}}_{\text {even}}+(-1)^{w_{h}+s}\\&\left( |1\rangle ^{\otimes ^{j\!-\!1}}_{\text {even}}\sigma _{x}R_{z}(\theta _{s})|\phi \rangle _{j}|1\rangle ^{\otimes ^{m\!-\!j\!-\!1}}_{\text {even}} +|1\rangle ^{\otimes ^{j\!-\!1}}_{\text {odd}}\sigma _{x}R_{z}(\theta _{s})|\phi \rangle _{j}|1\rangle ^{\otimes ^{m\!-\!j\!-\!1}}_{\text {odd}}\right) , \end{aligned} \end{aligned}$$

(B.4)

which is equal to $|1\rangle ^{\otimes ^{m\!-\!1}}_{\text {odd}}|\phi \rangle +(-1)^{w_{h}+s}|1\rangle ^{\otimes ^{m\!-\!1}}_{\text {even}}\sigma _{x}R_{z}(\theta _{s})|\phi \rangle$, that can be proved by the following equation

$$\begin{aligned} \begin{aligned}&|a\rangle |\phi \rangle +(-1)^{w_{h}+s}|(a+1)\,\text {mod}\, 2\rangle \sigma _{x}R_{z}(\theta _{s})|\phi \rangle \\&=|\phi \rangle |a\rangle +(-1)^{w_{h}+s}\sigma _{x}R_{z}(\theta _{s})|\phi \rangle |(a+1)\,\text {mod}\, 2\rangle ,\,a=\{0,1\}. \end{aligned} \end{aligned}$$

(B.5)

As a result, the state $|\varPhi _{h,s}\rangle _{r}^{m}$ will become $|\varPhi _{h,s}^{\prime }\rangle _{r}^{m}$, after the proper unitary operators. Similarly, while the result on $s_{p_{1}}$ is 0s, the receivers can do the same as the result is 1t, however, which will yield

$$\begin{aligned} |\varPhi _{h,t}^{\prime }\rangle _{r}^{m}=|1\rangle ^{\otimes ^{m\!-\!1}}_{\text {even}}|\phi \rangle +(-1)^{w_{h}}|1\rangle ^{\otimes ^{m\!-\!1}}_{\text {odd}}\sigma _{x}R_{z}(\vartheta _{t})|\phi \rangle . \end{aligned}$$

(B.6)