Skip to main content

Advertisement

Springer Nature Link
Log in

The uncertainty relation for quantum channels based on skew information

  • Published:
Quantum Information Processing Aims and scope Submit manuscript

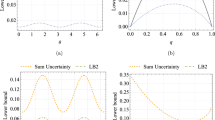
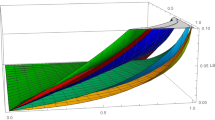
Abstract

Uncertainty relations are the basis of quantum mechanics. We study the uncertainty relation for quantum channels by skew information. An uncertainty relation for quantum channels is provided, which can be generalized to more than two channels. This uncertainty relation is shown to be independent of the Kraus representations of quantum channels. We then establish an uncertainty relation for skew information of coherence under different bases in qubit systems.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

Data availability

Data sharing not applicable to this article as no datasets were generated or analyzed during the current study.

References

  1. Heisenberg, W.: Über den anschaulichen Inhalt der quantentheoretischen Kinematik und Mechanik. Z. Phys. 43, 172 (1927)

    Article  ADS  MATH  Google Scholar 

  2. Robertson, H.P.: The Uncertainty Principle. Phys. Rev. 34, 163 (1929)

    Article  ADS  Google Scholar 

  3. Deutsch, D.: Uncertainty in quantum measurements. Phys. Rev. Lett. 50, 631 (1983)

    Article  ADS  MathSciNet  Google Scholar 

  4. Maassen, H., Uffink, J.B.M.: Generalized entropic uncertainty relations. Phys. Rev. Lett. 60, 1103 (1988)

    Article  ADS  MathSciNet  Google Scholar 

  5. Wu, S., Yu, S., Mølmer, K.: Entropic uncertainty relation for mutually unbiased bases. Phys. Rev. A. 79, 022104 (2009)

    Article  ADS  MathSciNet  Google Scholar 

  6. Coles, P.J., Berta, M., Tomamichel, M., Wehner, S.: Entropic uncertainty relations and their applications. Rev. Mod. Phys. 89, 015002 (2017)

    Article  ADS  MathSciNet  Google Scholar 

  7. Renes, J.M., Boileau, J.C.: Conjectured strong complementary information tradeoff. Phys. Rev. Lett. 103, 020402 (2009)

    Article  ADS  Google Scholar 

  8. Gour, G., Grudka, A., Horodecki, M., Kłobus, W., Łodyga, J., Narasimhachar, V.: Conditional uncertainty principle. Phys. Rev. A. 97, 042130 (2018)

    Article  ADS  Google Scholar 

  9. Grudka, A., Horodecki, M., Horodecki, P., Horodecki, R., Kłobus, W., Pankowski, Ł: Conjectured strong complementary-correlations tradeoff. Phys. Rev. A. 88, 032106 (2013)

    Article  ADS  Google Scholar 

  10. Friedland, S., Gheorghiu, V., Gour, G.: Universal uncertainty relations. Phys. Rev. Lett. 111, 230401 (2013)

    Article  ADS  Google Scholar 

  11. Puchała, Z., Rudnicki, Ł, Życzkowski, K.: Majorization entropic uncertainty relations. J. Phys. A: Math. Theor. 46, 272002 (2013)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  12. Tomamichel, M., Renner, R.: Uncertainty relation for smooth entropies. Phys. Rev. Lett. 106, 110506 (2011)

    Article  ADS  Google Scholar 

  13. Vallone, G., Marangon, D.G., Tomasin, M., Villoresi, P.: Quantum randomness certified by the uncertainty principle. Phys. Rev. A 90, 052327 (2014)

    Article  ADS  Google Scholar 

  14. Cao, Z., Zhou, H., Yuan, X., Ma, X.: Source-independent quantum random number generation. Phys. Rev. X 6, 011020 (2016)

    Google Scholar 

  15. Berta, M., Coles, P.J., Wehner, S.: Entanglement-assisted guessing of complementary measurement outcomes. Phys. Rev. A 90, 062127 (2014)

    Article  ADS  Google Scholar 

  16. Walborn, S.P., Salles, A., Gomes, R.M., Toscano, F., Ribeiro, P.H.S.: Revealing Hidden Einstein–Podolsky–Rosen nonlocality. Phys. Rev. Lett. 106, 130402 (2011)

    Article  ADS  Google Scholar 

  17. Schneeloch, J., Broadbent, C.J., Walborn, S.P., Cavalcanti, E.G., Howell, J.C.: Einstein–Podolsky–Rosen steering inequalities from entropic uncertainty relations. Phys. Rev. A 87, 062103 (2013)

    Article  ADS  Google Scholar 

  18. Giovannetti, V., Lloyd, S., Maccone, L.: Advances in quantum metrology. Nat. Photon. 5, 222 (2011)

    Article  ADS  Google Scholar 

  19. Wigner, E.P., Yanase, M.M.: Information contents of distributions. Proc. Natl. Acad. Sci. USA 49, 910 (1963)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  20. Luo, S.: Quantum versus classical uncertainty. Thero. Math. Phys. 143, 681 (2005)

    Article  MATH  Google Scholar 

  21. Zhang, L.M., Gao, T., Yan, F.L.: Tighter uncertainty relations based on Wigner–Yanase skew information for observables and channels. Phys. Lett. A. 387, 127029 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  22. Luo, S.: Wigner–Yanase skew information and uncertainty relations. Phys. Rev. Lett. 91, 180403 (2003)

    Article  ADS  Google Scholar 

  23. Luo, S., Sun, Y.: Coherence and complementarity in state-channel interaction. Phys. Rev. A. 98, 012113 (2018)

    Article  ADS  Google Scholar 

  24. Yu, C.: Quantum coherence via skew information and its polygamy. Phys. Rev. A. 95, 042337 (2017)

    Article  ADS  Google Scholar 

  25. Busch, P., Grabowski, M., Lahti, P.: Operational Quantum Physics. Springer, Berlin (1995)

    Book  MATH  Google Scholar 

  26. Nielsen, M.A., Chuang, I.L.: Quantum Computation and Quantum Information, 10th edn. Cambride University Press, Cambridge (2010)

    MATH  Google Scholar 

  27. Xu, J.: Coherence of quantum channels. Phys. Rev. A. 100, 052311 (2019)

    Article  ADS  MathSciNet  Google Scholar 

  28. Mani, A., Karimipour, V.: Cohering and decohering power of quantum channels. Phys. Rev. A. 92, 032331 (2015)

    Article  ADS  Google Scholar 

  29. Takahashi, M., Rana, S., Streltsov, A.: Creating and destroying coherence with quantum channels. Phys. Rev. A. 105, 060401 (2022)

    Article  ADS  MathSciNet  Google Scholar 

  30. Fu, S., Sun, Y., Luo, S.: Skew information-based uncertainty relations for quantum channels. Quantum Inf. Process. 18, 258 (2019)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  31. Zhang, Q.H., Wu, J.F., Fei, S.M.: A note on uncertainty relations of arbitrary N quantum channels. Laser. Phys. Lett. 18, 095204 (2021)

    Article  ADS  Google Scholar 

  32. Furuich, S., Yanagi, K., Kuriyama, K.: Trace inequalities on a generalized Wigner–Yanase skew information. J. Math. Anal. Appl. 356, 179 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  33. Fan, Y., Cao, H., Wang, W., Meng, H., Chen, L.: Uncertainty relations with the generalized Wigner–Yanase–Dyson skew information. Quantum Inf. Process. 17, 157 (2018)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  34. Streltsov, A., Adesso, G., Plenio, M.B.: Quantum coherence as a resource. Rev. Mod. Phys. 89, 041003 (2017)

    Article  ADS  MathSciNet  Google Scholar 

  35. Hu, M.L., Hu, X., Wang, J., Peng, Y., Zhang, Y.R., Fan, H.: Quantum coherence and geometric quantum discord. Phys. Rep. 762, 1 (2018)

    ADS  MathSciNet  MATH  Google Scholar 

  36. Yuan, X., Bai, G., Peng, T., Ma, X.: Quantum uncertainty relation using coherence. Phys. Rev. A. 96, 032313 (2017)

    Article  ADS  MathSciNet  Google Scholar 

Download references

Acknowledgements

M. J. Zhao is supported by the National Natural Science Foundation of China under Grants No. 12171044. Z. Wang is supported by Shandong Provincial National Natural Science Foundation under Grant No. ZR2020MA034. T. Li is supported by Projection of education and teaching, Beijing Technology and Business University, 2021.

Author information

Authors and Affiliations

  1. School of Science, Beijing Information Science and Technology University, Beijing, 100192, People’s Republic of China

    Nan Zhou & Ming-Jing Zhao

  2. School of Mathematics and Computer Application Technology, Jining University, Qufu, 273155, People’s Republic of China

    Zhen Wang

  3. School of Mathematics and Statistics, Beijing Technology and Business University, Beijing, 100048, People’s Republic of China

    Tao Li

Authors
  1. Nan Zhou
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Ming-Jing Zhao
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Zhen Wang
    View author publications

    You can also search for this author inPubMed Google Scholar

  4. Tao Li
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Ming-Jing Zhao.

Ethics declarations

Conflict of interest

The authors declare no competing interests.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhou, N., Zhao, MJ., Wang, Z. et al. The uncertainty relation for quantum channels based on skew information. Quantum Inf Process 22, 6 (2023). https://doi.org/10.1007/s11128-022-03760-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11128-022-03760-x

Keywords