Skip to main content
SpringerLink
Log in

Star network quantum steering

  • Published:
Quantum Information Processing Aims and scope Submit manuscript

Abstract

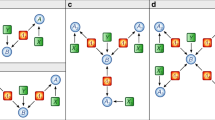
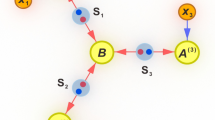
The topic of network steering in the independent-resource line network with intermediate parties being trusted and end parties being untrusted is proposed in [Jones et al. Phys Rev Lett 127(14):170405, 2021]. Where the problem that how entanglement and steerability of independent resources demonstrate network steerability was focused on deeply. In the paper, we propose the definition of quantum steering in the independent-resource star network by the corresponding local hidden state model, where the center party is untrusted and the remaining parties are trusted. Furthermore, we discover a number of kinds of relationships between entanglement (steerability) of independent resources and star network steerability.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5

Similar content being viewed by others

References

  1. Einstein, A., Podolsky, B., Rosen, N.: Can quantum-mechanical description of physical reality be considered complete? Phys. Rev. 47(10), 777 (1935)

    Article  ADS  MATH  Google Scholar 

  2. Popescu, S., Rohrlich, D.: Quantum nonlocality as an axiom. Found. Phys. 24(3), 379 (1993)

    Article  ADS  MathSciNet  Google Scholar 

  3. Barrett, J., Linden, N., Massar, S., Pironio, S., Popescu, S., Roberts, D.: Nonlocal correlations as an information-theoretic resource. Phys. Rev. A 712, 022101 (2005)

    Article  ADS  Google Scholar 

  4. Popescu, S.: Nonlocality beyond quantum mechanics. Nat. Phys. 10(4), 264 (2014)

    Article  Google Scholar 

  5. Nha, H., Carmichael, H.J.: Proposed test of quantum nonlocality for continuous variables. Phys. Rev. Lett. 93(2), 020401 (2004)

    Article  ADS  Google Scholar 

  6. Popescu, S., Rohrlich, D.: Thermodynamics and the measure of entanglemen. Phys. Rev. A 56(5), R3319 (1997)

    Article  ADS  Google Scholar 

  7. Eltschka, C., Siewert, J.: Quantifying entanglement resources. J. Phys. A Math. Theor. 47(42), 424005 (2014)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  8. Horodecki, R., Horodecki, P., Horodecki, M., Horodecki, K.: Quantum entanglement. Rev. Mod. Phys. 81(2), 865 (2009)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  9. Plenio, M.B., Virmani, S.S.: An introduction to entanglement measures. Quantum Inform. Comput. 7(1), 1 (2007)

    MathSciNet  MATH  Google Scholar 

  10. Zukowski, M., Zeilinger, A., Horne, M.A., Ekert, A.K.: Event-ready-detectors Bell experiment via entanglement swapping. Phys. Rev. Lett. 71(26), 4287 (1993)

    Article  ADS  Google Scholar 

  11. Norsen, T.: Bell locality and the nonlocal character of nature. Found. Phys. Lett. 19, 633 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  12. Chaves, R.: Polynomial bell inequalities. Phys. Rev. Lett. 116(1), 010402 (2016)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  13. Popescu, S.: Bell’s inequalities and density matrices: revealing hidden nonlocality. Phys. Rev. Lett. 74(14), 2619 (1995)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  14. Horodecki, R., Horodecki, P., Horodecki, M.: Violating Bell inequality by mixed spin-12 states: necessary and sufficient condition. Phys. Lett. A 200(5), 340 (1995)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  15. Uola, R., Costa, A.C.S., Nguyen, H.C., Gahne, O.: Quantum steering. Rev. Mod. Phys. 92(1), 015001 (2020)

    Article  ADS  MathSciNet  Google Scholar 

  16. Girdhar, P., Cavalcanti, E.G.: All two-qubit states that are steerable via Clauser-Horne-Shimony-Holt-type correlations are Bell nonlocal. Phys. Rev. A 94(3), 032317 (2016)

    Article  ADS  Google Scholar 

  17. Pan, G. Z., Zhao, J. L., Lin, Z., Yang, M., Zhang, G., Cao, Z. L.: Computable steering criterion for bipartite quantum systems. arXiv preprint arXiv:2010.01902 (2020)

  18. Branciard, C., Gisin, N., Pironio, S.: Characterizing the nonlocal correlations created via entanglement swapping. Phys. Rev. Lett. 104(17), 170401 (2010)

    Article  ADS  Google Scholar 

  19. Tavakoli, A., Skrzypczyk, P., Cavalcanti, D., Acin, A.: Nonlocal correlations in the star-network configuration. Phys. Rev. A 90(6), 062109 (2014)

    Article  ADS  Google Scholar 

  20. Rosset, D., Branciard, C., Barnea, T.J., Putz, G., Brunner, N., Gisin, N.: Nonlinear Bell inequalities tailored for quantum networks. Phys. Rev. Lett. 116(1), 010403 (2016)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  21. Krivchy, T., Cai, Y., Cavalcanti, D., Tavakoli, A., Gisin, N., Brunner, N.: A neural network oracle for quantum nonlocality problems in networks. NPJ Quantum Inf. 6(1), 1 (2020)

    Google Scholar 

  22. Armstrong, S., Wang, M., Teh, R.Y., Gong, Q., He, Q., Janousek, J., Bachor, H., Reid, M.D., Lam, P.K.: Multipartite Einstein CPodolsky CRosen steering and genuine tripartite entanglement with optical networks. Nat. Phys. 11(2), 167 (2015)

    Article  Google Scholar 

  23. Jones, B.D., Supic, I., Uola, R., Brunner, N., Skrzypczyk, P.: Network quantum steering. Phys. Rev. Lett. 127(14), 170405 (2021)

    Article  ADS  MathSciNet  Google Scholar 

  24. Ibanez, S., Li, Y.C., Chen, X., Muga, J.G.: Pulse design without the rotating-wave approximation. Phys. Rev. A 92(6), 062136 (2015)

    Article  ADS  Google Scholar 

  25. Jonckheere, E., Langbein, F.C., Schirmer, S.: Quantum networks: anti-core of spin chains. Quantum Inf. Process. 13, 1607 (2014)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  26. Van Meter, R.: Quantum networking[M]. John Wiley and Sons. (2014)

  27. Poderini, D., Agresti, I., Marchese, G., Polino, E., Giordani, T., Suprano, A., Valeri, M., Milani, G., Spagnolo, N., Carvacho, G., Chaves, R., Sciarrino, F.: Experimental violation of n-locality in a star quantum network. Nat. Commun. 11(1), 2467 (2020)

    Article  ADS  Google Scholar 

  28. Munshi, S., Kumar, R., Pan, A.K.: Generalized n-locality inequalities in a star-network configuration and their optimal quantum violations. Phys. Rev. A 104(4), 042217 (2021)

    Article  ADS  MathSciNet  Google Scholar 

  29. Tavakoli, A., Renou, M.O., Gisin, N., Brunner, N.: Correlations in star networks: from Bell inequalities to network inequalities. New J. Phys. 19(7), 073003 (2017)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  30. Andreoli, F., Carvacho, G., Santodonato, L., Chaves, R., Sciarrino, F.: Maximal qubit violation of n-locality inequalities in a star-shaped quantum network. New J. Phys. 19(11), 113020 (2017)

    Article  ADS  MATH  Google Scholar 

  31. Chitambar, E., Gour, G.: Quantum resource theories. Rev. Modern phys. 91(2), 025001 (2019)

    Article  ADS  MathSciNet  Google Scholar 

  32. Zhen, Y.Z., Xu, X.Y., Li, L., Liu, N.L., Chen, K.: The Einstein-Podolsky-Rosen steering and its certification. Entropy 21(4), 422 (2019)

    Article  ADS  MathSciNet  Google Scholar 

  33. Zhang, K., Wang, J.: Asymmetric steerability of quantum equilibrium and nonequilibrium steady states through entanglement detection. Phys. Rev. A 104(4), 042404 (2021)

    Article  ADS  MathSciNet  Google Scholar 

  34. Quintino, M.T., Brunner, N., Huber, M.: Superactivation of quantum steering. Phys. Rev. A 94(6), 062123 (2016)

    Article  ADS  Google Scholar 

  35. Jones, B.D., Supic, I., Uola, R., Brunner, N., Skrzypczyk, P.: Supplemental material network quantum steering. Phys. Rev. Lett. 127, 1704051–1704056 (2021). https://doi.org/10.1103/PhysRevLett.127.170405

    Article  Google Scholar 

Download references

Acknowledgements

Thanks for comments. The work was supported by the National Natural Science Foundation of China [Grant No. 12271394] and the Key Research and Development Program of Shanxi Province [Grant No. 202102 010101004].

Author information

Authors and Affiliations

  1. College of Mathematics, Taiyuan University of Technology, Taiyuan, 030024, People’s Republic of China

    Huijie Zhang, Shuyuan Yang & Kan He

Authors
  1. Huijie Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Shuyuan Yang
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Kan He
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Kan He.

Ethics declarations

Conflict of interest

The authors declare no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhang, H., Yang, S. & He, K. Star network quantum steering. Quantum Inf Process 22, 286 (2023). https://doi.org/10.1007/s11128-023-04042-w

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11128-023-04042-w

Keywords

Search

Navigation