Skip to main content
Springer Nature Link
Log in

Many-body localization transition of disordered Heisenberg XXX spin-1 chains

  • Published:
Quantum Information Processing Aims and scope Submit manuscript

Abstract

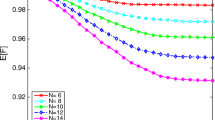
In this paper, the properties of many-body localization (MBL) in one-dimensional disordered Heisenberg XXX spin-1 chains are studied theoretically by using the methods of exact matrix diagonalization. We compare it with the MBL properties of the Heisenberg spin-1/2 chains. We first study properties of the eigenstates of the model through the excited-state fidelity. By analyzing the inflection point of excited-state fidelity curves, we can roughly determine the critical point of MBL phase transition. Moreover, for the case of random disorder, we calculated the bipartite entanglement entropy, and the critical points obtained from the intersection of curves for different systems sizes were basically consistent with those obtained from excited-state fidelity. Then we study the dynamical properties of the model by the dynamical behavior of diagonal entropy (DE), local magnetization and the time evolution of fidelity to further prove the occurrence of MBL phase transition in the disordered Heisenberg XXX spin-1 chain and distinguish the ergodic phase (thermal phase) and the many-body localized phase. We can illustrate that in the localized phase, the information of the initial state can be well protected if the disorder strength of the system is large enough. There are various forms of disorder, and we compare the effects of different forms of quasi-disorder and random disorder on MBL in this article. We also investigate the effect of non-uniform disorder external field on MBL. Our results reveal that disorder can cause the occurrence of MBL in the one-dimensional disordered Heisenberg XXX spin-1 chains. Furthermore, the form of disorder, the properties of the spin, and the size of the system all affect the critical point of the MBL phase transition.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

Data availability statement

We guarantee that all data and materials support our published claims and comply with field standards.

References

  1. Anderson, P.W.: Absence of diffusion in certain random lattices. Phys. Rev. Lett. 109, 1492 (1958)

    ADS  Google Scholar 

  2. Billy, J., et al.: Direct observation of Anderson localization of matter waves in a controlled disorder. Nature 453, 891–894 (2008)

    ADS  Google Scholar 

  3. Basko, D.M., Aleiner, I.L., Altshuler, B.L.: Metal-insulator transition in a weakly interacting many-electron system with localized single-particle states. Ann. Phys. 321, 1126–1205 (2006)

    ADS  Google Scholar 

  4. Safavi-Naini, A., Wall, M.L., Acevedo, O.L., Rey, A.M., Nandkishore, R.M.: Quantum dynamics of disordered spin chains with power-law interactions. Phys. Rev. A 99, 033610 (2019)

    ADS  Google Scholar 

  5. Macieszczak, K., Levi, E., Macri, T., Lesanovsky, I., Garrahan, J.P.: Coherence, entanglement, and quantumness in closed and open systems with conserved charge, with an application to many-body localization. Phys. Rev. A 99, 052354 (2019)

  6. Strek, W., Cichy, B., Radosinski, L., Gluchowski, P., Marciniak, L., Lukaszewicz, M., Hreniak, D.: Laser-induced white-light emission from graphene ceramics-opening a band gap in graphene. Light Sci. Appl 4, e237 (2015)

    ADS  Google Scholar 

  7. Ponte, P., Papić, Z., Huveneers, F., Abanin, D.A.: Many-body localization in periodically driven systems. Phys. Rev. Lett. 114, 140401 (2015)

    ADS  Google Scholar 

  8. Dutt, A., Minkov, M., Williamson, I.A.D., Fan, S.: Higher-order topological insulators in synthetic dimensions. Light Sci. Appl. 9, 131 (2020)

    ADS  Google Scholar 

  9. Chanda, T., Sierant, P., Zakrzewski, J.: Time dynamics with matrix product states: Many-body localization. arXiv:1908.06524

  10. Doggen, E.V.H., Schindler, F., Tikhonov, K.S., Mirlin, A.D., Neupert, T., Polyakov, D.G., Gornyi, I.V.: Many-body localization and delocalization in large quantum chains. Phys. Rev. B 98, 174202 (2018)

    ADS  Google Scholar 

  11. Doggen, E.V.H., Mirlin, A.D.: Many-body delocalization dynamics in long Aubry-André quasiperiodic chains. Phys. Rev. B 100, 104203 (2019)

    ADS  Google Scholar 

  12. Sierant, P., Zakrzewski, J.: Many-body localization of bosons in optical lattices. New J. Phys 20, 043032 (2018)

    ADS  Google Scholar 

  13. Kjáll, J.A., Bardarson, J.H., Pollmann, F.: Many-Body Localization in a Disordered Quantum Ising Chain. Phys. Rev. Lett. 113, 107204 (2014)

    ADS  Google Scholar 

  14. Luitz, D.J., Laflorencie, N., Alet, F.: Many-body localization edge in the random-field Heisenberg chain. Phys. Rev. B 91, 081103 (2015)

    ADS  Google Scholar 

  15. Elliott, B., Lim, S.P., Sheng, D.N.: Many-body localization and mobility edge in a disordered spin-12 Heisenberg ladder. Phys. Rev. B 2, 195153 (2015)

    Google Scholar 

  16. Bardarson, J.H., Pollmann, F., Moore, J.E.: Unbounded Growth of Entanglement in Models of Many-Body Localization. Phys. Rev. Lett. 109, 017202 (2012)

    ADS  Google Scholar 

  17. Serbyn, M., Papic, Z., Abanin, D.A.: Universal Slow Growth of Entanglement in Interacting Strongly Disordered Systems. Phys. Rev. Lett. 110, 260601 (2013)

    ADS  Google Scholar 

  18. Luitz, D.J., Laflorencie, N., Alet, F.: Extended slow dynamical regime close to the many-body localization transition. Phys. Rev. B 93, 060201(R) (2016)

    ADS  Google Scholar 

  19. Pino, M.: Entanglement growth in many-body localized systems with long-range interactions. Phys. Rev. B 90, 174204 (2014)

    ADS  Google Scholar 

  20. Deng, D.-L., Li, X., Pixley, J.H., Wu, Y.-L., Das Sarma, S.: Logarithmic entanglement lightcone in many-body localized systems. Phys. Rev. B 95, 024202 (2017)

    ADS  Google Scholar 

  21. Xu, K., Chen, J.-J., Zeng, Y., Zhang, Y.-R., Song, C., Liu, W., Guo, Q., Zhang, P., Xu, D., Deng, H., Huang, K., Wang, H., Zhu, X., Zheng, D., Fan, H.: Emulating Many-Body Localization with a Superconducting Quantum Processor. Phys. Rev. Lett. 120, 050507 (2018)

    ADS  Google Scholar 

  22. Torres-Herrera, E.J., Santos, L.F.: Extended nonergodic states in disordered many-body quantum systems. Ann. Phys. (Berlin) 529, 1600284 (2017)

  23. Bauer, B., Nayak, C.: Area laws in a many-body localized state and its implications for topological order, J. Stat. Mech. 2013 (2013)

  24. Huse, D.A., Nandkishore, R., Oganesyan, V., Pal, A., Sondhi, S.L.: Localization-protected quantum order. Phys. Rev. B 88, 014206 (2013)

    ADS  Google Scholar 

  25. Rubin, S., Hong, B., Fainman, Y.: Subnanometer imaging and controlled dynamical patterning of thermocapillary driven deformation of thin liquid films. Light Sci. Appl 8, 77 (2019)

    Google Scholar 

  26. Wang, Hanteng, Yeh, Hsiu-Chung., Kamenev, Alex: Many-body localization enables iterative quantum optimization. Nature Communications 13, 5503 (2022)

    ADS  Google Scholar 

  27. Qu, Y., Li, Q., Lu, C., Pan, M., Ghosh, P., Du, K., Qiu, M.: Thermal camouflage based on the phase- changing material GST. Light Sci. Appl. 7, 26 (2018)

    ADS  Google Scholar 

  28. Pal, A., Huse, D.A.: Many-body localization phase transition. Phys. Rev. B 82, 174411 (2010)

    ADS  Google Scholar 

  29. Potter, A.C., Vasseur, R., Parameswaran, S.A.: Universal properties of many-body delocalization transitions. Phys. Rev. X 5, 031033 (2015)

    Google Scholar 

  30. Petsch, S., Schuhladen, S., Dreesen, L., Zappe, H.: The engineered eyeball, a tunable imaging system using soft-matter micro-optics. Light Sci. Appl. 5, e16068 (2016)

    ADS  Google Scholar 

  31. Zhang, S.X., Yao, H.: Universal properties of many-body localization transitions in quasiperiodic systems. Phys. Rev. Lett. 121, 206601 (2018)

    ADS  Google Scholar 

  32. Biasco, S., Beere, H.E., Ritchie, D.A., Li, L., GilesDavies, A., Linfield, E.H., Vitiello, M.S.: Frequency- tunable continuous-wave random lasers at terahertz frequencies. Light Sci. Appl. 8, 43 (2019)

    ADS  Google Scholar 

  33. Canovi, E., Rossini, D., Fazio, R., Santoro, G.E., Silva, A.: Quantum quenches, thermalization, and many-body localization. Phys. Rev. B 83, 094431 (2011)

    ADS  Google Scholar 

  34. Serbyn, M., Papić, Z., Abanin, D.A.: Criterion for many-body localization-delocalization phase transition. Phys. Rev. X 5, 041047 (2015)

    Google Scholar 

  35. Bairey, E., Refael, G., Lindner, N.H.: Driving induced many-body localization. Phys. Rev. B 96, 020201(R) (2017)

    ADS  Google Scholar 

  36. Ponte, P., Chandran, A., Papic, Z., Abanin, D.A.: Periodically driven ergodic and many-body localized quantum systems. Ann. Phys. 353, 196–204 (2015)

    MathSciNet  ADS  Google Scholar 

  37. Etezadi, D., Warner, J.B.I.V., Ruggeri, F.S., Dietler, G., Lashuel, H.A., Altug, H.: Nanoplasmonic mid- infrared biosensor for in vitro protein secondary structure detection. Light Sci. Appl. 6, e17029 (2017)

    ADS  Google Scholar 

  38. Kubo, Kenn: Spin correlations in the S= 1 XXZ chain. Phys. Rev. B 42, 2 (1992)

    Google Scholar 

  39. Capponi, S., Dupont, M., Sandvik, A.W., Sengupta, P.: NMR relaxation in the spin-1 Heisenberg chain. Phys. Rev. B 100, 094411 (2019)

    ADS  Google Scholar 

  40. Malvezzi, A.L., et al.: Quantum correlations and coherence in spin-1 Heisenberg chains. Phys. Rev. B 93, 184428 (2016)

    ADS  Google Scholar 

  41. Romero-Isart, O., Eckert, K., Sanpera, A.: Quantum state transfer in spin-1 chains. Phys. Rev. A 75, 050303(R) (2007)

    MathSciNet  ADS  Google Scholar 

  42. Suzuki, T., Suga, S.: Quantized excitation spectra by magnon confinement in quasi-one-dimensional S = 1 spin system. Phys. Rev. B 98, 180406(R) (2018)

    ADS  Google Scholar 

  43. Aneck, I., Kennedy, T., Lich, E.H., Tasaki, H.: Rigorous results on valence-bond ground states in antiferromagnets. Phys. Rev. Lett. 57, 7 (1987)

    Google Scholar 

  44. Sen, D., Surendran, N.: Spin-1 chain with spin-1/2 excitations in the bulk. Phys. Rev. B 75, 104411 (2007)

    ADS  Google Scholar 

  45. Zhang, Y., et al.: Localization, multifractality, and many-body localization in periodically kicked quasiperiodic lattices. Phys. Rev. B 106, 054312 (2022)

    ADS  Google Scholar 

  46. Lee, M., Look, T.R., Lim, S.P., Sheng, D.N.: Many-body localization in spin chain systems with quasiperiodic fields. Phys. Rev. B 96, 075146 (2017)

    ADS  Google Scholar 

  47. Khemani, V., Sheng, D.N., Huse, D.A.: Two universality classes for the many-body localization transition. Phys. Rev. Lett. 119, 075702 (2017)

    ADS  Google Scholar 

  48. Setiawan, F., Deng, D.-L., Pixley, J.H.: Transport properties across the many-body localization transition in quasiperiodic and random systems. Phys. Rev. B 96, 104205 (2017)

    ADS  Google Scholar 

  49. Ghosh, S., Gidugu, J., Mukerjee, S.: Transport in the nonergodic extended phase of interacting quasiperiodic systems. Phys. Rev. B 102, 224203 (2020)

    ADS  Google Scholar 

  50. Iyer, S., Oganesyan, V., Refael, G., Huse, D.A.: Many-body localization in a quasiperiodic system. Phys. Rev. B 87, 134202 (2013)

    ADS  Google Scholar 

  51. Bloch, I., Dalibard, J., Zwerger, W.: Many-body physics with ultracold gases. Rev. Mod. Phys. 80, 885 (2008)

    ADS  Google Scholar 

  52. Roati, G., et al.: Anderson localization of a non-interacting Bose-Einstein condensate. Nature 453, 895–898 (2008)

    ADS  Google Scholar 

  53. Bordia, Pranjal, et al.: Coupling Identical one-dimensional many-body localized systems. Phys. Rev. Lett. 116, 140401 (2016)

    ADS  Google Scholar 

  54. Aramthottil, A.S., Chanda, T., Sierant, P., Zakrzewski, J.: Finite-size scaling analysis of the many-body localization transition in quasiperiodic spin chains. Phys. Rev. B 104, 214201 (2021)

    ADS  Google Scholar 

  55. Gu, S.-J.: Fidelity approach to quantum phase transitions. Int. J. Modern Phys. B 24, 4371–4458 (2010)

    MathSciNet  ADS  Google Scholar 

  56. Zanardi, Paolo, Giorda, Paolo, Cozzini, Marco: Information-theoretic differential geometry of quantum phase transitions. Phys. Rev. Lett. 99, 100603 (2007)

    ADS  Google Scholar 

  57. Albuquerque, A.F., Alet, F., Sire, C., Capponi, S.: Quantum critical scaling of fidelity susceptibility. Phys. Rev. B 81, 064418 (2010)

    ADS  Google Scholar 

  58. Sun, G.Y., Wei, B.B., Kou, S.P.: Fidelity as a probe for a deconfined quantum critical point. Phys. Rev. B 100, 064427 (2019)

    ADS  Google Scholar 

  59. Hu, T.T., Xue, K., Li, X.D., Zhang, Y., Ren, H.: Fidelity of the diagonal ensemble signals the many-body localization transition. Phys. Rev. E 94, 052119 (2016)

    ADS  Google Scholar 

  60. Hu, T.T., Xue, K., Li, X., Zhang, Y., Ren, H.: Excited-state fidelity as a signal for the many-body localization transition in a disordered Ising chain. Sci. Rep. 7, 1–8 (2017)

    Google Scholar 

Download references

Acknowledgements

This work was supported by the Plan for Scientific and Technological Development of Jilin Province (No. 20230101018JC).

Author information

Authors and Affiliations

  1. School of Physics, Northeast Normal University, Changchun, 130024, People’s Republic of China

    Taotao Hu, Yiwen Gao, Yining Zhang, Jiameng Hong, Yuting Li & Dongyan Guo

  2. College of Science, University of Shanghai for Science and Technology, Shanghai, 200093, People’s Republic of China

    Xiaodan Li

Authors
  1. Taotao Hu
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Yiwen Gao
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Yining Zhang
    View author publications

    You can also search for this author inPubMed Google Scholar

  4. Jiameng Hong
    View author publications

    You can also search for this author inPubMed Google Scholar

  5. Xiaodan Li
    View author publications

    You can also search for this author inPubMed Google Scholar

  6. Yuting Li
    View author publications

    You can also search for this author inPubMed Google Scholar

  7. Dongyan Guo
    View author publications

    You can also search for this author inPubMed Google Scholar

Contributions

Taotao Hu contributed the idea. Yiwen Gao, Yining Zhang, Jiameng Hong performed the calculations and prepared the figures. Yiwen Gao, Taotao Hu wrote the main manuscript. Xiaodan Li, Yuting Li and Dongyan Guo checked the calculations, and Taotao Hu improved the manuscript. All authors contributed to discussions and reviewed the manuscript.

Corresponding author

Correspondence to Taotao Hu.

Ethics declarations

Conflict of interest

The authors declare that they have no Conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Hu, T., Gao, Y., Zhang, Y. et al. Many-body localization transition of disordered Heisenberg XXX spin-1 chains. Quantum Inf Process 23, 142 (2024). https://doi.org/10.1007/s11128-024-04332-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11128-024-04332-x

Keywords