Improving the performance of practical phase-matching quantum key distribution with advantage distillation

Abstract

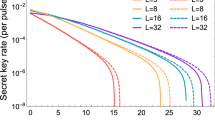
Phase-matching quantum key distribution (PM-QKD) is a promising protocol that can surpass the linear key-rate bound. Generally, inserting an advantage distillation (AD) step into PM-QKD can improve the secure key rate and transmission distance significantly in the asymptotic case. Here, we investigate the performance of practical PM-QKD systems with AD in the non-asymptotic case. Simulation results show that AD can dramatically improve the performance of practical PM-QKD systems. Moreover, we propose to estimate the information leakage using three mutually unbiased bases, namely X, Y and Z, to further enhance its performance, which demonstrates that using the error rates in X, Y and Z bases to estimate the information leakage can improve the secure key rate at high channel losses and misalignment errors.

Appendix

In general, the prepare-and-measure QKD is equivalent to an entanglement-based scheme, where Alice prepares the quantum state $\frac{1}{\sqrt{2} } \left( \mid {00} \rangle + \mid {11} \rangle \right)$, she keeps the first particle and sends the other to Bob through the quantum channel. After that, Alice and Bob take inputs from ${H_A} \otimes {H_B}$, where both ${H_A}$ and ${H_B}$ are two-dimensional Hilbert spaces, to apply the measurements of the Z, X and Y bases (the Z basis consists of $\mid {0} \rangle$ and $\mid {1} \rangle$, the X basis consists of $\mid + \rangle = \frac{1}{{\sqrt{2} }}\left( {\mid 0 \rangle + \mid 1 \rangle } \right)$ and $\mid - \rangle = \frac{1}{{\sqrt{2} }}\left( {\mid 0 \rangle - \mid 1 \rangle } \right)$, and the Y basis consists of $\mid +i \rangle = \frac{1}{{\sqrt{2} }}\left( {\mid 0 \rangle + i\mid 1 \rangle } \right)$ and $\mid -i \rangle = \frac{1}{{\sqrt{2} }}\left( {\mid 0 \rangle - i\mid 1 \rangle } \right)$). Because the quantum channel can be completely controlled by the eavesdropper Eve, Alice and Bob finally share the quantum state ${\sigma _{AB}} = \sum \nolimits _{i = 0}^3 {{\lambda _i}} \mid {{\Phi _i}} \rangle \langle {{\Phi _i}}\mid$, where ${\lambda _i}$ is the diagonal value of $\sigma _{AB}$ in the Bell basis and $\sum \nolimits _{i = 0}^3 {{\lambda _i}} = 1$. Note that, the Bell basis has the form $\mid {{\Phi _0}} \rangle = \frac{1}{{\sqrt{2} }}\left( {\mid { 0 0 } \rangle + \mid { 1 1 } \rangle } \right)$, $\mid {{\Phi _1}} \rangle = \frac{1}{{\sqrt{2} }}\left( {\mid { 0 0 } \rangle - \mid { 1 1 } \rangle } \right)$, $\mid {{\Phi _2}} \rangle = \frac{1}{{\sqrt{2} }}\left( {\mid { 0 1 } \rangle + \mid { 1 0 } \rangle } \right)$, and $\mid {{\Phi _3}} \rangle = \frac{1}{{\sqrt{2} }}\left( {\mid { 0 1 } \rangle - \mid { 1 0 } \rangle } \right)$. It is clear that the error rates in the Z, X and Y bases satisfy $\lambda _{2} + \lambda _{3} = E^{Z}$, $\lambda _{1} + \lambda _{3} = E^{X}$ and $\lambda _{1} + \lambda _{2} = E^{Y}$, respectively. Note that, Eve can choose the optimal $\lambda _{i}$ freely to eavesdrop if $\lambda _{i}$ is constrained by these error rates. And the final secret key rate between Alice and Bob can be expressed as [32]

$$\begin{aligned} \begin{aligned} { R \ge }&{ \mathop {\min }\limits _{{\lambda _0},{\lambda _1},{\lambda _2},{\lambda _3}}} { \left[ 1 - ({\lambda _0} + {\lambda _1})H(\frac{{{\lambda _0}}}{{{\lambda _0} + {\lambda _1}}}) \right. }\\&{ \left. - ({\lambda _2} + {\lambda _3})H \left( \frac{{{\lambda _2}}}{{{\lambda _2} + {\lambda _3}}} \right) - H({\lambda _2} + {\lambda _3}) \right] }, \end{aligned} \end{aligned}$$

(A1)

where $H(x)=-x{\log _2}x-(1-x){\log _2}(1-x)$ is the binary Shannon entropy function.

To improve the performance of QKD, Ref. [32] proposed the AD method, which can increase the correlations of raw keys between Alice and Bob. The procedure of AD is given as follows:

Step 1 Alice (Bob) splits her (his) raw key bits into blocks of size b, denoted by $\{ x_{1}, x_{2}, \ldots , x_{b} \} (\{ y_{1}, y_{2}, \ldots , y_{b} \})$.

Step 2 Alice chooses a bit $r\in \{ 0, 1 \}$ randomly, then sends the message $c = \{ c_{1}, c_{2}, \ldots , c_{b} \} = \{ x_{1}\oplus r, x_{2}\oplus r, \ldots , x_{b}\oplus r \}$ to Bob through an authenticated classical channel.

Step 3 Bob calculates the result of $\{ d_{1}, d_{2}, \ldots , d_{b}\} = \{ c_{1}\oplus y_{1}, c_{2}\oplus y_{2}, \ldots , c_{b}\oplus y_{b} \}$ to Alice. If the block $\{ d_{1}, d_{2}, \ldots , d_{b} \}$ is $\{ 0, 0, \ldots , 0 \}$ or $\{ 1, 1, \ldots , 1 \}$, they continue to step 4. Otherwise, the procedure of AD ends.

Step 4 Alice and Bob keep the first bit of their initial block (specially, $x_{1}$ and $y_{1}$) as their raw keys.

It is apparent that the successful probability of the AD method on the block of size b is $p_\textrm{succ} = (\lambda _{0} + \lambda _{1})^{b} + (\lambda _{2} + \lambda _{3})^{b}$, and the final quantum state shared between Alice and Bob is ${\tilde{\sigma }_{AB}} = \sum \nolimits _{i = 0}^3 {{\tilde{\lambda }_i}} \mid {{\Phi _i}} \rangle \langle {{\Phi _i}}\mid$, where ${{{\tilde{\lambda }}_0}=\frac{{{{({\lambda _0}+{\lambda _1})}^b}+{{({\lambda _0}-{\lambda _1})}^b}}}{2p_\textrm{succ}},}$ ${{{\tilde{\lambda }}_1}=\frac{{{{({\lambda _0}+{\lambda _1})}^b}-{{({\lambda _0}-{\lambda _1})}^b}}}{2p_\textrm{succ}},}$ ${{{\tilde{\lambda }}_2}=\frac{{{{({\lambda _2}+{\lambda _3})}^b}+{{({\lambda _2}-{\lambda _3})}^b}}}{2p_\textrm{succ}},}$ and ${{{\tilde{\lambda }}_3}=\frac{{{{({\lambda _2}+{\lambda _3})}^b}-{{({\lambda _2}-{\lambda _3})}^b}}}{2p_\textrm{succ}}.}$ Consequently, the final secret key rate of QKD with AD is [32]

$$\begin{aligned} \begin{aligned} {R \ge }&{ \mathrm{{ }}\mathop {\max }\limits _b \mathop {\min }\limits _{{\lambda _0},{\lambda _1},{\lambda _2},{\lambda _3}} \frac{1}{b}{p_\textrm{succ}} \left[ 1 - ({{\tilde{\lambda }}_0} + {{\tilde{\lambda }}_1})H\left( {\frac{{{{\tilde{\lambda }}_0}}}{{{{\tilde{\lambda }}_0} + {{\tilde{\lambda }}_1}}}} \right) \right. } \\&{ \left. - ({{\tilde{\lambda }}_2} + {{\tilde{\lambda }}_3})H\left( {\frac{{{{\tilde{\lambda }}_2}}}{{{{\tilde{\lambda }}_2} + {{\tilde{\lambda }}_3}}}} \right) - H\left( {{{\tilde{\lambda }}_0} + {{\tilde{\lambda }}_1}} \right) \right] }. \end{aligned} \end{aligned}$$

(A2)

From the procedure of AD and Eq. A2, it is clear that Alice and Bob can choose the optimal value b to maximize the final secret key rate.